Unit 6
Quantum Mechanics
A particlehas mass, it is located at some definite point, it can move from one place to another, it gives energy when slowed down or stopped. Thus, the particle is specified by
(1) Mass m
(2) Velocity v
(3) Momentum p and
(4) Energy E.
A waveis spread out over a relatively large region of space, it cannot be said to be located just here and there, it is hard to think of mass being associated with a wave. Actually, a wave is nothing but a rather spread out disturbance. A wave is specified by its
(1) Frequency
(2) Wavelength,
(3) Phase of wave velocity,
(4) Amplitude and
(5) Intensity
Radiation is a wave which is spread out over space and also a particle which is localized at a point in space.
However, this acceptance is essential because radiation sometimes behaves as a wave and at other times as a particle as explained below:
(1) Radiations including visible light, infra-red, ultraviolet, X-rays, etc. behave as waves in experiments based on interference, diffraction, etc. This is due to the fact that these phenomena require the presence of two waves at the same position at the same time. Obviously, it is difficult for the two particles to occupy the same position at the same time. Thus, we conclude that radiations behave like wave.
(2) Planck’s quantum theory was successful in explaining black body radiation, the photo electric effect, the Compton Effect, etc. and had clearly established that the radiant energy, in its interaction with matter, behaves as though it consists of corpuscles. Here radiation interacts with matter in the form of photon or quanta. Thus, we conclude that radiations behave like particle.
Louis de- Broglie in 1924 extended the wave particle parallelism of light radiations to all the fundamental entities of Physics such as electrons, protons, neutrons, atoms and molecules etc. He put a bold suggestion that the correspondence between wave and particle should not confine only to electromagnetic radiation, but it should also be valid for material practices, i.e. like radiation, matter also has a dual (i.e., particle like and wave like) character.
A moving particle is always associated with the wave and the particle is controlled by waves. This suggestion was based on the fact that nature loves symmetry, if radiation like light can act like wave some times and like a particle at other times, then the material particles (e.g., electron, neutron, etc.) should act as waves at some other times. These waves associated with particles are named de- Broglie waves or matter waves.
Expression for de- Broglie wavelength
The expression of the wavelength associated with a material particle can be derived on the analogy of radiation as follows:
Considering the plank’s theory of radiation, the energy of photon (quantum) is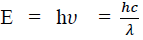 
Where c is the velocity of light in vacuum and is its wave length.
According to Einstein energy – mass relation
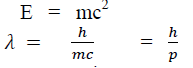
Where mc = p is momentum associated with photon.
If we consider the case of material particle of mass m and moving with a velocity v, i.e. momentum mv, then the wave length associated with this particle (in analogy to wave length associated with photon) is given by
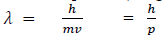
Different expressions for de-Broglie wavelength
(a) If E is the kinetic energy of the material particle then
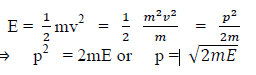
There for de Broglie wavelength is—
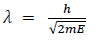
(b) When a charged particle carrying a charge ‘q’ is accelerated by potential difference v, then its kinetic energy K.E is given by
E = qV
Hence the de-Broglie wavelength associated with this particle is
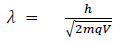
For an electron
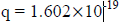
Properties of Matter Waves
(a) Lighter is the particle, greater is the wavelength associated with it.
(b) Smaller is the velocity of the particle, greater is the wavelength associated with it.
(c) When v = 0, then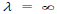
i.e. wave becomes indeterminate and if v =∞, then
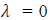. This shows that matter waves are generated only when material particles are in motion.
(d) Matter waves are produced whether the particles are charged particles or not
i.e., matter waves are not electromagnetic waves but they are a new kind of waves.
(e) It can be shown that the matter waves can travel faster than light i.e. the velocity of matter waves can be greater than the velocity of light.
(f) No single phenomenon exhibits both particle nature and wave nature simultaneously
Distinction between matter waves and electromagnetic waves
S.no. | Matter wave | Electromagnetic wave | ||
1
2
3
4
5 |
Matter wave require medium for propagation i.e., they cannot travel through vacuum. | Electromagnetic waves are produced only by accelerated charged particles
Wavelength depends on the energy of photon
Travel with velocity of light c= 3×108 m/s
Electric field and magnetic field oscillateperpendicular to each other.
Electromagnetic waves do not require medium |
Statement: It is impossible to determine simultaneously both the position and momentum of a quantum mechanical particle moving inside the wave packet with equal accuracy. In any simultaneous determination of the position and momentum of a particle, the product of the corresponding uncertainties inherently present in the measurement is equal to, greater than (h/4π).
Explanation: Consider a quantum particle moving inside a wave packet of width Δx. Then inside the wave packet it is not possible to find the exact position of the particle and any attempt to measure it will have an uncertainty (error) which less than or equal to Δx.
The maximum uncertainty involved in measuring the position of the particle within the wave packet is Δx. Since position cannot be measured accurately, there will be an uncertainty in measuring momentum also. According to Heisenberg’s uncertainty principle, the product of uncertainty involved in the measurement of these two quantities is given by the relation,
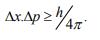
Where ∆x = uncertainty in position.
∆p = uncertainty in momentum.
It can be applied to any conjugate physical quantities such as energy and time, angular position and angular momentum etc.
Other forms of Uncertainties are:
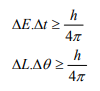
Where, ΔE, Δt are the uncertainties in the measurement of energy and time, and ΔL, Δθ are the uncertainties in the measurement of angular momentum and angular position.
In 1922, Arthur Compton studied the scattering of x-rays of known frequency from graphite and looked at the recoil electrons and the scattered x-rays.
According to wave theory, when an electromagnetic wave of frequency 
is incident on an atom, it would cause electrons to oscillate. The electrons would absorb energy from the wave and re-radiate electromagnetic wave of a frequency
. The frequency of scattered radiation would depend on the amount of energy absorbed from the wave, i.e. on the intensity of incident radiation and the duration of the exposure of electrons to the radiation and not on the frequency of the incident radiation. Compton found that the wavelength of the scattered radiation does not depend on the intensity of incident radiation but it depends on the angle of scattering and the wavelength of the incident beam. The wavelength of the radiation scattered at an angle θ is given by-
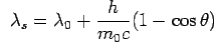
Where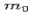 
is the rest mass of the electron
The constant 
is known as the Compton wavelength of the electron and it has a value 0.0024 nm. The spectrum of radiation at an angle θ consists of two peaks, one at
and the other at
. Compton effect can be explained by assuming that the incoming radiation is a beam of particles with
- Energy-
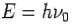
- Momentum –
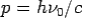
Consider a photon of energy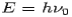
and momentum 
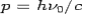colliding elastically with an electron at rest. Let the direction of incoming photon be along the x-axis. After scattering, the photon moves along a direction making an angle θ with the x-axis while the scattered electron moves making an angle φ. Let the magnitude of the momentum
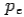of the scattered electron be while that of the
Scattered photon be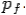
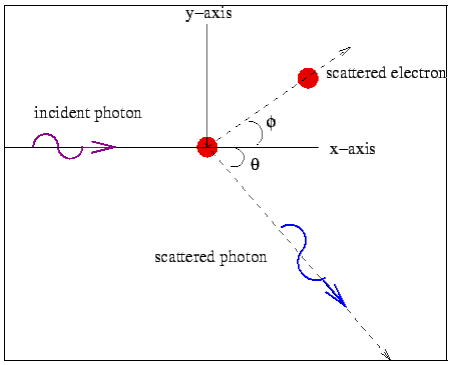
Conservation of momentum:
X- direction—
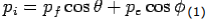---------Eq 1
Y- direction—
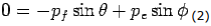---------Eq 2
From eq. 1 and 2—
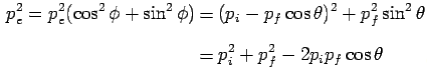---------Eq 3
Conservation of Energy: ---
If the rest mass of electron is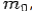 
and the initial energy is 
and the final energy is
Then-
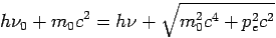--------Eq.4
On squaring Eq 4 we get--
On substitute Eq 3 for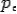 
we get—
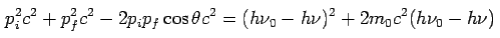
Put the value of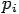 
and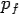 
we get—
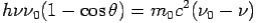
By using 
We get Compton formula-
-
Here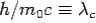 
is known as Compton wavelength
