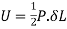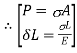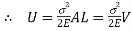Unit 6
Strain Energy
- Strain energy
When a body is subjected to gradual, sudden or impact load, the body deforms and work is done upon it. If the elastic limit is not exceeding, this work is stored in the body. This work done or energy stored in the body is called Strain Energy.
Energy is stored in the body during deformation process and this energy is called “Strain Energy”.
Strain Energy = Work done
- Resilience
Total strain energy stored in a body called resilience.
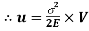where = stress and V = Volume of the body
- Proof Resilience:
Maximum strain energy which can be stored in a body is called proof resilience.
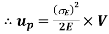where, 
Stress at elastic limit
- Modulus Resilience:
Maximum strain energy which can be stored in a body per unit volume, at elastic limit is called modulus of resilience.
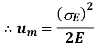
(1) Strain Energy due to gradually applied loading:
Consider a bar length L placed vertically and one end of it is attached at the ceiling.
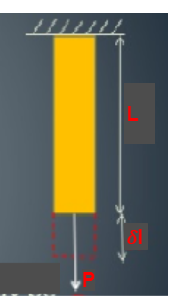
Let P = Gradually applied load
L = Length of bar
A =Cross-sectional area of the bar
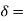Deflection produced in the bar
= Axial stress induced in the bar. It may be tensile or compressive,
Depending upon if the bar under consideration is under tensile or compressive load
E = Modulus of elasticity of bar material
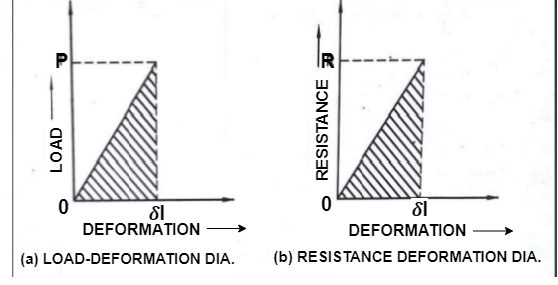
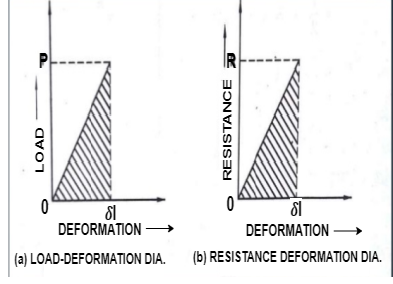
Work done on the bar = Area of the load – Deformation diagram
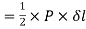
Work stored in the bar = Area of the resistance – Deformation diagram
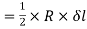
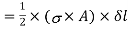
Now,
Work done = Work stored
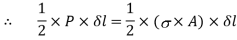
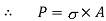
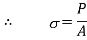
Strain Energy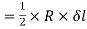 
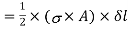
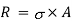
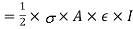
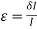
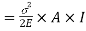
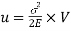strain energy due to gradual
(2) Strain Energy due to sudden loading:
When the load is applied suddenly the value of the load is P throughout the deformation.
But, Resistance R increase from O to R
Work done on the bar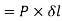 
Work done stored in the bar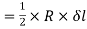 
…. (2)
Now, Work done = Work stored
× 
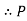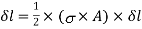
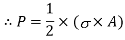
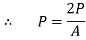
Hence the maximum stress intensity due to a suddenly applied load is twice the stress intensity produced by the load of the same magnitude applied gradually.
(3) Strain energy due to Impact loading:
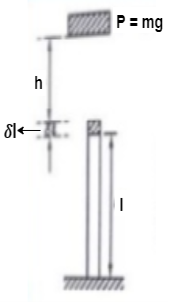
Load P is dropped through a height h, before it commences to load the bar.
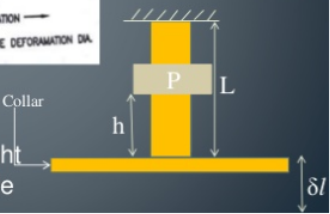
Work done on the bar = Force × Deformation
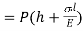…. (1) (
)
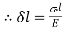Work stored in the bar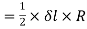 
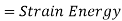
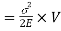… (2)
Now, Work done = Work stored
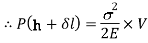
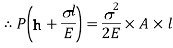
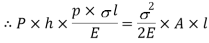
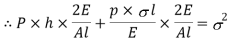
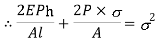
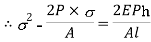
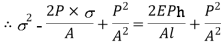
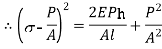
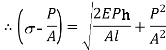
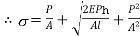
Stresses due to impact load
If load is applied suddenly, h=0
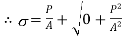
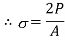
When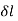 
is very small as compared to h, then
Work done = P × h
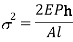
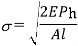
Strain energy for beams
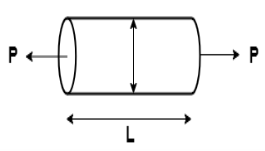
Consider a member subjected to a load P having Cross-sectional Area A and length L.
Let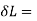 
Elongation in the bat.
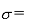Maximum stress induced in the member
When a load P acted on a body it undergoes a deformation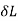
.
The earth plotted load Vs deformation as shown in fig.
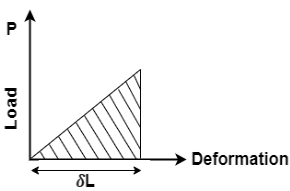
We know strain energy stored in a material is equal to area under the load-extension curve.
Strain energy stored = Work done by the load
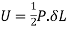
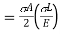
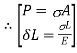
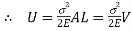
Strain energy and definition of a simply supported beam:
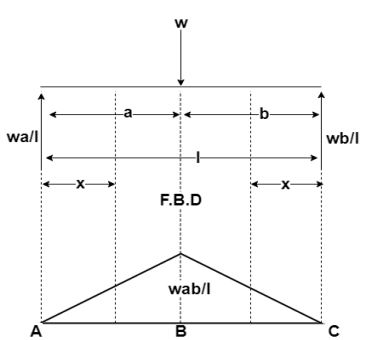
For portion AB,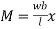
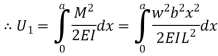
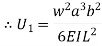
For Portion BC, 
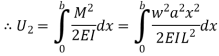
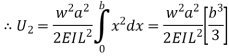
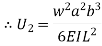
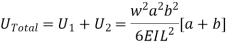
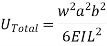
Now we know,
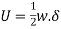
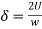
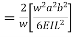
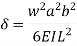
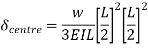
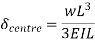
Strain Energy in Cylinder:
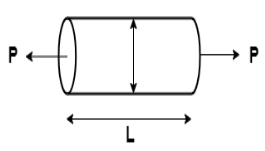
Consider a member subjected to a load P having cross sectional Area A and length L.
Let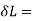 
Elongation in the bat.
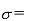Maximum stress induced in the member
When a load P acted on a body it undergoes a deformation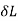
.
The earth plotted load Vs deformation as shown in fig.
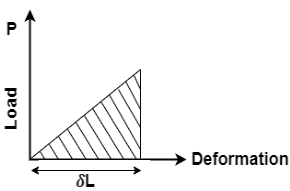
We know strain energy stored in a material is equal to area under the load-extension curve.
Strain energy stored = Work done by the load
