UNIT 4
DC Machines
The basic circuit model for a dc machine is shown below:
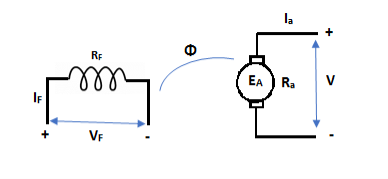
The machine operates in generating mode when the armature current is in the direction of induced emf Ea.
V = Ea – Ia Ra Ea> V
Pmech = Ea Ra = Pelect
The output power,
P0 = VIa
EaIa – VIa = Ia2 Ra
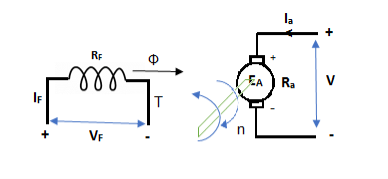
In this mode, the torque of electromagnetic origin is in opposite direction of rotation of armature.
[Pmech]gross = Shaft power = [Pmech]net + Rotational loss.
The average coil emf
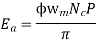
Wm = armature speed rad/s.
As parallel path emf is equal to armature emf,
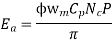
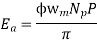
Np = turns / parallel path =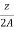 
Z = Total armature conductors
A = Number of parallel paths.
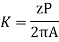
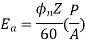
n = armature speed rpm
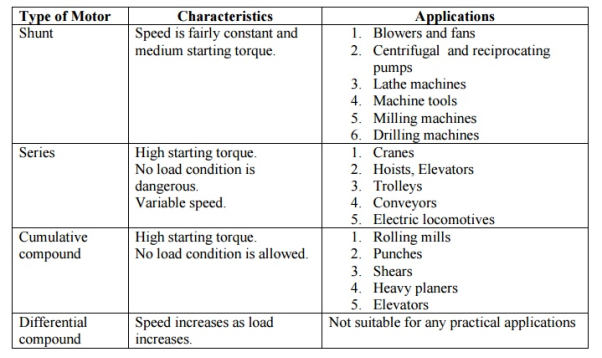
They are basically classified on the basis of their field excitation.
a) Separately excited generator: These generators have their field magnets energised from an independent external source of DC current. 
b) Self-Excited generator: In these types of generators the field magnets are energised by the current produced by the generators themselves. Due to residual magnetism, there is always some flux is present in the poles. There are three types of self-excited generators
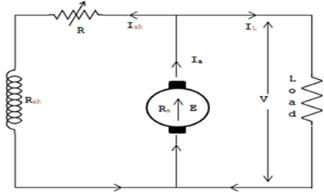
i) Shunt wound: The field windings are connected in parallel with the armature conductors and have full voltage of the generator applied
Across them.
Ii) Series wound: The field windings are connected in series with the armature conductors. They carry full load current.
Iii) Compound Wound: It is combination of few shunt and few series windings. In compound generator the shunt field is stronger than the series field. They are further classified as short shunt compound generator and long shunt compound generator depending on the shunt winding.
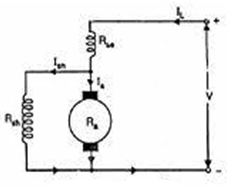
Fig: Short shunt DC generator
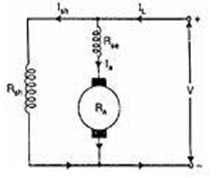
Fig: Long Shunt DC generator
In this mode, the armature current Ia flows opposite to the emf induced i.e. Ea. The basic circuit showing this mode is below:
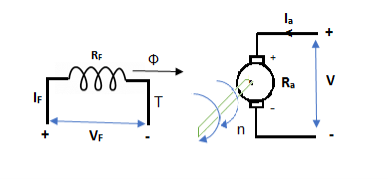
(Ea – Eb) is called as back emf here as it opposes the armature emf.
V = Ea + IaRa
Electrical power connected to mechanical form
Pelec / net = EaIa
Power input, Pi = V Ia
V Ia – EaIa = Ia2Ra = armature copper loss.
The back emf is given as
Eb =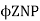 
In a DC motor when the armature rotates the conductors also rotate and hence cut the flux. According to the lower electromagnetic induction, the induced EMF is in the opposite direction (by Fleming’s Right hand rule) to the applied voltage. As it has opposite direction so it is referred to as back EMF (Eb).
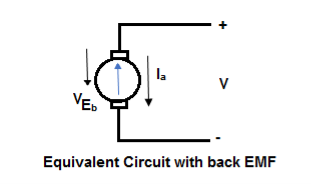
This back emf Ebis like battery put across main supply. This back emf is given as
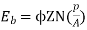N is in rpm.
- If speed of armature is high, Eb is large.
- If speed of armature is low, Eb is less.
Motor Torque
1) Armature Torque (Ta) of motor –
Power developed = Ta × 2πN W
As electrical power is converted to mechanical =EbIb
So, power developed = Power converted
Ta × 2πN = EbIb
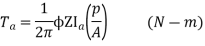
We can also conclude from the above eqn.
For series,
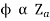
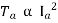
For shunt,
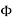is constant practically.
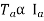
2) Shaft Torque (Tsh) –
The torque doing useful work is called as shaft torque, Tsh.
Motor output =Tsh × 2πN
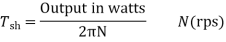
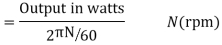
Due to iron and friction losses in motor Ta – Tsh difference torque exists called as lost torque.
Q. 1 A 4 pole dc generator runs at 650 rpm and generates emf of 220V. The armature is wave wound and has 790 conductors. If total flux/pole is 0.0145 Wb in each pole, find leakage coefficient.
Soln. Leakage coefficient =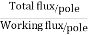
Working flux is given as
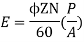
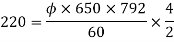
A = 2 for simple wave winding.
Leakage coefficient =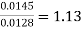
Q. 2A 4 pole lap wound dc shunt generator has a useful flux per pole of 0.05 Wb. The armature winding consists of 200 turns each of 0.06 u ohm resistance. Calculate the terminal voltage when running at 900 rpm with armature current of 50 A.
Soln. Terminal voltage V =Ea – Ia Ra
Ia Ra = 50×Ra.
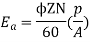
Z = 200×2=400 (each turn has two sides).
N = 900 rpm 
= 0.05 Wb P = A = 4
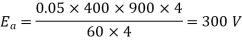
Total resistance of 200 turns = 200 × 0.004 = 0.8 ohm
As there are 4 parallel paths, so resistance of each path = 0.8/4 = 0.2 ohm
There are 4 resistances in parallel of each of 0.2 ohm
Ra = 0.2/4 = 0.05 ohm
Ia Ra = 50×0.05 = 25 V
V = Ea – Ia Ra = 300 – 25 = 275 V
Q. 3 A 220 V 4 pole wave wound dc series motor has 780 conductor on its armature. It has armature and series field resistance of 0.75 ohm. The motor takes a current of 30A. Find the speed and gross torque developed if flux/pole is 20 mWb?
Soln. Back emf,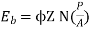 
Eb = V – IaRa = 220 – (30×0.75) = 197.5 V
197.5 = 20 × 10-3 ×780×N×0.75
N = 16.88 ≈ 17
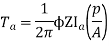
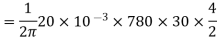
Ta = 158.97 Nm.
Q. 1 A 4 pole, 210 V shunt motor has 450 lap wound conductor. It takes 32 A from supply mains and develops output power of 4.5 kW. The field takes 1 A. The armature resistance is 0.09 ohm and flux per pole is 30 mWb. Calculate speed and torque.
Soln. Ia = 32-1 = 31 n
Eb = V – Ia Ra = 210 - (0.09×31) = 207.21 V
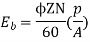
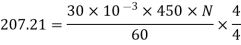
N = 920.9 rpm
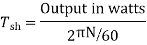
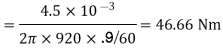
Q. 2 A 220 V, dc shunt motor has an armature resistance of 0.3 ohm and field resistance of 105 ohm. At no load speed is 1200 rpm and armature current is 2.3 A on application of rated load speed drops to 1120 rpm. Find the current and power input when motor delivers rated load.
Soln. N1 = 1200 rpm Eb1 = 220 – (0.3×2.3) = 219.31 V
N2 = 1120 rpm Eb2 = 220 – 0.3 Ia2
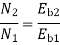
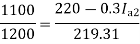
Ia2 = 37.93 A
Line current = Ia2 + Ish = 37.93 + (220/105) = 40 A
Power input = 220 × 40 = 8800 W
Q. 3 A 230 V shunt motor runs at 1000 rpm at no load and takes 6A. The total armature resistance is 0.2 ohm and field resistance is 210 ohm. Calculate the speed when loaded and taking 40 A. Assume flux constant.
Soln.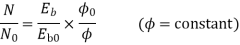 
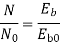
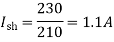
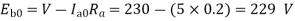
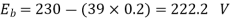
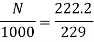
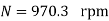
Q.4) The input to 230v DC shunt motor is 12kW. Calculate the torque developed, efficiency. No load current=6A, No load speed=1200rpm armature resistance=0.5Ω, shunt field resistance= 110Ω.
Sol: No load input =230x6=1380W
Ish=230/110 =2.1A
Armature current at no load = 6-2.1 = 3.9A
No load armature Cu loss= 3.92 x 0.5= 7.6W
Constant losses = 1380 – 7.6 = 1372.4W
When input is 12kW
Input current = 12000/230 = 52.17A
Armature current = 52.17 – 2.1=50.07A
Armature Cu loss = 50.072 x 0.5 =1253.69W
Total loss=1253.69+1372.4=2626.09W
Output= 12000-2626.09 =9373.9W
Efficiency = 9373.9/12000=0.781=78.1%
Q.5) A 230v DC series motor is running at a speed of 550rpm and draws 50A. Calculate at what speed the motor will run when developing half the torque. Total resistance of armature and field is 0.08Ω.
Sol: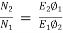 
=
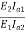
Ta α φIa
Ta α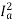 
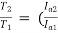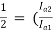)2
)2
Ia2 = 50x0.707=35.35A
E1=230- (50x0.08) =226V
E2=230- (35.35x0.08) =227.17V
=
=
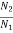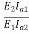
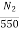N2= 781.97rpm
Q.6) A 230v shunt motor has an armature resistance of 0.3Ω and field resistance of 140Ω. The motor draws 5A at 1600rpm at no load. Calculate the speed of motor?
Sol: Ish= 230/140= 1.643A
Ia1=5-1.643=3.36A
Ia2=50-1.643=48.36A
E1= 230- (3.36x0.3) =226.34V
E2= 230- (48.36x0.3) =215.5V
=
=
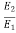
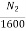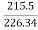
N2= 1523.37rpm
Q.7) A DC series motor drives a load, the torque if which varies as the square of the speed. Assuming magnetic circuit to remain unsaturated and negligible motor resistance. Calculate the reduction in motor terminal voltage which will reduce the motor speed half the value it has on full load.
Sol: Ta α φIa
Ta α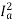 
Ta α 
N2 α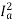 
=
=
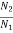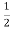
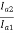Let V1 and V2 be voltage across motor in two cases.
E1=V1 and E2=V2
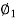αIa1
=
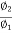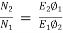
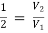x 2
= 
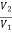% reduction in voltage = V1-V2/V1 x 100 = 4-1/4 x100 = 75%
% change in motor current = Ia1-Ia2/Ia1 x 100 = 50%
Q.8) A short shunt DC compound generator supplies 210 A at 100v the resistance of armature, series field and shunt field windings are 0.05Ω, 0.03Ω and 50Ω respectively. Find EMF generated.
Sol: For short shunt connection armature terminal voltage Va = 100+ (210x0.03) =106.3V
Shunt field current = 106.3/50 = 2.13A
Armature current =210+2.13 =212.13A
Induced emf = 106.30 + (212.13 x 0.05) = 116.9V
Q.9) The armature of a four pole DC shunt generator is lap wound and generates 210v when running at 500 rpm. Armature has 132 slots ,with 6 conductors/slot. If armature is rewound, wave connected, find EMF generated with same flux/pole running at 300rpm.
Sol: Total conductors Z = 132x6= 792
The emf E=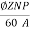 
For lap winding P=A
E=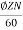 
210=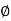
x 792 x 500/60
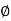= 31.82mWb
If wave connected number of parallel paths = 2
The emf E=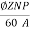 
= (31.82x10-3 x 792 x 300/60) (4/2)
E= 252V
Q.10) A 15kW 400V 350rpm dc shunt motor has current 30A at full load. The moment of inertia of rotating system is 6.5kg-m2. The starting current be 1.2 times of full load current. Find the full load torque?
Sol: Full load output = 15000W
Speed N=350rpm=5.83rps
Output = Tω
T = 15000/2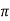
x5.83 = 409.3N-m
Q.11) A DC series motor operates at 600 rpm with line current of 110A from 220v main. It’s armature resistance is 0.2Ω and field resistance is 0.1ohm Find the speed at which motor runs at a line current of 25A, given flux at this current is 45% of flux at 110A.
Sol: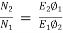 
= 0.45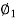 
E1=220 – (0.2+0.1) x 110=187V
E2=220 – (0.2+0.1) x 25 = 212.5V
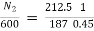
N2= 1515.15rpm
Q.12) A belt driven,120kWshunt generator running at 310 rpm on 220v busbars continues to run as a motor when the belt breaks, then taking 10kW. What will be its speed with armature resistance of 0.03 Ω field resistance of 60Ω and contact drop under each brush is 1v.
Sol: Input current = 120x1000/220 = 545.45A
Shunt current Ish= 220/60 = 3.67A
Armature current Ia= 545.5-3.67=541.83A
E2= 220 – (541.83 x 0.03)- 2x1[drop across brush]
E2=205.74V
The armature current Ia now becomes Ia= 545.5+3.67= 549.17A
As the system here runs as a generator.
E1= 220 – (549.17 x 0.03) +1x2 = 201.5V
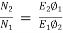
As shunt current is constant so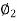 
= 
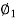
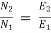
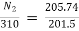
N2= 316.5rpm
