Unit IV
Decision Theory
Introduction
Decision theory deals with how to determine the best course of action when many options are available and the outcome cannot be reliably predicted.
It's hard to imagine a situation without such a decision problem, but we're primarily limited to problems that occur in the business, and the results can be explained in dollars of profit or revenue, cost or loss. It may be reasonable to consider these issue as the best alternative in the long run, with the highest profit or profit on average, or the lowest cost or loss. This criterion of optimality is not without its drawbacks, but it should serve as a useful guide to behavior in repetitive situations where the results are not critical. (Another criterion of optimality, maximizing expected usefulness, provides consistent decision makers with a more personal and subjective guide to behavior.)
The simplest decision problem is to list the probabilities associated with the possible monetary outcome of each alternative, calculate the expected monetary value of all alternatives, and select the alternative with the highest expected monetary value. You can solve it by doing. Determining the best alternative is a bit more complicated when:
The alternative involves a series of decisions.
Another class of problem often gets additional information about uncertain variables at a particular cost. This additional information is rarely completely accurate. Its value, and the maximum amount you are willing to pay to get it, must depend on the difference from the best you can expect to do with the help of this information.
And the best I expect to do without it. These are the types of problems we are about to start.
Decisions Situations
Very simply, the decision question is how to choose the best of the available options. The elements in question are possible alternatives (actions, actions), possible events (states, results of random processes), probabilities of these events, results associated with each possible combination of alternative events, and criteria (decision) Rule) The best option will be selected accordingly.
Grocery stores receive eggs every Thursday Morning. This shipment must continue until the next Thursday when the new shipment is received. All unsold eggs by Thursday will be destroyed. Eggs sell for $ 10 per $ 100 and cost $ 8 per $ 100. Weekly demand for eggs at this grocery store varies from week to week. From past experience, the following probability distributions are assigned to weekly demand.
Request
(Hundreds of eggs): 10 11 12 13 14
Probability: 0.1 0.2 0.4 0.2 0.1
This demand pattern is stable throughout the year, egg demand is not seasonal and trends are flat. The question is how many eggs need to be ordered and delivered every Thursday.
Possible alternatives, possible events, probabilities of these events, and associated results are shown in the payoff table.
(Entries show profit in hundred of dollar)
Event Alternative
hundred of egg (hundred of egg ordered
demanded | Probability | 10 | 11 12 13 14 |
10 | 0.1 | 20 | 12 4 − 4 −12 |
11 | 0.2 | 20 | 22 14 6 − 2 |
12 | 0.4 | 20 | 22 24 16 8 |
13 | 0.2 | 20 | 22 24 26 18 |
14 | 0.1 | 20 | 22 24 26 28 |
Expected profit: 20 21∗ 20 1† 8
To illustrate the structure of this table, suppose you ordered 12 (100) eggs and the purchase cost is 12x8 or 96 (hundreds of dollars). If 10 (100) eggs are requested, 10 will be sold and the revenue will be 10x10 or 100 ($ 00). The profit associated with this alternate event pair is 100-96 or 4 ($ 00). Similarly, if the demand is 12, the profit is (12x10)-(12x8) or $ 24. However, if the demand exceeds 12, only 12 can be sold and the profit remains at $ 24. We assume that if demand is not met, there will be no additional penalties such as loss of goodwill. Now consider the first option. If you order 10 pieces, the profit will be $ 20 no matter what the demand. In contrast, the last option offers the possibility of realizing a profit of $ 28, but also the possibility of a loss of $ 12.
As well as the possibility of intermediate profit values. In fact, the profit of each option can be represented by a random variable, which is a function of the random variable that represents demand. For example, if Y is a random variable that represents the profit of the third option, then the probability distribution of Y is:
y p(y
4 0.1
14 0.2
24 0.F
1.0
So if you order 12 eggs every Thursday morning, in the long run, you'll get a profit of $ 4 in one week out of ten weeks, a profit of $ 14 in two weeks, and $ 24 in seven weeks out of ten weeks. Benefits are realized. The expected profit, or long-term average profit, is simply the average of Y.
(4[(0.1[‡ (14[(0.2[ ‡ (24[(0.F[ = 20.
The expected profit of all choices is displayed in the last row of the payoff table. In this case, it makes a lot of sense to use expected profit as a criterion for choosing the best option. The alternative with the highest average long-term profit is also the one with the highest long-term gross profit. The second option with an expected profit of $ 21 is the best alternative under this standard.
Of course, the definition of a decision problem applies to many situations. In a sense, what to cook for dinner, who to marry, what clothes to buy, these are all decisions. Not all decision problems here relate to us. In most cases, the results are limited to business issues that are measured in dollars.
Profit, Revenue, or Cost, a reasonable measure of optimality is the alternative with the highest expected monetary value, that is, the highest expected profit or income, or the lowest expected cost. Is to choose an alternative probabilities can be interpreted as long-term relative frequencies and expected rewards, as these problems tend to be of a repetitive type in the long run, in return. However, similar optimization criteria can be applied to a wider class of decision problems. As explained in the next section
Decision makers can act in uncertain situations according to certain rational rules of conduct. In that case, the best action in the decision-making problem is the one with the highest expected utility. The expected utility of an action is calculated by roughly replacing each outcome with its "utility" and then by the personal value of the outcome to the decision maker, such as expected benefit or cost.
Types of Decisions- Making Environment
It is said that the main responsibility of all managers is decision making. The manager follows a series of steps to make the right decisions that benefit the company. This process is known as the decision-making process. However, the decision-making environment is also an important part of the process. Learn some important aspects of the decision-making environment.
The quality of decisions made by an organization determines the success or failure of the aforementioned business.
Therefore, you should consider all available information and alternatives before making any important decisions. The decision-making process is very helpful.
Another factor that influences these decisions is the environment in which they are made. There are several different types of environments in which these decisions are made.
Also, the type of decision-making environment influences the way decisions are made. There are three main types of decision-making environments. Let's take a brief look at each one.
Decision making Certainty, Risk and Uncertainty
1] Certainty
This type of environment is, by its very nature, very reliable and reliable. This means that all information is available and at hand. Such data is also easily available and not very expensive to collect.
Therefore, managers have all the information they need to make well-thought-out decisions. The manager can also select the best option by analyzing all the options and their results.
Another way to ensure a secure environment is for the manager to create a closed system. This means he chooses to focus on only a few options. He gets all the information available about such alternatives that he is analyzing. He ignores other factors that make the information unavailable. Such factors have nothing to do with him.
2] Uncertainty
In an uncertain decision-making environment, the information available to managers is often incomplete, inadequate, and unreliable.
In an uncertain environment, everything is in flux. Some external and random forces mean that the environment is the most unpredictable.
In these chaotic times, all variables change rapidly. However, managers need to fully understand this mayhem. He must create some order, obtain reliable data, and make the best decisions according to his judgment.
3] Risk
Multiple events can occur in risky situations. In other words, the manager must first check the likelihood and probability of an event occurring or not occurring.
Managers typically make this deduction based on past experience.
Even in this scenario, the manager has some information available. However, the availability and reliability of the information is not guaranteed. He needs to graph some alternative behavioral policies from the data he has.
Decision- Tree Analysis
What is Decision Tree Analysis?
Decision tree analysis is a graphic representation of the various alternative solutions available to solve a problem. When making a choice, the explanation is often decisive. Decision tree analysis is created by answering a few questions that continue until you make the final choice, each time you get a positive or negative answer.
Decision Process
Decision tree analysis is a scientific model and is often used in an organization's decision-making process. When making decisions, management already envisions alternative ideas and solutions. Decision trees provide a graphical representation of alternative solutions and possible choices, which makes it easier to make informed choices. This graphic representation is characterized by a tree-like structure that allows decision-making problems to be viewed in the form of flowcharts, each with a branch of alternative options.
What if-
Decision tree analysis makes good use of the "what if" idea. There are several options that take into account both the risks and benefits that a particular choice may pose. The decision tree becomes clear about the outcome of the decisions made, as possible alternatives are also clearly displayed.
Expression
There are several ways to represent a decision tree. This analysis is usually represented by lines, squares, and circles. Squares represent decisions, lines represent results, and circles represent uncertain results. Keeping the lines as far apart gives you plenty of space to add new considerations and ideas.
A decision tree representation can be created in four steps:
Example of Decision Tree Analysis
Suppose a for-profit company wants to increase sales and related profits next year.
You can then use the decision tree to map different alternatives. There are two options for increasing both sales and profits: 1-increasing advertising costs and 2-expanding sales activities. This will create two branches. Option 1 to 2 new options are born. That is, 1-1 is the new agency and 1-2 is to use the services of the existing agency. Option 2 presents two follow-up options in sequence. 2-1-Collaboration with agents or use of 2-2-Original sales support system.
The branch continues.
The next choice from 1-1 is:
1-1-1 Budget will increase by 10%-> Final result: Sales will increase by 6% and profit will increase by 2%
1-1-2 Budget will increase by 5%-> Final result: Sales will increase by 4% and profit will increase by 1.5%
Alternatives resulting from 1.2:
1-2-1 Budget increased by 10%-> Final result: Sales increased by 5%, profit increased by 2.5%
1-2-2 Budget increased by 5%-> Final result: Sales increased by 4%, profit increased by 12%
From 2.1 onwards it will probably look like this:
2-1-1 Setup with own dealer-> Final result: Sales increased by 20%, profit increased by 5%
2-1-2 Cooperation with existing dealers-> Final result: Sales increased by 12.5%, profit increased by 8%
From 2.2 onwards it will probably look like this:
2-2-1 Recruitment of new sales staff-> Final result: Sales increased by 15%, profit increased by 5%
2-2-2 Motivate existing sales staff-> End result: Sales will increase by 4% and profit will increase by 2%.The above example probably shows that the company chooses 1-2-2. This is because the forecast for this decision will increase profits by 12%.
This analysis provides clear proof and is especially useful in situations where it may be desirable to develop various alternatives to decision making in a structured way. This method is increasingly being used by practitioners and technicians to make diagnoses and identify car problems.
Key takeaways:
Introduction
The major pioneers of game theory were the 1940s mathematician John von Neumann and the economist Oskar Morgenstern. Mathematician John Nash is considered by many to provide the first significant extension of the work of von Neumann and Morgenstern!
The focus of theory of games may be a game that acts as a model for an interactive situation between rational players. The key to game theory is that the rewards of one player depend on the strategy implemented by the other player. The game identifies the player's identity, preferences, available strategies, and how these strategies affect results. Depending on the model, various other requirements and assumptions may be required.
According to game theory, the actions and choices of all participants influence their outcomes!
Let's start with Nash equilibrium
Nash equilibrium is the result reached, which means that once achieved, the payoff cannot be increased by unilaterally changing decisions. You can also think of it as "no regrets" in the sense that once you make a decision, you will not regret a decision that takes into account the consequences.
In most cases, the Nash equilibrium is achieved over time. However, once the Nash equilibrium is reached, it does not deviate. After learning how to find the Nash equilibrium, let's see how unilateral movements affect the situation. Does it make sense? The Nash equilibrium is described as "no regrets" because it should not be. It is generally believed that there is multiple equilibrium in a game.
This mainly happens in games that have more complex elements than the two choices of two players. In a simultaneous game that repeats over time, one of these multiple equilibrium is reached after trial and error. This scenario of various overtime choices before reaching equilibrium is most often performed in the business world when two companies are deciding on prices for compatible products such as airfares and soft drinks. The impact of game theory on economics and business
Game theory has revolutionized economics by addressing the critical problems of previous mathematical economic models. Neoclassical economics, for example, struggled to understand the expectations of entrepreneurs and was unable to cope with imperfect competition. Game theory turned its attention to the market process itself from steady-state equilibrium.
In business, game theory is undoubtedly useful for modeling competing behaviour between economic agents. Enterprises often have several strategic options that affect their ability to achieve economic benefits. For example, companies may face dilemmas such as discontinuing existing products, starting new product development, lowering prices compared to competitors, or adopting new marketing strategies. Economists tend to use game theory to understand the behaviour of oligopolistic businesses. This helps predict the possible consequences of a company engaging in certain actions such as price fixing or collusion.
The limits of game theory
The biggest problem with game theory, like most other economic models, is that it relies on the assumption that people are selfish and rational actors who maximize utility. We are social beings who often cooperate at our own expense and consider the welfare of others! Game theory cannot explain the fact that depending on the social situation and who the player is, the situation may or may not be in Nash equilibrium.
Two -person zero sum game payoff matrix
Determine which of the following two-person zero-sum games are strictly determinable and fair. Give optimum strategies for each player in the case of strictly determinable games:
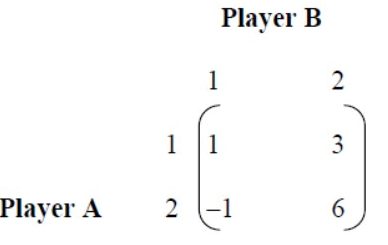
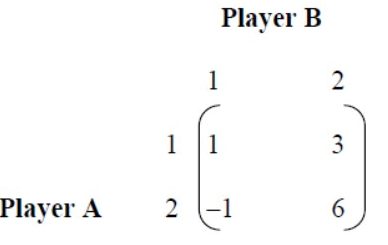
(a) 
(b) 
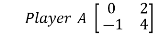
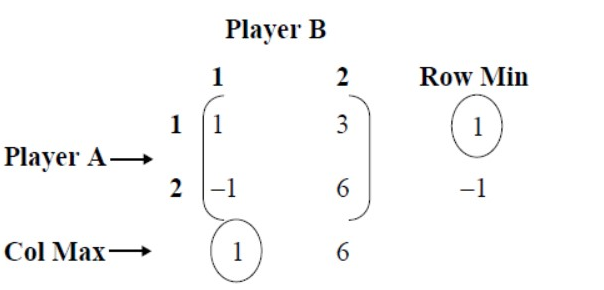
The payoff matrix for player A is
Player A | Player B | Row minima | |
B1 | B2 | ||
A1 | 5+ | 0* | 0 |
A2 | 0* | 2+ | 0 |
Column maxima | 5 | 2 |
|
The payoffs marked with [*] in each row reflect the minimum payoff and the payoffs marked with [+] in each column of the payoff matrix represent the full payoff. 
(maximinim) is the largest portion of the minimum lines.
Value) and the smallest Column Maximum part represents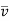 
(minimax value).
Thus obviously, we have 
=0 and 
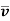2.
Since 
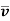, the game is not strictly determinable.
Pure Strategy Games (Saddle Point available). Principles of Dominance method
Issue of the Game
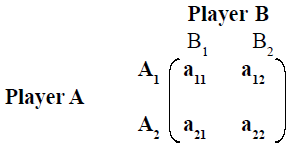
The game is worked out using the minimax method. In each row, find the smallest value and select the largest value from these values. Next, in each column, find the largest value and pick the smallest of these numbers. The following table shows the procedure.
Minimax Procedure
If Maximum value in row is equal to the minimum value in column, then saddle point exists.
Max Min = Min Max
1 = 1
Therefore, there is a saddle point.
The strategies are,
Player A plays Strategy A1, (A A1).
Player B plays Strategy B1, (B B1).
Value of game = 1.
Mixed strategies: Games without saddle point.
For any given pay off matrix without saddle point the optimum mixed strategies are shown in Table
Mixed Strategies
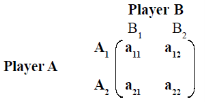
Let p1 and p2 be the probability for Player A.
Let q1 and q2 be the probability for Player B.
Let the optimal strategy be SA for player A and SB for player B.
Then the optimal strategies are given in the following tables.
Optimum Strategies
p1and p2 are determined by using the formulae,
p1 =
a22-a21
(a11+a22)-(a12+a21
and p2= 1-p1
q1=
a22-a21
(a11+a22)-(a12+a21
and q2= 1-q1
the value of the game w.r.t. player A is given by,
Value of the game, v =
a11 a22– a12a21
(a11+a22)-(a12+a21
Example: Solve the pay-off given table matrix and determine the optimal strategies and the value of game.
Game Problem
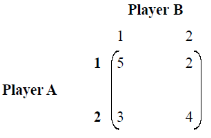
Solution: Let the optimal strategies of SA and SB is as shown in tables.
Optimal Strategies
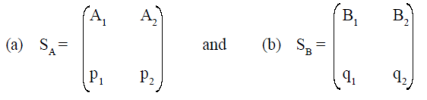
The given pay-off matrix is shown below in Table.
Pay-off Matrix or Maximin Procedure
Therefore, there is no saddle point and hence it has a mixed strategy. Applying the probability formula,
p1 =
a22-a21
(a11+a22)-(a12+a21
=
4-3
(5+4)-(2+3)
=
1
9-5
=
1
4
and p2= 1-p1
= 1-1/4= 3/4
q1=
a22-a21
(a11+a22)-(a12+a21
4-2
(5+4)-(2+3)
=
2
9-5
=
1
2
and q2= 1-q1
Value of the game, v =
a11 a22– a12a21
(a11+a22)-(a12+a21
=14/4
The optimum mixed strategies are shown in table below.
Optimum Mixed Strategies
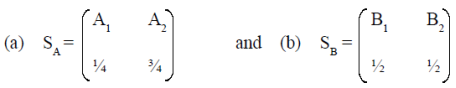
Key takeaway:
References:
