Unit - 4
Differentiability of a function at a point & in an interval
Derivability in an interval:
A function f(x) defined on [a, b] is derivable at the end point a which means f’(a) exists if
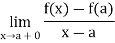
Or
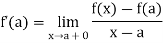
Similarly it is derivable at the end point b, if
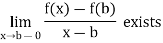
If a function is derivable at all points of an interval except the end points, it is said to be derivable in the open interval.
A function is derivable in the closed interval [a, b] if it is derivable in the open interval ]a, b[ and also at the end points a and b.
Example: A function f is defined on R by
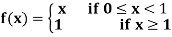
Consider the derivability at x = 1.
Sol:
Lf’(1) =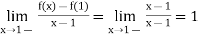 
Rf’(1) =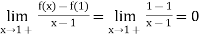 
Hence
Lf’(1) and Rf’(1) are not equal.
Thus
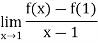
Does not exists. Which means f’(1) does not exists.
Example: A function f is defined as
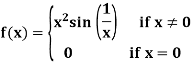
Is derivable at x = 0 but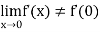 
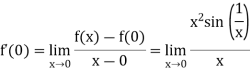
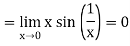
We know that from elementary calculus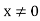
,
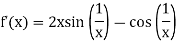
Clearly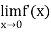 
does not exists and therefore there is no possibility of 
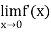being equal to f’(0).
Thus
f’(x) is not continuous at x = 0 but f’(0) exists.
Theorem: A function which is derivable at a point is necessarily continuous at that point.
Proof:
Let a function f be derivable at x = c.
So that
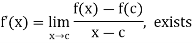
Now
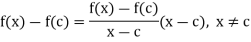
Taking limits as x tends to c, we get
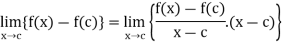
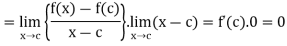
So that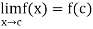 
and therefore f is continuous at x = c.
Key takeaways:
- A function f(x) defined on [a, b] is derivable at the end point a which means f’(a) exists if
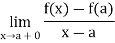
2. If a function is derivable at all points of an interval except the end points, it is said to be derivable in the open interval.
3. A function which is derivable at a point is necessarily continuous at that point.
Caratheodory's theorem:
Let f be defined on an interval I containing the point c.
Then f is differentiable at c if and only if there exists a function w on I that is continuous at c and satisfies
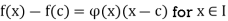……….(1)
In this case, we have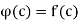 
Proof:
If f’(c) exists, we can define 
by
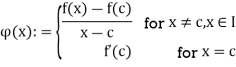
The continuity of w follows from the fact that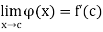 
If x = c, then both sides of
(1) equal 0,while if x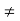 
c, then multiplication of 
by x - c gives (1) for all other x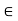 
I.
Now assume that a function w that is continuous at c and satisfying (1) exists. If
We divide (10) by x - c 
0, then the continuity of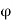 
implies that
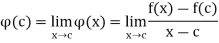
Exists. Therefore f is differentiable at c and f’(c) =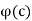 
Example: we consider the function f defined by f(x) =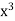 
For x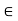 
R. For c 
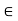R, we see from the factorization
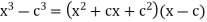
That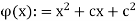 
satisfies the conditions of the theorem. Therefore, we conclude that f is differentiable at c 
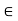R and that f’(c) =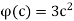 
Chain rule:
Let I, J be intervals in R, let g : I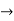 
R and f : J 
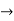R be functions such that f(J)
I, and let c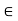 
J. If f is differentiable at c and if g is differentiable at f (c), then the composite function g o f is differentiable at c and
(g o f)’ (c) = g’(f(c)) . f’(c) ……(1)
Proof:
Since f’(x) exists, Caratheodory’s Theorem implies that there exists a function w on J such that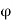 
(c) is continuous at c and f(x)- f(c) = 
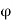(x) (x – c) for x 
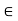J, and where 
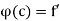(c). Also, since g’(f(c)) exists, there is a function 
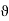defined on I such that 
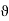is continuous at d := f f(c) and g(y) – g(d) = 
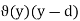for y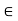 
I, where
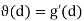. Substitution of y = f(x) and d = f(c) then produces
For all x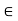 
J such that f (x) 
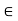I. Since the function 
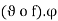is continuous at c and its
Value at c is g’(f(c)).f’(c) Caratheodory’s Theorem gives (1).
If g is differentiable on I, if f is differentiable on J, and if f(J)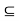 
I, then it follows from the Chain Rule that (g o f)’ = (g’ o f). f’ which can also be written in the form
D(g o f) = (Dg o f) .Df
Example: If f : I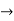 
R is differentiable on I and g(y) = 
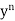for y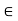 
R and
n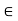 
N, then since g’(y) = 
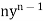, it follows from the Chain Rule
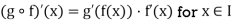
Therefore we have
Example: Suppose that f is defined by
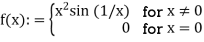
If we use the fact that Dsin x = cos x for all x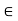 
R and apply the Product Rule and the Chain Rule
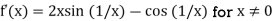
If x = 0, none of the calculational rules may be applied.
Consequently, the derivative of f at x = 0 must be found by applying the definition of derivative.
We find that
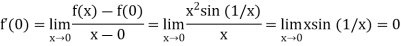
Hence, the derivative f’ of f exists at all x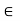 
R. However, the function f’ does not have a limit at x = 0, and consequently f’ is discontinuous at x = 0. Thus, a function f that is differentiable at every point of R need not have a continuous derivative f’.
Algebra of differentiable function:
The existence of the derivative of a function at a point depends on the existence of a limit
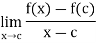
Therefore keeping in view the corresponding theorem on limits, we can establish the following fundamental theorem on derivative.
In the function f, g are derivable at c then the functions f + g, f – g, fg anf f/g (note- g(c) should be non-zero) are also derivable at c, and
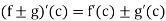
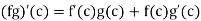
In order to illustrate the procedure, we will go through the following theorem:
Theorem: if f is derivable at c and f(c) is non-zero then the function 1/f is also derivable thereat, and
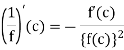
Since f is derivable at c, it is also continuous.
Again since f(c) is non-zero, there exists a neighbourhood of N of c wherein f does not vanish,
Now
Proceeding to limits when x tends to c, we have
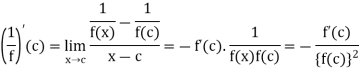
Thus the limit exists and equals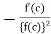 
Mean value theorem
As we know that, the function f : I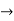 
R is said to have a relative maximum [respectively, relative minimum] at c 
I if there exists a neighborhood V :=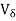 
(c) of c such that f(x) 
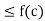[respectively, f(c) 
] for all x in V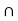 
I. We say that f has a relative extremum at c 
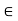I if it has either a relative maximum or a relative minimum at c.
Mean value theorem:
Suppose that f is continuous on a closed interval
I := [a, b], and that f has a derivative in the open interval (a, b). Then there exists at least one point c in (a, b) such that
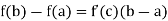
Proof:
Consider the function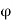 
defined on I by
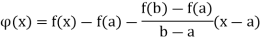
[The function w is simply the difference of f and the function whose graph is the line segment joining the points (a, f (a)) and (b, f (b))]
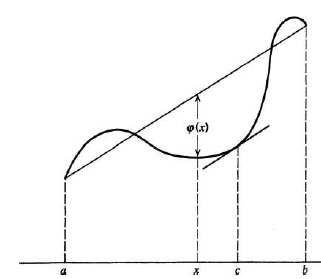
The hypotheses of Rolle’s Theorem are satisfied by 
since w is continuous on [a, b], differentiable on (a, b), and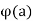 
= 
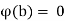. Therefore, there exists a point c in (a, b) such that
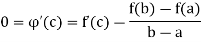
Hence, f(b) – f(a) = f’(c) (b –a)
Theorem:
Suppose that f is continuous on the closed interval I := [a, b], that f is differentiable on the open interval (a, b), and that f’(x) = 0 for x 
Then f is constant on I.
Proof:
We will show that f(x) = f(a) for all x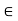 
I. Indeed, if x 
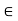I, x > a, is given, we apply the Mean Value Theorem to f on the closed interval [a, x]. We obtain a
Point c (depending on x) between a and x such that f(x) – f(a) = f’(c) (x – a) since f’(c) = 0 (by hypothesis), we deduce that f(x) – f(a) = 0. Hence, f(x) = f(a) for any x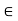 
Suppose that f and g are continuous on I := [a, b], that they are differentiable on (a, b), and that f’(x) = g’(x) for all x 
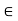(a, b). Then there exists a constant C such that f = g + C on I.
Key takeaways:
- The existence of the derivative of a function at a point depends on the existence of a limit
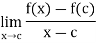
2. Suppose that f is continuous on a closed interval I := [a, b], and that f has a derivative in the open interval (a, b). Then there exists at least one point c in (a, b) such that
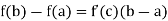
Interior Extremum Theorem:
Let c be an interior point of the interval I at which f : I 
R has a relative extremum. If the derivative of f at c exists, then f’(c) = 0.
Proof:
We will prove the result only for the case that f has a relative maximum at c; the
Proof for the case of a relative minimum is similar.
If f’(c)> 0, then there exists a neighborhood V 
I of c such that
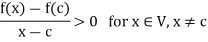
If x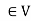
and x > c, then we have
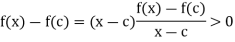
But this contradicts the hypothesis that f has a relative maximum at c. Thus we cannot
Have f’(c) > 0. Similarly, we cannot have f’(c) < 0. Therefore we must have f’(c) = 0.
Rolle’s theorem-
Suppose f(x) is a function defined on [a , b] and it satisfies the following conditions
1. f(x) is continuous in [a , b]
2. f(x) is differentiable in (a , b)
3. f(a) = f(b)
Then there exists atleast a point point c ϵ (a , b) , where a<b , such that f’(c) = 0
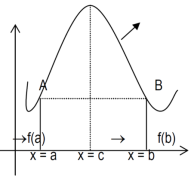
Proof: suppose y = f(x) is a function and A(a , f(a)) , B(b , f(b)) be two points on the curve f(x) and a,b are two end points. Now conditions for Rolle’s theorem-
1.f(x) is a continuous function in[a , b] , from the figure without breaks in between A&B on y = f(x).
2. f(x) is differentiable in (a , b), because joining A and B we get a line AB.
Slope of the line AB=0 then a point C at P also a tangent at P, or Q,R,S is parallel to x –axis.
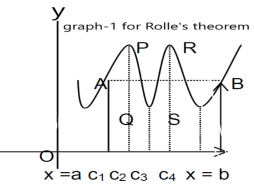
Slope of the tangent at P or Q,R,S , will be 0 ,even the curve y = f(x) decreases or increases, that means f(x) is constant.
Derivative of f(x),
f’(c) = 0
That’s why, f’(c) = 0
3. The slope of the line AB is equal to zero, that means the line AB is parallel to x-axis.
So that, f(a) = f(b)
Example: Verify Rolle’s theorem for the function f(x) = x(x+3)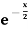 
in interval [-3, 0].
Sol. First we will differentiate the given function with respect to x, we get
f’(x) = (x²+3x)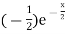 
+ (2x + 3)
= 
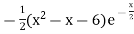
This shows that f’(x) exists for all x, therefore f(x) is continuous for all x.
Now, f(-3) = 0 and f(0) = 0 , so that f(-3) = f(0).
Here f(x) satisfies all the conditions of Rolle’s theorem,
Then,
f’(x) = 0 , which gives
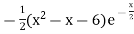= 0
We get,
X = 3 and x = -2
Here we can see that clearly -3<-2<0 , therefore there exists -2 ∈ (-3,0) such that
f’(-2) = 0
That means the Rolle’s theorem is true for the given function.
Example: Verify Rolle’s theorem for the given functions below-
1. f(x) = x³ - 6x²+11x-6 in the interval [1,3]
2. f(x) = x²-4x+8 in the interval [1,3]
Sol:
As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]
Also, f(1) = f(3) = 0
Now we find f’(x) = 0
3x² - 12x +11 = 0
We get, x = 2+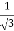 
and 2 -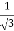 
Hence both of them lie in (1,3).
Hence the theorem holds good for the given function in interval [1,3]
(2) As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]
Also, f(1) = 1 -4 +8 = 5 and f(3) = 9 – 12 + 8 = 5
Hence f(1) = f(3)
Now the first derivative of the function,
f’(x) = 0
2x – 4 = 0 , gives
X = 2
We can see that 1<2<3, hence there exists 2 between 1 and 3. And f’(2) = 0.
This means that the Rolle’s theorem holds good for the given function and given interval.
Example: Verify the Rolle’s theorem for sin x in the interval [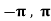
]
Sol. Suppose f(x) = sin x
We know that sin x is continuous for all x.
Now, f’(x) = cos x exists for all x in (
) and
f(
0
f(
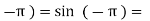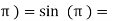0
Thus f(x) satisfies all the conditions of Rolle’s theorem.
Now,
f’(x) = 0 that gives, cos x = 0
x =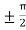 
Here we notice that both intervals lie in (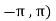
.
There exists, c =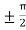 
So that, f’(c) = 0
The Rolle’s theorem has been verified.
Key takeaways:
1. Let c be an interior point of the interval I at which f : I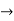 
R has a relative extremum. If the derivative of f at c exists, then f’(c) = 0.
2. Suppose f(x) is a function defined on [a , b] and it satisfies the following conditions
1. f(x) is continuous in [a , b]
2. f(x) is differentiable in (a , b)
3. f(a) = f(b)
Then there exists atleast a point point c ϵ (a , b) , where a<b , such that f’(c) = 0
The Intermediate Value Property of Derivatives:
Lemma: Let I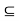 
R be an interval, let f : I 
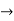R, let c 
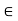I, and assume that f has a derivative at c. Then:
(a) If f’(c) > 0, then there is a number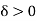 
such that f(x) > f(c) for 
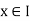such that c < x < c +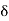 
(b) If f’(c) < 0, then there is a number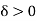 
such that f(x) > f(c) for 
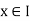such that c -
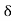< x < c.
Proof:
Since
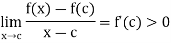
It follows that there is a number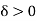 
such that if c 
and 0 < |x – c| <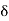 
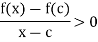
If x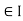 
also satisfies x 
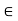c, then we have
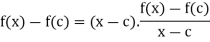
Hence, if 
and c < x < c + 
Similarly we can prove the second part.
Darboux’s theorem:
If f is differentiable on I = [a, b] and if k is a number between f’(a) and f’(b), then there is at least one point c in (a, b) such that f’(c) = k.
Proof: Suppose that f’(a) < k < f’(b). We define g on I by g(x) : = kx – f(x) for x 
Since g is continuous, it attains a maximum value of I. Since g’(a) = k – f’(a) > 0, it follows from above lemma that the maximum of g does not occur at x = a.
Similarly since g’(b) = k – f’(b) < 0, it follows from lemma (b) that the maximum does not occur at x = b.
Therefore, g attains its maximum at some c in (a, b).
Then we have 0 = g’(c) = k – f’(c).
Hence f’(c) = k.
Example: The function g: [-1, 1] 
defined by
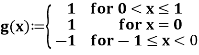
(which is a restriction of the signum function) clearly fails to satisfy the intermediate value property on the interval [-1, 1]. Therefore, by Darboux’s Theorem, there does not exist a function f such that f’(x) = g(x) for all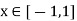 
In other words, g is not the derivative on [-1,1] of any function.
One very important use of the Mean Value Theorem is to obtain certain inequalities.
Whenever information concerning the range of the derivative of a function is available, this information can be used to deduce certain properties of the function itself.
Let’s illustrate by the following example:
Example-1: The exponential function f(x) =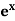 
has the derivative f’(x) = 
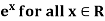. Thus f’(x) > 1 for x > 0 and f’(x) < 1 for x < 0. From these relationships, we drive the inequality,
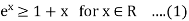with equality occurring if and only if x = 0.
If x = 0, we have equality with both sides equal to 1. If x > 0, we apply the Mean
Value Theorem to the function f on the interval [0, x]. Then for some c with 0 < c < x we have
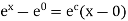
Since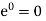 
and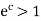 
. This becomes 
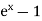> x so that we have 
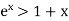for x > 0.
A similar argument establishes the same strict inequality for x < 0.
Thus the inequality (1) holds for all x, and equality occurs only if x = 0.
Example-2: The function g(x) := sin x has the derivative g’(x) = cos x for all x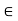 
R. On the basis of the fact that -1 
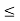cos x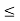 
1 for all x 
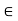R, we will show that
-x 
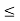sin x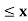 
for all x 
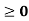…….. (1)
Indeed, if we apply the Mean Value Theorem to g on the interval [0, x], where x > 0, we Obtain
Sin x - sin 0 = (cos c)(x – 0)
For some c between 0 and x. Since sin 0 = 0 and -1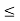 
cos c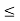 
1, we have -x 
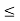sin x 
since equality holds at x = 0, the inequality (1) is established.
References:
1. Erwin Kreyszig, Advanced Engineering Mathematics, 9thEdition, John Wiley & Sons, 2006.
2. Advanced engineering mathematics, by HK Dass
3. Mathematical analysis by Dr. Anju panwar
4. Real analysis by Dr. Sachin Kaushal
5. Real analysis by Ajit kumar & S.Kumaresan
6. Principles of real analysis by S.C Malik
7. A BASIC COURSE IN REAL ANALYSIS- AJIT KUMAR
