Unit - 4
Contraction mappings and applications
The concept of contraction mapping is very useful in classical analysis.
Definition-
Let (X, d) be a metric space. A mapping T of X into itself is said to be a contraction (or contraction mapping) if there exists a real number a, 0 < a < 1, such that d(Tx, Ty)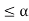 d(x, y) for all x, y
d(x, y) for all x, y 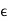 X.
X.
Note- a contraction mapping is uniformly continuous
A point x 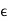 X is called a fixed point of the mapping T : X
X is called a fixed point of the mapping T : X  X if Tx = x.
X if Tx = x.
Theorem- Let T: X 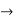 X be a contraction of the complete metric space (X, d). Then T has a unique fixed point.
X be a contraction of the complete metric space (X, d). Then T has a unique fixed point.
Proof:
Let 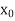
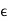 X and let
X and let 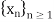 be the sequence defined iteratively by
be the sequence defined iteratively by 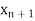 = T
= T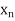 for n = 0, 1, 2, . . . . We shall prove that
for n = 0, 1, 2, . . . . We shall prove that 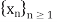 is a Cauchy sequence. For p = 1, 2, . . . , we have
is a Cauchy sequence. For p = 1, 2, . . . , we have
 ………..(1)
………..(1)
Where 0 < < 1 is such that
< 1 is such that
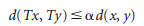
For all x, y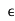 X.
X.
Repeated application of the inequality (1) gives
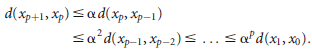
Now, let m, n be positive integers with m > n. By the triangle inequality,
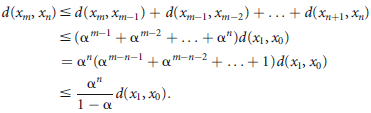
But 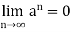 it follows
it follows 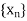 is a Cauchy sequence in (X, d), which is complete. Let y =
is a Cauchy sequence in (X, d), which is complete. Let y = 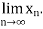 Since T is a contraction, it is continuous. It follows that Ty = T(
Since T is a contraction, it is continuous. It follows that Ty = T(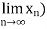 =
= 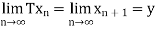 . Thus, y is a fixed point of T.
. Thus, y is a fixed point of T.
Moreover, it can be shown to be unique: If y 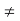 z are such that Ty = y and Tz = z, then d(y,z) = d(Ty,Tz)
z are such that Ty = y and Tz = z, then d(y,z) = d(Ty,Tz) 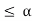 d(y,z)<d(y, z). This implies d(y,z) = 0, i.e., y = z.
d(y,z)<d(y, z). This implies d(y,z) = 0, i.e., y = z.
Proposition: Suppose 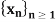 is a sequence in any metric space (X, d) and k a positive integer such that each of the k subsequences
is a sequence in any metric space (X, d) and k a positive integer such that each of the k subsequences
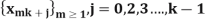
Converges to the same limit x. Then 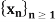 converges to x.
converges to x.
Proof:
Given  > 0, there exist positive integers
> 0, there exist positive integers 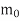 ,
,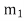 . . . ,
. . . ,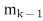 such that
such that
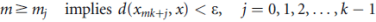
Take N = max {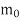 ,
,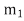 . . . ,
. . . ,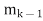 }. Consider any n
}. Consider any n 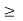 Nk. By the division algorithm, N = mk + j, where 0
Nk. By the division algorithm, N = mk + j, where 0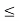 j
j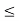 k - 1. Since m
k - 1. Since m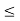 N -1 would imply that N
N -1 would imply that N  (N - 1)k+ j
(N - 1)k+ j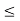 (N - 1)k+ (k- 1) = Nk - 1, we must have m>N - 1, so that m
(N - 1)k+ (k- 1) = Nk - 1, we must have m>N - 1, so that m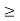 N
N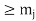 for j = 0, 1, 2, . . . ,k- 1. It follows that d(
for j = 0, 1, 2, . . . ,k- 1. It follows that d(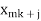 ,x)<
,x)<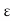 , i.e., d(
, i.e., d(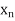 ,x)<
,x)<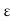 .
.
This has been proved for any n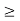 Nk. Therefore,
Nk. Therefore, 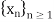 converges to x.
converges to x.
Proposition- Let X consist of continuous mappings from [a, b] into [l,m], so that X is a subspace of the space C[a, b] of all continuous real-valued functions defined on [a, b]. Then X is a complete metric space.
Proof:
Since X 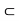 [a, b] and the latter is complete, it is enough to show that X is a closed subset of it. Accordingly, we assume that f
[a, b] and the latter is complete, it is enough to show that X is a closed subset of it. Accordingly, we assume that f 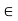 [a, b] is a limit point of X and proceed to show that f
[a, b] is a limit point of X and proceed to show that f 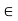 X. There exists a sequence
X. There exists a sequence 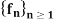 in X that converges to f with respect to the metric of C[a, b]. For each x 2 [a, b], we have
in X that converges to f with respect to the metric of C[a, b]. For each x 2 [a, b], we have
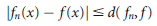
And hence 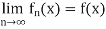 But for each x
But for each x 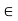 [a, b], we have
[a, b], we have
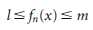
And so,
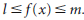
Which shows that f  X. This completes the proof.
X. This completes the proof.
Picard’s existence theorem-
Consider the domain D: = {(x,y) 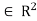 : |x-
: |x-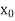 | < a, |y-
| < a, |y-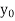 | < b } . Assume that f: D
| < b } . Assume that f: D 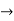 R is a continuous function satisfying the Lipschitz condition in the y-variable uniformly in the x-variable, that is,
R is a continuous function satisfying the Lipschitz condition in the y-variable uniformly in the x-variable, that is,
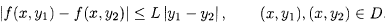
Then there exists a 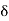 > 0 and g:[
> 0 and g:[ 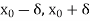 ]
]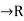 which is a solution of the initial value problem-
which is a solution of the initial value problem-
g’(x) = f (x, g(x)) satisfying the initial condition g(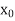 ) =
) = 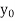
Proof:
By an application of the fundamental theorem of calculus, equivalent to the integral equation
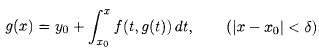
Let M > 0 be such that |f (x, y)| 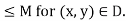
Choose 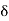 > 0 such that
> 0 such that
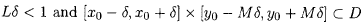
Define
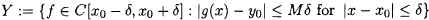
Then Y is closed in 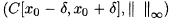 and hence is a complete metric space. The map
and hence is a complete metric space. The map
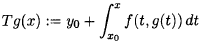
Is a contraction on Y.
Definition
A (metric) space X is said to be connected if the only sets which are both open and closed in X are 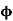 and the full space X, when X is a metric space.
and the full space X, when X is a metric space.
A subset A of a metric space X is said to be connected if A is a connected space when considered as a (metric) space with the induced (or subspace) topology. More explicitly, this amounts to saying that (A, 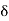 ) is connected, where .5 is the restriction of the metric d on X to A.
) is connected, where .5 is the restriction of the metric d on X to A.
Definition:
A metric space (X, d) is said to be disconnected if there exist two nonempty subsets A and B of X such that
(i) X = A 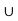 B;
B;
(ii) A 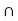
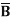 =
=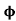 and
and 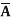
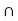 B =
B = 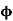
That is, the subsets must be nonempty, together they must constitute the whole space and neither may contain a point of the closure of the other. If no such subsets exist, then (X, d) is said to be connected.
Theorem- Let (R, d) be the space of real numbers with the usual metric. A subset I 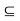 R is connected if and only if I is an interval, i.e., I is of one of the following forms:
R is connected if and only if I is an interval, i.e., I is of one of the following forms:
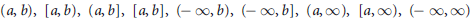
Proof:
Let I be a connected subset of real numbers and suppose, if possible, that I is not an interval. Then there exist real numbers x, y, z with x < z < y and x, y 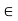 I but z
I but z 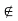 I . Then I may expressed as I = A
I . Then I may expressed as I = A 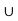 B, where
B, where
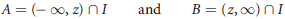
Since x 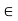 A and y
A and y 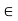 B, therefore, A and B are nonempty; also, they are clearly disjoint and open in I. Thus, I is disconnected
B, therefore, A and B are nonempty; also, they are clearly disjoint and open in I. Thus, I is disconnected
To prove the converse, suppose I is an interval but is not connected. Then there are nonempty subsets A and B such that
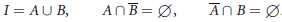
Pick x 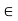 A and y
A and y 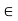 B and assume that x < y. Observe that [x, y]
B and assume that x < y. Observe that [x, y] 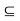 I , for I is an interval. Define
I , for I is an interval. Define
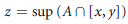
The supremum exists since A 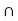 [x, y] is bounded above by y and it is nonempty, as x is in it. Then z
[x, y] is bounded above by y and it is nonempty, as x is in it. Then z 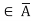 . (We shall show that if z
. (We shall show that if z 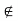 A, then z is a limit point of A. Let
A, then z is a limit point of A. Let  > 0 be arbitrary. By the definition of supremum, there exists some element a
> 0 be arbitrary. By the definition of supremum, there exists some element a 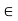 A such that z -
A such that z - 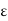 < a
< a 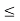 z, i.e., every neighbourhood of z contains a point of A.) Hence, z
z, i.e., every neighbourhood of z contains a point of A.) Hence, z 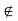 B, for
B, for 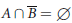 ; in particular, x
; in particular, x 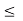 z < y.
z < y.
If z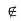 A, then x < z < y and z
A, then x < z < y and z 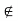 I. This contradicts the fact that [x, y]
I. This contradicts the fact that [x, y] 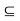 I . If z
I . If z 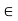 A, then for
A, then for
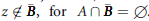
So there exists a d > 0 such that 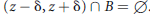
This implies that there exists 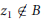 satisfying the inequality z < z1 < y. Then x
satisfying the inequality z < z1 < y. Then x 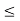 z < z1 < y and z1
z < z1 < y and z1 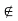 I, for z1 being greater than sup (A
I, for z1 being greater than sup (A 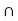 [x, y]) is not in A. This contradicts the fact that [x, y]
[x, y]) is not in A. This contradicts the fact that [x, y] 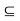 I.
I.
Theorem-
Let (X, 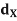 ) be a connected metric space and f: (X,
) be a connected metric space and f: (X, 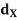 )
) 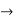 (Y,
(Y, 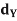 ) be a continuous mapping. Then the space f (X) with the metric induced from Y is connected.
) be a continuous mapping. Then the space f (X) with the metric induced from Y is connected.
Proof:
The map f : X 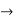 f (X) is continuous. If f (X) were not connected, then there would be, a continuous mapping, g say, of f (X) onto the discrete two element space (
f (X) is continuous. If f (X) were not connected, then there would be, a continuous mapping, g say, of f (X) onto the discrete two element space (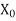 ,
, 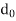 ). Then g o f :X
). Then g o f :X 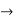
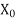 would also be a continuous mapping of X onto
would also be a continuous mapping of X onto 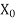 , contradicting the connectedness of X.
, contradicting the connectedness of X.
Theorem-
Let I = [_ 1, 1] and let f: I 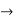 I be continuous. Then there exists a point c
I be continuous. Then there exists a point c 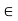 I such that f (c) = c.
I such that f (c) = c.
Proof:
If f (- 1) = -1 or f (1) = 1, the required conclusion follows; hence, we can assume that f (-1) > -1 and f (1) < 1. Define
g(x) = f (x) - x, x 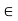 I :
I :
Note that g is continuous, being the difference of continuous functions, and that it satisfies the inequalities g(-1) = f (-1) + 1 > 0 and g(1) = f (1) - 1 < 0. Hence, by the Weierstrass intermediate value theorem, there exists c 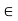 (- 1, 1) such that g(c) = 0, that is, f (c) = c.
(- 1, 1) such that g(c) = 0, that is, f (c) = c.
Theorem-
If Y is a connected set in a metric space (X, d) then any set Z such that Y 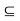 Z
Z 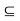
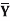 is connected.
is connected.
Proof:
Suppose A and B are two nonempty open sets in Z such that A 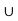 B = Z and A
B = Z and A 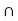 B =
B = 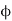 1; as Y is dense in Z, Y
1; as Y is dense in Z, Y 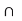 A and Y
A and Y 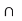 B are nonempty open sets in Y and we have
B are nonempty open sets in Y and we have
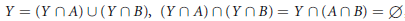
a contradiction.
Local Connectedness-
A metric space (X, d) is said to be locally connected if, for x 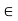 X, there is a base of connected neighbourhoods of x. Thus, X is locally connected if and only if the family of all open connected sets is a base for the open subsets of X
X, there is a base of connected neighbourhoods of x. Thus, X is locally connected if and only if the family of all open connected sets is a base for the open subsets of X
Theorem- Let (X, d) be a metric space. X is locally connected if and only if the connected components of the open sets in X are open in X. (For any subset A of X, the connected components of the points of the subspace A are called the connected components of A).
Proof:
Let G be an open subset of X, and C be a component of G, and {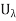 :
:  L} be a basis consisting of open connected sets for the open sets of X.
L} be a basis consisting of open connected sets for the open sets of X.
Let x 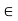 C. Since x
C. Since x 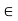 G, there is a
G, there is a 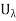 such that x
such that x 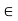
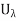
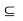 G; but since C is the component of x and
G; but since C is the component of x and 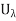 is connected, x
is connected, x 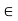
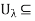 C.We have thus shown that C is a union of open subsets of X and is, therefore, open.
C.We have thus shown that C is a union of open subsets of X and is, therefore, open.
On the other hand, if O is any open set containing a point x  X, the connected component of x in the subspace O is a connected neighbourhood of x contained in O; hence, X is locally connected.
X, the connected component of x in the subspace O is a connected neighbourhood of x contained in O; hence, X is locally connected.
Theorem- Let O be a nonempty open subset of (R, d). Then O is a union of an
At most countable family of open intervals, no two of which have common points.
Proof:
The connected components of O are intervals and that they are open subsets of O and, therefore, of R as well. Thus, they are open intervals. Note that O 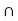 Q, where Q denotes the set of rationals in R, is countable and each component of O contains a point of O
Q, where Q denotes the set of rationals in R, is countable and each component of O contains a point of O 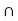 Q. The mapping r
Q. The mapping r 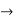 C(r) of O
C(r) of O  Q into the set of connected components of O is onto and, therefore, the set of connected components of O is countable.
Q into the set of connected components of O is onto and, therefore, the set of connected components of O is countable.
Path-wise Connectedness
Definition-
Let (X, d) be a metric space, Y  X and I = [0, 1]. A path in Y is a continuous mapping f : I
X and I = [0, 1]. A path in Y is a continuous mapping f : I  Y . If f : I
Y . If f : I  Y is a path in Y, we call f (0)
Y is a path in Y, we call f (0) 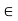 Y its initial point, f (1)
Y its initial point, f (1) 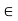 Y its final point and say that f joins f (0) and f (1) or that f runs from f (0) to f (1). A subset Y of X is said to be path-wise connected if, for any two points in Y, there exists a path running from one to the other, i.e. the points can be ‘‘joined by’’ a path in Y.
Y its final point and say that f joins f (0) and f (1) or that f runs from f (0) to f (1). A subset Y of X is said to be path-wise connected if, for any two points in Y, there exists a path running from one to the other, i.e. the points can be ‘‘joined by’’ a path in Y.
Note- Path-wise connectedness is also known as arc-wise connectedness.
Bounded sets-
A metric space (X, d) is said to be compact if every open covering G of X has a finite subcovering, that is, there is a finite subcollection {G1,G2, . . . , Gn} 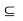 G such that
G such that
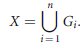
A nonempty subset Y of X is said to be compact if it is a compact metric space with the metric induced on it by d.
If G is an open covering of X, then the collection F of complements of sets in G is a collection of closed sets whose intersection is empty, and, conversely.
Thus, a metric space (X,d) is compact if and only if every collection of closed sets with empty intersection has a finite subcollection with empty intersection.
Note- A collection F of sets in X is said to have the finite intersection property if every finite subcollection of F has a nonempty intersection.
Proposition-
(i) (X, d) is compact;
(ii) every collection of closed sets in (X, d) with empty intersection has a finite subcollection with empty intersection;
(iii) every collection of closed sets in (X, d) with the finite intersection property has nonempty intersection.
Definition-
Let (X, d) be a metric space and e be an arbitrary positive number.
Then a subset A 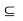 X is said to be an «-net for X if, given any x
X is said to be an «-net for X if, given any x 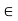 X, there exists a point y
X, there exists a point y 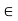 A such that d(x, y) <
A such that d(x, y) < 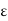 . In other words, A is an
. In other words, A is an 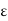 -net for X if
-net for X if
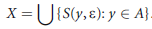
A finite subset of X that is an e-net for X is called a finite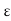 -net for X.
-net for X.
Definition-
The metric space (X, d) is said to be totally bounded if, for any 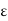 > 0, there exists a finite
> 0, there exists a finite 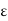 -net for (X, d). A nonempty subset Y of X is said to be totally bounded if the subspace Y is totally bounded.
-net for (X, d). A nonempty subset Y of X is said to be totally bounded if the subspace Y is totally bounded.
Proposition- A totally bounded metric space is bounded.
Proof:
Let (X, d) be totally bounded and suppose 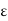 > 0 has been given. Then there exists a finite
> 0 has been given. Then there exists a finite 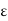 -net for X, say A. Since A is a finite set of points, d(A) = sup{d(y, z): y, z
-net for X, say A. Since A is a finite set of points, d(A) = sup{d(y, z): y, z 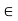 A} <
A} < 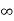 . Now, let x1 and x2 be any two points of X. There exist points y and z in A such tha
. Now, let x1 and x2 be any two points of X. There exist points y and z in A such tha
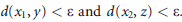
It follows, using the triangle inequality, that
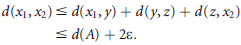
So that
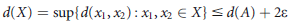
And, hence, X is bounded.
Proposition-
Let (X, d) be a compact metric space. Then (X, d) is totally bounded.
Proof:
For any given 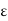 > 0, the collection of all balls S(x,
> 0, the collection of all balls S(x, 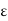 ) for x
) for x 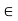 X is an open cover of X. The compactness of X implies that this open cover contains a finite subcover. Hence, for
X is an open cover of X. The compactness of X implies that this open cover contains a finite subcover. Hence, for 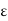 > 0, X is covered by a finite number of open balls of radius
> 0, X is covered by a finite number of open balls of radius  , i.e., the centres of the balls in the finite subcover form a finite
, i.e., the centres of the balls in the finite subcover form a finite 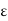 -net for X. So, X is totally bounded.
-net for X. So, X is totally bounded.
Note- A metric space is compact if and only if it is complete and totally bounded.
Proposition-
Any continuous function f from a compact (metric) space (X, d) to another metric space (Y, d) is bounded, that is, f (X) is a bounded subset of Y.
Proof:
Fix any y 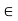 Y. For each n
Y. For each n 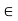 N, the set B(y, n) is an open set in Y and hence
N, the set B(y, n) is an open set in Y and hence
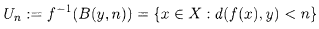
Is open. Note also that U 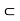
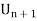 . The collection {
. The collection {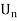 : n
: n 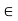 N} is an open cover of the compact space X. If {
N} is an open cover of the compact space X. If {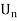 : 1
: 1 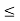 i
i 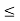 m} is a finite subcover and if N := max{
m} is a finite subcover and if N := max{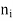 }, then X =
}, then X = 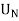 , that is, f (X)
, that is, f (X) 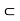 B(y, N).
B(y, N).
Hence f (X) is bounded.
Theorem:
Any continuous function from a compact (metric) space to R attains its bounds.
Or
Let X be compact and f: X —> R be continuous. Let M := M := sup{ f (x) : x 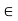 X} and m := inf{f (x) : x
X} and m := inf{f (x) : x 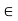 X } . Then there
X } . Then there
Exist 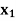 ,
, 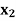
 X such that f (
X such that f (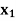 ) = M and f (
) = M and f (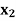 ) = m.
) = m.
Proof:
There exists an 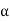
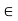 R such that |f(x)|
R such that |f(x)| 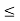
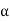 for all x
for all x 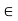 X. Hence m and M exist as real numbers.
X. Hence m and M exist as real numbers.
We shall prove the existence of 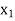 by contradiction. If there is no p
by contradiction. If there is no p 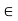 X such that
X such that
f (p) = M , then the set

Form an open cover of X.
(For, if x 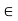 X, then f(x) <M and hence by Archimedean property of R , there exists n
X, then f(x) <M and hence by Archimedean property of R , there exists n 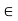 N such that n > 1/M-f(x) hence f(x) < M - 1/n or x
N such that n > 1/M-f(x) hence f(x) < M - 1/n or x 
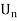 .) Note that
.) Note that 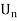
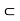 Un+ 1 for all n. As in the last proof, we conclude that X =
Un+ 1 for all n. As in the last proof, we conclude that X = 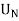 for some N. But this leads to the contradiction: sup{ f (x) : x
for some N. But this leads to the contradiction: sup{ f (x) : x 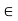 X}
X} 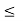 M — 1/n !
M — 1/n !
Theorem- Any continuous function from a compact metric space to any other metric space is uniformly continuous.
Proof:
Let f: (X, d) 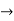 (Y, d) be continuous. Assume that X is compact.
(Y, d) be continuous. Assume that X is compact.
We need to prove that f is uniformly continuous on X.
A naive attempt would runs as follows. For a given 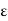 > 0, for each x, there exists
> 0, for each x, there exists 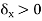 by continuity of f at x. Since X is compact, the open cover {B(x,
by continuity of f at x. Since X is compact, the open cover {B(x,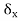 ): x
): x 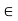 X} admits a finite subcover, say, {B(
X} admits a finite subcover, say, {B(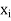 ,
, 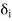 ) : 1
) : 1 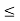 j
j 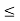 n} where
n} where 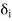 =
= 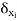 . One may be tempted to believe that if we set
. One may be tempted to believe that if we set 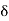 = min{
= min{ 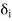 : 1
: 1  i
i 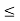 n, it might work. See where the problem is. Once you arrive at a complete proof, you may refer to the proof below. We now modify the argument and complete the proof. Given
n, it might work. See where the problem is. Once you arrive at a complete proof, you may refer to the proof below. We now modify the argument and complete the proof. Given 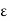 > 0, by the continuity of f at x, there exists an
> 0, by the continuity of f at x, there exists an 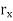 > 0 such that
> 0 such that
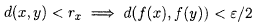
Instead of the open cover {B(x, 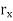 ) : x
) : x  X}, we consider the open
X}, we consider the open
Cover {B(x, 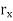 /2) : X
/2) : X 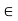 X and apply compactness. Let {B(x„
X and apply compactness. Let {B(x„ 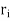 /2) : 1
/2) : 1 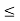 i
i 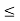 n} be a finite subcover. (Here
n} be a finite subcover. (Here 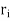 =
= 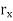 „ 1
„ 1 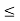 j
j 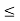 n.) Let
n.) Let
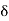 := min{
:= min{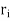 /2 : 1
/2 : 1 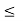 j
j 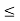 n}. Let x, y
n}. Let x, y 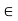 X be such that d(x, y) <
X be such that d(x, y) < 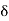 . Now
. Now
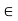 B (
B ( ,
,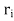 /2) for some i. Since d(x, y) <
/2) for some i. Since d(x, y) < 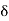 , we see that
, we see that
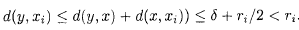
Thus y  B (
B ( ,
, ). It follows that
). It follows that
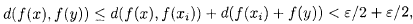
By our choice of  . Thus f is uniformly continuous.
. Thus f is uniformly continuous.
Note- Let f be a homeomorphism of a metric space (X, d) onto a metric space (Y, d). Then X is compact if and only if Y is compact.
References:
- S. Kumaresan, Topology of Metric Spaces, Narosa Publishing House, Second Edition 2011
- Topology of metric spaces, S. Kumarsen, Alpha science international Ltd.
- Metric spaces, Satish shirali and Harkrishan L. Vasudeva, Springer.