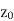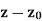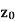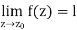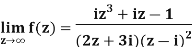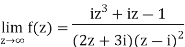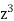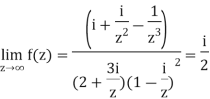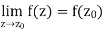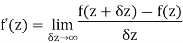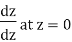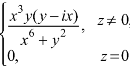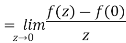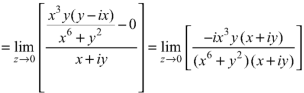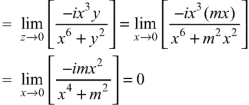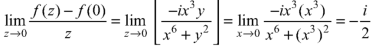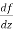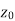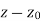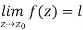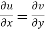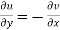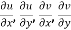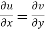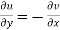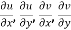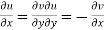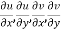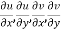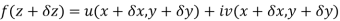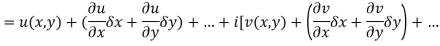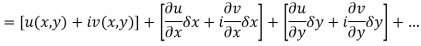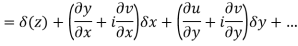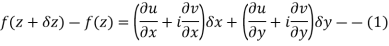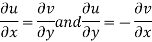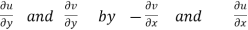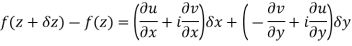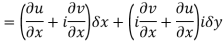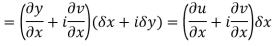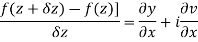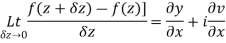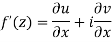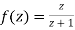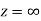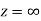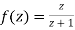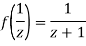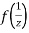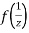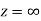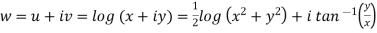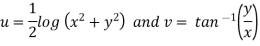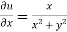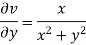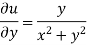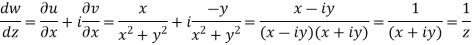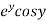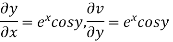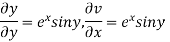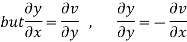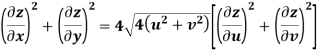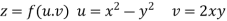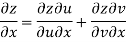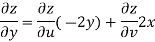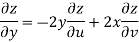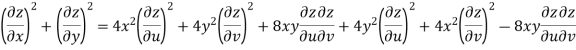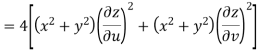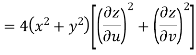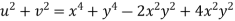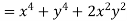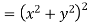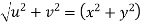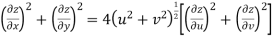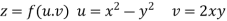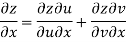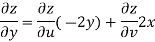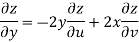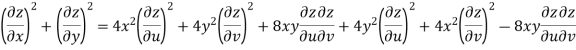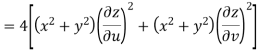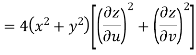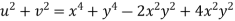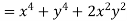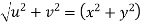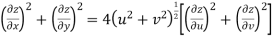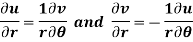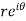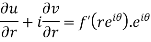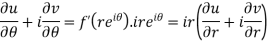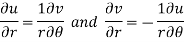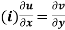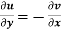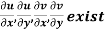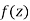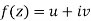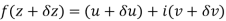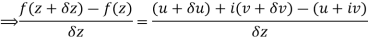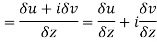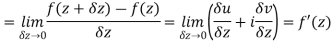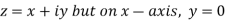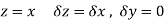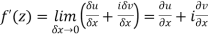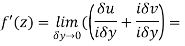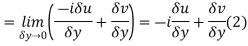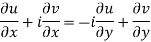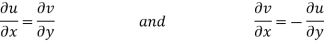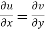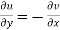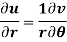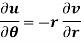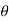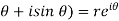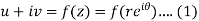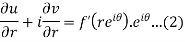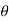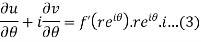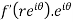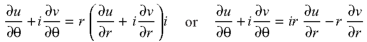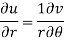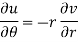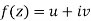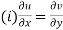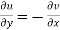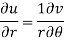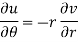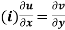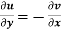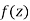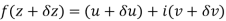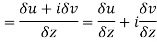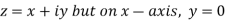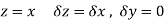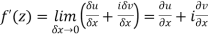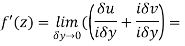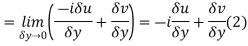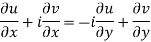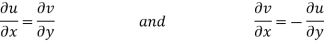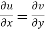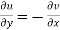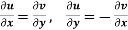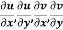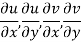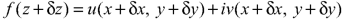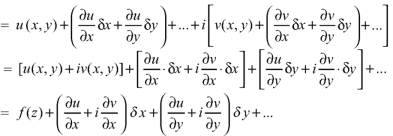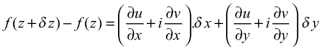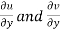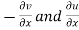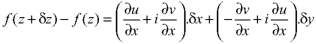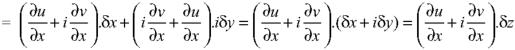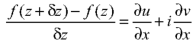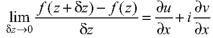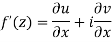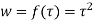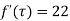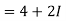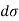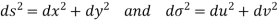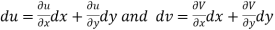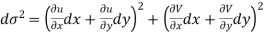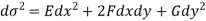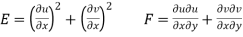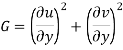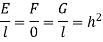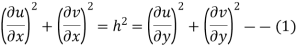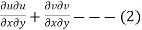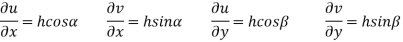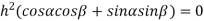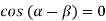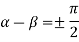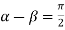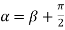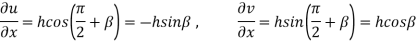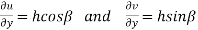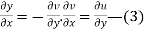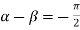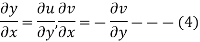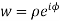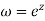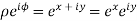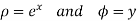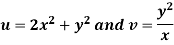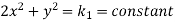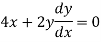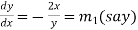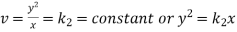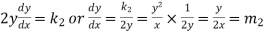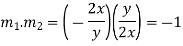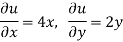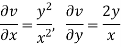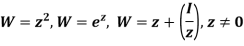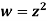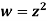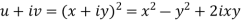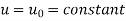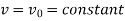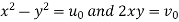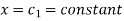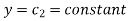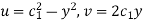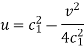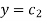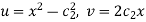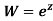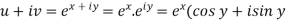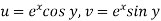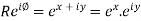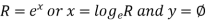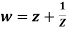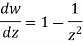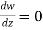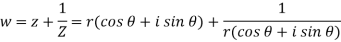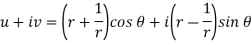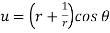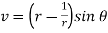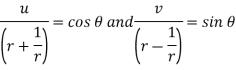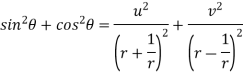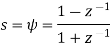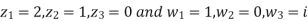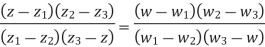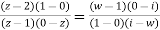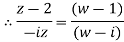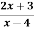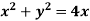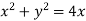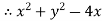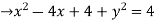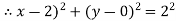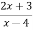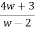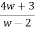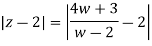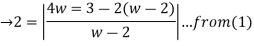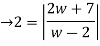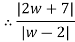Unit 2
Complex Variables
In the narrow sense of the term, the theory of function of a complex variable is the theory of analytic functions (cf. Analytic function) of one or several complex variables. As an independent discipline, the theory of functions of a complex variable took shape in about the middle of the 19th century as the theory of analytic functions.
Complex function-
x + iy is a complex variable which is denoted by z If for each value of the complex variable z = x + iy in a region R, we have one or more than one values of w = u + iv, then w is called a complex function of z. And it is denoted as- w = u(x , y) + iv(x , y) = f(z) |
Neighbourhood of 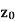
Let a point | Is called ε- neighbourhood of |
Limit of a function of a complex variable-
Suppose f(z) is a single valued function defined at all points in some neighbourhood of point 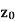 -
-
The-
|
Example-1: Find-
|
Sol.
Here we have-
Divide numerator and denominator by
|
Continuity- A function w = f(z) is said to be continuous at z = 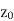 , if
, if
|
Also if w = f(z) = u(x , y) + iv(x , y) is continuous at z = 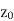 then u(x , y), v(x , y) are also continuous at z =
then u(x , y), v(x , y) are also continuous at z = 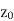 .
.
Differentiability-
Let f(z) be a single valued function of the variable z, then
|
Provided that the limit exists and has the same value for all the different ways in which 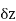 approaches to zero.
approaches to zero.
Example-2: if f(z) is a complex function given below, then discuss
|
Sol.
If z→0 along radius vector y = mx
But along
In different paths we get different value of |
Key takeaways
| 2. Limit of a function of a complex variable- Suppose f(z) is a single valued function defined at all points in some neighbourhood of point The-
|
A function 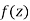 is said to be analytic at a point
is said to be analytic at a point 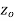 if f is differentiable not only at
if f is differentiable not only at 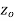 but every point of some neighborhood at
but every point of some neighborhood at 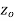 .
.
Note-
1. A point at which the function is not differentiable is called singular point.
2. A function which is analytic everywhere is called an entire function.
3. An entire function is always analytic, differentiable and continuous function. (converse is not true)
4. Analytic function is always differentiable and continuous but converse is not true.
5. A differentiable function is always continuous but converse is not true.
The necessary condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are- 1. 2. Provided Equation (1) and (2) are known as Cauchy-Riemann equations. |
The sufficient condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are- 1. 2.
|
Important note-
1. If a function is analytic in a domain D, then u and v will satisfy Cauchy-Riemann conditions.
2. C-R conditions are necessary but not sufficient for analytic function.
3. C-R conditions are sufficient if the partial derivative are continuous.
State and prove sufficient condition for analytic functions
Statement – The sufficient condition for a function 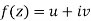 to be analytic at all points in a region R are
to be analytic at all points in a region R are
1 2 |
Proof:
Let f(z) be a simple valued function having
Ignoring the terms of second power and higher power
We know C-R equation
Replacing Respectively in (1) we get
Show that Ans: The function f(z) is analytic at Since
Now |
Example-1: If w = log z, then find 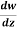 . Also determine where w is non-analytic.
. Also determine where w is non-analytic.
Sol.
Here we have Therefore-
Again-
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0). So that w is analytic everywhere but not at z = 0
|
Example-2: Prove that the function 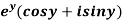 is an analytical function.
is an analytical function.
Sol.
Let Let
Hence C-R-Equation satisfied. |
Example-3: Prove that
|
Sol.
Given that
Since
V=2xy
Now
But
Hence Given that
Since
V=2xy
Now
But
Hence
|
Example-4: Show that polar form of C-R equations are-
|
Sol
. z = x + iy =
U and v are expressed in terms of r and θ. Differentiate it partially w.r.t. r and θ, we get-
By equating real and imaginary parts, we get-
|
Key takeaways
- A function
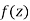 is said to be analytic at a point
is said to be analytic at a point 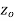 if f is differentiable not only at
if f is differentiable not only at 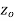 but every point of some neighbourhood at
but every point of some neighbourhood at 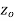 .
. - A point at which the function is not differentiable is called singular point.
- A function which is analytic everywhere is called an entire function.
- If a function is analytic in a domain D, then u and v will satisfy Cauchy-Riemann conditions.
- C-R conditions are necessary but not sufficient for analytic function.
- C-R conditions are sufficient if the partial derivative are continuous
In Cartesian form-
Theorem; The necessary condition for a function 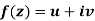 to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
Provided, |
Proof:
Let
Along real axis
Then f’(z), becomes-
Along imaginary axis
From equation (1) and (2)
Equating real and imaginary parts
Therefore-
These are called Cauchy Riemann Equations. |
C-R equation in polar from-
C-R equations in polar form are-
|
Proof:
As we know that- x = r cos z = x + iy = r ( cos
Differentiate (1) partially with respect to r, we get-
Now differentiate (1) with respect to
Substitute the value of
Equating real and imaginary parts, we get-
Proved |
Key takeaways
2. C-R equations in polar form are-
|
Necessary condition for function f(z) to be analytic-
Theorem; The necessary condition for a function 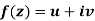 to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
Provided, |
Proof:
Let
Along real axis
Then f’(z), becomes-
Along imaginary axis
From equation (1) and (2)
Equating real and imaginary parts
Therefore-
These are called Cauchy Riemann Equations. |
Sufficient condition for function f(z) to be analytic-
Theorem-
The sufficient conditions of a function f(z) = u + iv to be analytic at all the points in the region R are-
1. 2. |
Proof:
Suppose f(z) be a single-valued function which has-
At each point in the R region, then the Cauchy-Reimann equations are satisfied. By Taylor’s theorem-
Ignore the terms of higher power.
We know that from C-R equations-
Replace We get-
Proved |
Conformal mapping
If the sense of the relation as well as magnitude of the angle is preserved the transformation is said to be conformal.
Note-An analytic function f (z) is conformal everywhere except at its critical points where f (z) = 0.
Example-1: Find the conformal transformation of 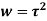 .
.
Answer. Let
|
Theorem: If W=f(z) represents a conformal transformation of a domain D in the z-plane into a domain D of the W plane then f(z) is an analytic function of z in D.
Proof:
We have u+iv=u(x,y)+iv(x,y) So that u=u(x, y) and v=v(x,y) Let ds and
Now Hence Or Where
Now
Where h depends on x and y only and is not zero. Thus the conditions for an isogonal transformation
And The equation are satisfied if we get
Then substituting these values in 2 we get
Taking
Also Hence Similarly
The equation (4) are the well-known Cauchy -Reimann Conformal mapping |
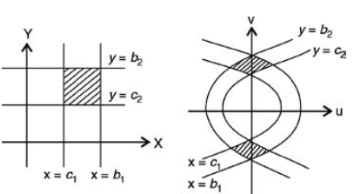
Example: Show that the mapping 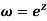 is conformal in the whole of the z plane.
is conformal in the whole of the z plane.
Sol.
Let z=x+iy
Then
|
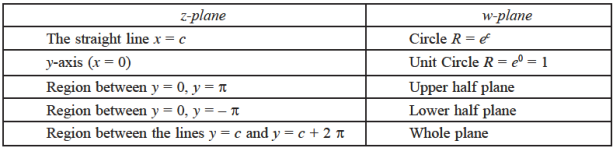
Consider the mapping of the straight-line x=a in z plane the w plane which gives 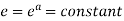 which is a circle in the w plane in the anticlockwise direction similarly the straight-line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight-line y=b is mapped into 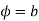 which is a radius vector in the w plane.
which is a radius vector in the w plane.
The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector 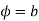 is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping 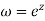 is conformal.
is conformal.
Example: Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
|
Sol.
Let Differentiate (1), we get-
Now-
Differentiate (3), we get-
As we know that for the condition for orthogonallity, from (2) and (4)
So that these two curves cut orthogonally. Here,
And
Here the C-R equation is not satisfied so that the function u + iv is not analytic. Hence the transformation is not conformal. |
Key takeaways
- If the sense of the relation as well as magnitude of the angle is preserved the transformation is said to be conformal.
- An analytic function f (z) is conformal everywhere except at its critical points where f (z) = 0
Transformation:
Sol.
Now equating real and imaginary parts, we get-
Case-1: if
Case-2: if
So that-
Which is a parabola with focus at origin, v = 0 As axis and open to the left. Similarly
So that-
These parabolas are orthogonal to each other. w = z2 is conformal everywhere except at z = 0 where w = 2z = 0.
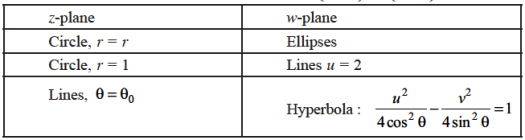
Transformation:
Now equating real and imaginary parts-
Again
Hence
Transformation:
At z = So the transformation is not conformal at z =
Now
|
Bilinear transformation is a correction of backwards difference method.
The bilinear transformation (also known as Tuatn’s method transformation) is defined as substitution:
|
Example 1:
Find the bi-linear transformation which aps points z=2,1,0 ontpo the points w=1,0,i
Sol.
Let
Thus we have
=
|
Example 2:
How that the bilinear transformation
w= |
in the z-plane to 4u+3=0 in w-plane.
Sol.
Consider
Thus, centre of the circle is (h,k) Thus in z-plane it is given as Consider w= W(z-4) = 2z+3 Wz-4w=2z+3 Wz-2z=4w+3 Z(w-2) = (4w+3)
z-2 =
|
References
- E. Kreyszig, “Advanced Engineering Mathematics”, John Wiley & Sons, 2006.
- P. G. Hoel, S. C. Port And C. J. Stone, “Introduction To Probability Theory”, Universal Book Stall, 2003.
- S. Ross, “A First Course in Probability”, Pearson Education India, 2002.
- W. Feller, “An Introduction To Probability Theory and Its Applications”, Vol. 1, Wiley, 1968.
- N.P. Bali and M. Goyal, “A Text Book of Engineering Mathematics”, Laxmi Publications, 2010.
- B.S. Grewal, “Higher Engineering Mathematics”, Khanna Publishers, 2000.
- T. Veerarajan, “Engineering Mathematics”, Tata Mcgraw-Hill, New Delhi, 2010
- Higher engineering mathematics, HK Dass