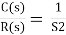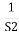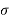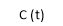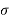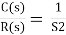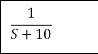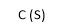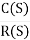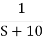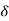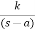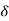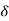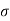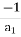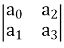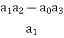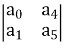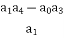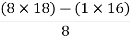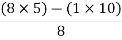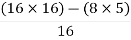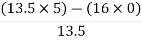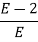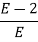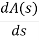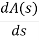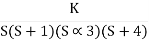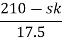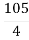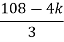A stable system always gives bounded output for bounded input and the system is known as BIBO stable
A linear time invariant (LTI) system is stable if,
The system is BIBO stable
In absence of the input the output tends towards zero
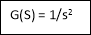
 For system
For system
Fig 1 Control system with G(s) = 1/s2
For R(s) = 1 C(S) =
R (t) =
|
T Bounded Unbounded Fig 2 Input R(t) Fig 3 Input and output for
|
So, system unstable.
[R(s) = 1]
C(t) = e-10t C(t)
|

 t= 0
t= 0
e-10t
t
e-10t C(t)

1
 t
t
0
Fig 4 BIBO stable figure
Effect of location of Poles on stability
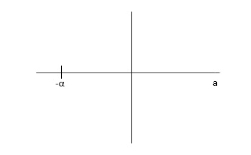
A) Real and negative G(s)= 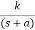
C(t) -a
|
Fig 5 Output for G(s)= 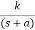
Stable
B) Poles on right half of S- plane
G(s) =
0 t
Unstable: Unbounded Output
|
Fig 6 Input and Output for G(s) = 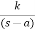
(C)Poles at origin
iw C(t)
t
|
Marginally stable
Fig 7 Input and output for G(s)=
4) Complex poles on left half of S-plane
S1,S2= -α ± iw
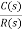 =
= 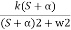
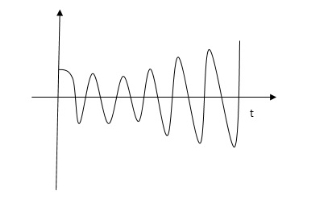
C(t) = k e- αt Cos wt
|
|
Fig 8 Pole location and output for C(t) = k e- αt Cos wt
At t - ∞ C(t) – 0 so, system stable
5) Complex poles on right half of S-plane
S1,S2 = α ± iw.
C(t) = k eαt COS wt.
 C(t) increases exponentially with t ∞
C(t) increases exponentially with t ∞
So, unstable.
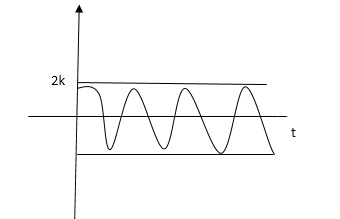
6) Poles on iw axis: S1,S2 = ± iw.
0
|
Fig 9 Output response of C(t) = k COS wt.
The system is marginally stable than sustained oscillations.
Key takeaways
- Consider a system with characteristic equation
aoSm + a1 Sm-1 ……………..am=0.
2. All the coefficients of above equation should have same sign.
3. There should be no missing term.
The above two condition are necessary but not sufficient condition for stability
It states that the system is stable if and only if all the elements is the first column have the same algebraic sign. If all elements are not of the same sign then the number of sign changes of elements in first column equals the number of roots of the characteristic equation in the right half of S-plane.
Consider the following characteristic equation:
Continue the same procedure to find new rows.
a0 Sn + a1 Sn-1 ………….an = 0 where a0,a1,,,,,,,,,,,,,,,,,,,,an have same sign and are non-zero. Step1 Arrange coefficients in rows Row1 ao a2 a4 Row2 a1 a3 a5 Step2 Find third row from above two rows Row1 a0 a2 a4 Row2 a1 a3 a5 Row3 a1 a3 a5 a1 = a3 =
|
Q1) for the given polynomials below determine the stability of the system
S4+2S3+3S2+4S+5=0
1) Arranging Coefficient in Rows
|
For row S2 first term
S2 = 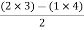 = 1
= 1
For row S2 Second term
S2 = 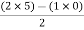 = 5
= 5
For row S1:
S1 = 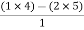 = -6
= -6
For row S0
S0 = 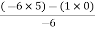 = 5
= 5
As there are two sign change in the first column, So there are two roots or right half of S-plane making system unstable.
Q2. Using Routh criterion determine the stability of the system with characteristic equation S4+8S3+18S2+16S+S = 0
Sol :-
Arrange in rows.
|
For row S2 first element
S1 = Second terms = For S1 First element = For S0 First element =
|
Special Cases of Routh Hurwitz Criterions
- When first element of any row is zero.
In this case the zero is replaced by a very small positive number
E and rest of the array is evaluated.
Eg.(1) Consider the following equation
S3+S+2 = 0
Replacing 0 by E
Now when E
Two sign changes hence two roots on right side of S-plans
|
II) When any one row is having all its terms zero.
When array one row of Routh Hurwitz table is zero, it shown that the X is attests one pair of roots which lies radially opposite to each other in this case the array can be completed by auxiliary polynomial. It is the polynomial row first above row zero.
Consider following example S3 + 5S2 + 6S + 30
For forming auxiliary equation, selecting row first above row hang all terms zero. A(s) = 5S2 e 30
Again forming Routh array
|
No sign change in column one the roots of Auxiliary equation A(s)=5s2+ 30-0
5s2+30 = 0
S2 α 6= 0
S = ± j 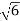
Both lie on imaginary axis so system is marginally stable.
Q3. Determine the stability of the system represent by following characteristic equations using Routh criterion
1) S4 + 3s3 + 8s2 + 4s +3 = 0 2) S4 + 9s3 + 4S2 – 36s -32 = 0 1) S4+3s3+8s2+4s+3=0
No sign change in first column to no rows on right half of S-plane system stable. S4+9S3+4S2-36S-32 = 0
Special case II of Routh Hurwitz criterion forming auxiliary equation A1 (s) = 8S2 – 32 = 0
|
One sign change so, one root lies on right half S-plane hence system is unstable.
Q4. For using feedback open loop transfer function
G(s) = 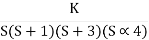
find range of k for stability Soln:- Findlay characteristics equation . CE = 1+G (s) H(s) = 0 H(s) =1 using feedback CE = 1+ G(s) 1+ S(S+1)(S+3)(S+4)+k = 0 (S2+5)(S2+7Sα12)αK = 0 S4α7S3α1252+S3α7S2α125αK = 0 S4+8S3α19S2+125+k = 0 By Routh Hurwitz Criterion
For system to be stable the range of K is 0< K <
|
Q5. The characteristic equation for certain feedback control system is given. Find range of K for system to be stable.
Soln
S4+4S3α12S2+36SαK = 0
For stability K>0
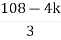 > 0
> 0
K < 27
Range of K will be 0 < K < 27
Relative Stability Criterion:
Routh stability criterion deals about absolute stability of any closed loop system. For relative stability we need to shift the S-plane and the apply the Routh criterion.
|
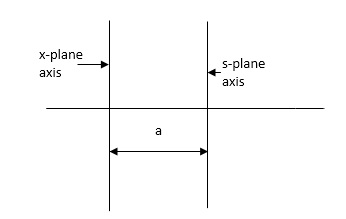
Fig 10 Location of Pole for relative stability
The above fig 10 shows the characteristic equation is modified by shifting the origin of S-plane to S1= -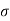 .
.
S = Z-S1
After substituting new valve of S =(Z-S1) applying Routh stability criterion, the number of sign changes in first column is the number of roots on right half of S-plane
Q6. Check if all roots of equation
S3+6S2+25S+38 = 0, have real poll more negative than -1.
Soln:-
No sign change in first column, hence all roots are in left half of S-plane. Replacing S = Z-1. In above equation (Z-1)3+6(Z-1)2+25(Z-1)+38 = 0 Z3+ Z23+16Z+18=0
|
No sign change in first column roots lie on left half of Z-plane hence all roots of original equation in S-domain lie to left half 0f S = -1
Key takeaway
- Special Cases of Routh Hurwitz Criterions
When first element of any row is zero.
In this case the zero is replaced by a very small positive number E and rest of the array is evaluated.
2. When any one row is having all its terms zero.
3. When array one row of Routh Hurwitz table is zero, it shown that the X is attests one pair of roots which lies radially opposite to each other in this case the array can be completed by auxiliary polynomial. It is the polynomial row first above row zero.
References:
1. “Modern Control Engineering “, K. Ogata, Pearson Education Asia/
PHI, 4th Edition, 2002.
2. “Automatic Control Systems”, Benjamin C. Kuo, John Wiley India
Pvt. Ltd., 8th Edition, 2008.
3. “Feedback and Control System”, Joseph J Distefano III et al.,
Schaum’s Outlines, TMH, 2nd Edition 2007.
4. J. Nagarath and M.Gopal, “Control Systems Engineering”, New Age
International (P) Limited, Publishers, Fourth edition – 2005