Module-1

Differential calculus-1

1.1 Review of elementary calculus, curves - angle between the radius vector and tangent

Polar curves-

Angle between the radius vector and tangent-

If 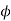be the angle between the radius vector and the tangent at any point of the curve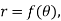

Length of the perpendicular from pole on the tangent-

If p be the perpendicular from the pole on the tangent, then-

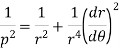
And

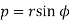
Note-

Polar sub-tangent is-

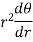
And polar sub-normal is-

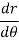
Example: Find out the angle of intersection of the curves

Sol.

We eliminate ‘r’ to find the point of intersection of the curves-

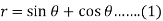
And

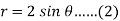
Then-

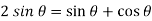
Or

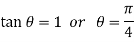
For (1)-

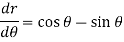
So that-

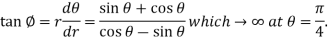
Thus

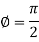
For equation (2)-

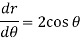
So that-

Thus

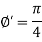
Therefore the angle of intersection of the curves-

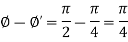
Key takeaways-

1. Polar sub-tangent is-

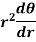
2.     And polar sub-normal is-

1.2 Angle between two curves, pedal equation

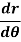
Angle of intersection of two curves-

We follow the procedure given below to find out the angle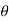

1. Find the point of intersection (P) of the curves by solving their equations.
2. Find the value of dy/dx at P for the two curves (
3. Find angle

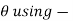
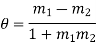
Note- If then the curves cut orthogonally.

Pedal equation-

The relation between ‘p’ and ‘r’ is called pedal equation of the curve where ‘r’ is the radius vector of any point on the curve and ‘p’ be the length of the perpendicular from the pole on the tangent at that point.

Exampe-1: Find the pedal equation of the parabola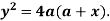

Sol.

Equation of the tangent of the parabola can be written as-

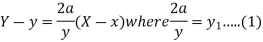
The perpendicular distance of this tangent from (0,0)-

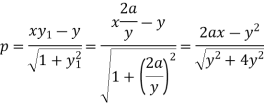
Implying-

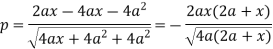
Or

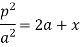
Now-

Or

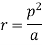
Therefore the pedal equation is-

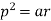
Example: Find the pedal equation of the ellipse-

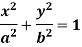
Sol.

Here we have-

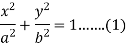
Equation of the tangent at (x, y) is-

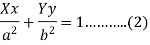
The length of perpendicular ‘p’ from (0, 0) on (2)-

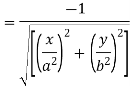
Or

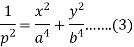
Also-

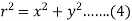
Put the value of 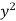from (4) in (1), we get-

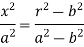
Then from (1)-

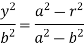
Now put these values of 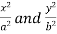in (3), we get-

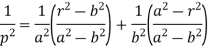
Or

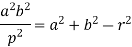
Which is the required pedal equations.

Key takeaways-

1. Angle of intersection of two curves-

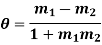
2. 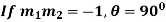      then the curves cut orthogonally.

1.3 Curvature and radius of curvature-Cartesian and polar forms

Curvature 

Let M be any point on the given curve and be its neighborhood.

Let s be an arc of the curve at M   and 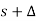s be the arc at 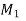shown in the figure and   be an angle made by the tangent at point M and 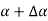  at the point 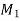of the curve. Then the infinitely small length of the arc M 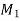i.e. 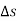 and angle made by the tangent  between them is   .

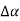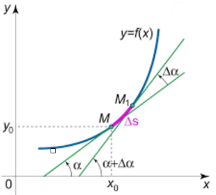
Then the curvature of the curve when M tends to 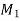is

i.e. the derivative of the angle made by the tangent to the  curve with respect to the arc  at that point.

The unit of curvature is radian per length.

Radius of curvature: The radius of curvature is the reciprocal of the curvature of the curve at the point. It is denoted by .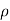

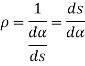
We know that slope is 

Or

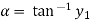
Differentiating both side with respect to x we get,

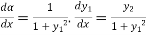
By chain rule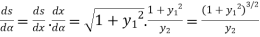

Hence    

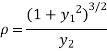
Example-1: Show that for a rectangular hyperbola, ?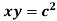

Sol.

Given curve .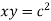

Differentiating both side with respect to x.

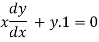
Or    ……(i)

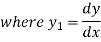
Again differentiating with respect to x.

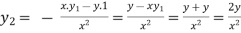using (i)

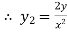….(ii)

The radius of curvature  is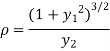
 

Substituting values from (i) and (ii) we get

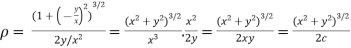by given curve.

Hence 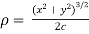is proved.

Example2:

Find the radius of curvature at of the catenary?

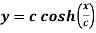Sol.

Given catenary 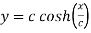    … (i)

Differentiating (i) with respect to x.

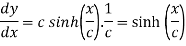
Also consider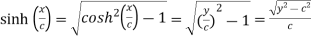

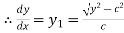….. (ii)

Again differentiating with respect to x , we get

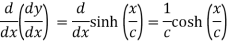
….(iii)

The radius of curvature  is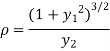
 

Substituting values from (i),(ii) and (iii) we get

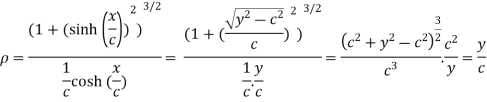
Hence the radius of curvature at 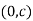is .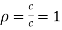

Radius of Curvature in parametric form:

Let the co-ordinates be defined in the form of functions depend on one  independent variable t.

Suppose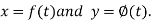

We will calculate the values in term of variables depend on t.

By chain rule we have

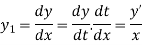
And

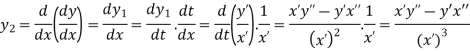
By

Example:

Find the radius of curvature at any point on

1. Ellipse .

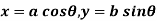The parametric equation of the ellipse is given by

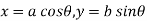
As we know by chain rule

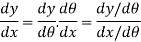
Hence

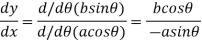
Or

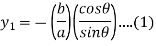
Again by chain rule we have

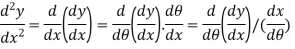
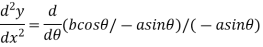
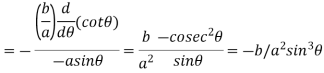
Hence 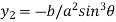…..(ii)

The radius of curvature is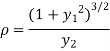
 

Substituting values from (i), and (ii) we get

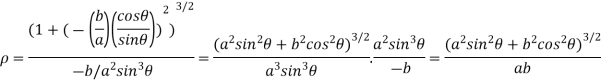
Newton’s formula for radius  of curvature at origin:

1. If x-axis is tangent  to a curve  at the origin, then

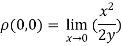
b.     If y-axis is tangent  to a curve  at the origin, then

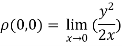
c.      When  the tangent is  at the origin and neither  on x-axis nor on  y-axis then

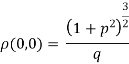
Where.

Note: To find the tangent at the origin of the curve we will equate the lowest degree term in the equation to zero.

Example: Find the radius of curvature at the origin for

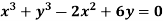
Sol.

Given curve is 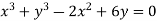  ….(i)

Equating lowest degree term to zero we get

   ..(ii)

Tangent at the origin is on the x-axis.

Dividing (i) by y , we get

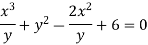
The radius of curvature at the origin on the x-axis is 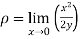and using (ii)

.

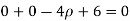
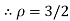Example: Find the radius of curvature at the origin for-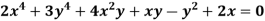
 

Given curve …(i)

Equating lowest degree term to zero we get

   ..(ii)

Tangent at the origin is on the y-axis.

Dividing (i) by x, we get

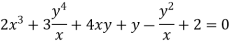
The radius of curvature at the origin on the x-axis is 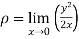and using (ii)

.

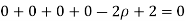
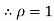Example: Find the radius of curvature at the origin for-

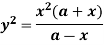
Sol. Given curve

Or 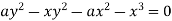…(i)

Equating lowest degree term to zero we get

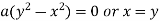   ..(ii)

Tangent at the origin is neither on x-axis nor on y-axis.

Putting in (i), 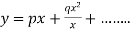where  .

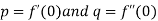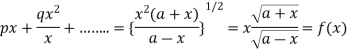
Where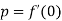

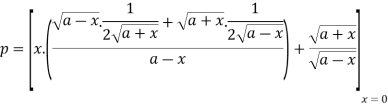
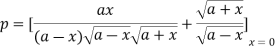
Hence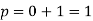

Again

.

When  the tangent is  at the origin and neither  on x-axis nor on  y-axis then

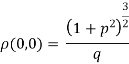
Substituting the values in p and q   we get,

Key takeaways-

1. Radius of curvature: The radius of curvature is the reciprocal of the curvature of the curve at the point. It is denoted by .

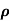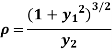
2.     Radius of Curvature in parametric form:

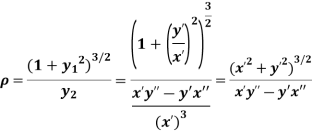
3.       To find the tangent at the origin of the curve we will equate the lowest degree term in the equation to zero.

1.4 Centre and circle of curvature (All without proof-formulae only) -applications to evolutes and involutes. 

Centre of curvature-

Centre of curvature at any point P (x, y) on the curve y = f(x) is given by-

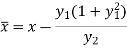
And

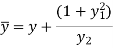
Circle of curvature at P-

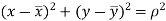
Example: Find the coordinates of the centre of curvature at any point of the cycloid-

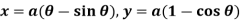
Sol.

Here we have-

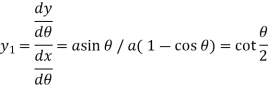
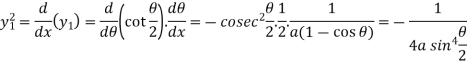
Then centre of curvature-

On putting the above value in formulas, we get-

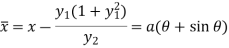
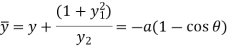
Evolutes and involutes-

Definition- The locus of centres of curvature of a given curve is called the evolute of that curve.

The locus of the center of curvature C of a variable point P on a curve is called the evolute of the curve and the curve itself is called involute of the evolute.

Evolute is nothing but an equation of the curve.

Method to find the evolute-

1. If a curve equation is given and we need to prove left hand side is equal to right hand side (L.H.S = R.H.S), then

First of all find the curvature C(X,Y), where

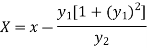
And

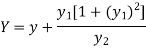
Then take left hand side and put X in place of x and Y in place of y.

2. When the curve is given and we need to find the evolute, then-

Find the curvature first and then re-write as x in terms of X and y in terms of Y and then put in the given curve

Properties of envelope and evolute-

1. The normal at any point of a curve is a tangent to its evolute touching at the corresponding centre of curvature.

2. The evolute is one only but there can be infinite involutes.

3. The difference between the radii of curvature at two points of a curve is equal to the length of the arc of the evolute between the two corresponding points.

Example: Prove that the evolute of parabola is given by

Sol. It is given that-

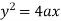
If (X,Y) are the coordinates of the centre of curvature at any point P(x , y) on the curve y = f(x), then X and Y are given as-

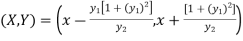  …………….. (1)

Now consider the equation of parabola (given)

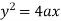
On differentiating w.r.t x-

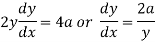
Again differentiating w.r.t.x-

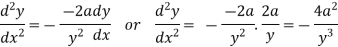
Put these derivatives in (1), we get-

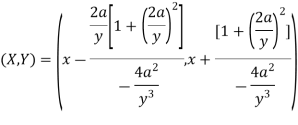
Now consider X,

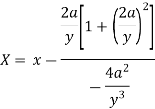
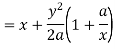
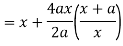
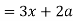
Here we get-

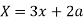
Now consider,

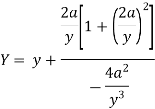
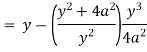
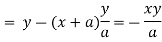
Here we get-

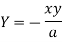
Now

Taking L.H.S of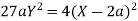

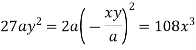
Taking R.H.S of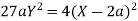

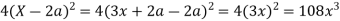
Hence proved.

Example: Find the evolute of the ellipse .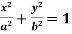

Sol. It is given that-

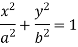
The parametric equations are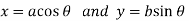

Now,

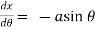and

So that-

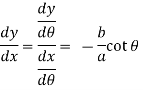
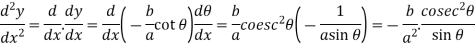
Which gives,

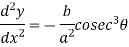
Co-ordinates of centre of curvature are (X , Y),

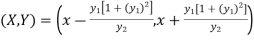  …………….. (1)

Consider X,

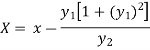
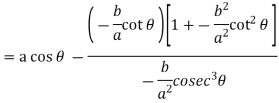
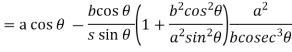
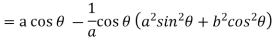
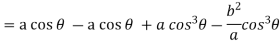
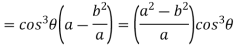
We get-

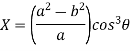
Now consider Y,

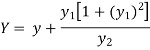
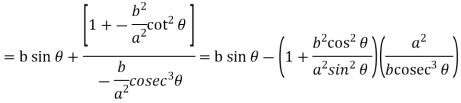
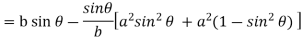
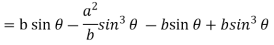
So that we get-

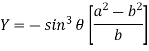
Eliminating 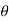from X and Y, we get,

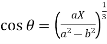and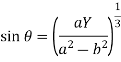
 

We know that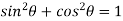
 

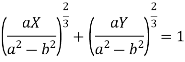
Which gives on solving-

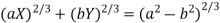
Which is the required evolute.

Envelop of a family of curves-

Let us consider a family of plane curves (single-parameter) which is defined by the following equation-

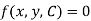
Here C is a parameter.

Then the envelope of this family of curves is a curve such that at every point it touches one of the curves of the family.

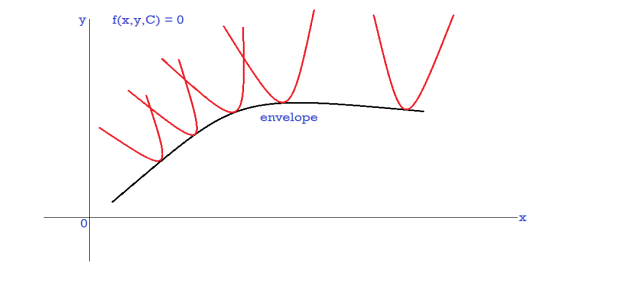
In the figure black curve is an envelope.

Note-

1. Envelope- A curve which touches each member of a family of curves is called envelope of that family of curves.

2. Envelope of a family of curves-

The locus of the ultimate points of interaction of consecutive members of a family of curve is called the envelope of the family of curves.

Example:

Find the envelope of 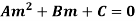, where m is the parameter and A, B and C are function x and y.

Sol. It is given that-

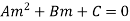   ………………….. (1)

Now differentiate equation (1) with respect to ‘m’,

We get-

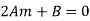, gives

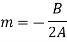
Put these values in equation (1)-

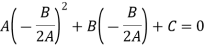
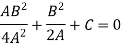
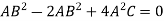
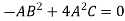
Which gives-

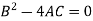
Which is the required envelope.

Example: Find the envelope of the family of 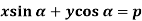, where 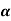is the parameter.

Sol. It is given that-

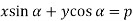   ………….. (1)

Now differentiate equation (1) with respect to ‘’,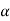

We get-

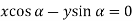   ………………… (2)

Eliminate from both equations-

We get-

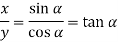
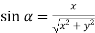                    And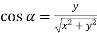               

Put these values in (1) -

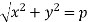
On squaring both sides, we get-

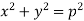
Which is the required envelope.

Example: Find the envelope of the family of straight lines 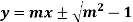, where ‘m’ is the parameter.

Sol. It is given that-

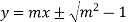
Which can be written as-

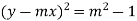
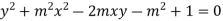
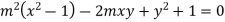, which is a quadratic equation.

Here the value of coefficients of the quadratic equation-

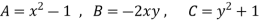
The condition for envelope in case of a quadratic equation is-

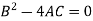
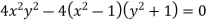
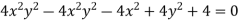
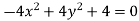
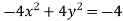
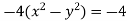
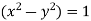
So that the envelope is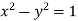

Key takeaways-

1. Centre of curvature-

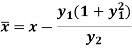
And

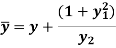
2.     Circle of curvature at P-

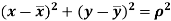
3.     Envelope- A curve which touches each member of a family of curves is called envelope of that family of curves.

4.     Envelope of a family of curves-

The locus of the ultimate points of interaction of consecutive members of a family of curve is called the envelope of the family of curves.

References:

• C.Ray Wylie, Louis C.Barrett : "Advanced Engineering Mathematics", 6th Edition, 2. McGraw-Hill Book Co., New York, 1995.
• James Stewart : “Calculus -Early Transcendentals”, Cengage Learning India Private Ltd., 2017.
• B.V.Ramana: "Higher Engineering Mathematics" 11th Edition, Tata McGraw-Hill, 2010.
• Srimanta Pal & Subobh C Bhunia: “Engineering Mathematics”, Oxford University Press, 3rd Reprint, 2016.
• Gupta C.B., Singh S.R. And Mukesh Kumar: “Engineering Mathematics for Semester I & II”, Mc-Graw Hill Education (India) Pvt.Ltd., 2015.