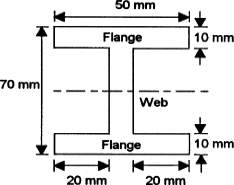
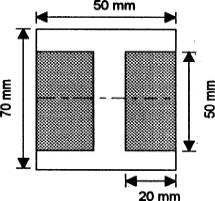
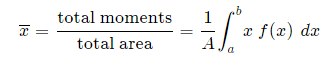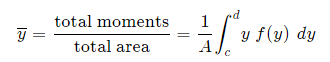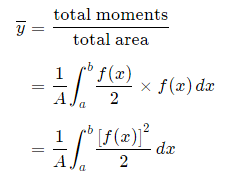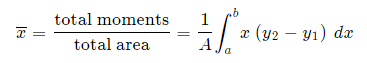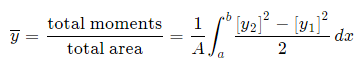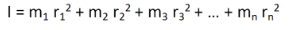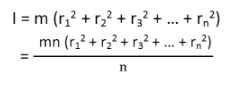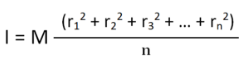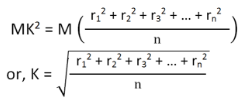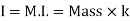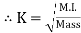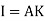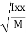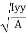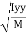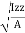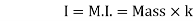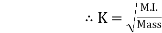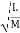|
|
|
|
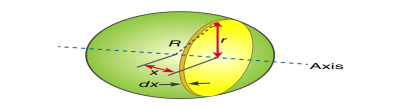
Shapes | Figure | x̄ | ȳ | Area |
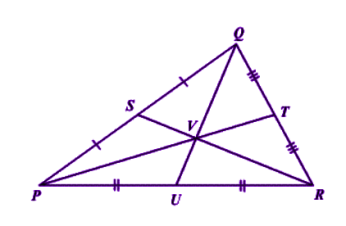
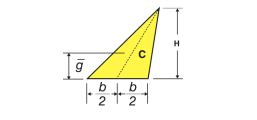
Triangular area |
| – | h/3 | bh/2 |
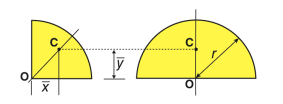
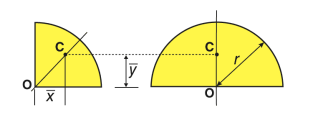
Quarter-circular area |
| 4r/3π | 4r/3π | πr2/4 |
Semi-circular area |
| 0 | 4r/3π | πr2/2 |
Quarter-elliptical area |
| 4a/3π | 4b/3π | πab/4 |
Semi elliptical area |
| 0 | 4b/3π | πab/2 |
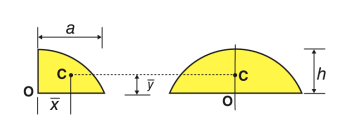
Semi parabolic area |
| 3a/8 | 3h/5 | 2ah/3 |
Parabolic area |
| 0 | 3h/5 | 4ah/3 |
Parabolic spandrel |
| 3a/4 | 3h/10 | ah/3 |
|
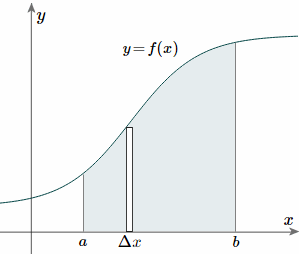
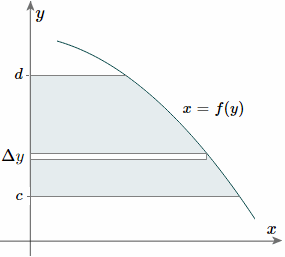
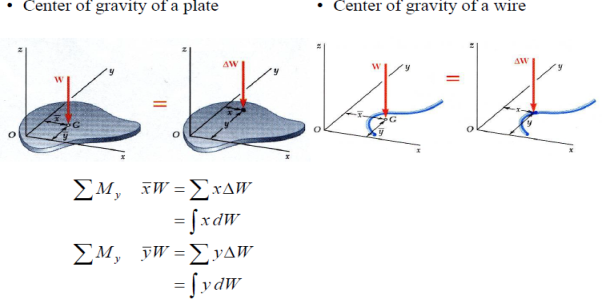
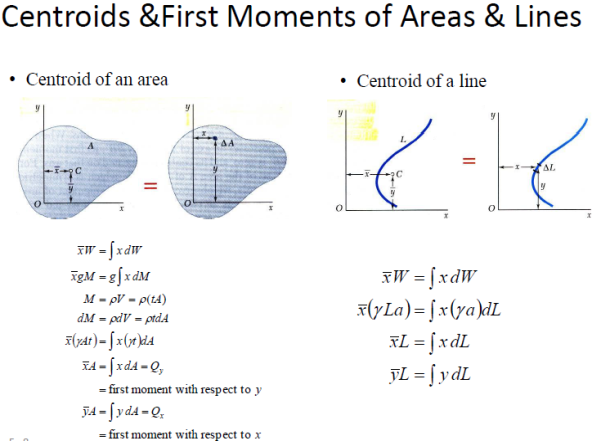
And, considering the moments in the y-direction about the x-axis and re-expressing the function in terms of y, we have:
|
|
|
|
|
|
|
|
|
|
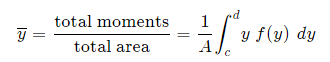
|
|
|
|
Then,
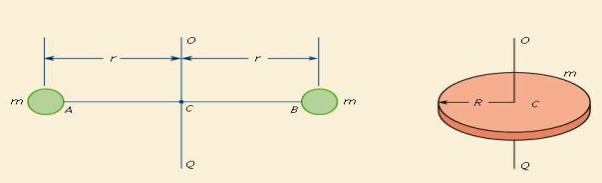
Distance from A’B’ = r + hI = ∑m (r + h)2I = ∑m (r2 + h2 + 2rh)I = ∑mr2 + ∑mh2 + ∑2rhI = Ic + h2∑m + 2h∑mrI = Ic + Mh2 + 0I = Ic + Mh2Hence, the above is the formula of parallel axis theorem.
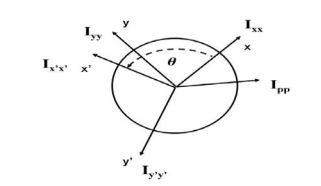
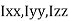 be the moments of inertia of figure or plane section about two axis x and y which are perpendicular to each other at point O, then moment of Inertia of that plane section about Z axis which is perpendicular to the plane [&(
be the moments of inertia of figure or plane section about two axis x and y which are perpendicular to each other at point O, then moment of Inertia of that plane section about Z axis which is perpendicular to the plane [&(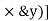 is given by :
is given by :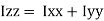
|
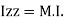 of plane area about Z axis passing through C.G.
of plane area about Z axis passing through C.G.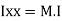 of plane area about X axis passing through C.G
of plane area about X axis passing through C.G of plane area about Y axis passing through C.G
of plane area about Y axis passing through C.G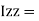 Is also called as polar moment of Inertia (J) Key takeaways:
Is also called as polar moment of Inertia (J) Key takeaways:1) Parallel Axis Theorem Formula
I = Ic + Mh22)If all the particles have the same mass then equation (3) becomes
We can write mn as M which signifies the total mass of the body. Now the equation becomes
………… (4) From equation (4), we get
From the above equation, we can infer that the radius of gyration can also be defined as the root-mean-square distance of various particles of the body from the axis of rotation. What is the use of radius of gyration? The radius of gyration is used to compare how various structural shapes will behave under compression along an axis. It is used to predict buckling in a compression beam or member. OR If entire mass of body be assumed to be concentrated at a certain point which is located at a distance K from given axis such that
Then Distance K is known as Radius of Gyration Thus Radius of Gyration is defined as the Distance from the axis of reference where whole mass of the body is assumed to be concentrated For plane figure having negligible mass, we can consider area for finding radius of gyration Thus M.I. of an area (plane figure)
Key takeaways: 1) Distance K is known as Radius of Gyration
Then 2) Radius of Gyration is defined as the Distance from the axis of reference where whole mass of the body is assumed to be concentrated
|
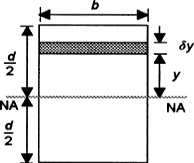
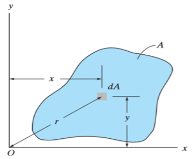
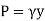 , where,
, where, 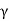 is the specific weight of fluid.The force acting on a acting on the differential area of the plate is given by
is the specific weight of fluid.The force acting on a acting on the differential area of the plate is given by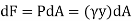 The moment of this this force about the x-axis is
The moment of this this force about the x-axis is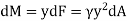 Integrating
Integrating 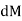 over the entire area of the plate yields
over the entire area of the plate yields 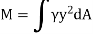
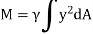 The integral part of the moment equation is called area moment of inertia about x-axis
The integral part of the moment equation is called area moment of inertia about x-axis 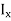
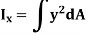 Similarly, area moment of inertia about y-axis is given as,
Similarly, area moment of inertia about y-axis is given as,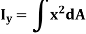
|
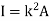 Where, A is the total areaAnd k is referred as radius of gyrationRadius of gyration for area of a plate about an axis is defined as the radial distance to a point which would have a same moment of inertia as the plate’s actual distribution of area, if the total area of the plate were concentrated.It is given by,
Where, A is the total areaAnd k is referred as radius of gyrationRadius of gyration for area of a plate about an axis is defined as the radial distance to a point which would have a same moment of inertia as the plate’s actual distribution of area, if the total area of the plate were concentrated.It is given by,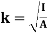
|
|
|
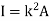
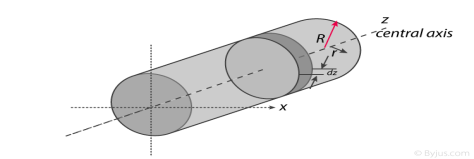
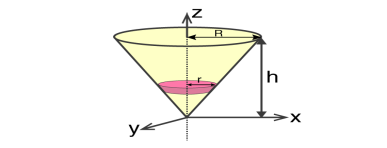
2) Moment of inertia of Solid Cone = I = 3/10 MR2
3) Moment Of Inertia of Sphere = I = ⅖ MR2
|
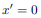 then, we will have
then, we will have  and
and 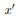 will be a principal axis. This can be shown by looking at the definition of the products of inertia.
will be a principal axis. This can be shown by looking at the definition of the products of inertia.
|
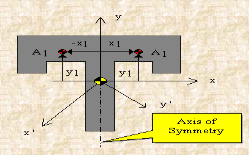
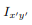 can be decomposed into two integrals for the two halves of the body at either side of the plane
can be decomposed into two integrals for the two halves of the body at either side of the plane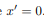 . The integrand on one half
. The integrand on one half 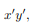 will be equal in magnitude and opposite in sign to the integrand on the other half (because
will be equal in magnitude and opposite in sign to the integrand on the other half (because  will change sign). Therefore, the integrals over the two halves will cancel each other and the product of inertia
will change sign). Therefore, the integrals over the two halves will cancel each other and the product of inertia 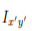 will be zero. (As will the product of inertia
will be zero. (As will the product of inertia  ) Also, if the body is symmetric with respect to two planes passing through the center of mass which are orthogonal to the coordinate axis, then the tensor of inertia is diagonal, with
) Also, if the body is symmetric with respect to two planes passing through the center of mass which are orthogonal to the coordinate axis, then the tensor of inertia is diagonal, with 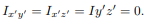
|
|