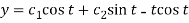Question Bank

Unit–2

Linear differential equations of higher order

Question-1: Solve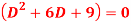

Sol.

Its auxiliary equation is-

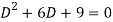
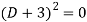
Where-

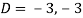
Therefore the complete solution is-

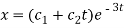
Question-2: Find the P.I. Of (D + 2)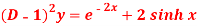

Sol.

P.I. =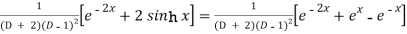

Now we will evaluate each term separately-

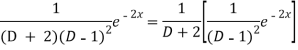
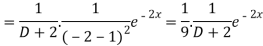
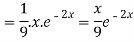
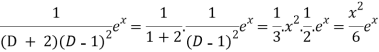
And

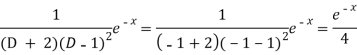
Therefore-

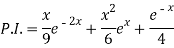
Question-3: Solve (D – D’ – 2 ) (D – D’ – 3) z =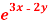

Sol.

The C.F. Will be given by-

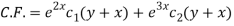
Particular integral-

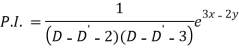
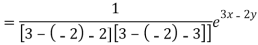
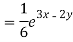
Therefore the complete solution is-

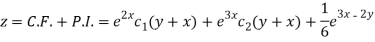
Question-4: Find the P.I. Of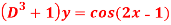

Sol.

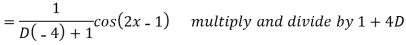
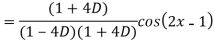
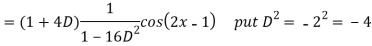
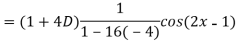
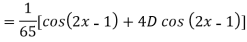
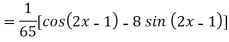
Question-5: Find the P.I. Of (D + 1) (D + D’ – 1)z = sin (x + 2y)

Sol.

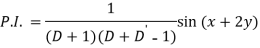
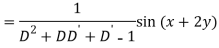
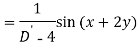
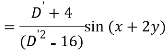
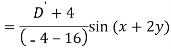
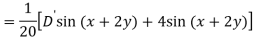
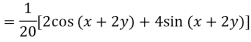
Question-6: Find P.I. Of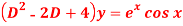

Sol. P.I =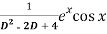

Replace D by D+1

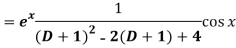
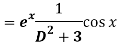
Put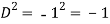

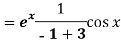
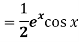
Question-7: Find P.I. Of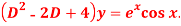

Sol.

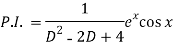
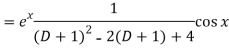
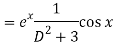
Put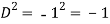
 

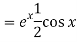
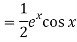
Question-8: Solve-

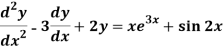
Sol.

The given equation can be written as-

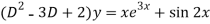
Its auxiliary equation is-

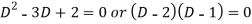
We get-

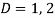
So that the C.F. Will be-

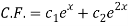
Now we will find P.I.-

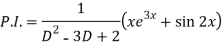
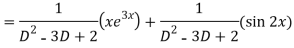
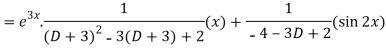
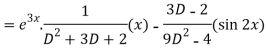
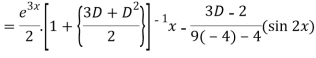
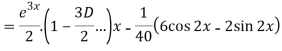
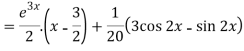
Therefore the complete solution is-

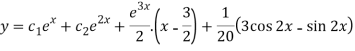
Question-9: Solve the following DE by using variation of parameters-

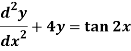
Sol. We can write the given equation in symbolic form as-

To find CF-

It’s A.E. Is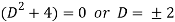

So that CF is-     

To find PI-

Here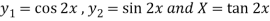

Now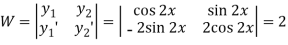

Thus PI =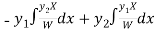 

            = 

            = 

             = 

             = 

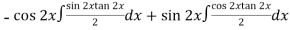
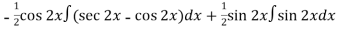
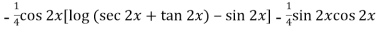
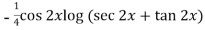
So that the complete solution is-

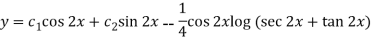
Question-10: Solve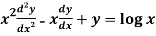

Sol. As it is a Cauchy’s homogeneous linear equation.

Put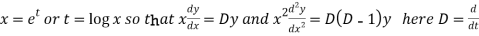

Then the equation becomes [D(D-1)-D+1]y = t   or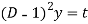

Auxiliary equation-

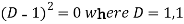
So that-

   C.F.=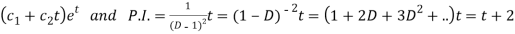

Hence the solution is- 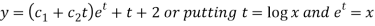, we get-

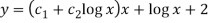
Question-11: Solve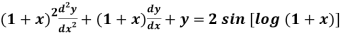

Sol. As we see that this is a Legendre’s linear equation.

Now put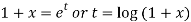

So that-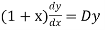

And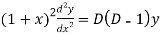

Then the equation becomes- D (D – 1)y+ Dy + y = 2 sin t

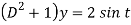
Its auxiliary equation is-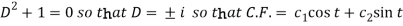

And particular integral-

               P.I. =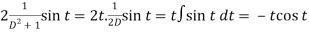

Note -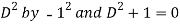

Hence the solution is -