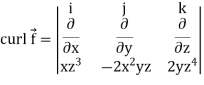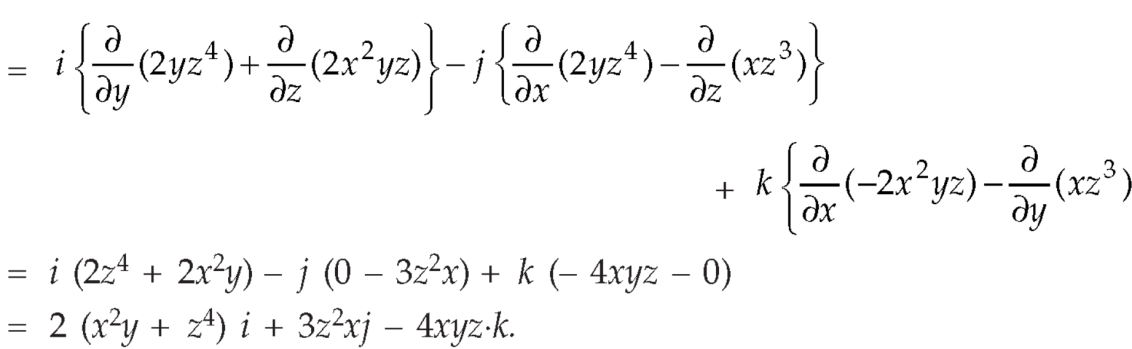Unit – 3
Multivariable calculus: Differentiation
Q1) Evaluate
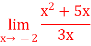
A1)
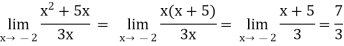
Q2) Evaluate
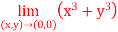
A2)
1. 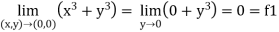
2. 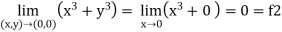
Here f1 = f2
3. Now put y = mx, we get
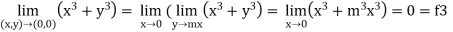
Here f1 = f2 = f3
Now put y = mx²
4. 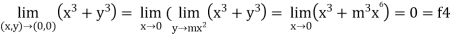
Therefore,
F1 = f2 = f3 =f4
We can say that the limit exists with 0.
Q3) Calculate 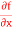 and
and  for the following function
for the following function
f (x, y) = 3x³-5y²+2xy-8x+4y-20
A3) To calculate 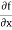 treat the variable y as a constant, then differentiate f (x, y) with respect to x by using differentiation rules,
treat the variable y as a constant, then differentiate f (x, y) with respect to x by using differentiation rules,
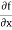 =
= 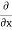 [3x³-5y²+2xy-8x+4y-20]
[3x³-5y²+2xy-8x+4y-20]
= 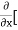 3x³] -
3x³] - 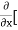 5y²] +
5y²] + 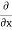 [2xy] -
[2xy] -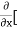 8x] +
8x] +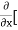 4y] -
4y] - 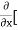 20]
20]
= 9x² - 0 + 2y – 8 + 0 – 0
= 9x² + 2y – 8
Similarly partial derivative of f (x, y) with respect to y is:
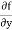 =
= 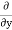 [3x³-5y²+2xy-8x+4y-20]
[3x³-5y²+2xy-8x+4y-20]
= 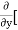 3x³] -
3x³] - 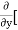 5y²] +
5y²] + 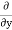 [2xy] -
[2xy] -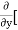 8x] +
8x] +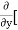 4y] -
4y] - 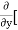 20]
20]
= 0 – 10y + 2x – 0 + 4 – 0
= 2x – 10y +4.
Q4) If 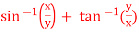 , then show that-
, then show that- 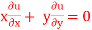
A4) Here we have,
u = 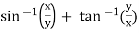 …………………. (1)
…………………. (1)
now partially differentiate eq. (1) w.r.t to x and y, we get

= 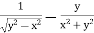
Or
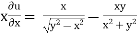 ………………. (2)
………………. (2)
And now,
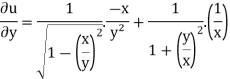
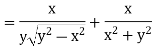
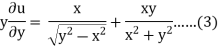
Adding eq. (1) and (3), we get
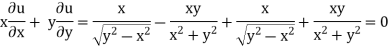
Hence proved.
Q5) Find the directional derivative of 1/r in the direction  where
where 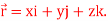
A5) Here 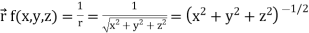
Now,
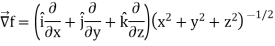
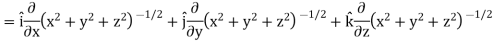
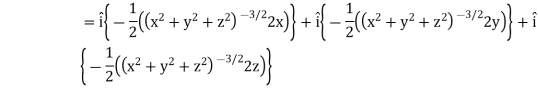
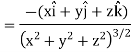
And 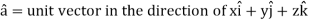
We know that-
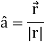
So that-
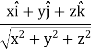
Now,
Directional derivative-
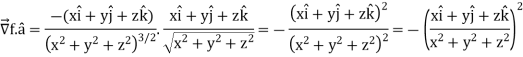
Q6) Find the directional derivative of 1/r in the direction  where
where 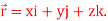
A6) Here 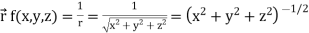
Now,
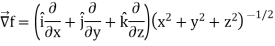
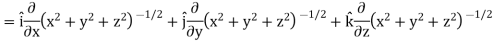
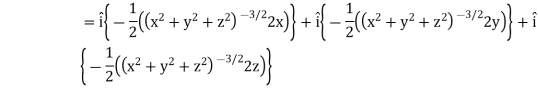
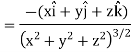
And 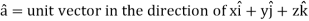
We know that-
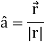
So that-
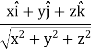
Now,
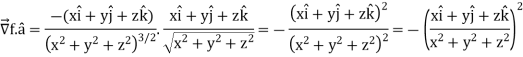
Q7) Find the directional derivative of
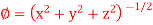
At the points (3, 1, 2) in the direction of the vector  .
.
A7) Here it is given that-
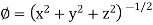
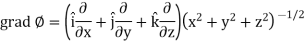
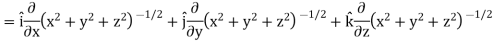
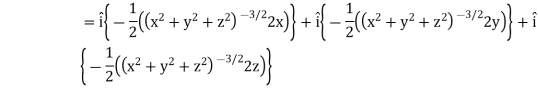

Now at the point (3, 1, 2)-
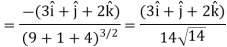
Let 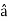 be the unit vector in the given direction, then
be the unit vector in the given direction, then
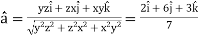 at (3, 1, 2)
at (3, 1, 2)
Now,
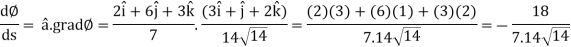
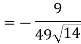
Q8) Find  if u = x³y⁴ where x = t³ and y = t².
if u = x³y⁴ where x = t³ and y = t².
A8) As we know that by definition,  =
= 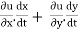
 3x²y⁴3t² + 4x³y³2t = 17t¹⁶.
3x²y⁴3t² + 4x³y³2t = 17t¹⁶.
Q9) If z is the function of x and y , and x =  , y =
, y = 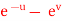 , then prove that,
, then prove that,
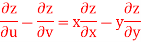
A9) Here , it is given that, z is the function of x and y & x , y are the functions of u and v.
So that,
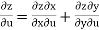 ……………….(1)
……………….(1)
And,
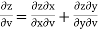 ………………..(2)
………………..(2)
Also there is,
x = 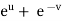 and y =
and y =  ,
,
Now,
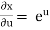 ,
, 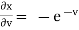 ,
, 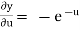 ,
, 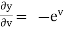
From equation(1) , we get
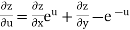 ……………….(3)
……………….(3)
And from eq. (2) , we get
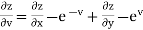 …………..(4)
…………..(4)
Subtracting eq. (4) from (3), we get
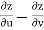 =
= 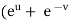 )
)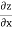 – (
– (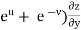
= x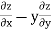
Hence proved.
Q10) If 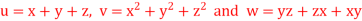 then prove that grad u, grad v and grad w are coplanar.
then prove that grad u, grad v and grad w are coplanar.
A10)
Here- 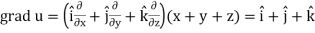
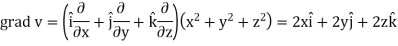
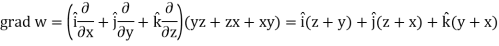
Now-
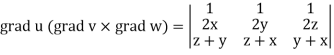
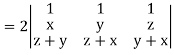
Apply 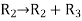
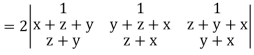
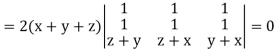
Which becomes zero.
So that we can say that grad u, grad v and grad w are coplanar vectors.
Q12) If 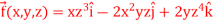 then find the divergence and curl of
then find the divergence and curl of 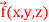 .
.
A12)
We know that-
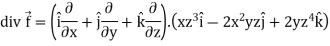
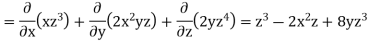
Now-