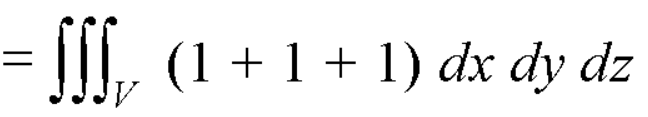Unit – 4

Multivariable calculus: Integration

Q1) Evaluate , where dA is the small area in xy-plane.

A1)     Let, I =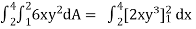 

=

= 

= 

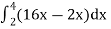
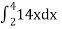
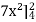
= 84 sq. unit.

which is the required area.

Q2) Evaluate the following double integral,

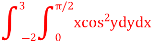
A2) Let,

I =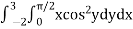 

On solving the integral, we get

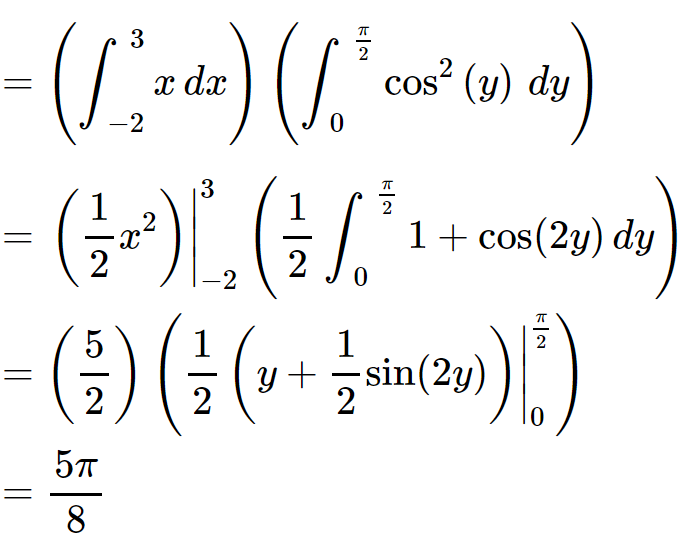
Q3) Evaluate

A3) Let the integral,

 I =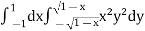

=

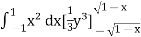
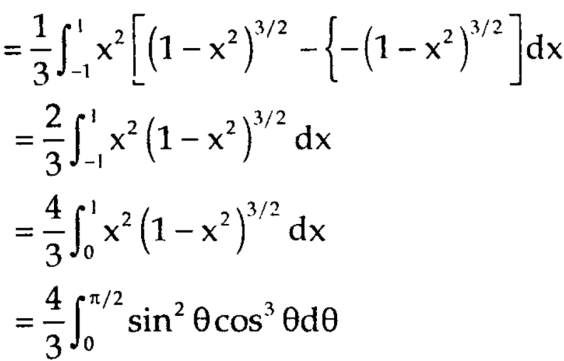
Put x = sinθ

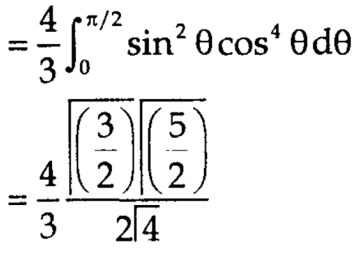
= π / 24   ans.

Q4) Evaluate

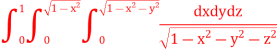
A4) Let

I     =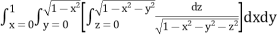    

=    

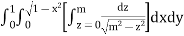
(Assuming m = )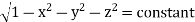

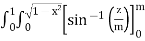= dxdy

=

=

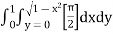
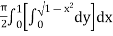
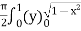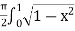= dx

=  dx

= 

=

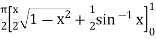
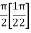
I =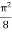

Q5) Find Volume of the tetrahedron bounded by the co-ordinate’s planes and the plane

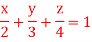
A5) Volume =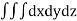                                                 ………. (1)   

Put          ,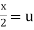  

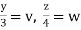
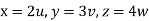
  From     equation (1) we have

   V =

=24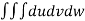

=24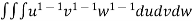  (u+v+w=1) By Dirichlet’s theorem.

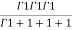
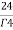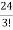=24

=  == 4

Volume =4

Q6)

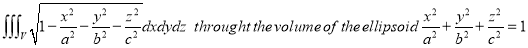
A6) -   Put

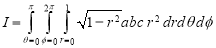
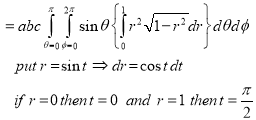
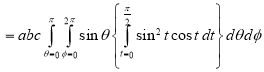
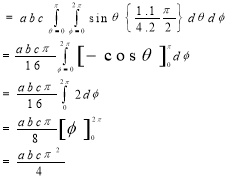
Q7) Express as single integral and evaluate dy dx + dy dx.

A7) 

Given: I  =  dy dx + dy dx

I  =  I1 + I2

The limits of region of integration I1 are

x = – ; x =  and y = 0, y = 1 and I2 are x = – 1,

x = 1 and y = 1, y = 3.

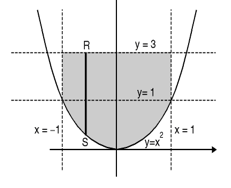
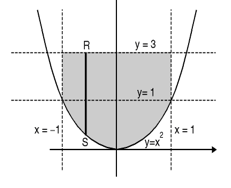
The region of integration is as shown in Fig.

To consider the complete region take a vertical strip SR along the strip y varies from y = x2 to

y = 3 and x varies from x = –1 to x = 1. Fig.

I =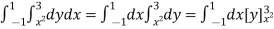

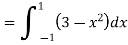
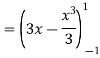
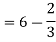
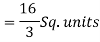
Q8) Evaluate-

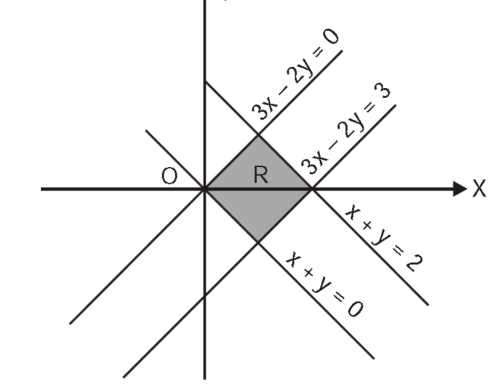
Where- R is the region bounded by a parallelogram- x + y = 0, x + y = 2, 3x – 2y = 0, 3x – 2y = 3.

A8)

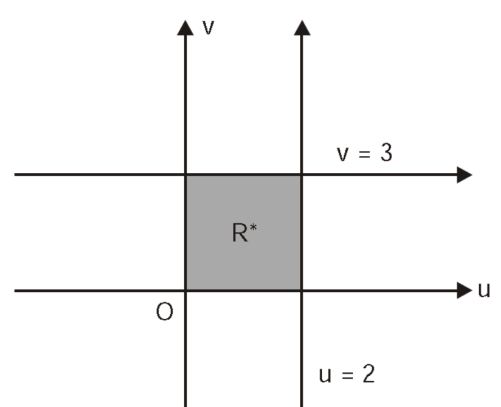
By changing the variables x, y to the new variables u and v, by the substitution x + y = u, 3x – 2y = v, the given parallelogram R reduces to a rectangle

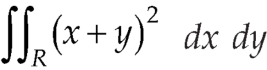
The required Jacobian is-

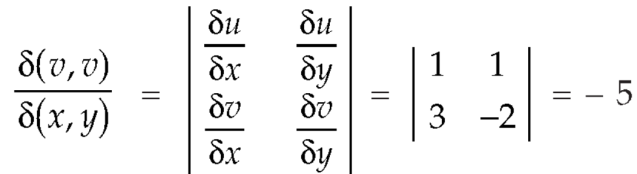
Since u = x + y and u = x + y = 2, here u varies from 0 to 2  while v varies from 0 to 3.

Since-

3x – 2y = v = 0, 3x – 2y = v = 3

Therefore, the integral will be in new variables-

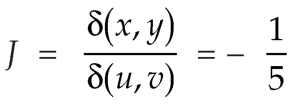
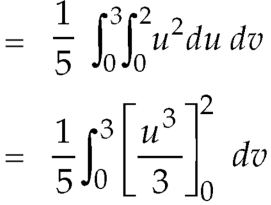
which is the required answer.

Q9) Evaluate 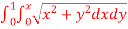the transformation is x = v and y = uv.

A9) Here the region of integration R is the triangle which is bounded by y = 0, x = 1 and y = x

Here put,

X = u and y = uv, we get-

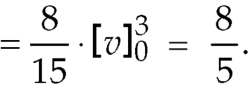
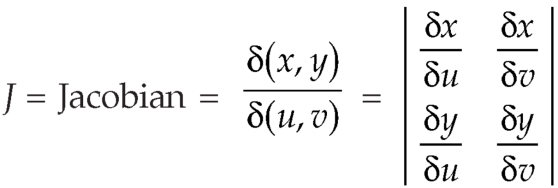
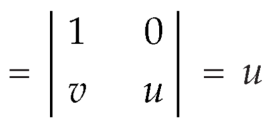
Here x varies from 0 to 1 while y varies from 0 to x.

Since u = x so u varies from 0 to 1

Here, similarly, since , so that v varies from 0 to 1. Thus-

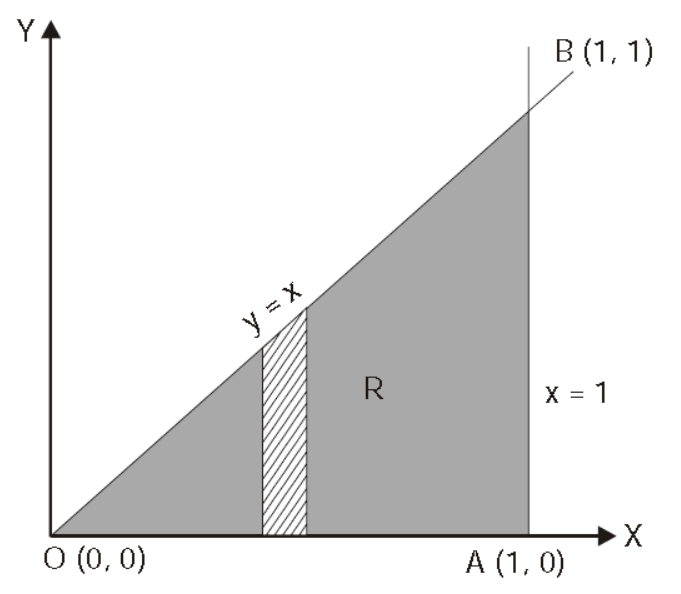
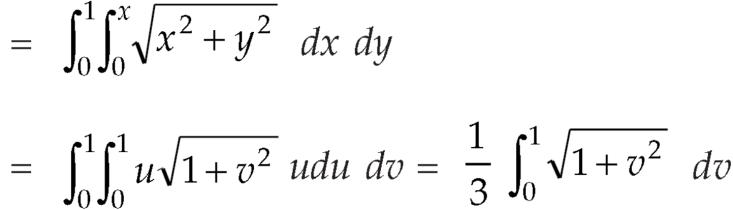
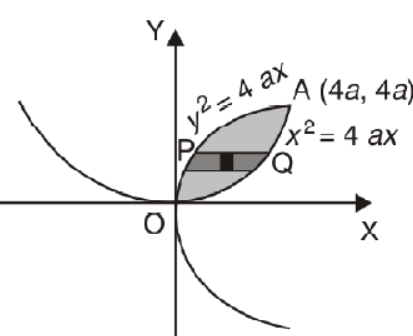
Q10) Find the area between the parabola y ² = 4ax and another parabola x² = 4ay.

A10) Let,

y² = 4ax ………………... (1)

and

x² = 4ay…………………. (2)

then if we solve these equations, we get the values of points where these two curves intersect

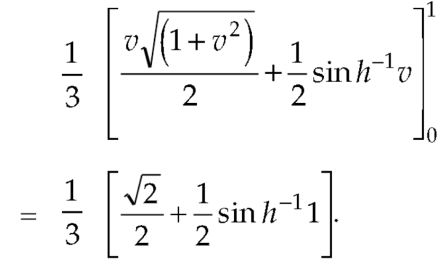
x varies from y²/4a to 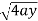and y varies from o to 4a,

Now using the concept of double integral,

Area =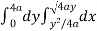 

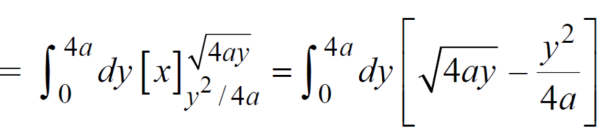
Q11) Apply Green’s theorem to evaluate 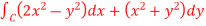where C is the boundary of the area enclosed by the x-axis and the upper half of circle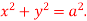

A11) We know that by Green’s theorem-

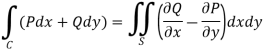
And it given that-

Now comparing the given integral-

P =   and   Q =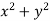

Now-

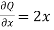and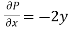

So that by Green’s theorem, we have the following integral-

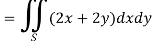
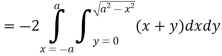
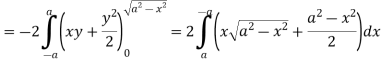
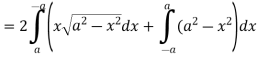
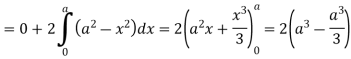
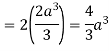
Q12) Verify green’s theorem in xy-plane for 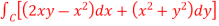where C is the boundary of the region enclosed by

A12) On comparing with green’s theorem,

We get-

P = 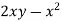and Q =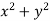

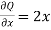and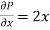

By using Green’s theorem-

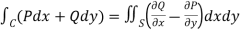  ………….. (1)

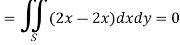
And left-hand side=

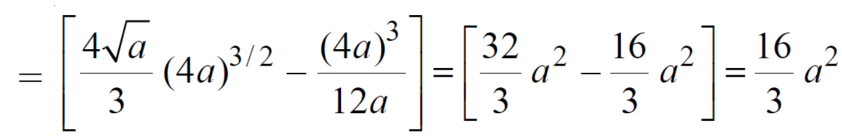………….. (2)

Now,

Along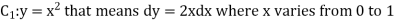

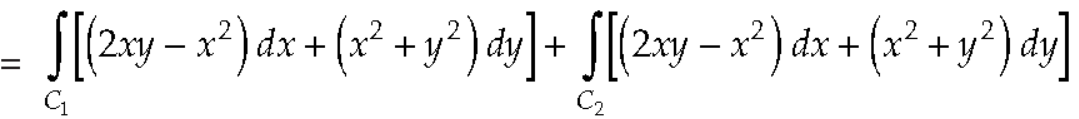
Along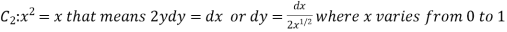

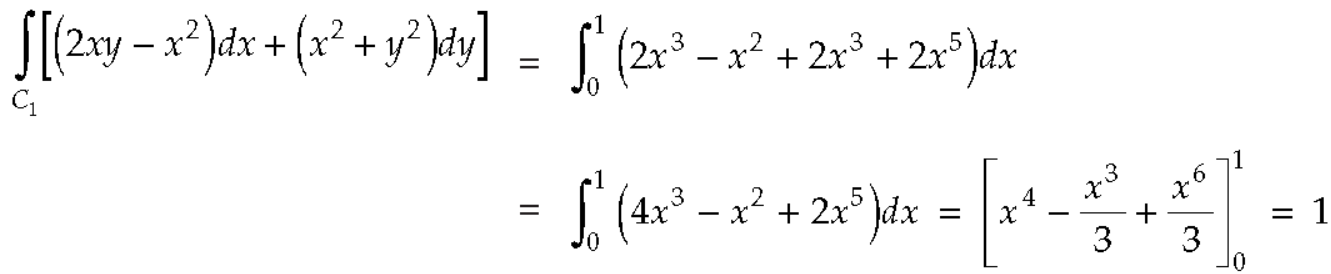
Put these values in (2), we get-

L.H.S. = 1 – 1 = 0

So that the Green’s theorem is verified.

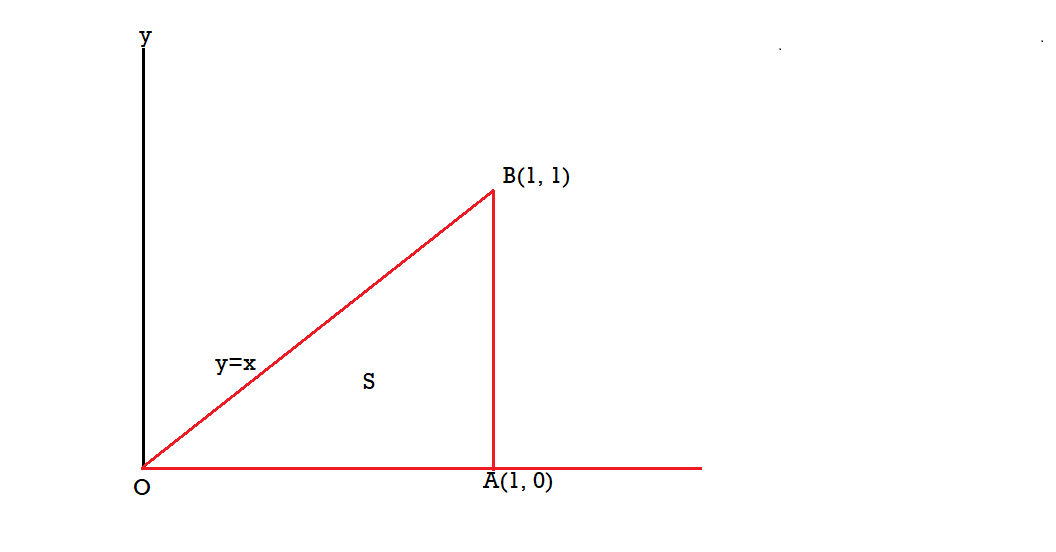
Q13) If 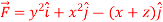and C is the boundary of the triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0), then evaluate 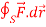by using Stoke’s theorem.

A13) Here we see that z-coordinates of each vertex of the triangle is zero, so that the triangle lies in the xy-plane and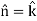

Now,

Curl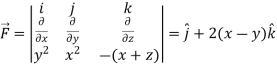

Curl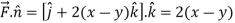

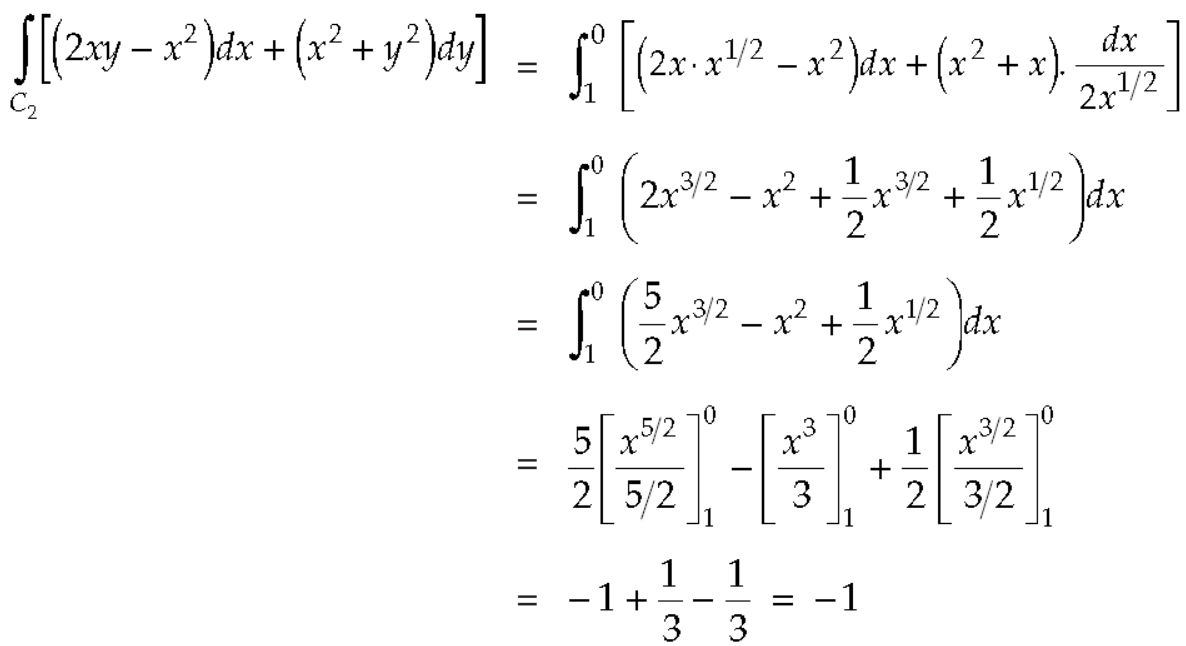
The equation of the line OB is y = x

Now by stoke’s theorem,

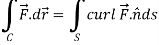
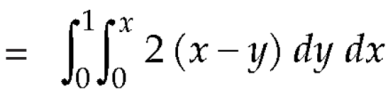
Q14) Show that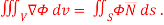 

A14) By divergence theorem, 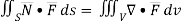 ...… (1)

Comparing this with the given problem let 

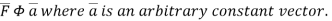
Hence, by (1)

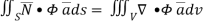                                    …………. (2)

Now,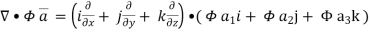

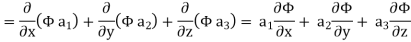
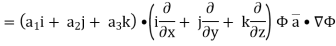
Hence, from (2), We get,

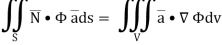
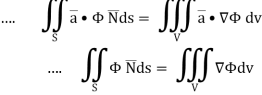
Q15) By using divergence theorem to evaluate-

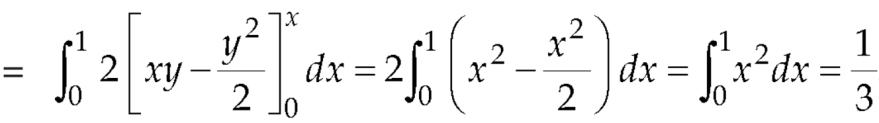
Where-

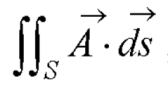
And S is the surface of the sphere .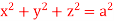

A15)

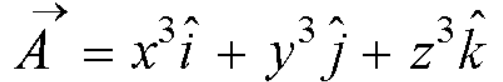
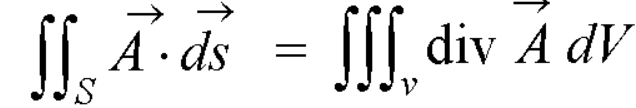
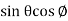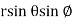On putting x = , y = , z = 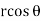, we get-

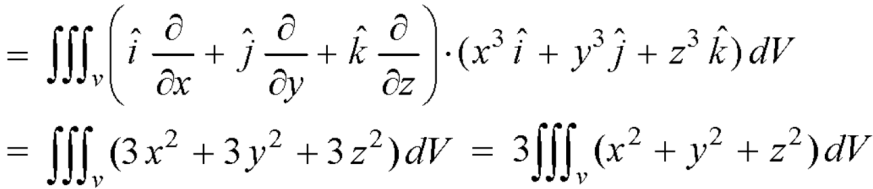
Q16) Use the divergence theorem to evaluate-

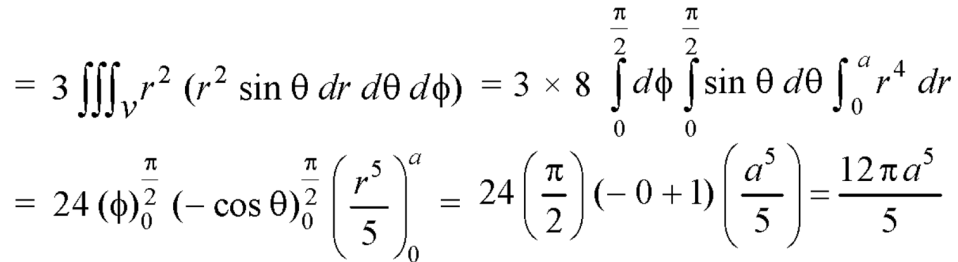
Over the surface of a sphere of radius ‘a’.

A16)

Here we have-

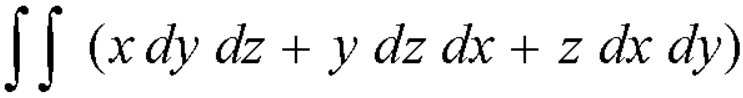
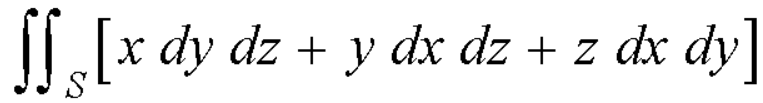
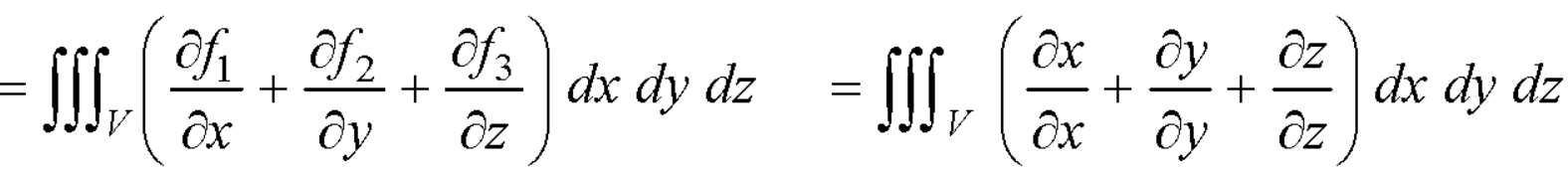
= 3 × Volume of the sphere