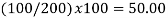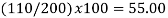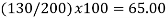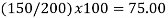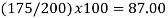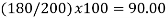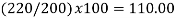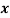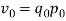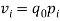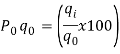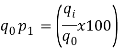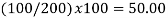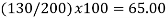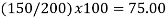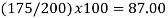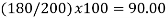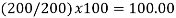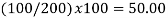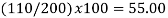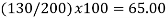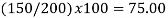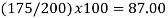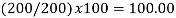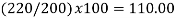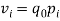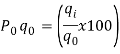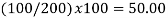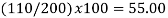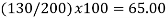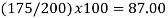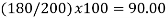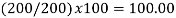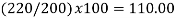Unit -VI

Index numbers

1. What is Index Number?

Answer - Index numbers are intended to measure the degree of economic changes over time. These numbers are values stated as a percentage of a single base figure. Index numbers are important in economic statistics. In simple terms, an index (or index number) is a number displaying the level of a variable relative to its level (set equal to 100) in a given base period.

2. What are the different types of Index Numbers?

Types of Index Numbers

Simple Index Number: A simple index number is a number that measures a relative change in a single variable with respect to a base. These type of Index numbers are constructed from a single item only. This ratio is then finally converted to a percentage 100 Value in Base Year Value in Period Index for any Period x i i = i.e. Simple Price Index for period i = 1,2,3 ... Will be 100 0 0 x p pP i i = …………(6-1) Similarly, Simple Quantity Index for period i = 1,2,3 ... Will be 100 0 0 x q qQ i i = …………(6-2)   Composite Index Number: A composite index number is a number that measures an average relative change in a group of relative variables with respect to a base. A composite index number is built from changes in a number of different items.   Price index Numbers: Price index numbers measure the relative changes in prices of a commodity between two periods. Prices can be either retail or wholesale. Price index number is useful to comprehend and interpret varying economic and business conditions over time. Quantity Index Numbers: These types of index numbers are considered to measure changes in the physical quantity of goods produced, consumed or sold of an item or a group of items.   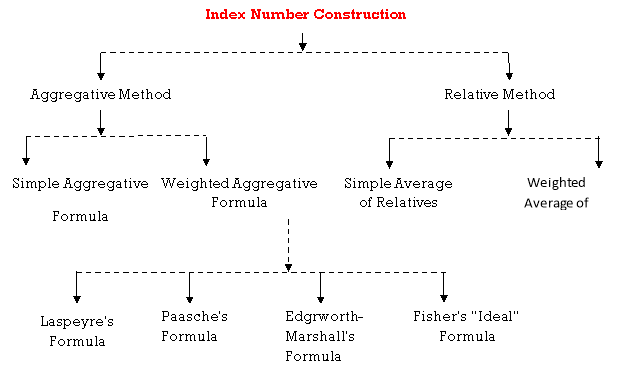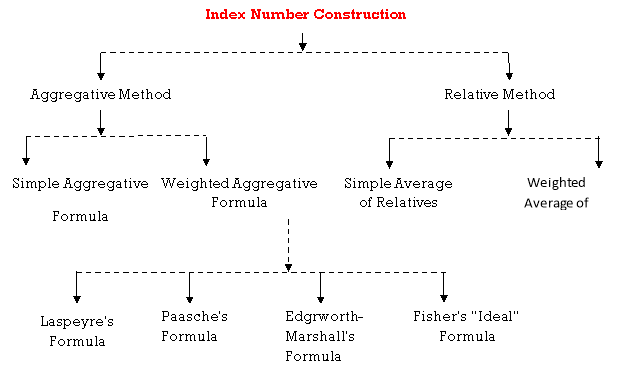Methods of constructing index numbers: There are two methods to construct index numbers: Price relative and aggregate methods (Srivastava, 1989)

In aggregate methods, the aggregate price of all items in a given year is expressed as a percentage of same in the base year, giving the index number.

Weighted aggregative index numbers: These index numbers are the simple aggregative type with the fundamental difference that weights are assigned to the various items included in the index.

3. State the characteristics of Index Numbers.

Characteristics of index numbers:

1. Index numbers are specialised averages.
2. Index numbers measure the change in the level of a phenomenon.
3. Index numbers measure the effect of changes over a period of time.

Index Numbers

4.     Explain problems in the construction of Price Index Number.

Answer -

BASE SHIFTING. The need for shifting the base may arise either

(i) when the base period of a given index number series is to be made more recent, or

(ii) when two index number series with different base periods are to be compared, or

(iii) When there is need for splicing two overlapping index number series.

Whatever be the reason, the technique of shifting the base is simple:

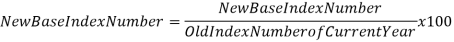Example

Reconstruct the following indices using 1997 as base: Year : 1991 1992 1993 1994 1995 1996 1997 1998 Index : 100 110 130 150 175 180 200 220

Shifting the Base Period Year Index Number (1991 = 100) Index Number (1997 = 100) 1991 100 1992 110 1993 130 1994 150 1995 175 1996 180 1997 200 1998 220

SPLICING TWO OVERLAPPING INDEX NUMBER SERIES

Splicing two index number series means reducing two overlapping index series with Different base periods into a single series either at the base period of the old series (one with an old base year), or at the base period of the new series (one with a recent Base year). This actually amounts to changing the weights of one series into the Weights of the other series.

Splicing the New Series to Make it Continuous with the Old Series

Here we reduce the new series into the old series after the base year of the former. As Shown in Table 6.8.2(i), splicing here takes place at the base year (1980) of the new Series. To do this, a ratio of the index for 1980 in the old series (200) to the index of 1980 in the new series (100) is computed and the index for each of the following years in the new series is multiplied by this ratio

Splicing the New Series with the Old Series Year Price Index (1976=100) (Old Series) Price Index (1980=100) (New Series) Spliced Index Number [New Series (200/100)] 1976 1977 1978 1979 1980 1981 1982 1983 1984 100 120 146 172 200 -- -- -- -- -- -- -- -- 100 110 116 125 140 100 120 146 172 200 220 232 250 280

Splicing the Old Series to Make it Continuous with the New Series

This means reducing the old series into the new series before the base period of the Letter. As shown in Table , splicing here takes place at the base period of the New series. To do this, a ratio of the index of 1980 of the new series (100) to the index Of 1980 of the old series (200) is computed and the index for each of the preceding Years of the old series are then multiplied by this ratio.

Splicing the Old Series with the New Series   Year Price Index (1976=100) (Old Series) Price Index (1980=100) (New Series) Spliced Index Number [Old Series (100/200)] 1976 1977 1978 1979 1980 1981 1982 1983 1984 100 120 146 172 200 -- -- -- -- -- -- -- -- 100 110 116 125 140 50 60 73.50 86 100 110 116 125 140

22222Index Numbers

5. Calculations forIndex of Weighted Average of Quantity Relatives (Base Year = 1980) Item Fish Mutton Chicken 7500 10620 9900 10000 13750 10800 9000000 1152000 1100000 1200000 1472000 1200000 Sum  28020 34370 3152000 3872000
Index of Weighted Average of Quantity Relatives, using
(i)   as the value weights
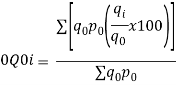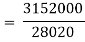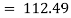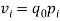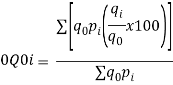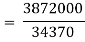(ii) as the value weights

6. Reconstruct the following indices using 1997 as base: Year : 1991 1992 1993 1994 1995 1996 1997 1998 Index : 100 110 130 150 175 180 200 220

Shifting the Base Period Year Index Number (1991 = 100) Index Number (1997 = 100) 1991 100 1992 110 1993 130 1994 150 1995 175 1996 180 1997 200 1998 220

7. Briefly explain Test of index number.

Answer - Test of index number -

Unit Test: 

This test states that the formula for constructing an index number should be independent of the units in which prices and quantities are expressed. All methods, except simple aggregative method, satisfy this test.

Time Reversal Test:

This test guides whether the method works both ways in time forward and backward. To quote Fisher, Time Reversal Test is the test which gives the same ratio between one point of comparison and the other, for calculation of index number, no matter which of the two is taken as base. In other words, when the index numbers of the two years are constructed by reversing the base year, they should be reciprocals of each other so that their product is unity.

Symbolically the test is represented as:

P01 X P10 = 1

Where P01 is the index for time “1” on time “0” as base and P10 is the index for time “0” on time “1” as base. If the product is not unity, the method suffers from time bias.

Time reversal test is satisfied by

1. Simple aggregative method
2. Fisher’s method
3. Marshall-Edgeworth’s method and
4. Kelly’s method.

Factor Reversal Test: 

Fisher has described this test in the following words: Just as each formula should permit the interchange of the two items without giving inconsistent results, so it ought to permit interchanging the prices and quantities without giving inconsistent result, i.e., the two results multiplied together should give the true value ratio.”

In simple words, the test means that the change in the price multiplied by the change in the quantity should be equal to the total change in the value. The total value of a given commodity in a given year is the product of the quantity and the price per unit (total value = p x q) . The ratio of the total value in one year to the total value in the preceding year is p1q1 / p0q0. Symbolically the test is represented as

P01 x Q01 = Σp1q1 / Σp0q0 V01

Where P01 denotes price index and Q01 , quantity index number.

This test is satisfied by Fisher’s method only.

Circular Test: 

According to this, if indices are constructed for year one based on year zero, for year two based on year one and for year zero based on year two, the product of all the indices should be equal to 1.

Symbolically

P01 X P12 X P20 = 1

This test is satisfied by

1. Simple aggregative method and
2. Kelly’s method.

8. What is Customer price index?

Answer - Customer price Index

The Consumer Price Index (CPI) measures the average price change of a set of consumer goods and services. CPIs can be calculated for single items or a predetermined group of items. All of these items are defined as "household goods and services."

Some of the household goods and services that CPI might measure include:

• Food
• Transportation
• Health and medical care
• Clothing
• Energy
• Education
• Recreation

Typically, the CPI is used to measure inflation and determine the cost of living at a given time. It provides important insight for economists, governments and companies.

9. What are the uses of Customer Price Index?

Uses of Customer price Index

The consumer price index is mainly used to measure inflation over a given period of time. It can also be leveraged to determine the cost of living.

CPI is mainly used to determine the efficacy of economic policies. Inflation indicates the health (or lack thereof) of an economy, so tracking it and responding to it appropriately is important for policymakers. When inflation sharply increases or decreases, the CPI provides economists and policymakers insight into how a government's economic policy affects the market.

This gives the ability to better understand whether current policies are working properly and how a government might be able to create better policies in the future. 

The inflation rates and cost of living that CPI identifies can have a huge impact on the financial lives of consumers. In fact, when inflation is identified, the Federal Reserve will often take actions to stop an economy from overheating. This can ultimately result in loans becoming more expensive for borrowers.

CPI can also be used to shape government assistance policies. It will often help determine who is eligible for programs such as social security and other help. It also can result in wage adjustments to account for cost-of-living.

Unit -VI

Index numbers

1. What is Index Number?

Answer - Index numbers are intended to measure the degree of economic changes over time. These numbers are values stated as a percentage of a single base figure. Index numbers are important in economic statistics. In simple terms, an index (or index number) is a number displaying the level of a variable relative to its level (set equal to 100) in a given base period.

2. What are the different types of Index Numbers?

Types of Index Numbers

Simple Index Number: A simple index number is a number that measures a relative change in a single variable with respect to a base. These type of Index numbers are constructed from a single item only. This ratio is then finally converted to a percentage 100 Value in Base Year Value in Period Index for any Period x i i = i.e. Simple Price Index for period i = 1,2,3 ... Will be 100 0 0 x p pP i i = …………(6-1) Similarly, Simple Quantity Index for period i = 1,2,3 ... Will be 100 0 0 x q qQ i i = …………(6-2)   Composite Index Number: A composite index number is a number that measures an average relative change in a group of relative variables with respect to a base. A composite index number is built from changes in a number of different items.   Price index Numbers: Price index numbers measure the relative changes in prices of a commodity between two periods. Prices can be either retail or wholesale. Price index number is useful to comprehend and interpret varying economic and business conditions over time. Quantity Index Numbers: These types of index numbers are considered to measure changes in the physical quantity of goods produced, consumed or sold of an item or a group of items.   Methods of constructing index numbers: There are two methods to construct index numbers: Price relative and aggregate methods (Srivastava, 1989)

In aggregate methods, the aggregate price of all items in a given year is expressed as a percentage of same in the base year, giving the index number.

Weighted aggregative index numbers: These index numbers are the simple aggregative type with the fundamental difference that weights are assigned to the various items included in the index.

3. State the characteristics of Index Numbers.

Characteristics of index numbers:

1. Index numbers are specialised averages.
2. Index numbers measure the change in the level of a phenomenon.
3. Index numbers measure the effect of changes over a period of time.

Index Numbers

4.     Explain problems in the construction of Price Index Number.

Answer -

BASE SHIFTING. The need for shifting the base may arise either

(i) when the base period of a given index number series is to be made more recent, or

(ii) when two index number series with different base periods are to be compared, or

(iii) When there is need for splicing two overlapping index number series.

Whatever be the reason, the technique of shifting the base is simple:

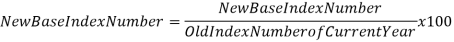Example

Reconstruct the following indices using 1997 as base: Year : 1991 1992 1993 1994 1995 1996 1997 1998 Index : 100 110 130 150 175 180 200 220

Shifting the Base Period Year Index Number (1991 = 100) Index Number (1997 = 100) 1991 100 1992 110 1993 130 1994 150 1995 175 1996 180 1997 200 1998 220

SPLICING TWO OVERLAPPING INDEX NUMBER SERIES

Splicing two index number series means reducing two overlapping index series with Different base periods into a single series either at the base period of the old series (one with an old base year), or at the base period of the new series (one with a recent Base year). This actually amounts to changing the weights of one series into the Weights of the other series.

Splicing the New Series to Make it Continuous with the Old Series

Here we reduce the new series into the old series after the base year of the former. As Shown in Table 6.8.2(i), splicing here takes place at the base year (1980) of the new Series. To do this, a ratio of the index for 1980 in the old series (200) to the index of 1980 in the new series (100) is computed and the index for each of the following years in the new series is multiplied by this ratio

Splicing the New Series with the Old Series Year Price Index (1976=100) (Old Series) Price Index (1980=100) (New Series) Spliced Index Number [New Series (200/100)] 1976 1977 1978 1979 1980 1981 1982 1983 1984 100 120 146 172 200 -- -- -- -- -- -- -- -- 100 110 116 125 140 100 120 146 172 200 220 232 250 280

Splicing the Old Series to Make it Continuous with the New Series

This means reducing the old series into the new series before the base period of the Letter. As shown in Table , splicing here takes place at the base period of the New series. To do this, a ratio of the index of 1980 of the new series (100) to the index Of 1980 of the old series (200) is computed and the index for each of the preceding Years of the old series are then multiplied by this ratio.

Splicing the Old Series with the New Series   Year Price Index (1976=100) (Old Series) Price Index (1980=100) (New Series) Spliced Index Number [Old Series (100/200)] 1976 1977 1978 1979 1980 1981 1982 1983 1984 100 120 146 172 200 -- -- -- -- -- -- -- -- 100 110 116 125 140 50 60 73.50 86 100 110 116 125 140

22222Index Numbers

5. Calculations forIndex of Weighted Average of Quantity Relatives (Base Year = 1980) Item Fish Mutton Chicken 7500 10620 9900 10000 13750 10800 9000000 1152000 1100000 1200000 1472000 1200000 Sum  28020 34370 3152000 3872000
Index of Weighted Average of Quantity Relatives, using
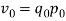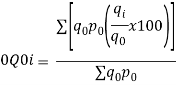(i)   as the value weights
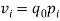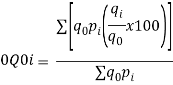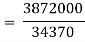(ii) as the value weights

6. Reconstruct the following indices using 1997 as base: Year : 1991 1992 1993 1994 1995 1996 1997 1998 Index : 100 110 130 150 175 180 200 220

Shifting the Base Period Year Index Number (1991 = 100) Index Number (1997 = 100) 1991 100 1992 110 1993 130 1994 150 1995 175 1996 180 1997 200 1998 220

7. Briefly explain Test of index number.

Answer - Test of index number -

Unit Test: 

This test states that the formula for constructing an index number should be independent of the units in which prices and quantities are expressed. All methods, except simple aggregative method, satisfy this test.

Time Reversal Test:

This test guides whether the method works both ways in time forward and backward. To quote Fisher, Time Reversal Test is the test which gives the same ratio between one point of comparison and the other, for calculation of index number, no matter which of the two is taken as base. In other words, when the index numbers of the two years are constructed by reversing the base year, they should be reciprocals of each other so that their product is unity.

Symbolically the test is represented as:

P01 X P10 = 1

Where P01 is the index for time “1” on time “0” as base and P10 is the index for time “0” on time “1” as base. If the product is not unity, the method suffers from time bias.

Time reversal test is satisfied by

1. Simple aggregative method
2. Fisher’s method
3. Marshall-Edgeworth’s method and
4. Kelly’s method.

Factor Reversal Test: 

Fisher has described this test in the following words: Just as each formula should permit the interchange of the two items without giving inconsistent results, so it ought to permit interchanging the prices and quantities without giving inconsistent result, i.e., the two results multiplied together should give the true value ratio.”

In simple words, the test means that the change in the price multiplied by the change in the quantity should be equal to the total change in the value. The total value of a given commodity in a given year is the product of the quantity and the price per unit (total value = p x q) . The ratio of the total value in one year to the total value in the preceding year is p1q1 / p0q0. Symbolically the test is represented as

P01 x Q01 = Σp1q1 / Σp0q0 V01

Where P01 denotes price index and Q01 , quantity index number.

This test is satisfied by Fisher’s method only.

Circular Test: 

According to this, if indices are constructed for year one based on year zero, for year two based on year one and for year zero based on year two, the product of all the indices should be equal to 1.

Symbolically

P01 X P12 X P20 = 1

This test is satisfied by

1. Simple aggregative method and
2. Kelly’s method.

8. What is Customer price index?

Answer - Customer price Index

The Consumer Price Index (CPI) measures the average price change of a set of consumer goods and services. CPIs can be calculated for single items or a predetermined group of items. All of these items are defined as "household goods and services."

Some of the household goods and services that CPI might measure include:

• Food
• Transportation
• Health and medical care
• Clothing
• Energy
• Education
• Recreation

Typically, the CPI is used to measure inflation and determine the cost of living at a given time. It provides important insight for economists, governments and companies.

9. What are the uses of Customer Price Index?

Uses of Customer price Index

The consumer price index is mainly used to measure inflation over a given period of time. It can also be leveraged to determine the cost of living.

CPI is mainly used to determine the efficacy of economic policies. Inflation indicates the health (or lack thereof) of an economy, so tracking it and responding to it appropriately is important for policymakers. When inflation sharply increases or decreases, the CPI provides economists and policymakers insight into how a government's economic policy affects the market.

This gives the ability to better understand whether current policies are working properly and how a government might be able to create better policies in the future. 

The inflation rates and cost of living that CPI identifies can have a huge impact on the financial lives of consumers. In fact, when inflation is identified, the Federal Reserve will often take actions to stop an economy from overheating. This can ultimately result in loans becoming more expensive for borrowers.

CPI can also be used to shape government assistance policies. It will often help determine who is eligible for programs such as social security and other help. It also can result in wage adjustments to account for cost-of-living.