Unit – 2
AC Circuits
Q1) Prove that the average value of sinusoid ally varying alternating current is 0.636 times maximum value of alternating current.
A1) The arithmetic mean of all the value over complete one cycle is called as average value

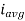 =
= 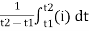
For the derivation we are considering only hall cycle.
Thus 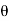 varies from 0 to ᴫ
varies from 0 to ᴫ
i = Im Sin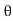
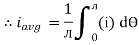
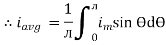
Solving
We get
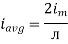
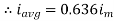
Similarly, Vavg=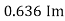
The average value of sinusoid ally varying alternating current is 0.636 times maximum value of alternating current.
Q2) Prove that the RMS value of sinusoidal alternating current is 0.707 times the maximum value of alternating current.
A2) The RMS value of AC current is equal to the steady state DC current that required to produce the same amount of heat produced by ac current provided that resistance and time for which these currents flows are identical.
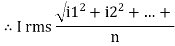
I rms = 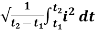
Direction for RMS value:
Instantaneous current equation is given by
i = Im Sin 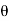
but 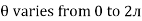
I rms = 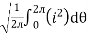
= 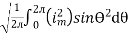
=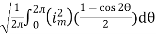
=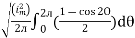
Solving
=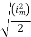
=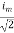
Similar we can derive
V rms= 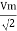 or 0.707 Vm
or 0.707 Vm
 the RMS value of sinusoidally alternating current is 0.707 times the maximum value of alternating current.
the RMS value of sinusoidally alternating current is 0.707 times the maximum value of alternating current.
Q3) Explain Resonance in series RLC circuit
A3) It is defined as the phenomenon which takes place in the series or parallel R-L-C circuit which leads to unity power factor
Voltage and current in R-L-C ckt are in phase with each other.
Resonance is used in many communication circuits such as radio receiver.
Resonance in series RLC -> series resonance in parallel->antiresonance/parallel resonance
Condition for resonance
XL=XC
Resonant frequency (fr): For given values R-L-C the inductive reactance XL becomes exactly equal to the capacitive reactance XC only at one particular frequency. This frequency is called as resonant frequency and denoted by ( fr)
Expression for resonant frequency (fr)
We know that
XL = 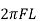 - inductive reactance
- inductive reactance
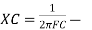 capacitive reactance
capacitive reactance
At a particle or frequency f=fr,the inductive and capacitive reactance are exactly equal
Therefore, XL = XC ----at f=fr
i.e. 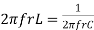
Therefore, 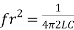
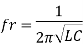
and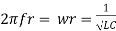 rad/sec
rad/sec
Q4) Define: Reactance, Impedance and Power factor.
A4) Inductive Reactance (XL)
It is opposition to the flow of an AC current offered by inductor.
XL = ω L But ω = 2 ᴫ F
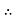 XL = 2 ᴫ F L
XL = 2 ᴫ F L
It is measured in ohm
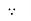 XL∝FInductor blocks AC supply and passes dc supply zero
XL∝FInductor blocks AC supply and passes dc supply zero
Capacitive Reactance (Xc)
It is opposition to the flow of ac current offered by capacitor
Xc = 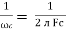
Measured in ohm
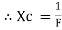 Capacitor offers infinite opposition to dc supply
Capacitor offers infinite opposition to dc supply 
Impedance (Z)
The ac circuit is to always pure R pure L and pure C it well attains the combination of these elements. “The combination of R1 XL and XC is defined and called as impedance represented as
Z = R +i X
Ø = 0
 only magnitude
only magnitude
R = Resistance, i = denoted complex variable, X =Reactance XL or Xc
Polar Form
Z = 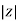 L I
L I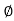
Where 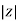 =
=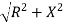
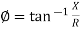 Measured in ohm
Measured in ohm
Power factor (P.F.)
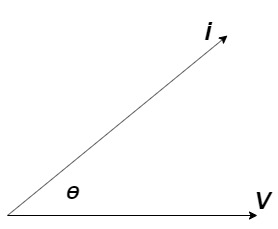
It is the cosine of angle between voltage and current
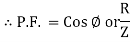
If Ɵis –ve or lagging (I lags V) then lagging P.F.
If Ɵ is +ve or leading (I leads V) then leading P.F.
If Ɵ is 0 or in phase (I and V in phase) then unity P.F.
Q5) Explain Ac circuit containing pure Inductors
A5)
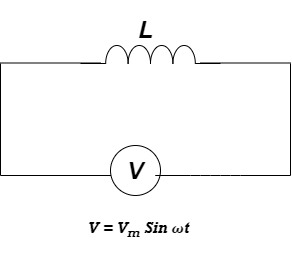
Consider pure Inductor (L) is connected across alternating voltage. Source
V = Vm Sin ωt
When an alternating current flow through inductance it set ups alternating magnetic flux around the inductor.
This changing the flux links the coil and self-induced emf is produced
According to faradays Law of E M I
e = 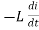
at all instant applied voltage V is equal and opposite to self-induced emf [ lenz’s law]
V = -e
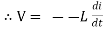 =
= 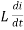
But V = Vm Sin ωt
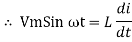
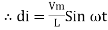 dt
dt
Taking integrating on both sides
 dt
dt
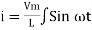 dt
dt
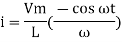
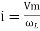 (-cos
(-cos 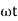 )
)
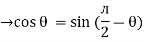
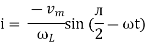
but sin (–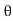 ) = sin (+
) = sin (+ )
)
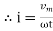 sin (
sin (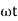 -
- 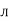 /2)
/2)
And Im= 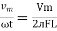
 /2)
/2)
 /2
/2
= -ve
= lagging
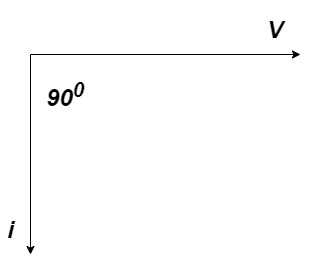
= I lag v by 900
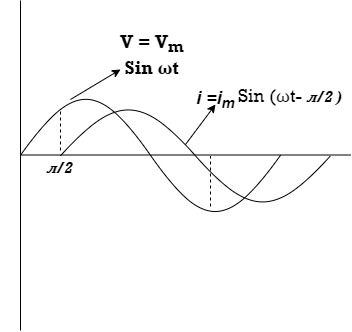
Phasor:
Power P = Ѵ. I
= Vm sin wt Im sin (wt 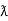 /2)
/2)
= Vm Im Sin wt Sin (wt –  /s)
/s)
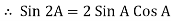 ①
①
And
Sin (wt -  /s) = - cos wt ②
/s) = - cos wt ②
Sin (wt – 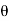 ) = - cos
) = - cos 
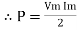 sin 2 wt from ① and ②
sin 2 wt from ① and ②
The average value of sin curve over a complete cycle is always zero
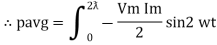
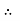 Pavg = 0
Pavg = 0
Q6) Explain the impedance of series circuit?
A6) Series RLC circuit:
Applying KVL to the series RLC circuit shown in the figure above at t= 0 gives the following basic relation :
V = vR(t) + vC(t ) + vL(t)
Representing the above voltages in terms of the current iin the circuit we get the following integral differentia lequation:
Ri + 1/C∫ 𝒊𝒅𝒕 + L. (di/dt)= V
To convert it into a differential equation it is differentiated on both sides with respect to time and we get
L(d2 i/dt2 )+ R(di/dt)+ (1/C)i = 0
This can be written in the form
[s2 + (R/L)s + (1/LC)].i = 0 where ‘s’ is an operator equivalent to (d/dt) And the corresponding characteristic equation is then given by
[s2 + (R/L)s + (1/LC)] = 0
This is in the standard quadratic equation form and the rootss1ands2are given by
s1,s2 =− R/2L±√[(R/2L)2− (1/LC)]= −α ±√(α 2– ω0 2 )
where α is known as the same exponential damping coefficient and
ω0is known as the same Resonant frequency
as explained in the case of Parallel RLC circuit and are given by :
α = R/2L and ω0= 1/ √LC and A1 and A2must be found by applying the given initial conditions.
Here also we note three basic scenarios with the equations for s1 and s2 depending on the relative sizes of αand ω0 (dictated by the values of R, L, and C).
CaseA: α > ω0,i.e when (R/2L) 2>1/LC , s1 and s2 will both be negative real numbers, leading to what is referred to as an over damped response given by : i (t) = A1e s1t+ A2e s2t. Sinces1 and s2 are both be negative real numbers this is the (algebraic) sum of two decreasing exponential terms. Since s2 is a larger negative number it decays faster and then the response is dictated by the first term A1e s1t .
Case B : α = ω0, ,i.e when (R/2L) 2=1/LCs1 and s2are equal which leads to what is called a critically damped response given by : i (t) = e −αt (A1t + A2) Case C : α < ω0,i.e when (R/2L) 2 .
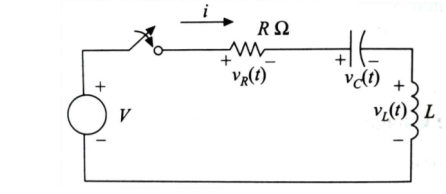
Q7) Explain the impedance of parallel circuit?
A7) Let us first consider the simple parallel RLC circuit with DC excitation as shown in the figure below.
For the sake of simplifying the process of finding the response we shall also assume that the initial current in the inductor and the voltage across the capacitor are zero.
Then applying the Kirchhoff’s current law (KCL )( i = iC +iL ) to the common node we get the following differential equation:
(V-v) /R = 1/L 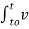 dt’ + C. dv/dt
dt’ + C. dv/dt
where v = vC(t) = vL(t) is the variable whose value is to be obtained. When we differentiate both sides of the above equation once with respect to time we get the standard Linear second-order homogeneous differential equation
C. (d 2 v / dt 2) + (1/R) ( dv/dt) + (1/L).v =0
(d 2 v / dt 2) + (1/RC) ( dv/dt) + (1/LC).v =0
whose solution v(t) is the desired response.
This can be written in the form:
[s 2 + (1/RC)s + (1/LC)].v(t) = 0 where ‘s’ is an operator equivalent to (d/dt) and the corresponding characteristic equation is then given by :
[s 2 + (1/RC)s + (1/LC)] = 0
This equation is usually called the auxiliary equation or the characteristic equation. If it can be satisfied, then our assumed solution is correct. This is a quadratic equation and the roots s1 and s2are given as :
s1= − 1/2RC+√[(1/2RC) 2− 1/LC]
s2= − 1/2RC−√[ (1/2RC)2− 1/LC ]
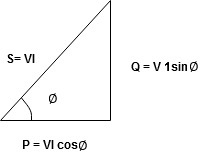
Q8) Define Real Power, Reactive Power, Apparent Power, Power Factor?
A8)
S= V × I
Unit - Volte- Ampere (VA)
In kilo – KVA
2. Real power/ True power/Active power/Useful power : (P) it is defined as the product of rms value of voltage and current and the active component or it is the average or actual power consumed by the resistive path (R) in the given combinational circuit.
It is measured in watts
P = VI 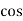 Φ watts / KW, where Φ is the power factor angle.
Φ watts / KW, where Φ is the power factor angle.
3. Reactive power/Imaginary/useless power [Q]
It is defined as the product of voltage, current and sine B and I
Therefore,
Q= V.I 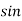 Φ
Φ
Unit –V A R
In kilo- KVAR
As we know power factor is cosine of angle between voltage and current
i.e. ɸ. F = cos ɸ
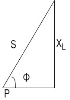
In other words, also we can derive it from impedance triangle
Now consider Impedance triangle in R.L.ckt
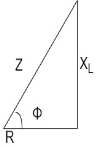
From triangle ,
Now 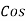 Φ – power factor=
Φ – power factor= 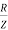
Power factor = 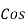 Φ or
Φ or 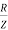
Q9) Explain the AC circuit consisting of pure capacitance?
A9)
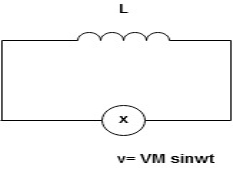
Consider pure capacitor C is connected across alternating voltage source
Ѵ = Ѵm Sin wt
Current is passing through capacitor the instantaneous charge ɡ produced on the plate of capacitor
ɡ = C Ѵ
ɡ = c Vm sin wt
the current is rate of flow of charge
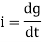
i=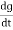 (cvm sin wt)
(cvm sin wt)
i = c Vm w cos wt
then rearranging the above eqth.
i = 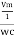 cos wt
cos wt
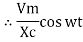
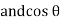 = sin (wt +
= sin (wt + 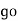 X/2)
X/2)
i = 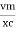 sin (wt + X/2)
sin (wt + X/2)
but 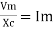
 X/2)
X/2)

= leading
= I leads V by 900
Waveform :
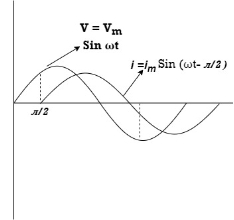
Phase
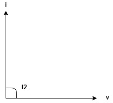
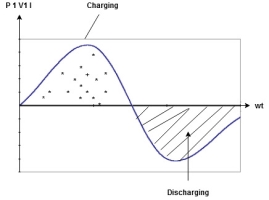
Power P= Ѵ. i
= [Vm sinwt] [ Im sin (wt + X/2)]
= Vm Im Sin wt Sin (wt + X/2)]
 (cos wt)
(cos wt)
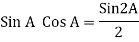
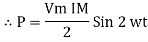
 to charging power waveform [resultant].
to charging power waveform [resultant].
Q10) Explain the series R-L circuit?
A10) Series R-L Circuit
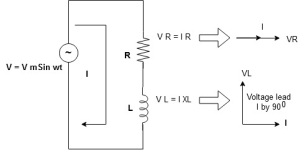
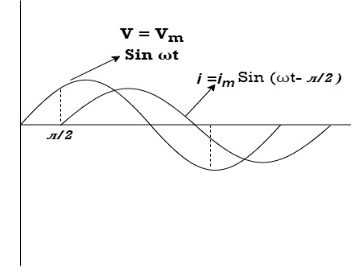
Consider a series R-L circuit connected across voltage source V= Vm sin wt
As some I is the current flowing through the resistor and inductor due do this current voltage drops arcos R and L R  VR = IR and L
VR = IR and L  VL = I X L
VL = I X L
 Total V = VR + VL
Total V = VR + VL
V = IR + I X L  V = I [R + X L]
V = I [R + X L]
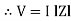
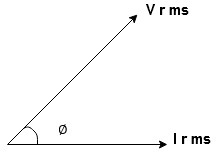
Take current as the reference phasor : 1) for resistor current is in phase with voltage 2) for Inductor voltage leads current or current lags voltage by 90 0.
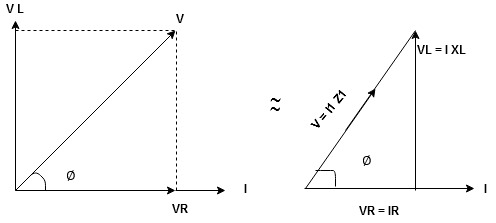
For voltage triangle
Ø is power factor angle between current and resultant voltage V and
V = 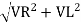

V = 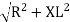

where Z = Impedance of circuit and its value is 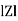 =
= 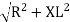
Impedance Triangle
Divide voltage triangle by I

Rectangular form of Z = R+ixL
and polar from of Z = 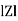 L +
L + 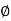
(+ j X L +  because it is in first quadrant )
because it is in first quadrant )
Where 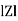 =
= 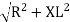
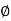 + Tan -1
+ Tan -1 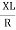
Current Equation :
From the voltage triangle we can sec. that voltage is leading current by  or current is legging resultant voltage by
or current is legging resultant voltage by 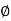
Or i = 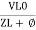 =
= 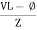 [ current angles - Ø )
[ current angles - Ø )
Resultant Phasor Diagram from Voltage and current eqth.
Wave form
Power equation
P = V .I.
P = Vm Sin wt Im Sin wt – Ø
P = Vm Im (Sin wt) Sin (wt – Ø)
P = 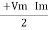 (Cos Ø) - Cos (2wt – Ø)
(Cos Ø) - Cos (2wt – Ø)
Since 2 sin A Sin B = Cos (A-B) – Cos (A+B)
P = 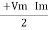 Cos Ø -
Cos Ø - 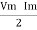 Cos (2wt – Ø)
Cos (2wt – Ø)
Average Power
pang = 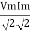 Cos Ø
Cos Ø
Since ② term become zero because Integration of cosine come from 0 to 2ƛ
 pang = Vrms Irms cos Ø watts.
pang = Vrms Irms cos Ø watts.
Power Triangle :
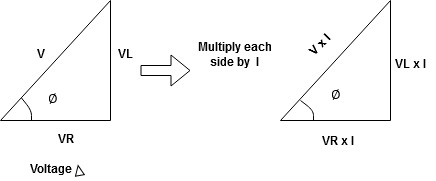
From 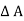
VI = VRI + VLI B
Now cos Ø in 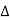 A =
A = 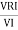
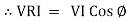 ①
①
Similarly Sin 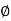 =
= 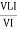

Apparent Power Average or true Reactive or useless power
Or real or active
-Unit (VI) Unit (Watts) C/W (VAR) denoted by (Ø)
Denoted by [S] denoted by [P]
Power 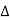 for R L ekt.
for R L ekt.