Unit – 1

Partial Differential Equations

Q1) Solve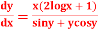

A1) We have,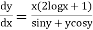

Separating the variables we get

(sin y + y cos y ) dy ={ x (2 log x +1} dx

Integrating both the sides we get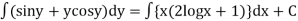

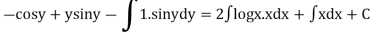
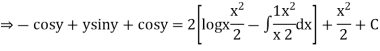
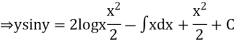
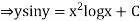
Q2) Solve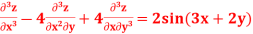

A2)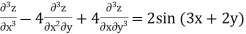

Putting

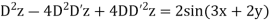
A.E. is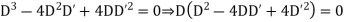

Put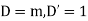

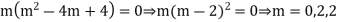
C.F. is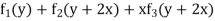

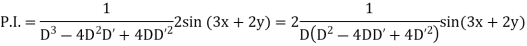
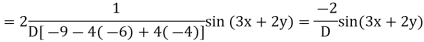
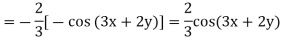
General solution is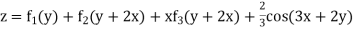

Q3) Find the general integral of the equation

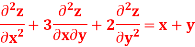
A3)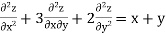

With 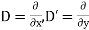the given equation can be written in the form 

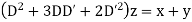
Writing D = m and D’=1the auxiliary equation is

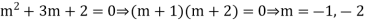
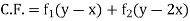
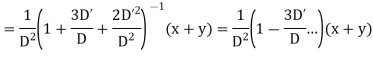
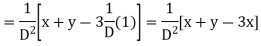
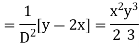
Hence the complete solution is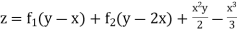

Q4) Solve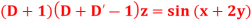

A4)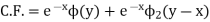

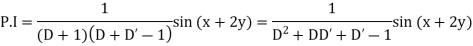
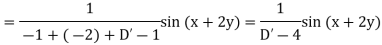
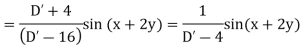
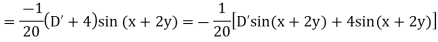
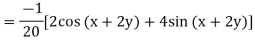
Hence the solution is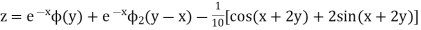

Q5) Solve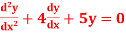

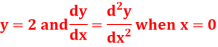
A5) Here the auxiliary equation is

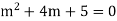
Its root are

The complementary function is

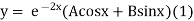
On putting y = 2 and x = 0 in (1) we get

2 =A

On putting A = 2 in (1) we have

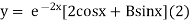
On Differentiating (2) we get

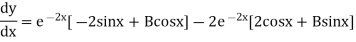
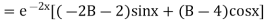
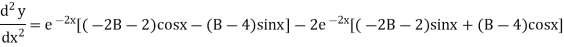
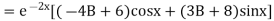
But,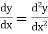

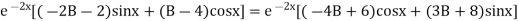
On putting x = 0, we get

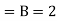
(2) becomes,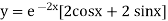

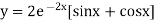
Q6) A rod of length 1 with insulated sides is initially at a uniform temperature u. Its ends are suddenly cooled to 0° Celsius and are kept at that temperature. Prove that the temperature function u (x, t) is given by

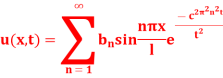
Where is determined from the equation.

A6) Let the equation for the conduction of heat be

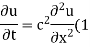
Let us assume that u = XT, where X is a function of x alone and T that of t alone.

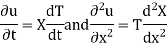
Substituting these values in (1) we get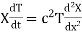

i.e.

Let each side be equal to a constant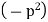

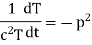
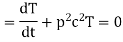
And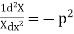

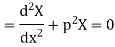
Solving (3) and (4) we have

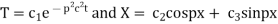
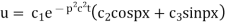
Putting x = 0, u = 0 in (5), we get

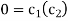
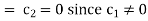
(5) becomes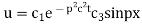

Again putting x = l, u =0 in (6), we get

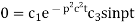
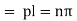
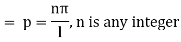
Hence (6) becomes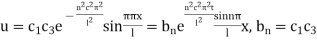

This equation satisfies the given conditions for all integral values n. Hence taking n = 1, 2, 3,…, the most general solution is

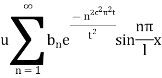
By initial conditions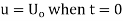

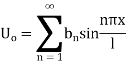
Q7) The ends A and B of a rod 20 cm long having the temperature at 30 degree Celsius and at 80 degree Celsius until steady state prevails. The temperature of the ends are changed to 40 degree Celsius and 60 degree Celsius respectively. Find the temperature distribution in the rod at time t.

A7) The initial temperature distribution in the rod is

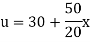
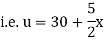
And the final distribution (i.e. steady state) is

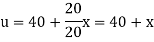
To get u in the intermediate period, reckoning time from the instant when the end temperature were changed we assumed

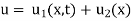
Where 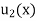is the steady state temperature distribution in the rod (i.e. temperature after a sufficiently long time) and 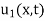is the transient temperature distribution which tends to zero as t increases.

Thus,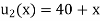

Now 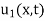satisfies the one dimensional heat flow equation

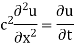
Hence u is of the form

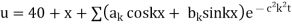
Since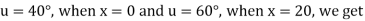

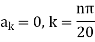
Hence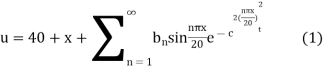

Using the initial condition i.e.

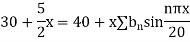
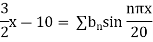
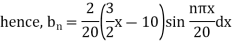
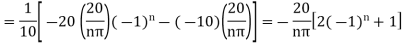
Putting this value of 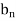n (1), we get

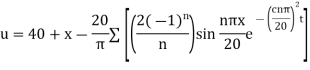
Q8) Find the solution of the wave equation

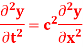
Such that is a constant) when x = 1 and y = 0 when x =0

A8)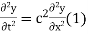

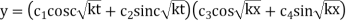
Put y = 0, when x = 0

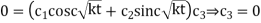
(2) is reduced to

)

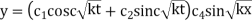
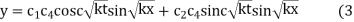Put 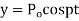when x=1

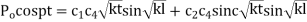
Equating the coefficient of sin and cos on both sides

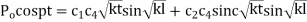
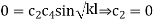
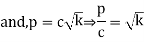
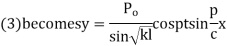
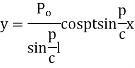
Q9) Use the method of separation of variables to solve equation

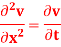
Given that v = 0 when t→ as well as v =0 at x = 0 and x = 1.

A9)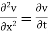  

Let us assume that v = XT where X is a function of x only and T that of t only

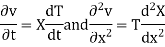
Substituting these values in (1), we get

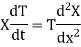
Let each side of (2) equal to a constant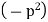

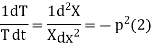
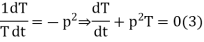
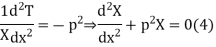
Solving (3) and (4) we have

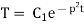
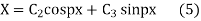
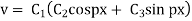
Putting x = 0, v = 0 in (5) we get

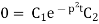
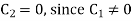
On putting the value of 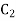in (5) we get

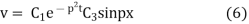
Again putting x = l, v= 0 in (6) we get

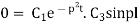
Since 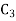cannot be zero.

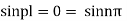
Inputting the value of p in (6) it becomes

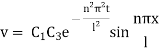
Hence,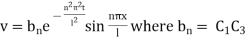

This equation satisfies the given condition for all integral values of n. Hence taking n = 1, 2, 3,… the most general solution is

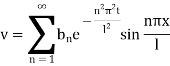
Q10) Prove that

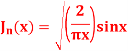
A10) We know that

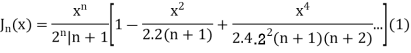
Substituting 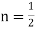in (1) we obtain