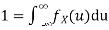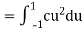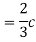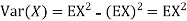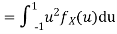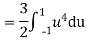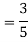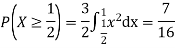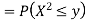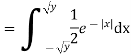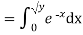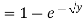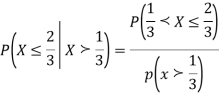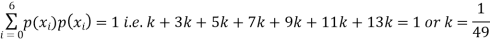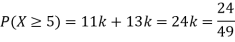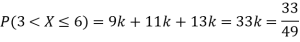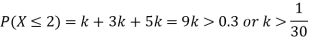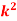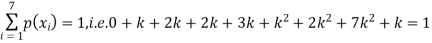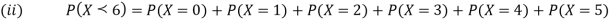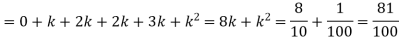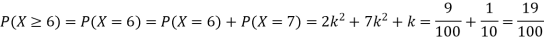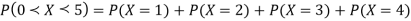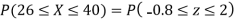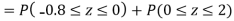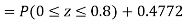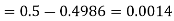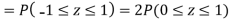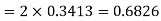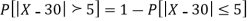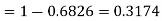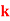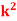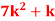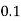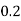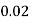M3
Unit-2Continuous Probability Distributions Q1) Let X be a random variable with PDF given by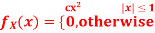 a, Find the constant c.b. Find EX and Var (X).c. Find P(X
a, Find the constant c.b. Find EX and Var (X).c. Find P(X 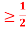 ).S1)
).S1)
Q2) Let X be a continuous random variable with PDF given by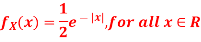 If
If 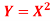 , find the CDF of Y.S2) First we note that
, find the CDF of Y.S2) First we note that 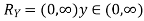 , we have
, we have
Q3) Let X be a continuous random variable with PDF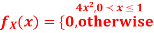 Find
Find 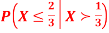 .S3) We have
.S3) We have
Q4) If a continuous random variable X has the following probability density function: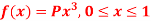 Then find-1. P[0.2 < X < 0.5]S4)Here f(x) is the probability density function, then-
Then find-1. P[0.2 < X < 0.5]S4)Here f(x) is the probability density function, then-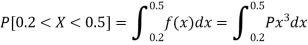
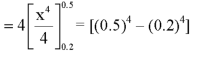
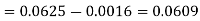 Q5) The probability density function of a variable X is
Q5) The probability density function of a variable X is
(i) Find  (ii) What will be e minimum value of k so that
(ii) What will be e minimum value of k so that  S5) (i) If X is a random variable then
S5) (i) If X is a random variable then
Q6) A random variate X has the following probability function
(i) Find the value of the k.(ii) 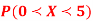 S6) (i) If X is a random variable then
S6) (i) If X is a random variable then
Q7) X is a normal variate with mean 30 and S.D. 5, find the probabilities that(i) 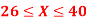 (ii)
(ii)  (iii) |X-30|≥5S7) We have μ =30 and
(iii) |X-30|≥5S7) We have μ =30 and 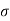 =5
=5
Q8) In a test on 2000 electric bulbs, it was found that the life of a particular make was normally distributed with an average life of 2040 hours and standard deviation of 60 hours. Estimated number of bulbs likely to burn forMore than 2150 hours Less than 1950 hours and More than 1920 hours and but less than 2 160 hours S8)Here μ = 2040 hours and 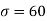 hours(a) For x = 2150,
hours(a) For x = 2150, 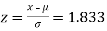 Area against z = 1.83 in the table III = 0.4664We however require the area to the right of the ordinate at z = 1.83. This area = 0.5-0.4664=0.0336Thus the number of bulbs expected to burn for more than 2150 hours. = 0.0336×2000 = 67 approximately(b) For x = 1950,
Area against z = 1.83 in the table III = 0.4664We however require the area to the right of the ordinate at z = 1.83. This area = 0.5-0.4664=0.0336Thus the number of bulbs expected to burn for more than 2150 hours. = 0.0336×2000 = 67 approximately(b) For x = 1950, 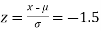 The area required in this case is to be left of z = -1.33
The area required in this case is to be left of z = -1.33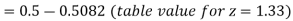
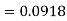 Therefore the number of bulbs expected to burn for less than 1950 hours
Therefore the number of bulbs expected to burn for less than 1950 hours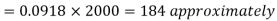 (c) When x = 1920,
(c) When x = 1920, 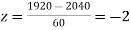 When x = 2160,
When x = 2160, 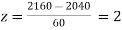 The number of bulbs expected to learn for more than 1920 hours but less than 2160 hours will be represented by the area between z = -2 and z = 2. This is twice the area from the table for z =2, i.e. 2 × 0.4772=0.9544Thus required number of bulbs = 0.9544 × 2000 = 1909 nearly Q9) Following probability distribution
The number of bulbs expected to learn for more than 1920 hours but less than 2160 hours will be represented by the area between z = -2 and z = 2. This is twice the area from the table for z =2, i.e. 2 × 0.4772=0.9544Thus required number of bulbs = 0.9544 × 2000 = 1909 nearly Q9) Following probability distribution
Find: (i) k (ii) 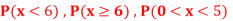 (i) Distribution function (ii) If
(i) Distribution function (ii) If 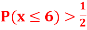 find minimum value of C (iii) Find
find minimum value of C (iii) Find 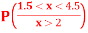 S9)If P(x) is p.m.f –
S9)If P(x) is p.m.f – 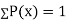
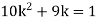
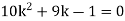
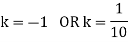 (i)
(i)
(ii) 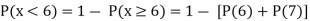
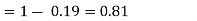
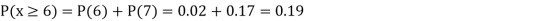
 (iii)
(iii)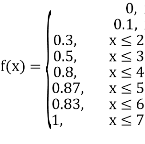 (iv)
(iv) 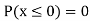
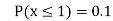
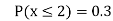
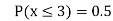
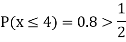
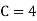 (v)
(v)
Q10) I choose real number uniformly at random in the interval [a, b], and call it X. Buy uniformly at random, we mean all intervals in [a, b] that have the same length must have the same probability. Find the CDF of X.S10) 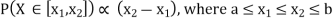 Since
Since  we conclude
we conclude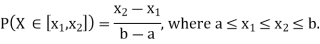 Now, let us find the CDF. By definition
Now, let us find the CDF. By definition 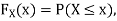 thus immediately have
thus immediately have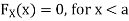
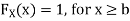 For
For 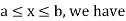
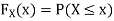
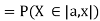
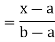 Thus, to summarize
Thus, to summarize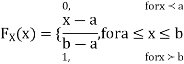 Note that hear it does not matter if we use “<” or “≤” as each individual point has probability zero, so for example
Note that hear it does not matter if we use “<” or “≤” as each individual point has probability zero, so for example 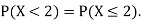 Figure 4.1 shows the CDF of X. As we expect the CDF starts at 0 at end at 1.
Figure 4.1 shows the CDF of X. As we expect the CDF starts at 0 at end at 1.
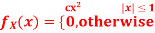 a, Find the constant c.b. Find EX and Var (X).c. Find P(X
a, Find the constant c.b. Find EX and Var (X).c. Find P(X 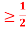 ).S1)
).S1)
Thus we must have b. To find EX we can write
In fact, we could have guessed EX = 0 because the PDF is symmetric around x = 0. To find Var (X) we have
c. To find
|
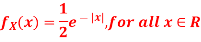 If
If 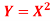 , find the CDF of Y.S2) First we note that
, find the CDF of Y.S2) First we note that 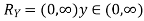 , we have
, we have
Thus, |
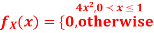 Find
Find 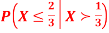 .S3) We have
.S3) We have
|
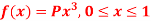 Then find-1. P[0.2 < X < 0.5]S4)Here f(x) is the probability density function, then-
Then find-1. P[0.2 < X < 0.5]S4)Here f(x) is the probability density function, then-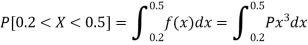
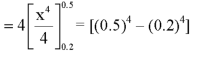
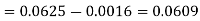 Q5) The probability density function of a variable X is
Q5) The probability density function of a variable X isX | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
P(X) | k | 3k | 5k | 7k | 9k | 11k | 13k |
 (ii) What will be e minimum value of k so that
(ii) What will be e minimum value of k so that  S5) (i) If X is a random variable then
S5) (i) If X is a random variable then
(ii)Thus minimum value of k=1/30. |
x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
P (x) | 0 | k | 2k | 2k | 3k |
|
|
|
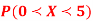 S6) (i) If X is a random variable then
S6) (i) If X is a random variable then
|
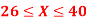 (ii)
(ii)  (iii) |X-30|≥5S7) We have μ =30 and
(iii) |X-30|≥5S7) We have μ =30 and 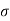 =5
=5
(i) When X = 26,z = -0.8, when X =40, z =-2
(ii) When X =45, z =3
(iii)
|
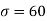 hours(a) For x = 2150,
hours(a) For x = 2150, 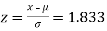 Area against z = 1.83 in the table III = 0.4664We however require the area to the right of the ordinate at z = 1.83. This area = 0.5-0.4664=0.0336Thus the number of bulbs expected to burn for more than 2150 hours. = 0.0336×2000 = 67 approximately(b) For x = 1950,
Area against z = 1.83 in the table III = 0.4664We however require the area to the right of the ordinate at z = 1.83. This area = 0.5-0.4664=0.0336Thus the number of bulbs expected to burn for more than 2150 hours. = 0.0336×2000 = 67 approximately(b) For x = 1950, 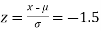 The area required in this case is to be left of z = -1.33
The area required in this case is to be left of z = -1.33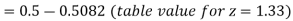
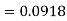 Therefore the number of bulbs expected to burn for less than 1950 hours
Therefore the number of bulbs expected to burn for less than 1950 hours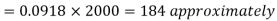 (c) When x = 1920,
(c) When x = 1920, 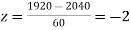 When x = 2160,
When x = 2160, 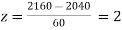 The number of bulbs expected to learn for more than 1920 hours but less than 2160 hours will be represented by the area between z = -2 and z = 2. This is twice the area from the table for z =2, i.e. 2 × 0.4772=0.9544Thus required number of bulbs = 0.9544 × 2000 = 1909 nearly Q9) Following probability distribution
The number of bulbs expected to learn for more than 1920 hours but less than 2160 hours will be represented by the area between z = -2 and z = 2. This is twice the area from the table for z =2, i.e. 2 × 0.4772=0.9544Thus required number of bulbs = 0.9544 × 2000 = 1909 nearly Q9) Following probability distribution X | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
P(x) | 0 |
|
|
|
|
|
|
|
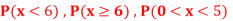 (i) Distribution function (ii) If
(i) Distribution function (ii) If 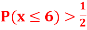 find minimum value of C (iii) Find
find minimum value of C (iii) Find 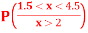 S9)If P(x) is p.m.f –
S9)If P(x) is p.m.f – 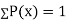
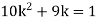
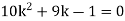
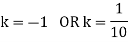 (i)
(i)X | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
P(x) | 0 |
|
|
|
|
|
|
|
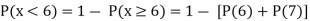
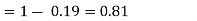
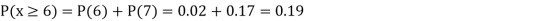
 (iii)
(iii)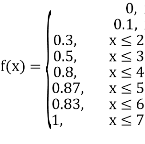 (iv)
(iv) 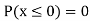
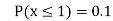
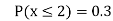
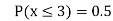
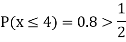
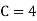 (v)
(v)
|
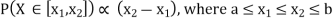 Since
Since  we conclude
we conclude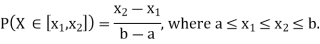 Now, let us find the CDF. By definition
Now, let us find the CDF. By definition 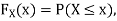 thus immediately have
thus immediately have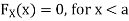
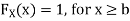 For
For 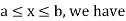
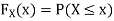
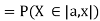
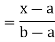 Thus, to summarize
Thus, to summarize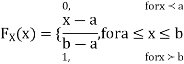 Note that hear it does not matter if we use “<” or “≤” as each individual point has probability zero, so for example
Note that hear it does not matter if we use “<” or “≤” as each individual point has probability zero, so for example 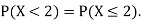 Figure 4.1 shows the CDF of X. As we expect the CDF starts at 0 at end at 1.
Figure 4.1 shows the CDF of X. As we expect the CDF starts at 0 at end at 1. 0 matching results found