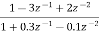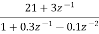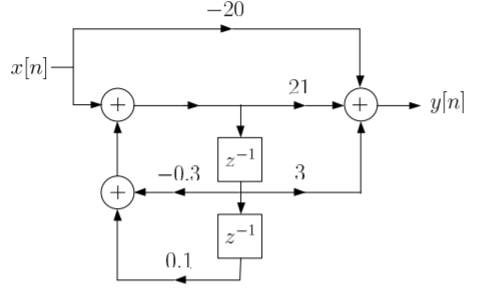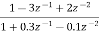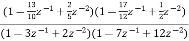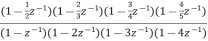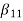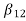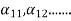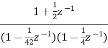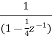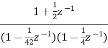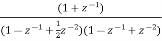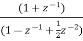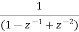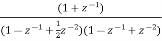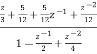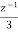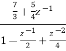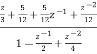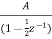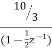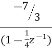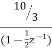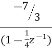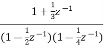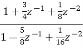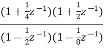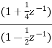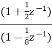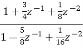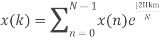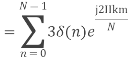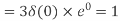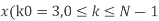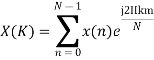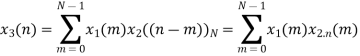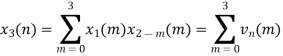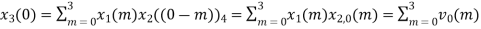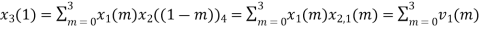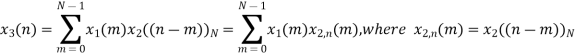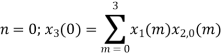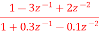
A1) H(z)= Writing above transfer function in standard form for parallel realisation we get H(z)=-20+ The structure is shown below
Fig 1 Parallel Realisation of H(z)=
|
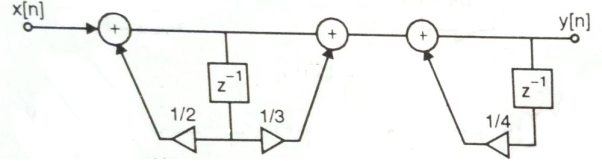
 . Realise the cascade form IIR filter.
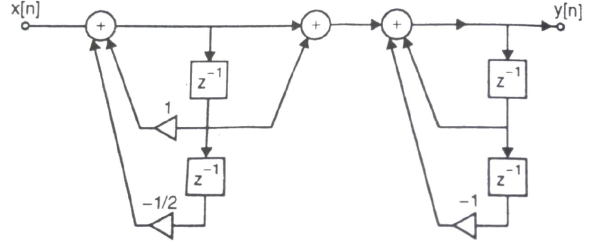
. Realise the cascade form IIR filter.A2) H(z)= The above function can be simplified as H(z)=
Fig 2 Cascade IIR Form Hence, using the above structure and placing the values of
|
 y(n-1) +
y(n-1) +  y(n-2) = x(n) +
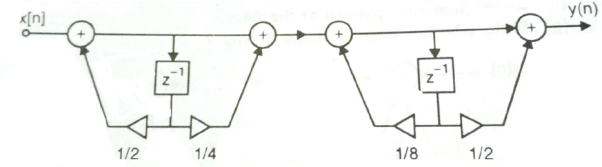
y(n-2) = x(n) +  x(n-1) realise using cascade form?
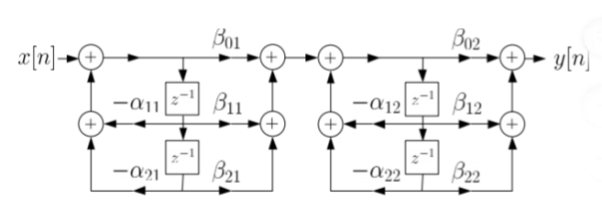
x(n-1) realise using cascade form?A3) The system transfer function is given as H(z) = Y(z)/X(z) Taking z transform of y(n) - Y(z) - H(z)= Again, simplifying the above function to get into standard cascade form we ca write H(z) = = H1(z)+H2(z) H1(z)= H2(z)= The final structure is shown below
Fig 3 Cascade Form of H(z) = |
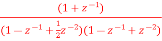 . Realise the cascade form?
. Realise the cascade form?A4) H(z)= Writing the above in standard form for cascade realisation H1(z)= H2(z)= The cascade structure is shown below
Fig 4 Cascade Form of H(z)= |
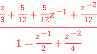
A5) H(z)= Taking Z common and then dividing the above function to convert it into standard form for parallel realisation we get H(z)=Z [ The parallel structure is shown below
Fig 5 Parallel Realisation of H(z)=
|
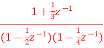
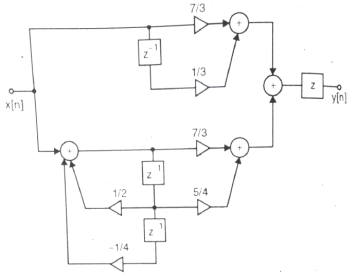
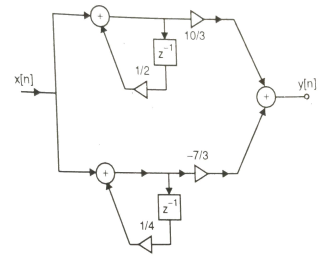
A6) Converting the above function to standard form using partial fraction technique H(z)= Solving for A and B we get A= 10/3 B= -7/3 H(z) = H1(z) = H2(z) = The parallel form realisation is shown below
Fig 6 Parallel Realisation of H(z)= |
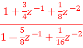 . Realise using cascade form?
. Realise using cascade form?A7) H(z) = Writing in standard form H(z) = H1(z) = H2(z) = The cascade structure is shown below
Fig 7 Cascade Form of H(z) =
Q8) Compute the N-point DFT of x(n)=3δ(n).
A8)
|
A9) We know that,
Substituting the value of x(n),
|
A10)
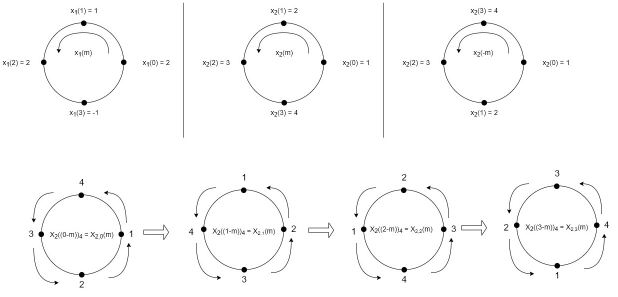
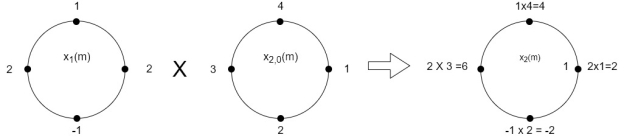
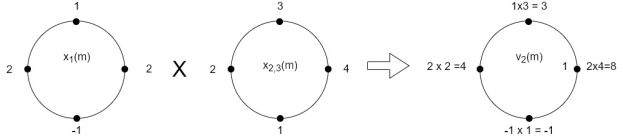
Fig 1 Circular Convolution of x1(n)= {2,1,2,-1} and x2(n)= {1,2,3,4}
(2)
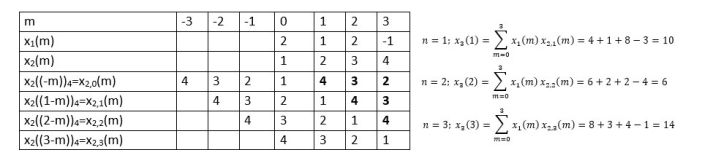
When n=0;
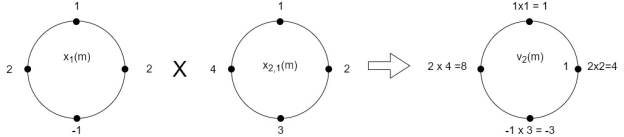
The sum of samples of v0(m) gives x3(0) ⸫ x3(0)=2+4+6-2=10 When n=1;
The sum of samples of v1(m) gives x2(1) ⸫ x3(1)=4 + 1 +8-3=10
(3)When n=2;
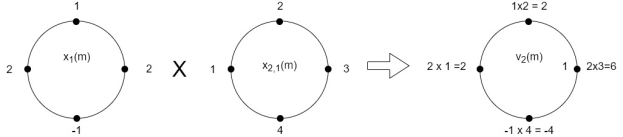
The sum of samples of v2(m) gives x3(2) ⸫ x3(2)=6+2+2-4=6
(4) When n=3;
The sum of samples of v3(m) gives x3(3) ⸫ x3(3)=8+ 3+ 4-1= 14 x3(n)={10,10,6,14}
= x1(0) x x2,0(0) + x1(1) x2,0(1) + x1(2) x2,0(2) + x1(3) x2,0(3) = 2 x 1 + 1 x 4 + 2 x 3 + (-1) x 2 = 2 +4 +6 -2 =10
|