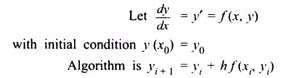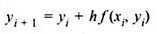undefined | unit 2 stability constraints in synchronous grids

PS2

Unit - 2

Stability Constraints in Synchronous Grids

Q1) Derive the Swing Equation of a Synchronous Machine?

A1) The differential equation that relates the angular momentum (M) the acceleration power (Pa) and the rotor angle (δ) is known as swing equation.

Consider the generator shown in the above figure. It receives mechanical power Pm at the shaft torque Tm and the angular speed ω via shaft from the prime-mover. It delivers electrical power Pe to the power system network. The generator develops electromechanical torque Te in opposition to the shaft torque Ts. At steady state, Tm = Te

Accelerating torque acting on the rotor is given by

Ta = Tm – Te

Multiplying by ω on both sides, we get power acceleration (Pa):

Pa = Pm – Pe

Pa=Ta ω = J*α*ω= M* α

Therefore, 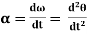= angular acceleration

, (

Θ – it is more convenient to measure the angular position of rotor with respect to synchronously rotation frame.

(δ=θ-ωt) of reference, ωt=0, hence

(δ=θ) then,

, This equation is known as Swing Equation

Incase damping power D is to be added then the equation is

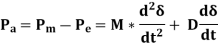
Q2) Derive the equation for Phase Angle in Swing Equation?

A2) Swing curve, plot of power angle δ vs time t, can be obtained by solving the swing equation. Fig. (a) Stable system and (b) unstable system.

Swing curves are used to determine the stability of the system. If the rotor angle δ reaches a maximum and then decreases, then it shows that the system has transient stability (figure-a). On the other hand, if the rotor angle δ increases indefinitely, then it shows that the system is unstable (figure-b).

Studying the stability of a generator connected to an infinite bus

Complex power is given by

S=P+jQ=VI* or P-jQ =V*I

Thus, the real power, P= Re (V*I)

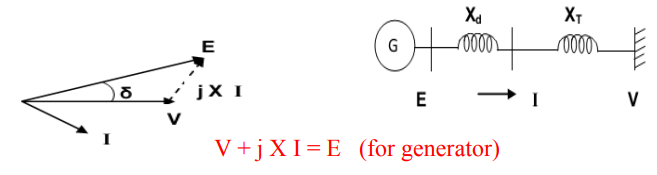
Where

V- is the voltage at infinite bus,

E- is the external voltage of generator,

X- is the total reactance

The internal voltage E leads V by angle δ, thus

Electric Output Power,

As a result of Swing Equation for generator is

The graphical plot of power angle equation in 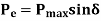for generator & motor is shown in figure

Q3) How can you reduce Loss of Synchronism in a bus system?

A3) a) Reducing the magnitude of disturbance: Large disturbances are typically due to faults which are cleared by line tripping. Reducing the duration of fault is one method of preventing loss of synchronism. These days, it is possible to clear faults within 3-5 cycles (taking into account relay and circuit breaker opening times).

b) Planning: This would mean augmenting the transmission network with new transmission lines. Increasing the power transfer capability of transmission lines by compensating transmission reactance with series capacitors and shunt capacitors.

c) Control: The short term or excess capacity of many equipment can be used to enhance stability by appropriate controls. For example, excitation systems can allow for short-term boosting of field voltage. Also, capacitors can be switched into the network in series and shunt. The switching may be done by means of circuit breakers or power electronic controls (power electronic controllers also allow for continuous control; this will be discussed in later modules).

d) Operation: During operation, it is ensured that the system is operated such that there exists sufficient "margin" for the system to withstand a credible disturbance (like a fault). This imposes a constraint on the level of power transfers which are permissible -- for larger levels of power flow, the synchronous generators are more likely to lose synchronism after a large disturbance. A system operator continuously monitors a power system and ensures that this is unlikely to happen. If the system is unlikely to withstand a credible disturbance, then preventive control actions like reducing the power transfer levels are taken. This may be achieved by re-scheduling power generated at various generators.

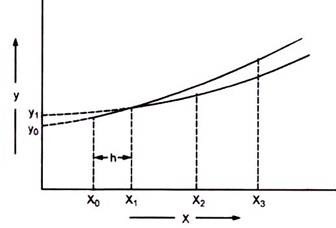
Q4) Derive the Euler’s method graphically?

A4) Euler’s Method:

It is a single step process.

To determine solution of xi + 1, we consider dependence at only one earlier point, given by xi.

The algorithm will be as follows:

Since we know the dependence at the earlier point from the initial condition, therefore, no difficulty occurs when determining solution at xi for the first order differential equation.

Also, the step size can be changed at any stage.

Geometrical integration of the above algorithm, given as per the equations shown here, can be interpreted from the figure below:

In the above figure, the part of the curve within the interval h is approximated by the segment of a straight line whose slope is the same as that at the start of the sub-interval.

This method has theoretical importance only.

Q5) Derive the Modified Euler’s equation?

A5)

This method is also called predictor-corrector method.

In this method, the slope is approximated at the middle of the interval.

The algorithm for the predictor formula is, as given below. It is the same as the Eulers’ method formula where we obtain yi+1 at xi + 1 with the initial condition of yi at xi.

The algorithm for the corrector formula is, as shown below. Using this formula, we apply correction to the value of yi + 1 as obtained with the help of corrector formula

Q6) Derive the Swing’s equation using Runge-Kutta 4th order?

A6) The swing equation can be written as-

, input power is assumed to be constant

The above equation can be split into 2 single order equations-

(i)

(ii)

We have ω & δ as a function of time t. Let us assume that x=t, y=δ & z=ω.

Hence, 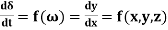and

The first step in the transient stability study is the load flow equation to obtain system condition disturbance. Each bus must be defined by P, Q, V, δ and 2 of them should be known. Let us assume that the ith machine is represented by a constant voltage source behind transient reactance .

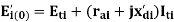, where

Eti= terminal voltage of i – th machine

rai= armature resistance of i-th machine

Iti= current through i-th machine

Also, in rectangular form

The initial internal voltage angle (power angle) is given by-

Machine current before disturbance calculated from-

for i=1, 2, 3…, m,

Where Pti & Qti are real & reactive power generation of i-th machine & Eti is the terminal voltage of i-th machine, taken as reference phasor

(0) initial mechanical input power= electrical air gap power 𝑃𝑒𝑖 prior to disturbance and can be obtained from-

For steady state, 𝛿0 = (0). For the transient analysis (when disturbance occurs due to fault), the network admittance matrix must be now changed to include equivalent circuit of machines and changes in loads. Each element representing a machine is a branch of a new bus and each element representing a load is a link to ground. According to Runge-kutta derivation, it is required to solve 2 simultaneous differential equations.

General expression of a Runge-Kutta 4th order numerical technique

Where,

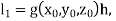
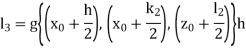
According to Runge-Kutta 4th order numerical technique

k’s & i’s are the changes δi & ωi respectively obtained using derivatives evaluated at predetermined points.

Q7) Derive the Swing’s Equation using Equal Area Criterion?

A7) The Equal Area Criterion is concerned with transient stability. It is in fact a very easy graphical method used. It is for deciding the transient stability of single machine or else two-machine system against infinite bus. Over a lossless line, the real power transmitted will be Pe=Pm sinδ. Consider a fault occurs in a synchronous machine which was operating in steady state. Here, the power delivered is given by Pe=Pm. For clearing a fault, the circuit breaker in the faulted section should have to be opened up. This process takes 5/6 cycles and the successive post-fault transient will take an additional few cycles. The prime mover which is giving the input power is driven with the steam turbine. For turbine mass system, the time constant is in the order of few seconds and for the electrical system, it is in milliseconds. Thus, while the electric transients take place, the mechanical power remains stable. The transient study mainly looks into the capability of the power system to retrieve from the fault and to give the stable power with a new probable load angle (δ).

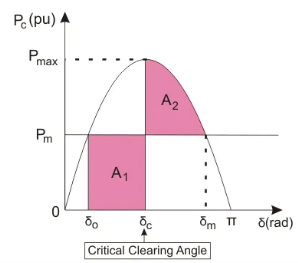
breakers opened and the real power is decreased to zero. But the Pm will be the power angle curve is considered which is shown in fig.1. Imagine a system delivering ‘Pm’ power on an angle of δ0 (fig.2) is working in a steady state. When a fault occurs; the circuit stable. As a result, accelerating power, Pa=Pm

The power differences will result in rate of change of kinetic energy stored within the rotor masses. Therefore, due to the stable influence of non-zero accelerating power, the rotor will accelerate. Consequently, the load angle (δ) will increase.
Now, we can consider an angle δc at which the circuit breaker re-closes. The power will then come back to the usual operating curve. At this moment, the electrical power will be higher than the mechanical power. But, the accelerating power (Pa) will be negative. Therefore, the machine will get decelerate. The load power angle will still continue to increase because of the inertia in the rotor masses. This increase in load power angle will stop in due course and rotor of the machine will start to decelerate or else the synchronization of the system will get lose.

The Swings equation is given by-

Multiply both sides by dt & integrate it for two arbitrary load angles which are δ0 & δc

Assume at the time of fault the machine will accelerate & after clearing of fault it will decelerate. Hence the required equations are

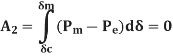
The system will reach steady state.

When A2 = A1, the margin of the stability limit is defined by this condition. Here, the clearing angle is given by δcr, the critical clearing angle.

Since, A2 = A1, we will get

The critical clearing angle is related to the equality of areas, it is termed as equal area criterion. It can be used to find out the utmost limit on the load which the system can acquire without crossing the stability limit.

Q8) What is the Impact of stability constraints on Power System?

A8) During operation, it is ensured that the system is operated such that there exists sufficient margin for the system to withstand a disturbance. It is ensured that phase angular difference across transmission paths is not too large. Thus, angular stability puts a limit on the levels of power which can be transferred securely. One of the constraints for long distance AC transmission (other than thermal / voltage constraints) is the large phase angular difference which is required to transmit a given amount of power. The modified load ability of a line by taking into account stability limits is shown in the figure on the right. It is not easy to specify stability limits as they depend on the parameters of power system components and the operating conditions. However, for convenience it is specified by the maximum phase angular difference which is allowed across the line.

Long distance transmission requires us to use high voltages and lower currents (otherwise resistive losses will be too large). So, step-up will still be required (it is not feasible (as yet) to generate power by ac or dc machines at high voltage). So, transformers will be used to step-up generated ac voltage to higher ac voltage, and then rectify it to DC using power electronic converters. DC is again inverted to ac at the receiving end of the line. Thus, a dc interconnection will require 2 large ac to dc converters at either end. Voltage profile along a line is not an issue since series inductive and shunt capacitive effects are not manifested in steady state for DC transmission. Resistive drop is usually small. Power Flow can be controlled using firing angle of thyristors used in the rectifier and inverter. Power flow is independent of the phase angular difference between sending (rectifier) and receiving (inverter) ends. So angular stability constraint is not there. In fact, if two areas are connected by only dc inter-connections, then even frequency of the two areas need not be the same.

Q9) What is the impact on stability using Series Compensation?

A9) 1. The lower line impedance improves stability. When capacitor bank is inserted into the transmission line, the rotor angle (δ) reduces for the same amount of power transfer due the effect of compensation. Reduction in angle δ allows rotor to operate at a lower rotor angle with increased stability limit.

2. The lower line impedance improves voltage regulation. By inserting capacitor bank into the transmission line net impedance of the line reduces resulting into lesser voltage drop along the transmission line and better voltage regulation.

3. Adding series capacitance provides a method of controlling the division of load among several lines. By controlling degree of compensation of capacitor bank installed in a several bus systems, amount of the load shared among the lines can be controlled. It gives the better control of load among several lines.

4. Increasing the loading capacity of a line improves the utilization of the transmission system and therefore the return on the capital investment. Series compensated transmission lines allow power transfer at the same voltage level over longer transmission lines than uncompensated lines. This better utilizes the existing transmission network, which is cost effective and quicker rather than building new or additional parallel lines

5. Increase power transfer capacity.

Q10) What is Generation Rescheduling?

A10) Generators are rescheduled such that it doesn’t exceed the transfer limits of the transmission line. It is done based on creating a population containing generation limits which satisfies the demand. The rescheduling of generators makes the generation to operate at an equilibrium away from the one determined by equal incremental costs. Mathematical models are involved for obtaining the corresponding cost signals. The results are used in congestion pricing as an indicator for the market participants in rearranging the power injections & extractions. Rescheduling cost is the cost involved in rescheduling the generation.

Q11) Define an Infinite Bus?

A11) A system having a constant voltage and constant frequency regardless of the load is called an Infinite Bus bar system. Thus, an infinite bus has a large power system. The amount of real and reactive power is drawn or supplied, does not affect its voltage and frequency. They both remain constant. In a power system, normally more than one alternator operates in parallel. The machine may be located at different places. A group of machines located in one place may be treated as a single large machine. The machine connected to the same bus, but separated by transmission lines of low reactance, may be grouped into one large machine. The operation of one machine connected in parallel with such a large system comprising many machines is of great interest. The capacity of the system is so large that its voltage and frequency is considered constant. The connection or disconnection of a single small machine or a small load on such a system would not affect the magnitude and phase of the voltage and frequency. The system behaves like a large generator having internal impedance zero and infinite rotational inertia.

Q12) Draw the flow chart of Power System stability?

A12)

Q13) What happens when machine is in synchronization?

A13) When a synchronous machine is connected to a grid, it is important to follow a particular procedure so that the transients which occur are minimised and the incoming generator is "pulled into synchronism". This includes ensuring that the generator voltages and the system voltages are in the same phase sequence and the voltage magnitude of the generator and the grid at the point of interconnection are nearly equal. Additionally: a) The electrical speed of the incoming generator should be almost equal to the grid frequency. b) The phase angular difference between the generator voltage and the grid voltage at the point of interconnection at the instant of interconnection should be small. The situation is analogous to connecting two moving masses by a spring. To avoid excessive stretch in the spring when the masses are inter-connected (which may cause the spring to break), one would need to have the two masses to be close to each other and to be moving at nearly the same speed at the time of interconnection. If the interconnection is done smoothly, without breakage of the spring, then the masses move together at the same speed after transients die out. Thereafter, the masses are held together by the spring unless a large disturbance causes the spring to break. In practice, a plant operator starts rotating the generator by introducing a prime mover torque. He ramps up the speed to a value which is very close to the grid frequency by adjusting the prime mover torque. He then switches on the field excitation and brings voltage close to the grid voltage at the point of interconnection by adjusting the field voltage. Using an instrument called a synchroscope, he monitors the phase angular difference and switches on the interconnecting circuit breaker at the instant when the phase angular difference is very small.

Q14) How does one prevent loss of synchronism due to disturbances?

A14) a) Reducing the magnitude of disturbance: Large disturbances are typically due to faults which are cleared by line tripping. Reducing the duration of fault is one method of preventing loss of synchronism. These days, it is possible to clear faults within 3-5 cycles (taking into account relay and circuit breaker opening times).

Q15) Why DC transmission is preferred especially when transmitting power over long distances?

A15) Long distance transmission requires us to use high voltages and lower currents (otherwise resistive losses will be too large). So, step-up will still be required (it is not feasible (as yet) to generate power by ac or dc machines at high voltage). So, transformers will be used to step-up generated ac voltage to higher ac voltage, and then rectify it to DC using power electronic converters. DC is again inverted to ac at the receiving end of the line. Thus, a dc interconnection will require 2 large ac to dc converters at either end. 2. Voltage profile along a line is not an issue since series inductive and shunt capacitive effects are not manifested in steady state for DC transmission. Resistive drop is usually small. 3. Power Flow can be controlled using firing angle of thyristors used in the rectifier and inverter. Power flow is independent of the phase angular difference between sending(rectifier) and receiving(inverter) ends! So angular stability constraint is not there. In fact, if two areas are connected by only dc inter-connections, then even frequency of the two areas need not be the same.

Q16) Define Power System Stability?

A16) Power system stability refers to the ability of synchronous machines to move from one steady-state operating point following a disturbance to another steady-state operating, without losing synchronism. There are three types of power stability:

1-Steady-state: involves slow or gradual changes in operating due to variation on loads.

2-Transient: Involves major disturbances such as (loss of generation due to faults and sudden load changes). This case deviations from synchronous frequency (50 Hz), and changing the machine power angles (our subject). In many cases, transient stability is determined during the first swing of machine power angles following a disturbance (typically 1s).

3-Dynamic: After a fault, an individual synchronous machine may remain in synchronism during the first swing. After this, strong electromechanical oscillations occur may cause the system to have natural oscillations. The system is said to be dynamically stable.

Q17) Define Critical Clearing Angle & Critical Clearing Time?

A17) If a fault occurs in a system, δ begins to increase under the influence of positive accelerating power, and the system will become unstable. * if δ becomes very large. There is a critical angle within which the fault must be cleared. This angle is known as the critical clearing angle. Consider a system in figure operating with mechanical input Pm at steady angle δ0 (Pm =Pe) at point 'a' on the power angle diagram shown in the diagram in the next slide this is steady state.

If a three-phase short circuit occurs at the point F of the outgoing radial line, the terminal voltage goes to zero and electrical power output of the generator reduces to zero (Pe = 0) and the state point drops to 'b'. The acceleration area A1 starts to increase while the state point moves along bc. At time t c corresponding clearing angle δC, the fault is cleared by the circuit breaker. t c is called clearing time and δC is called clearing angle. After the fault is cleared, the system becomes healthy and transmits power Pe = Pmax sinδ, the state point shifts to "d" on the power angle curve. The rotor now decelerates and the decelerating area A2 begins to increase while the state point moves along de.

Q18) What are the different methods for Voltage Stability Analysis?

A18) Different methods exist in the literature for carrying out a steady state voltage stability analysis. The conventional methods can be broadly classified into the following types.

1. P-V curve method.

2. V-Q curve method and reactive power reserve.

3. Methods based on singularity of power flow Jacobian matrix at the point of voltage collapse.

4. Continuation power flow method

Q19) What is V-Q curve method and reactive power reserve?

A19) The V-Q curve method is one of the most popular ways to investigate voltage instability problems in power systems during the post transient period. Unlike the P-V curve method, it doesn’t require the system to be represented as two-bus equivalent. Voltage at a test bus or critical bus is plotted against reactive power at that bus. A fictitious synchronous generator with zero active power and no reactive power limit is connected to the test bus. The power-flow program is run for a range of specified voltages with the test bus treated as the generator bus. Reactive power at the bus is noted from the power flow solutions and plotted against the specified voltage. The operating point corresponding to zero reactive power represents the condition when the fictitious reactive power source is removed from the test bus. Voltage security of a bus is closely related to the available reactive power reserve, which can be easily found from the V-Q curve of the bus under consideration. The reactive power margin is the MVAR distance between the operating point and either the nose point of the V-Q curve or the point where capacitor characteristics at the bus are tangent to the V-Q curve. Stiffness of the bus can be qualitatively evaluated from the slope of the right portion of the V-Q curve. The greater the slope is, the less stiff is the bus, and therefore the more vulnerable to voltage collapse it is. Weak busses in the system can be determined from the slope of V-Q curve. For the simple two-bus system shown in Figure 1.2, equations of V-Q curves for constant power loads can be derived as follows. the power angle δ is computed for specified active power and used in for a range of values of voltage and different active power levels, normalized V-Q curves are shown in. The critical point or nose point of the characteristics corresponds to the 7 voltages where dQ/dV becomes zero. If the minimum point of the V-Q curve is above the horizontal axis, then the system is reactive power deficient. Additional reactive power sources are needed to prevent a voltage collapse. Curves for p=1.00 and p=0.75 signify reactive power deficient busses. Busses having V-Q curves below the horizontal axis have a positive reactive power margin. The system may still be called reactive power deficient, depending on the desired margin.

Q20) What are the factors affecting Transient Stability?

A20) Various methods which improve power system transient stability are

1. Improved steady-state stability a) Higher system voltage levels b) Additional transmission line c) Smaller transmission line series reactance d) Smaller transfer leakage reactance e) Series capacitive transmission line compensation f) Static var compensators and flexible ac transmission systems (FACTs)

2. High speed fault clearing

3. High speed reclosure of circuit breaker

4. Single pole switching

5. Large machine inertia, lower transient reactance

6. Fast responding, high gain exciter

7. Fast valving

8. Breaking resistor

Sign Up