Unit - 2

Introduction to bulk and sheet metal forming

Q1. A 300-mm-wide strip 25-mm thick is fed through a rolling mill with two powered rolls each of radius = 250 mm. The work thickness is to be reduced to 22 mm in one pass at a roll speed of 50 rev/min. The work material has a flow curve defined by K = 275 MPa and n = 0.15, and the coefficient of friction between the rolls and the work is assumed to be 0.12. Determine if the friction is sufficient to permit the rolling operation to be accomplished. If so, calculate the roll force, torque, and horsepower.

ANS:

The draft attempted in this rolling operation is

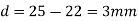
the maximum possible draft for the given coefficient of friction is

Since the maximum allowable draft exceeds the attempted reduction, the rolling operation is feasible. To compute rolling force, we need the contact length L and the average flow stress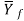. The contact length is given by

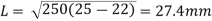
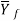is determined from the true strain:

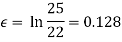
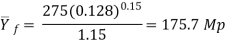
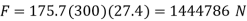
and the power is obtained as

Q2. A cylindrical workpiece is subjected to a cold upset forging operation. The starting piece is 75mm in height and 50mm in diameter. It is reduced in the operation to a height of 36mm.The work material has a flow curve defined by K = 350MPa and n = 0.17.Assume a coefficient of friction of 0.1.Determine the force as the process begins, at intermediate heights of 62mm, 49 mm, and at the final height of 36 mm.

ANS:

Workpiece volume

At the moment contact is made by the upper die, h = 75mmandthe force F = 0.At the start of yielding, h is slightly less than 75 mm, and we assume that strain = 0.002, at which the flow stress is

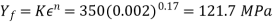
The diameter is still approximately D = 50mmand area

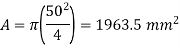
For these conditions, the adjustment factor Kf is computed as

The forging force is

At h = 62 mm,

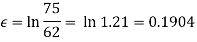
Assuming constant volume, and neglecting barreling,

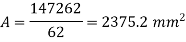
And

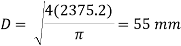
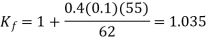
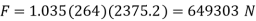
Similarly, at h = 49 mm, F = 955,642 N; and at h = 36 mm, F = 1,467,422 N

Q3. A billet 75mmlong and 25mmin diameter is to be extruded in a direct extrusion operation with extrusion ratio rx = 4.0. The extrudate has a round cross section. The die angle (half angle) = 900. The work metal has a strength coefficient = 415 MPa, and strain-hardening exponent = 0.18. Use the Johnson formula with a = 0.8 and b = 1.5 to estimate extrusion strain. Determine the pressure applied to the end of the billet as the ram moves forward.

ANS: Let us examine the ram pressure at billet lengths of L =75mm (starting value), L = 50 mm, L = 25 mm, and L =0.We compute the ideal true strain, extrusion strain using Johnson’s formula, and average flow stress:

L = 75mm:With a die angle of 900, the billet metal is assumed to be forced through the die opening almost immediately; thus, our calculation assumes that maximum pressure is reached at the billet length of 75mm. For die angles less than 90, the pressure would build to a maximum as the starting billet is squeezed into the cone-shaped portion of the extrusion die.

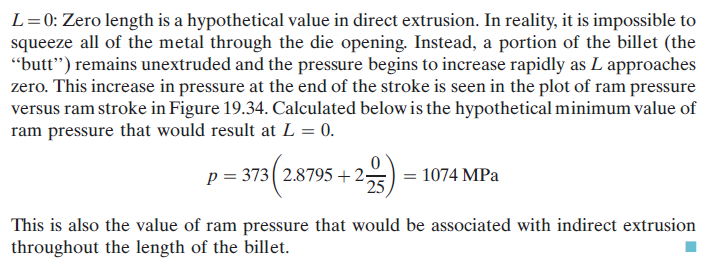
Q4. Wire is drawn through a draw die with entrance angle =150. Starting diameter is 2.5 mm and final diameter = 2.0 mm. The coefficient of friction at the work–die interface = 0.07. The metal has a strength coefficient K = 205 MPa and a strain-hardening exponent n = 0.20. Determine the draw stress and draw force in this operation.

ANS:

The values of D and Lc can be determined as.

Q5. A round disk of 150-mm diameter is to be blanked from a strip of 3.2-mm, half-hard cold rolled steel whose shear strength = 310 MPa. Determine (a) the appropriate punch and die diameters, and (b) blanking force.

ANS: The clearance allowance for half-hard cold-rolled steel is Ac = 0.075. Accordingly,

The blank is to have a diameter = 150 mm, and die size determines blank size. Therefore,

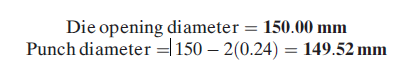
To determine the blanking force, we assume that the entire perimeter of the part is blanked at one time. The length of the cut edge is

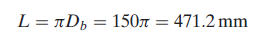
and the force is

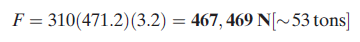
Q6. A sheet-metal blank is to be bent. The metal has a modulus of elasticity = 205 (103) MPa, yield strength = 275 MPa, and tensile strength = 450 MPa. Determine (a) the starting blank size and (b) the bending force if a V-die is used with a die opening dimension = 25 mm.

ANS: (a)The starting blank =44.5 mm wide. Its length = 38+Ab+25 (mm).For the included angle α’ =1200, the bend angle α = 600.The value of Kba = 0.33 since R/t = 4.75/3.2 =1.48 (less than 2.0).

Q7. A drawing operation is used to form a cylindrical cup with inside diameter = 75 mm and height = 50mm. The starting blank size = 138 mm and the stock thickness = 2.4mm. Based on these data, is the operation feasible?

ANS:

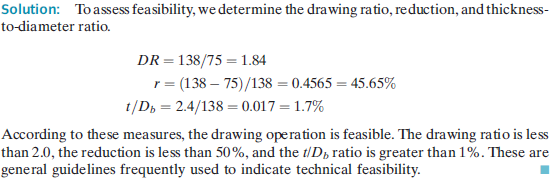
Q8. A blanking die is to be designed to blank the part outline shown in the figure below. The material is 4 mm thick stainless steel (the allowance for the stainless steel is a = 0.075). Determine the dimensions of the blanking punch and the die opening.

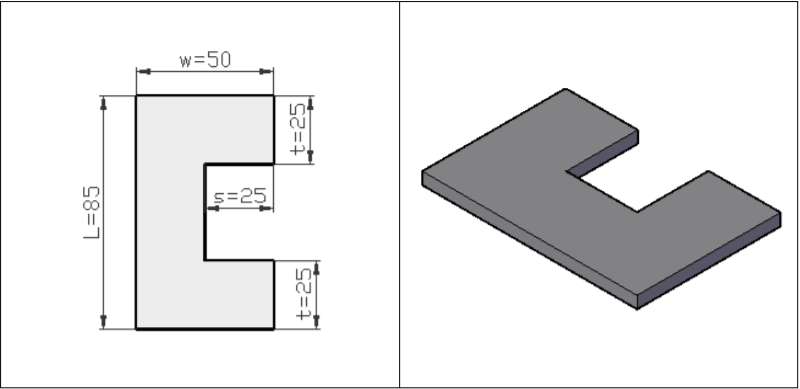
ANS:

Since a = 0.075, the clearance is given by,

c = 0.075 (4) = 0.3 mm.

Blanking die dimensions: the same as for the part in the figure:

L = 85 mm w = 50 mm t = 25 mm s = 25 mm

Blanking punch dimensions:

Length L = 85 - 2(0.3) = 84.4 mm

Width w = 50 - 2(0.3) = 49.4 mm

Top and bottom t widths = 25 - 2(0.3) = 24.4 mm

The s = 25 mm inset dimension remains the same.

Q9. A blanking operation is to be performed on 2 mm thick cold rolled steel. The part is circular with diameter = 75 mm. Determine: a) the appropriate punch and die sizes for this operation if the allowance for the cold rolled steel is a = 0.075. b) the blanking force required if the steel has a shear strength = 325 MPa and the tensile strength is 450 MPa

ANS:

(a) Since a = 0.075, the clearance is given by,

c = 0.075 (2) = 0.15 mm.

 Thus the Punch diameter Dh is calculated as

 Dh = Db - 2c = 75.0 - 2(0.15) = 74.70 mm.

and the Die diameter is Db

= 75 mm.

(b) the blanking force is given by

F = StL

The thick of the metal stock t is given by the problem as t = 2 mm

The length of cut edge is calculated as:

L = πD = 75π = 235.65 mm

 Thus the blanking force is

F = 325 (2) (235.65) = 153,200 N

Q10. A cup is to be drawn in a deep drawing operation. The height of the cup is 75 mm and it’s inside diameter = 100 mm. The sheet metal thickness = 2 mm. If the blank diameter = 225 mm, determine (a) drawing ratio, (b) reduction, and (c) thickness-to-diameter ratio. (d) Does the operation seem feasible?

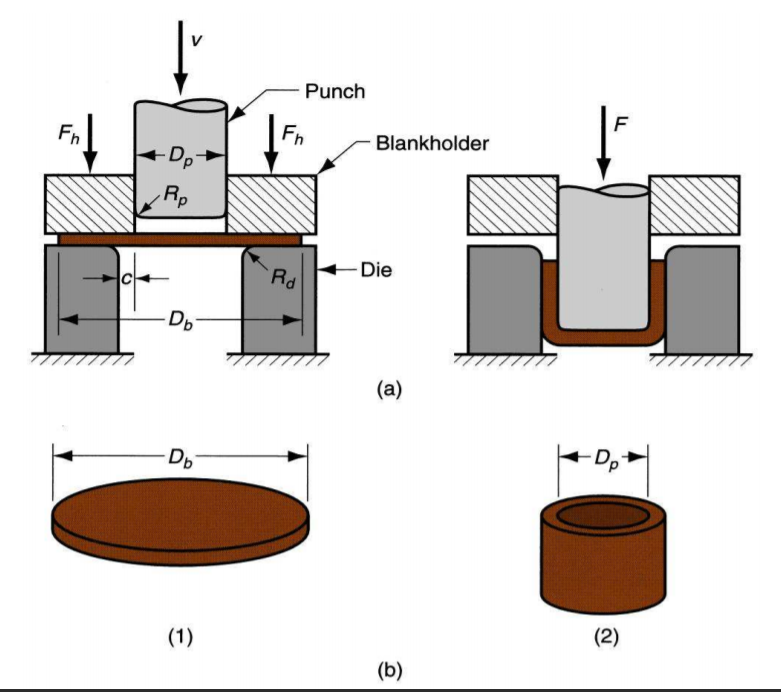
ANS: (a) Drawing ratio DR = Db/Dp = 225/100 = 2.25

(b) Reduction r = (Db - Dp)/Db = (225 - 100)/225 = 0.555 = 55.5%

(c) Thickness-to-diameter ratio t/Db = 2/225 = 0.0089 = 0.89%

(d) Feasibility? No!

DR is too large (greater than 2),

 r is too large (greater than 50%),

and t/D is too small (less than 1%)