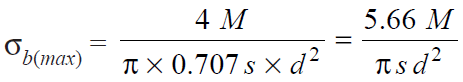Module - 3
Riveted Joints
Q1) Define the rivet joint. Explain the terminology in rivet joint.
A1) A Rivet is a short cylindrical rod having a head and a tapered tail. The main body of the rivet is called shank.
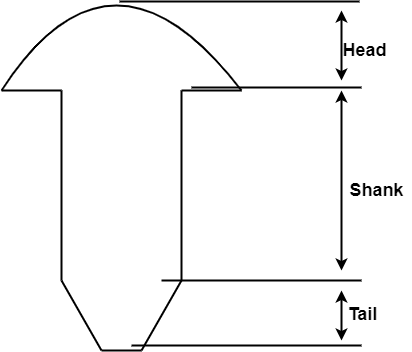
Figure 1: Rivet joint
Important terms used in rivet joints
Few parameters, which are required to specify arrangement of rivets in riveted joints are as follows:
a) Pitch: This is the distance between two centres of the consecutive rivets in a Single row (usual symbol p).
b) Back Pitch: This is the shortest distance between two successive rows in a multiple riveted joint (usual symbol bp).
c) Diagonal pitch: This is the distance between the centres of rivets in adjacent rows of zigzag riveted joint (usual symbol pd).
d) Margin or marginal pitch: This is the distance between the centres of the rivet hole to the nearest edge of the plate (usual symbol m).
Q2) Derive the expression for rivet joints for designing the boiler tank.
A2) Design of boiler joints
In general, for longitudinal joint, butt joint is adopted, while for circumferential joint, lap joint is preferred.
Design of longitudinal butt joint
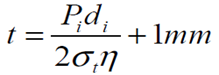
Where,
1 mm is the allowance for corrosion
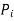 = Internal steam pressure
= Internal steam pressure
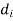 = Internal diameter of the boiler shell
= Internal diameter of the boiler shell
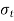 = Permissible stress of the shell material
= Permissible stress of the shell material
2. Diameter of rivets: The diameter of the rivets may be determined from the empirical relation, d = 6 t for t ≥ 8mm
KEYWAY POINT:
1) The diameter of rivet should not be less than the plate thickness.
2) If the plate thickness is less than 8 mm, the diameter of the rivet is determined by equating the shearing resistance of the rivet to its crushing resistance.
3. Pitch of the rivets: The pitch of the rivets may be obtained by equating the tearing resistance of the plate to the shearing resistance of the rivets. However, it should be noted that,
1) The pitch of the rivets should not be less than 2d.
2) The maximum value of the pitch, for a longitudinal joint is given by,
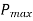 = ct + 41.28mm where ‘c’ is a constant.
= ct + 41.28mm where ‘c’ is a constant.
Note: If the pitch of the rivets obtained by equating the tearing resistance to the shearing resistance is more than Pmax, then the value of Pmax can be adopted.
4. Row (transverse) pitch:
(i). For equal number of rivets in more than one row for lap joint or butt joint, the row pitch should not be less than, 0.33 p + 0.67d for zig-zag riveting and 2d, for chain riveting.
(ii). For joints in which the number of rivets in the rows is half the number of rivets in the inner rows, and if the inner rows are chain riveted, the distance between the outer row and the next row, should not be less than, 0.33 p + 0.67d or 2d, whichever is greater. The distance between the rows in which there are full number of rivets, should not be less than 2d.
(iii). For joints in which the number of rivets in outer row is half the number of rivets in inner rows, and if the inner rows are zig-zag riveted, the distance between the outer row and the next row, should not be less than, 0.2 p +1.15d. The distance between the rows in which there are full number of rivets (zig-zag), should not be less than, 0.165 p + 0.67d.
Note: p is the pitch of the rivets in the outer row.
5. Thickness of butt straps: The thickness of butt strap(s) is given by, (in no case it should not be less than 10 mm).
 = 1.125t , for ordinary single butt strap (chain riveting)
= 1.125t , for ordinary single butt strap (chain riveting)
When two unequal widths of butt straps are employed, the thickness of butt straps are given by, 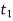 = 0.75t , for wide strap on the inside and
= 0.75t , for wide strap on the inside and 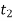 = 0.625t , for narrow strap on the outside.
= 0.625t , for narrow strap on the outside.
Note: The thickness of butt strap, in no case, shall be less than 10 mm.
6. Margin: The margin ‘m’ is generally followed as 1.5d.
Q3) Define structural joint and also mention the important terminology.
A3) The movements in hardened material that can cause cracking can originate from:
Joints will be designated by terminology based on the following characteristics: resistance, configuration, formation, location, type of structure and function.
Q4) Explain the stress in bolt joint.
A4) Initial tightening load
When a nut is tightened over a screw following stresses are induced:
a) Tensile stresses due to stretching of the bolt
b) Torsional shear stress due to frictional resistance at the threads.
c) Shear stress across threads
d) Compressive or crushing stress on the threads
e) Bending stress if the surfaces under the bolt head or nut are not perfectly normal to the bolt axis.
The initial tension in the bolt may be estimated by an empirical relation P1=284 d kN, where the nominal bolt diameter d is given in mm. The relation is used for making the joint leak proof.
If leak proofing is not required half of the above estimated load may be used. However, since initial stress is inversely proportional to square of the diameter-
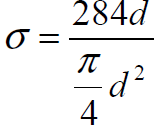
Bolts of smaller diameter such as M16 or M8 may fail during initial tightening.
Q5) Discuss design of bolt joints with centre and eccentric load.
A5) There are many applications of the bolted joints which are subjected to eccentric loading such as a wall bracket, pillar crane, etc. The eccentric load may be
Eccentric Load Acting Parallel to the Axis of Bolts:
Consider a bracket having a rectangular base bolted to a wall by means of four bolts as shown in Fig. 3A little consideration will show that each bolt is subjected to a direct tensile load of 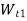 =
= 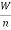
Where n is the number of bolts.
Figure: Eccentric load acting parallel to the axis of bolts.
Let w be the load in a bolt per unit distance due to the turning effect of the bracket and let 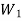 and
and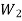 be the loads on each of the bolts at distances
be the loads on each of the bolts at distances 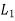 and
and 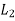 from the tilting edge.
from the tilting edge.
Load on each bolt at distance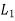 ,
,
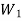 = w.
= w.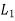
and moment of this load about the tilting edge
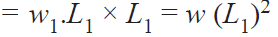
Similarly, load on each bolt at distance 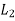 ,
,
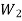 = w.
= w.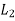
And moment of this load about the tilting edge
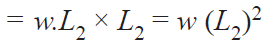
Total moment of the load on the bolts about the tilting edge
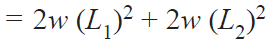 …..(i)
…..(i)
Also, the moment due to load W about the tilting edge
= W.L …..(ii)
From equations (i) and (ii), we have
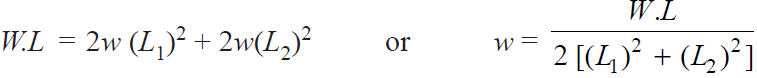 …..(iii)
…..(iii)
The most heavily loaded bolts are those which are situated at the greatest
distance from the tilting edge. Tensile load on each bolt at distance L2,
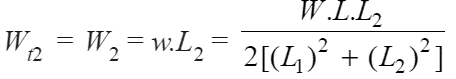
and the total tensile load on the most heavily loaded bolt,
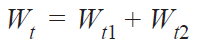 ……(iv)
……(iv)
If dc is the core diameter of the bolt and σt is the tensile stress for the bolt material, then total tensile load,
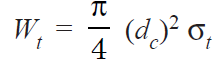 …….(v)
…….(v)
From equations (iv) and (v), the value of dc may be obtained.
Eccentric Load Acting Perpendicular to the Axis of Bolts
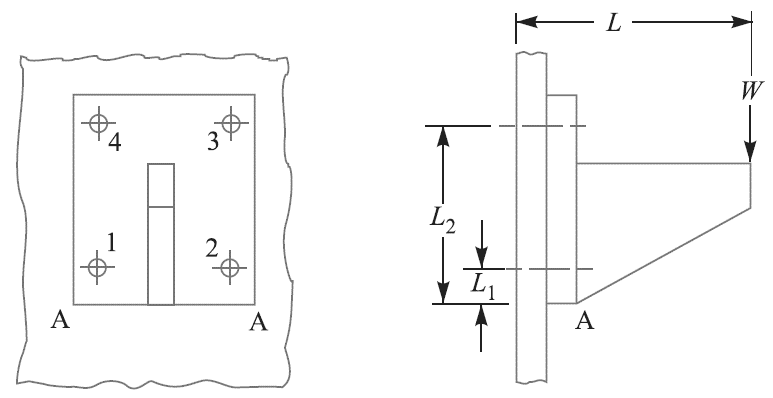
Figure: Eccentric load perpendicular to the axis
The bolts are subjected to direct shearing load which is equally shared by all the bolts. Therefore, direct shear load on each bolts,
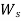 = W/n, where n is number of bolts.
= W/n, where n is number of bolts.
In this case, bolts 3 and 4 are heavily loaded.
∴ Maximum tensile load on bolt 3 or 4,
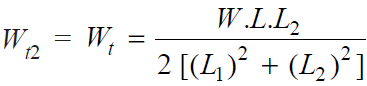
When the bolts are subjected to shear as well as tensile loads, then the equivalent loads may be determined by the following relations:
Equivalent tensile load,
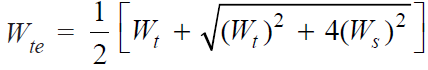
And equivalent shear load,
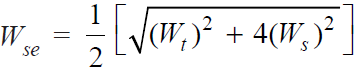
Knowing the value of equivalent loads, the size of the bolt may be determined for the given allowable stresses.
Q6) Discuss design of bolt joints on a bracket with circular base with eccentric load.
A6) Eccentric Load on a Bracket with Circular Base
Consider a round flange bearing of a machine tool having four bolts
as shown in Figure 5.
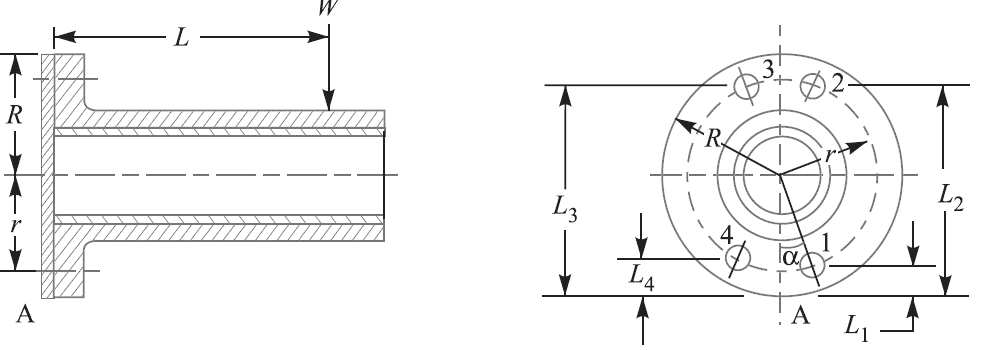
(a) (b)
Figure 5: Eccentric load on a bracket with circular base.
Let R = Radius of the column flange,
r = Radius of the bolt pitch circle,
w = Load per bolt per unit distance from the tilting edge,
L = Distance of the load from the tilting edge, and
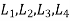 = Distance of bolt centres from the tilting edge A.
= Distance of bolt centres from the tilting edge A.
On equating the external moment W × L to the sum of the
resisting moments of all the bolts,
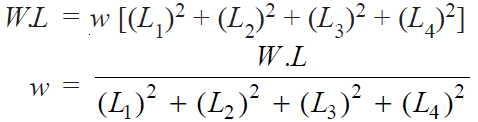 ………….(i)
………….(i)
From the geometry of the Fig.(b),
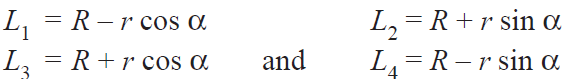
Substituting these values in equation (i),
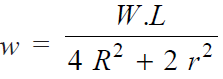
∴ Load in the bolt situated at 1 is
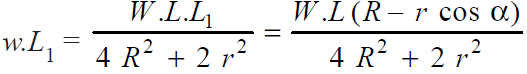
This load will be maximum when cos α is minimum i.e. when cos α = – 1 or α = 180°.
∴ Maximum load in a bolt is
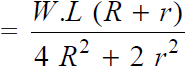
In general, if there are n number of bolts, then load in a bolt
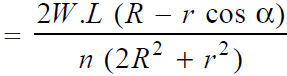
and maximum load in a bolt,
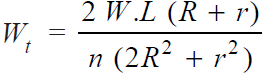
If the direction of load is fixed, then the maximum load on the bolts may be reduced by locating the bolts in such a way that two of them are equally stressed. In such a case, maximum load is given by
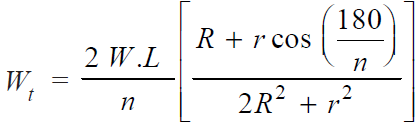
Q7) Discuss the category of welded joints.
A7) Categories of welded joints
Welded joints are primarily of two kinds: -
a) Lap or fillet joint: obtained by overlapping the plates and welding their edges. The fillet joints may be single transverse fillet, double transverse fillet or parallel fillet joints.
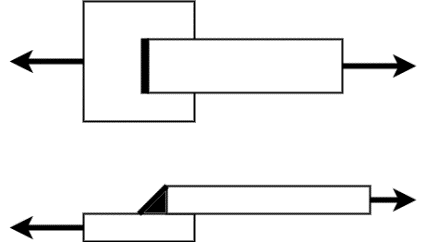
Figure: Single transverse lap joint
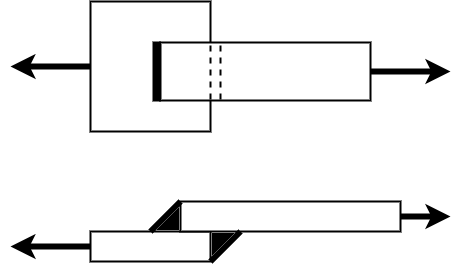
Figure: Double transverse lap joint

Figure: Parallel lap joint
b) Butt joints: formed by placing the plates edge to edge and welding them. Grooves are sometimes cut (for thick plates) on the edges before welding. According to the shape of the grooves, the butt joints may be of different types, e.g.,
a) Square butt joint

b) Single V-butt joint, double V-butt joint

c) Single U-butt joint, double U-butt joint
d) Single J-butt joint, double J-butt joint
e) Single bevel-butt joint, double bevel butt joint

Q8) Mention the Design of welded joints subjected to axial load.
A8) Axially Loaded Unsymmetrical Welded Sections
Consider an angle section as shown in Figure.
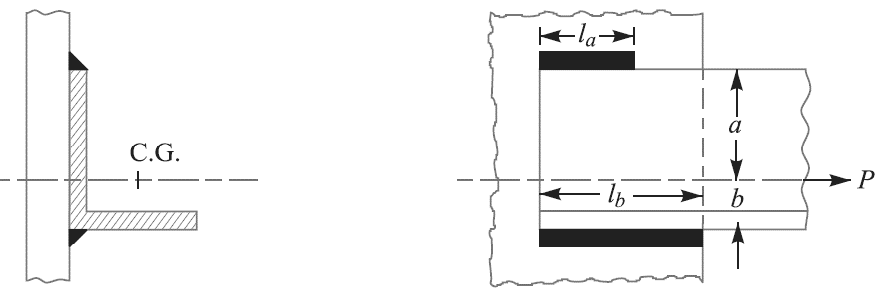
Figure: Axially loaded unsymmetrical welded section
Let 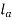 = Length of weld at the top,
= Length of weld at the top,
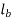 = Length of weld at the bottom,
= Length of weld at the bottom,
l = Total length of weld = 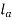 +
+ 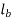
P = Axial load,
a = Distance of top weld from gravity axis,
b = Distance of bottom weld from gravity axis, and
f = Resistance offered by the weld per unit length.
Moment of the top weld about gravity axis= la × f × a
and moment of the bottom weld about gravity axis= lb× f × b
Since the sum of the moments of the weld about the gravity axis must be zero, therefore,
la × f × a – lb× f × b = 0
or la × a = lb× b ...(i)
We know that l = la + lb...(ii)
∴ From equations (i) and (ii), we have
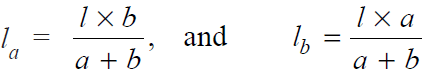
Q9) Mention the Design of welded joints subjected to torsional load.
A9) Consider a vertical plate attached to a horizontal plate by two identical fillet welds as shown in Figure given below:
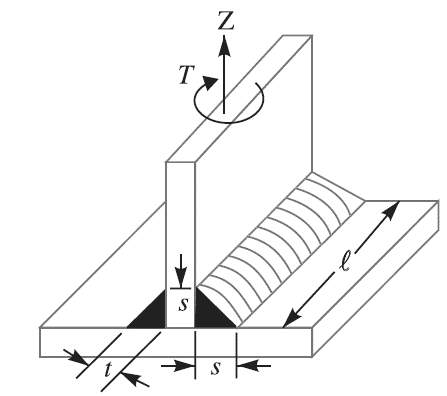
Figure: Long fillet weld subjected to torsion.
Let T = Torque acting on the vertical plate,
l = Length of weld,
s = Size (or leg) of weld,
t = Throat thickness, and
J = Polar moment of inertia of the weld section
The effect of the applied torque is to rotate the vertical plate about the Z-axis through its mid point. This rotation is resisted by shearing stresses developed between two fillet welds and the horizontal plate. It is assumed that these horizontal shearing stresses vary from zero at the Z-axis and maximum at the ends of the plate. This variation of shearing stress is analogous to the variation of normal stress over the depth (l) of a beam subjected to pure bending.
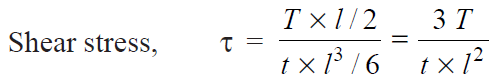
The maximum shear stress occurs at the throat and is given by-
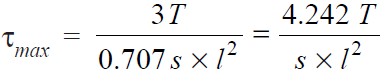
Q10) Mention the Design of welded joints subjected to bending load.
A10) Consider a circular rod connected to a rigid plate by a fillet weld as shown in Figure given below.
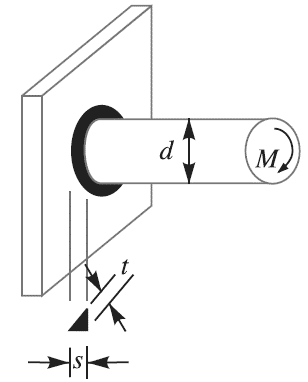
Figure: Circular fillet weldsubjected to bending moment
Let d = Diameter of rod,
M = Bending moment acting on the rod,
s = Size (or leg) of weld,
t = Throat thickness,
Z = Section modulus of the weld section
=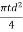
We know that the bending stress,
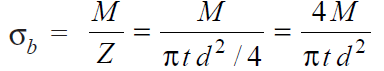
The maximum bending stress occurs on the throat of the weld which is inclined at 45° to the horizontal plane.
∴ Length of throat, t = s*sin 45° = 0.707*s
And maximum bending stress,