Unit-5
Design of transmission Elements
Q1) Explain spur gear with design considerations.
A1) Spur gear:
The gears connecting two parallel and coplanar shafts are known as spur gear. System of gear teeth:
b. The 14.50 composite systems are used for general purpose gears. It is stronger but has no interchange ability. The tooth profile of this system has cycloidal curves at the top and bottom and involute curve at the middle portion.
c. The tooth profile of the 14.50 full depth involute systems was developed for use with gear hobs for spur and helical gears.
d. The tooth profile of the 200 full depth involute systems may be cut by the hobs. The increase of the pressure angle from14.50 to 200 results in a stronger tooth, because the tooth acting as a beam is wider at the base.
e. The 200 stub involute systems have a strong tooth to take heavy loads.
Contact ratio:
It is defined as the ratio of the length of the arc of contact to the circular pitch.
Contact ratio=Length of arc of contact/p
Where, p=circular pitch=πm
Design considerations:
Following are the considerations met in the design of gear drive:
Q2) Explain the gear tooth strength for spur gear.
A2) It is determined from an equation known as Lewis and the load carrying ability of the toothed gear as determined by this equation gives satisfactory results.
Consider each tooth as cantilever beam loaded by a normal load (WN)
It is resolved into two components that is tangential (WT) component and radial component (WR) acting perpendicular and parallel to the line of tooth respectively.
The tangential component (WT) induces a bending stress which tends to break the tooth.
The radial component (WT) induces a compressive stress of relatively small magnitude; therefore its effect on the tooth may be neglected. Hence, the bending stress is used as the basis for design calculations.
The critical or the section of maximum bending stress may be obtained by drawing a parabola through A and tangential to the tooth curves at B and C.
The tooth is larger than the parabola at every section except BC is the section of maximum stress or the critical section.
Let, h=Length of the tooth
T= thickness of the tooth
b =width of the face
The maximum value of the bending stress at the section BC is given by
σw =My/I, where, M= maximum bending moment
y=half of the thickness, I=moment of inertia about the centre.
Substituting, the values for M, y and I in above equation, we get
σw=[(WT*h)t/2]/(bt3/12)
=(WT*h)6/bt2
Let, t=xpc and h=kpc where x and k are constants
WT= σw b(x2pc2)/6kpc
The value of y in terms of the number of teeth may be expressed as follows:
y =0.124-(0.684/T), for 14.50 composite and full depth involute system.
= 0.154-(0.912/T), for 200 full depth involute system.
=0.175- (0.841/T), for 200 stub system.
Q3) Explain force components on the tooth of helical gear.
A3)
The direction of tangential component for a driving gear is opposite to the direction of rotation, and that for a driven gear is same as the direction of rotation.
2. Radial Component of force (WN):
The radial component on the pinion acts toward the centre of the pinion and on the gear acts towards the centre of the gear.
3. Axial Component of force (WA): The direction of the thrust component for the driven gear will be opposite to that driving gear.
WA=WTtanα
Q4) Explain the design of helical gear.
A4) Design of helical gears:
The procedure for designing of helical gear:
Step 1: Calculate tangential tooth load: Calculate the tangential tooth load from the power transmitted and pitch line velocity as given, WT=(P/v)Cs
Step 2: Find Virtual Number of Teeth: Find the virtual number of teeth on the wheel by using equation, TE=T/(cos3α).
On the basis of virtual number of teeth, find the Lewis form factor and decide the weaker wheel.
Step 3: Apply for the Lewis equation, =(σ0*Cv)bπmy’
Step 4: Find the maximum wear strength: Ww=DpbQK/cos2α
Q5) A pair of parallel helical gears consists of a 20 teeth pinion meshing with a 100 teeth gear. The pinion rotates at 720 rpm. The normal pressure angle is 20°, while the helix angle is 25°. The face width is 40 mm and the normal module is 4 mm. The pinion as well as the gear is made of steel 4OC8 (Sut = 600 N/mm2) and heat treated to a surface hardness of 300 BHN. The service factor and the factor of safety are 1.5 and 2 respectively. Assume that the velocity factor accounts for the dynamic load and calculate the power transmitting capacity of gears.
A5) Given np= 720 rpm, zp = 20, zg = 100, mn = 4 mm, b = 40 mm, helix angle = 25°, normal pressure angle = 20°
Sut = 600 N/mm2 , BHN = 300, Cs = 1.5, fs = 2, Y= 0.3475
(I) Beam strength
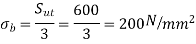
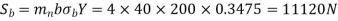
(II) Wear strength
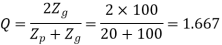
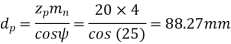
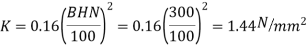
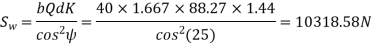
Since wear strength is lower than beam strength, pitting is the criterion of failure.
(III) Tangential force due to rated torque
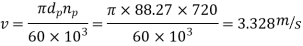
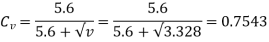
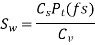
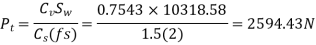
(IV) Power transmitting capacity of gears
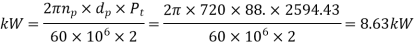
Q6) A pair of parallel helical gears consists of a 20 teeth pinion meshing with a 40 teeth gear. The helix angle is 25° and the normal pressure angle is 20°. The normal module is 3 mm.
Calculate
(i) The Transverse Module;
(Ii) The Transverse Pressure Angle;
(Iii) The Axial Pitch;
(Iv) The Pitch Circle Diameters Of The Pinion And The Gear;
(V) The centre distance;
A6)Given zp= 20, zg = 40, mn= 3 mm, helix angle= 25°, normal pressure angle = 20°.
(i) Transverse module
Using the relation
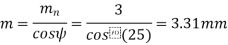
(ii) Transverse pressure angle (α)
Using the relation
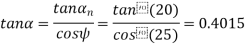
Or 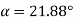
(iii) Axial pitch (pa)
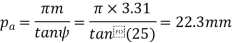
(iv) Pitch circle diameters of the pinion and the gear
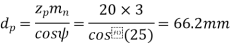
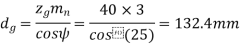
(v) Centre distance
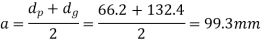
Q7) A pair of bevel gears transmitting 7.5 kW at 300 rpm is shown in figure. The pressure angle is 20°. Determine the components of the resultant gear tooth force.
A7) Given kW = 7.5, np = 300 rpm, pressure angle = 20°, Dp =150 mm, Dg = 200 mm ,b = 40 mm
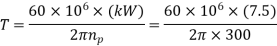
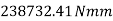
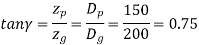
Or = 36.87˚
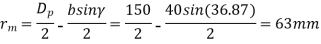
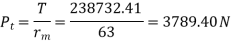
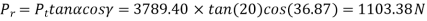
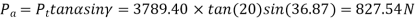
Q8) A pair of bevel gears, with 20°pressure angle, consists of a 20 teeth pinion meshing with a 30 teeth gear. The module is 4 mm, while the face width is 20 mm. The material for the pinion and gear is steel 50C4 (Sut = 750 N/mm2). The gear teeth are lapped and ground (Class-3) and the surface hardness is 400 BHN. The pinion rotates at 500 rpm and receives 2.5 kW power from the electric motor. The starting torque of the motor is 150% of the rated torque. Determine the factor of safety against bending failure and against pitting failure.
A8) Given kW = 2.5, np = 500 rpm, zp = 20, zg = 30, m = 4 mm, b = 20 mm, Sut = 750 N/mm2 , BHN = 400 starting torque = 150% (rated torque), machining grade = Class-3, θ = 20º
Y=0.33715, C=11400 N/mm
(I) Beam strength
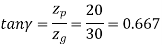
Or 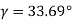
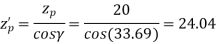
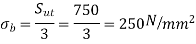
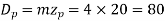
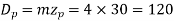
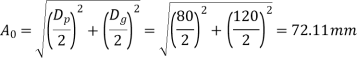
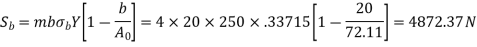
(II) Wear strength
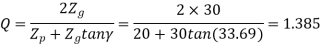
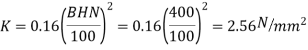
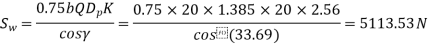
(III) Tangential force due to rated torque
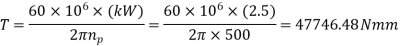

(IV) Dynamic load by Buckingham’s equation
The error for Class-3 gear teeth with 4 mm module is 0.0125 mm. (from data sheet)
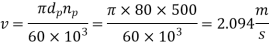
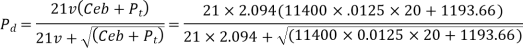
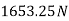
(V) Effective Load
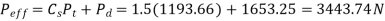
(VI) Factor of safety
Against bending failure
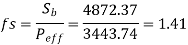
Against pitting failure
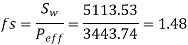
Q9) How to design a bevel gear system:
A9) For designing of bevel gear, below mentioned are following steps or procedures needs to be followed:
Q10) Discuss the deflection of helical compression and expansion spring:
A10) Total active length of the wire:
l =length of one coil ×number of active coil
= πD×n
Θ=angular deflection of the wire when acted upon by the torque T
Axial deflection of the spring,
Delta=θ×D/2=θ×R
We know that,
T/J=Ϡ/R=Gθ/l
Where, J=polar moment of inertia of the spring wire.
=π/32d4
d =diameter of wire, and G=modulus of rigidity for the material of the spring wire.
Now, θ=Tl/GJ =((W×D/2)πD.n)/(G×π/32d4)
=16WD3.n/Gd4
Substituting the value of θ in equation (1), we get
=16WD2.n/Gd4 ×D/2
=8WD3n/Gd4=8WC3n/Gd
And, the stiffness of the spring or spring rate, K=W/delta=Gd4/8D3.n= Constant