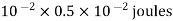Unit - 2

The Continuity Equation

Q1) A) Two insulated charged copper spheres A and B half their centres separated by a distance of 50 cm. What is the mutual force of repulsion if charge on each is coulomb? The radii of A and B is negligible compared to distance of separation.

B) What is the force of repulsion of each sphere is charged double of the above amount and the distance between them is halved?

A1)

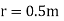
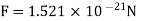
B)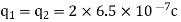

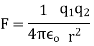
Q2) The electrostatic force on a small sphere of charge 0.4μc due to another small sphere of charge -0.8μc in air is 0.2 N.

(a)  What is the distance between the two spheres?

(b) What is the force on the second sphere due to the first?

A2) Here,

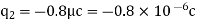
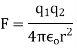
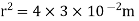
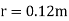
B) Force on second sphere is same 0.2N=F

Q3) A point charge of 2.0 μc is at the centre of a cubic Gaussian surface 90 cm on edge. What is the electric flux through the surface?

A3) Here q= 20 μc=2×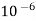

L=90 cm

=

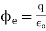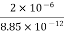
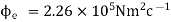
Q4) Consider uniform electric field E,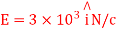

Field is along positive x direction

Surface area =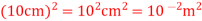

(a)What is the flux of this field through a square of 10 cm on a side whose plane is parallel to y z plane?

(b)What is the flux through the same square is the normal to its plane 30-degree angle with x axis?

A4) (a) When plane is parallel to y z plane

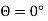
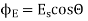
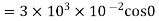
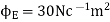
(b)When normal to the plane makes an angle of 30 degree with x-axis then =30°

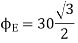
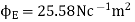
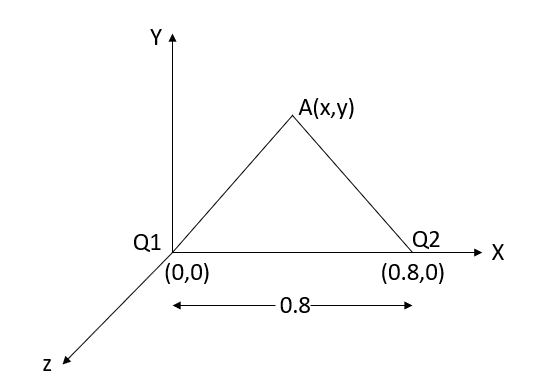
Q5) Two particles having charges and coulombs are spaced 0.8 metre apart. Determine the electric field at a point A situated at a distance of 0.5 metre from each of the particles.

A5) Let the two particles have charge coulombs then the point A will be as shown in (1). Let the origin of the three axis be located at the point charge 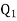then the coordinates of will be (0,0) (0.8,0) respectively.

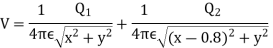
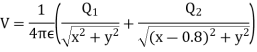
Differentiating partially with respect to x and y we get

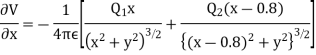
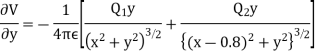
For the location of point A

x=0.4 and y= 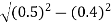=0.3

Substituting these values, we have

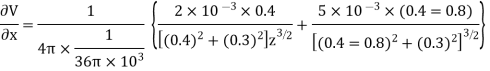
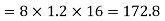
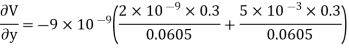
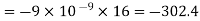
Since the field is desired in xy plane

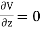  and field intensity E is given by

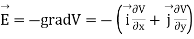
Substituting the values of and we get

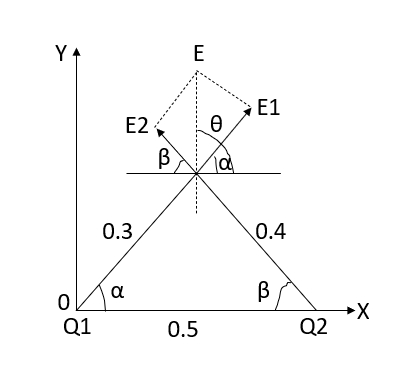
Q6) Determine the electric intensity at a point A located at a distance of 0.3 metre and 0.4 metre respectively from charges spaced 0.5 metre apart. has to charge coulombs while   coulombs.

A6) Let the electric intensity is due to be respectively and let the origin be located at 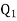as shown in figure (2). Then the angle 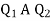will be 90°.

The magnitude of the individual intensities from equation will be

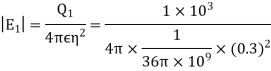
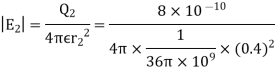
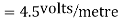
In order to add these intensities vectorially, thin components along x and y direction will be used.

(i) The x component of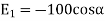

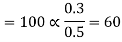
(ii)  The x component of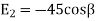

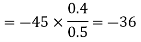
(iii) The y components of

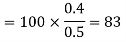
(iv) The y component of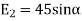

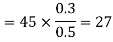
The total x components of 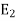let us say 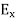will be

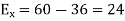
And the total y component of E let us say 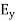will be

Resultant electric intensity has a magnitude E=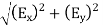

Q7) Derive an expression for the potential difference at any point between spherical shells in terms of applied potential using Laplace Equation.

A7) Since the medium between shells is uncharged, Laplace Equation can be applied. In spherical coordinates lapis equation is given by

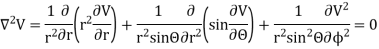
by symmetry the field and therefore the potential function depends upon the radial distance r alone

Therefore,

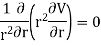
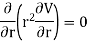
Integrating we have

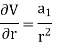
Integrating again

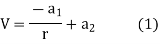
If the radius of spherical shells A and B are 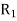and R and v is the voltage we have

When,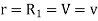

Substituting these values in equation (1)

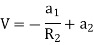
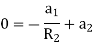
Solving these two equations for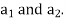

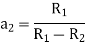
Substituting values of

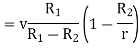
Q8) A positive charge of 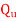coulombs per cubic metre occupies the volume of sphere. At point in the inferior at a distance r from the centre, a small proverb charge of +2 is inserted. What is the force acting on the probe charge?

A8) Force P on the probe charge at a point P lying at t a distance r from the centre, as shown in fig.

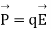
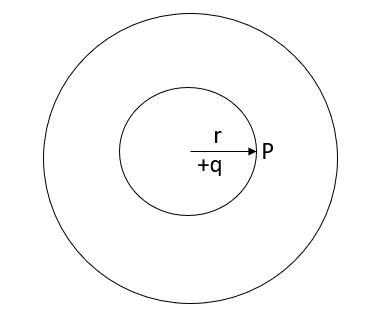
Figure A small probe charge +2 inserted at a distance r from the centre

On applying Gaussian law will yield

But the surface of sphere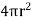

Therefore,

While the volume of a sphere is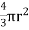

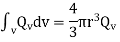
Putting these values in above equation we get

We know that,

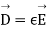
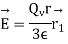
Putting this value of E in 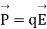we get

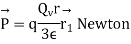
Q9) Using Laplace Equation, determine the field intensity over a gap between coaxial cylinders.

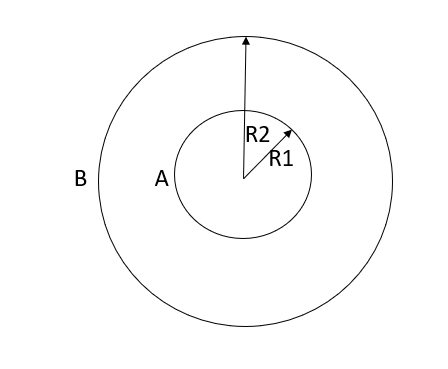
A9) Let the inner and outer radius of the coaxial cylinder be respectively as shown in figure. We have to determine the field within the coaxial cylinder subject to boundary condition.

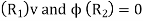
Since the nature of boundaries are of cylindrical nature, writing Laplace Equation in cylindrical form

If the cable is long, then except near ends there will be no variation with z. Father because of cylindrical symmetry potential cannot be a function of 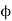so equation reduces to

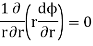
Integrating the differential equation twice we have

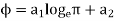
Where 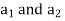are constants of integration and can be evaluated from the boundary conditions

Thus,

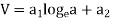
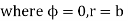
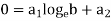
Solving above two equations we have

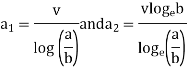
Putting these values of and above

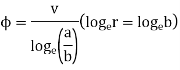
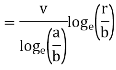
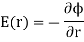
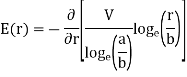
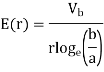
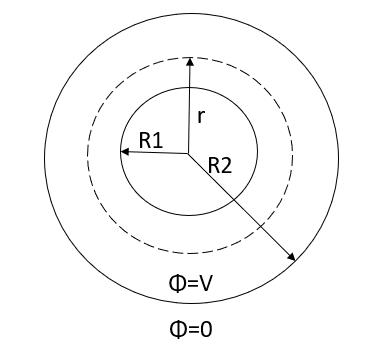
Figure: Cross section of coaxial cylinder

Q10) A point charges 1, -2, -3 and 3μc are located on the x-axis at x=1,2,3 and 4 meter respectively. What energy is stored in the field.

A10) Energy stored=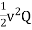

Let us find out the potential V using equation.

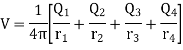
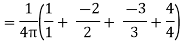
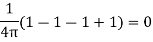
Hence energy stored in the field is zero.

Q11) A radio antenna 1 cm diameter conductor is stretched horizontally 10 metre above the ground. Determine the capacitance of the antenna per unit length. Neglect the end effects and consider the ground to be perfect.

A11) h= 10 metres

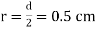
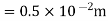
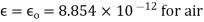
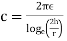
farad

p farad

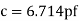
Q12) A 2 μF capacitor is charged by connecting it across a 100 V DC supply is now disconnected and the capacitor connected across another 2 μF capacitor. Assuming no leakage, determine the potential difference between the plates of each capacitor and energy connected on amount of energy stored as 2 cases.

A12) When the capacitor is connected across a 100 V D.C energy stored 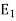is given by

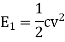
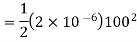
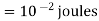
Now with 100 v supply this capacitor is charged and charge capacitor is charged and charge Q accumulated on 2 μF capacitor is

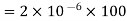
Where 100 v D.C supply is replaced by another capacitor of 2 μF. The charge 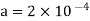coulombs get redistributed to a common potential V such that

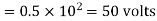
Energy stored 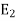is given by

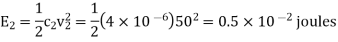
Loss of energy=