UNIT-2

First order ordinary differential equations

Question-1: Solve-

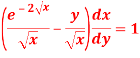
Sol. We can write the given equation as-

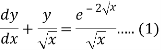
So that-

I.F. =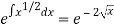

The solution of equation (1) will be-

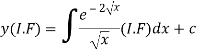
Or

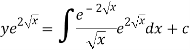
Or

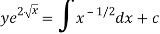
Or

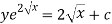
Question-2: Solve-

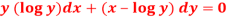
Sol.

We can write the equation as-

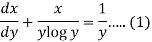
We see that it is a Leibnitz’s equation in x-

So that-

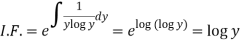
Therefore the solution of equation (1) will be-

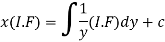
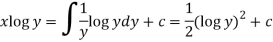
Or

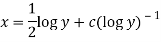
Question-3: Solve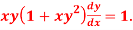

Sol.

We can write the equation as-

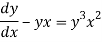
On dividing by 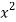, we get-

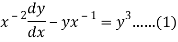
Put 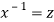so that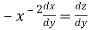

Equation (1) becomes,

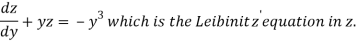
Here,

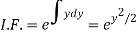
Therefore the solution is-

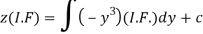
Or

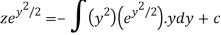
Now put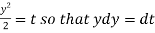

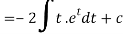
Integrate by parts-

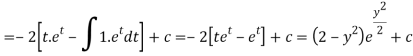
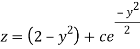
Or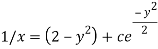
 

Question-4: Solve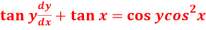

Sol. Here given,

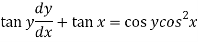
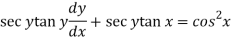
Now let z = sec y, so that dz/dx = sec y tan y dy/dx

Then the equation becomes-

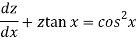
Here,

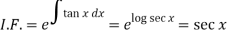
Then the solution will be-

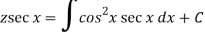
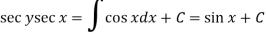
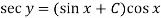
Question-5: Solve-

Sol. Here given-

We can re-write this as-

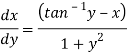
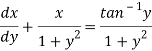
Which is a linear differential equation-

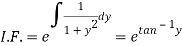
The solution will be-

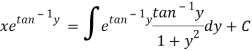
Put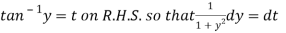

Question-6: Solve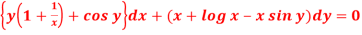

Sol.

Here M = 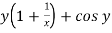and N =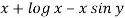

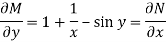
Then the equation is exact and its solution is-

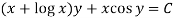
Question-7: Determine whether the differential function ydx –xdy = 0 is exact or not.

Solution.  Here the equation is the form of  M(x , y)dx + N(x , y)dy = 0

  But, we will check for exactness,

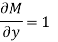
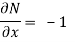
These are not equal results, so we can say that the given diff. Eq. Is not exact.

Question-8: Solve-

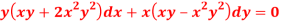
Sol.

Here we have-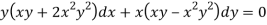
 

Now divide by xy, we get-

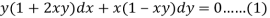
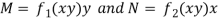
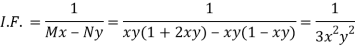
Multiply (1) by 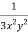, we get-

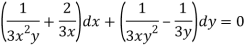
Which is an exact differential equation-

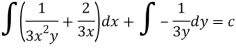
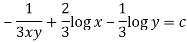
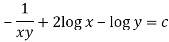
Question-9: Solve-

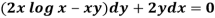
Sol.

Here given,

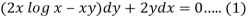
M = 2y and N = 2x log x - xy

Then-

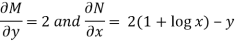
Here,

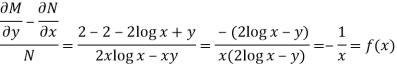
Then,

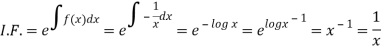
Now multiplying equation (1) by 1/x, we get-

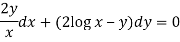
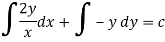
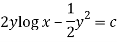
Question-10: Solve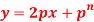

Sol.

Here we have-

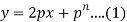
Now differentiate it with respect to x, we get-

Or

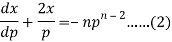
This is the Leibnitz’s linear equation in x and p, here

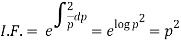
Then the solution of (2) is-

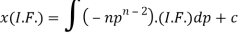
Or

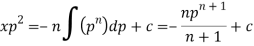
Or

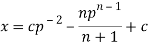
Put this value of x in (1), we get

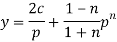
Question-11: Solve-

Sol.

Put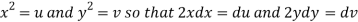

So that-

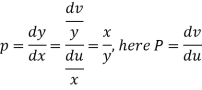
Then the given equation becomes-

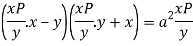
Or

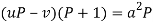
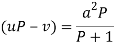
Or

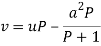
Which is the Clairaut’s form.

Its solution is-

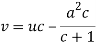
i.e.