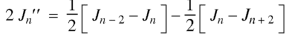UNIT-3

UNIT-3

UNIT-3

Ordinary differential equations higher order

Question-1: Solve (4D² +4D -3)y =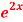 

Solution: Auxiliary equation is 4m² +4m – 3 = 0

    We get,             (2m+3)(2m – 1) = 0

                               m =   ,

Complementary function:  CF is  A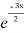+ B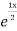

Now we will find particular integral,

                         P.I. = 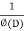f(x)

=   .

=    .

=    .

=   . =   .

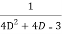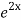
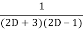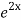
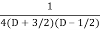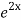
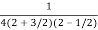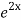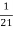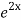
General solution is y =  CF + PI

                                                    = A+ B  .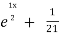

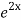
Question-2: Solve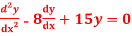

Ans. Given,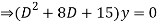

Here Auxiliary equation is

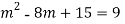
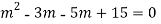
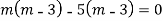
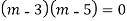
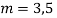
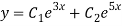
Question-3: Solve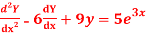

Ans. Given,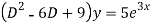

Auxiliary equation is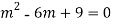

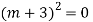
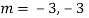
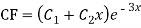
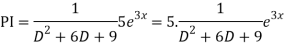
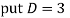
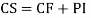
Question-4:

Given,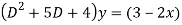

For CF,

Auxiliary equation are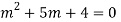

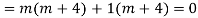
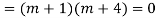
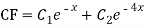
For PI

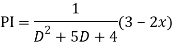
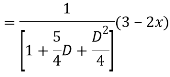
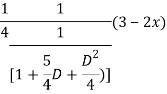
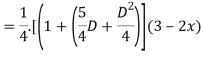
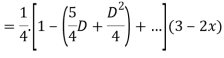
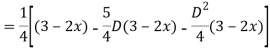
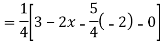
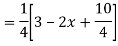
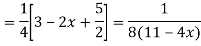
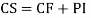
Question-5: Solve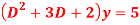

Ans. The AE is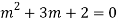

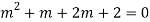
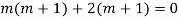
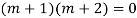
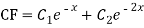
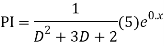
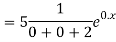
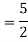
Complete solution = CF + PI

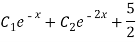
Question-6: Solve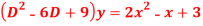

Ans. The AE is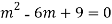

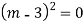
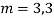
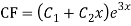
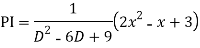
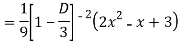
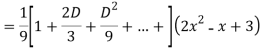
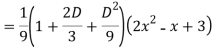
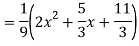
Complete solutio0n is y= CF + PI

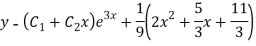
Question-7: Solve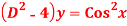

Ans. The AE is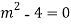

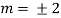
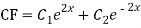
We know,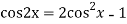

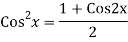
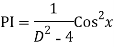
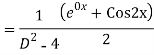
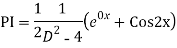
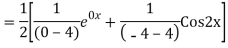
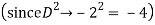
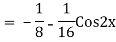
Complete solution is y= CF + PI

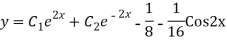
Question-8: Solve the following simultaneous differential equations-

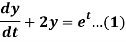
....(2)

Solution:

Consider the given equations

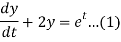
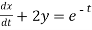....(2)

Consider eq(1),(2)

Dx+2y = et....(1)

Dx +2x =e-t....(2)

Eliminating ‘x’ from both the equations we get,

12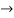 2Dx + 2y = 2et

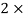D 2Dx +D2y = e-t

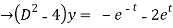
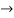y = Ae2t + Be-2t +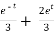

Question-9: Find the P.I. Of (D + 2)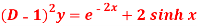

Sol.

P.I. =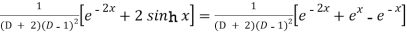

Now we will evaluate each term separately-

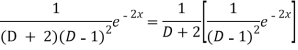
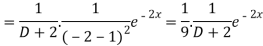
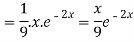
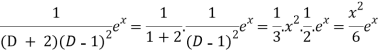
And

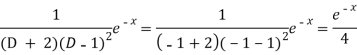
Therefore-

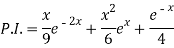
Question-10: Find the P.I. Of (D + 1) (D + D’ – 1)z = sin (x + 2y)

Sol.

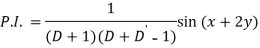
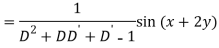
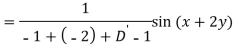
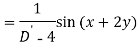
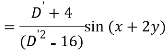
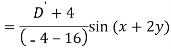
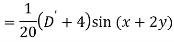
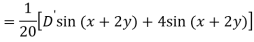
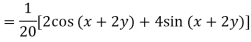
Question-11: Solve the following DE by using variation of parameters-

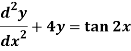
Sol. We can write the given equation in symbolic form as-

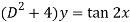
To find CF-

It’s A.E. Is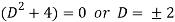

So that CF is-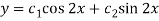     

To find PI-

Here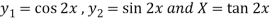

Now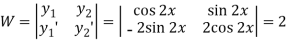

Thus PI =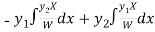 

            = 

            = 

             = 

             = 

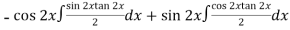
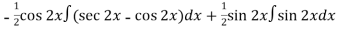
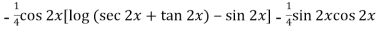
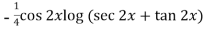
So that the complete solution is-

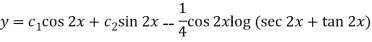
Question-12: Solve

Ans. Let,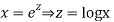

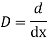
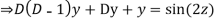
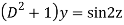
AE is

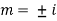
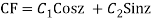
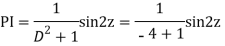
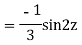
y= CF + PI

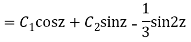
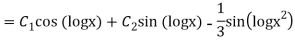
Question-13: Solve

Sol.

Here we have-

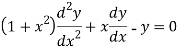
Let the solution of the given differential equation be-

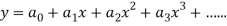
Since x = 0 is the ordinary point of the given equation-

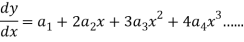
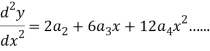
Put these values in the given differential equation-

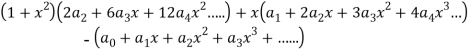
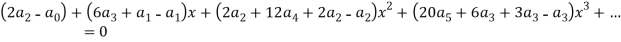
Equating the coefficients of various powers of x to zero, we get-

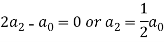
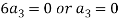
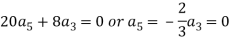
Therefore the solution is-

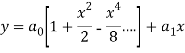
Solve

Sol.

Here we have-

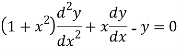
Let the solution of the given differential equation be-

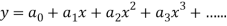
Since x = 0 is the ordinary point of the given equation-

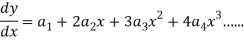
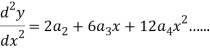
Put these values in the given differential equation-

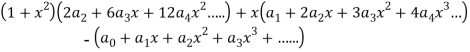
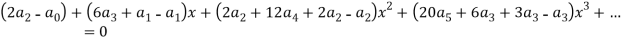
Equating the coefficients of various powers of x to zero, we get-

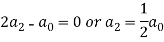
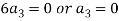
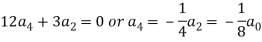
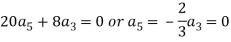
Therefore the solution is-

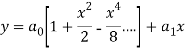
Question-14: Express in terms of Legendre polynomials.

Sol.

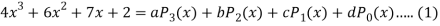
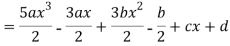
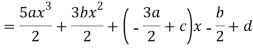
By equating the coefficients of like powers of x, we get-

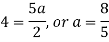
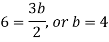
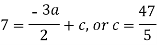
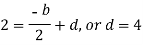
Put these values in equation (1), we get-

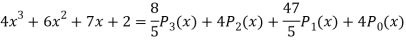
Question-15: Show that-

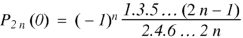
Sol.

We know that

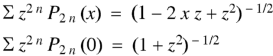
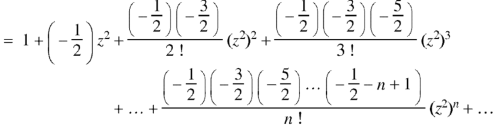
Equating the coefficients of both sides, we have-

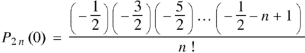
Question-16: Prove that-

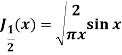
Sol.

As we know that-

Now put n = 1/2 in equation (1), then we get-

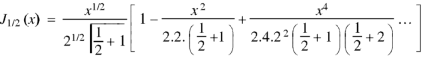
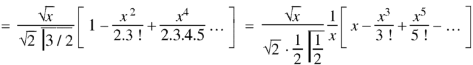
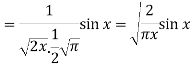
Hence proved.

Question-17: Show that-

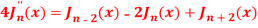
By using recurrence relation.

Sol.

We know that-

The recurrence formula-

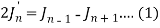
On differentiating, we get-

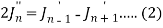
Now replace n by n -1 and n by n+1 in (1), we have-

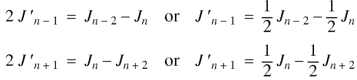
Put the values of 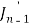and 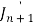from the above equations in (2), we get-