Question Bank
UNIT 4
Introduction to Beams
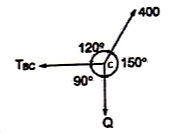
Question 1)Find the axial force in the bar AB & AC as shown in figure.
Answer:
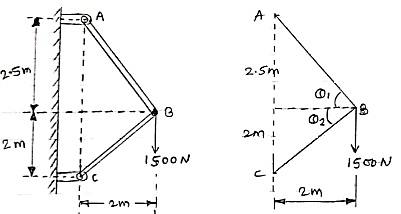
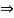Consider the free body diagram of all forces at B.
Q1 = tan -1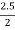 
= 51.34º
Q2 = tan-1 
= 45º
Let the forces developed in the member AB & AC are FAB &FBC respectively. The force diagram will be as follows at B.
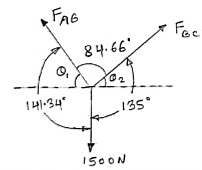Applying Lami’s Theorem:
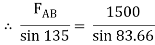
FAB = 1067.19 N
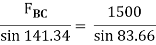
FBC = 942.81 N
Question 2. Find the tensions in the string AB, BC, CD, DE of the given system as shown in figure. String BC is horizontal & pulley D is frictionless.
Answer:
The string passes over the pulley & is attached to weight w = 400 N.
Thus TCD = TDE = 400 N
Consider joint C & apply Lami’s theorem
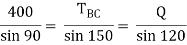
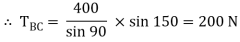
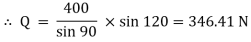
Now consider FBD at joint B
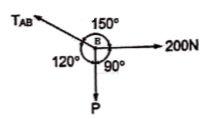
By using Lami’s theorem
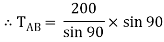
TAB = 230.94 N
Question 3) Two identical spheres having weight 1000 N are kept in a container as shown in figure. Find the reaction at all contact surfaces.
Answer:
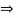Consider the free body diagram of sphere B as shown below:
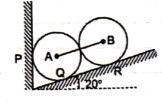
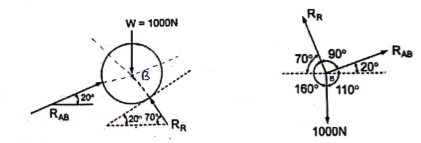
By lami’s theorem
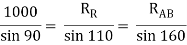
RRR = 939.69 N
= RAB
Now consider FBD of sphere A
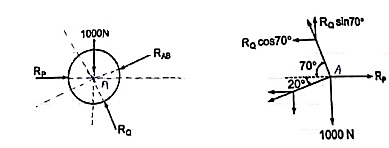
∑Fx= 0
-RB cos 20 – RQ cos 70 + Rp= 0
-342.02 cos 20 – RQ sin 70 + Rp= 0
Rp = 321.39 + 0.34 RQ------------------(1)
∑ fy= 0
RQ sin 70 – 342.02 sin 20 – 1000 = 0
RQ = 1188.66 N - put in equation (1)
Rp = 725.5 N
Question 4) Two members AB & BC are supporting a load of 200 kN as shown in figure. Find the forces developed in the members AB & BC
Answer:
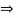Consider the free body diagram of joint B as shown in figure
Assume that member BC is in compression & AB is in tension. By using Lami’s theorem at B,
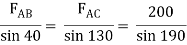
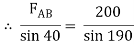
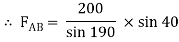
FAB = -740.33 kN
-ve sign indicates that member AB is not in Tension but is in compression.
Now,
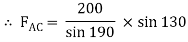
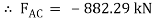
-ve sign indicates that member BC is not in compression but in tension.
Question 5) A Sphere of weight 200 N rests on a smooth inclined plane. A sphere is kept in equilibrium by means of cable as shown. Find tension in cable and reaction.
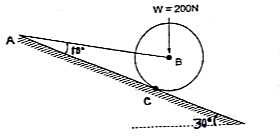
Answer:
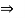Consider the FBD of sphere B as shown in figure above.
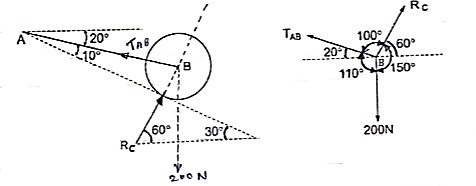
Applying lami’s theorem, we have
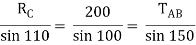
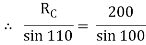
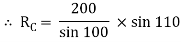
RC = 190.84 N
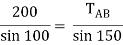
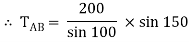
TAB = 101.54 N
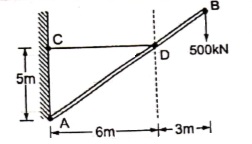
Question 6) The bar AB supports a load of 500 kN as shown in figure. The bae is kept in equilibrium by means of horizontal rope. Find tension in rope and reaction at A,
Answer:
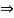Consider the FBD as shown
Applying conditions of equilibrium,
∑fx= 0
RHA – TCD = 0
RHA = TCD ------- (1)
∑fy= 0
RVA – 500 = 0
RVA = 500 kN
Taking moment about point A,
∑MA = 0
-(TCD × 5 ) + (500 × 9) = 0
-TCD × 5 = -4500
TCD =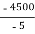 
TCD = 900 kN
Putting the value in equation (1)
RHA = TCD
RHA = 900 kN
Question7) A bar of weight 200 N is hinged at A and pulled by the string attached at B as shown in figure. The length of bar is 5m. Find the support reactions at A & corresponding tension in string.
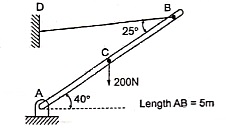
Answer:
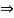Consider triangle AEC
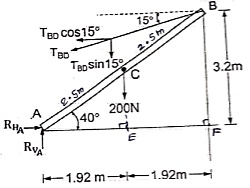
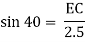
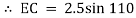
EC = 1.61 m
Consider triangle AEC
AE = 1.92 m
Applying conditions of equilibrium
∑fX = 0
-TBD cos15 + RHA = 0 -------- (1)
∑fy= 0
RVA – 200 – TBD sin15 = 0
RVA – 200 – 0.26TBD = 0 ---------(2)
Taking moment at A
∑MA = 0
-(TBD cos15 × 3.2) + (TBD sin15 × 3.84) + (200 × 1.92) = 0
-3.09 TBD + 0.99 TBD + 384 = 0
-2.1 TBD = -384
TBD = 182.86 N ---put in (1) & (2)
RHA = TBD cos15 = 182.86 cos15
RHA = 196.62 N
RVA = 200 + 0.26TBD = 200 + (0.26 × 182.86)
RVA = 247.54 N
Question 8) Explain roller supports.
Answer:
- Roller Support: -
Roller support is show in two ways as shown:
A free body diagram of roller support is shown in figure given below
- Roller support offers only one reaction; which is always perpendicular to the base or plane of roller.
- All the steel trusses of the bridges have one of their ends supported on roller.
- Main advantages of the support are that beam can easily move towards left or right due to expansion & contraction.
Question 9) Explain hinge support.
Answer:
Hinge support
- In this case, end of the beam is hinged to the support as shown in figure above.
- This type of support offers two reactions, one is parallel to base and perpendicular to the base
- All the steel trusses of the bridges have one of their end roller supported & other end is hinged
- Main advantage of this support is that beam remains stable.
If both ends of the beam are roller supported, then beam cannot be stable. Thus, one support of beam is made roller supported and other is hinged
Question 10) Explain the types of beams?
Answer:
Types of Beam: -
- Simply supported beam –
A beam which is just resting on the supports at the end without any connection is known as simply supported beam. It is generally used for vertical landing system.
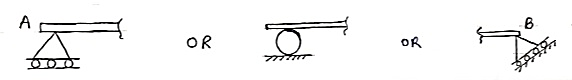
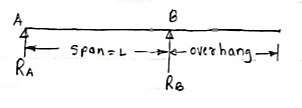
2. Overhanging Beam –
A beam which is supported at the intermediate point other than ends is called as overhanging beam. Here portion of beam is extended beyond the support
a)
(singly overhanging beam)
b)
(doubly overhanging beam)
3. Cantilever Beam –
A beam which is fixed at one end is called as cantilever beam
Here, there are three reactions components:
- Vertical reaction at A (RVA)
- Horizontal reaction at A (RHA)
- Fixing moment at A (MA)
We can assume any direction for above components.
4. Continuous Beam:
A beam having more than two support is called as continuous beam.
5. Compound Beam:
When two or more beams are joined together by using internal hinge; or when one beam rests over another beam by using internal roller, then such beam is called as compound beam.
