Question Bank
UNIT 3
Friction
Question 1) Determine the horizontal force P needed to just start moving the 300 N create up the plane as shown in figure. Take 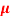 = 0%
= 0%
 Answer:
Answer:
For the equilibrium
 = 0
= 0
 P – RNcos 70 – FScos 20 = 0
P – RNcos 70 – FScos 20 = 0
 P – RNcos 70 –
P – RNcos 70 – 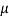 s FScos 20 = 0
s FScos 20 = 0
 P – 0.342 RN 0.094 RN = 0
P – 0.342 RN 0.094 RN = 0
 P = 0.436 RN
P = 0.436 RN
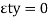
 – 300 + RN Sin 70 – FS Sin 20 = 0
– 300 + RN Sin 70 – FS Sin 20 = 0
 – 300 + RN Sin 70 –
– 300 + RN Sin 70 –  s FS Sin 20 = 0
s FS Sin 20 = 0
 – 300 + RN Sin 70 – 0.1 RN Sin 20 = 0
– 300 + RN Sin 70 – 0.1 RN Sin 20 = 0
 – 300 + 0.94 RN – 0.342 RN = 0
– 300 + 0.94 RN – 0.342 RN = 0
 0.9058 RN = 300
0.9058 RN = 300
 RN = 331.2 N
RN = 331.2 N
 n 1
n 1
P = 0.436  331.2
331.2
P = 144.4 N ------------------- force required
Question 2): A body of weight 50 N is along rough horizontal plane A pull of 18 N acting at an angle of 140 with the horizontal find coefficient of static friction.

Answer:
Considering the equilibrium of block
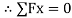
 18 Cos 14 – Fs = 0
18 Cos 14 – Fs = 0
 Fs = 17.46 N
Fs = 17.46 N

RN + 18 Sin 14 – 50 = 0
RN = 45.64 N
As Fs = 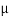 S RN
S RN
17.46 =  S
S  45.64
45.64
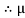 S = 0.386
S = 0.386
Question 3): A block of mass m rests on frictional plane which makes an angle  with horizontal as shown if the coefficient Of friction between the block & frictional plane is 0.2, determine angle
with horizontal as shown if the coefficient Of friction between the block & frictional plane is 0.2, determine angle  for limiting friction.
for limiting friction.
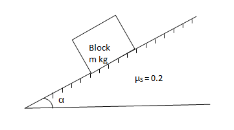
Answer:
For limiting friction
Tan 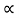 tan
tan  S =
S = 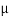 S
S
 tan-1
tan-1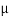 S
S
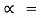 tan-1
tan-1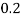
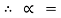 11.310
11.310
Question 4) Determine whether the block shown is in equilibrium and find the magnitude and direction of friction force when  = 350 and P = 100 N.
= 350 and P = 100 N.
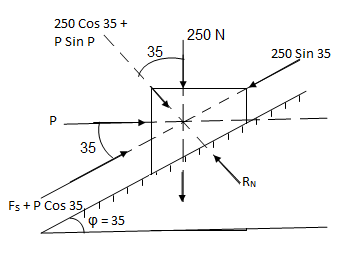
Answer:
Let us select inclined plane as x-x axis & perpendicular to plane as y-y axis
Let Fs = static frictional force which is required to maintain equilibrium.
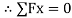 resolving force along x-axis
resolving force along x-axis
 Fs + 100 Cos 35 – 250 Sin 35 = 0
Fs + 100 Cos 35 – 250 Sin 35 = 0
 Fs – 61.48 = 0
Fs – 61.48 = 0
 Fs = 61.48 N ----- frictional force
Fs = 61.48 N ----- frictional force
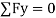 --resolving force along y-axis
--resolving force along y-axis
 -(250 Cos 35 + P Sin 35) + RN = 0
-(250 Cos 35 + P Sin 35) + RN = 0
 -250 Cos 35 – 100 Sin 35 + RN = 0
-250 Cos 35 – 100 Sin 35 + RN = 0
-262.15 + RN = 0
 RN = 262.15 N -------- normal reaction
RN = 262.15 N -------- normal reaction
Maximum frictional force can be developed is given by
Fs max = 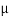 S
S RN = 0.3
RN = 0.3  262.15
262.15
Fs max = 78.65 N
As frictional force required maintaining equilibrium (61.48 N) is less than max. Frictional force,
 Equilibrium will be maintained
Equilibrium will be maintained
Question5 

At what position from the base, will be induce the slipping of ladder.
Answer:
Take  S = 0.34 at all contact surface.
S = 0.34 at all contact surface.
Weight of man = 60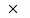 9.81 =588.6 N
9.81 =588.6 N
Weight of ladder 
AB = 8 m
Impending motion
 Ac = 8 Cos 54
Ac = 8 Cos 54
 BC = 8 Sin 54
BC = 8 Sin 54
 ɑ = 2.35 m
ɑ = 2.35 m
 b = x Cos 54
b = x Cos 54
 FSA =
FSA = 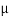 S
S  RNA
RNA
 FSB =
FSB = 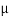 S
S  RNB
RNB
Considering the equilibrium of ladder
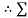 FX = 0
FX = 0
       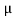 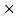     |
 

From eqn …..1
RNB = 0.34 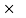

|
Taking moment about point A,
 MA = 0
MA = 0
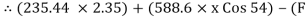 SB
SB Cos 54) – (RNB
Cos 54) – (RNB 8 Sin 54) = 0
8 Sin 54) = 0
 (
(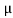 S
S  RNB
RNB  4.7) – (6.47 RNB) = 0
4.7) – (6.47 RNB) = 0
 1.598 RNB – 6.47 RNB = 0
1.598 RNB – 6.47 RNB = 0
 345.97x – 8.068 RNB = 553.284
345.97x – 8.068 RNB = 553.284
 345.97x – 8.068
345.97x – 8.068  251.14 = 553.284
251.14 = 553.284
 345.97x = 1472.913
345.97x = 1472.913
X = 4.257 m
Ans :
At X = 4.257 m from point A, along the ladder, man can climb without slipping.Beyond this distance, ladder will be slipping.
Question 6: A 137 kg block is supported by a rope whichIs wrapped 1.5 times around a horizontal Rod knowing that coeff. Of static frictionBetween rope and rod is 0.15, determineThe range of values of P for which Equilibrium is maintained.
Answer:
Weight of block = 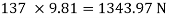
Angle of contact = LAP Angle
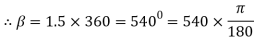
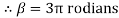
A) Case I : when P > 1343.97 N
Force P tends to move the rope down ward and block moves upward. Thus the part rope on which force P is acting is height side and rope to which block is attached is slack side.
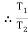 =
=  =
= 
 P = 5525.34 N
P = 5525.34 N
B) Case II : when P < 1343.97 N
 force P is less than 1343.97 N, so block will move down ward & the side rope on which P acts will move upward.
force P is less than 1343.97 N, so block will move down ward & the side rope on which P acts will move upward.
 Rope side to which block is attached is tight side and another side is slack side.
Rope side to which block is attached is tight side and another side is slack side.
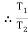 =
=  =
= 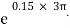
 P = 326.92 N
P = 326.92 N
 Range of P for equilibrium is 326.92 N
Range of P for equilibrium is 326.92 N 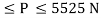
Question 7: Two cylinders are connected by a rope that passesOver two fixed rods as shown. Knowing that theCoefficient of static friction between the rope andThe rods is 0.40, determine the range of the mass m Of cylinder D for which equilibrium is maintained.
Weight of cylinder = 50  9.81 = 490.5 N
9.81 = 490.5 N

Answer:
Lap angle = 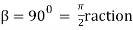
 S = 0.40
S = 0.40
A) Case I :
Weight of cylinder A > weight of cylinder B.
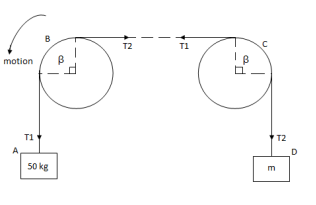
In this case cylinder A will tends to move downward while B will tends to move upward
Consider part AB of the Rope as AB rope is moving down,
Tension in height side T1 = 
Tension in slack side = T2 = unknown.
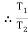 =
= 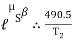 =
= 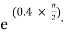 = 1.874
= 1.874
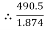 = T2
= T2
 T2 = 261.74 N
T2 = 261.74 N
Consider part CD, of the rope. As rope CD is moving upward,
Tension in tight side T1 = T2 of rope AB
Tension in tight side, T1 = 261.74 N
Tension in slack side = T2 = (m  9.81)
9.81)
    | 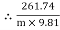  |
B) Case II :
Weight of cylinder A < weight of cylinder B
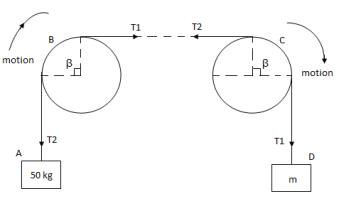
In this case cylinder a will tends to move upward while cylinder B will tends to move downward
Consider part AB of the rope moving upwards
Tension in slack side = side A = 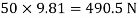
 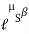  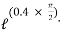 |   |
Consider part CD of rope, Rope CD is moving downwards
Tension in tight side = T1 = (m 9.81) N
9.81) N
Tension in slack side = T2 = T1 of rope AB = 919.197 N
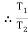 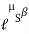 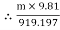  | 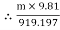  |
Range of mass m for equilibrium to be maintained is
14.23 kg 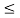 m
m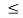 175.59 kg
175.59 kg
Question 8) A Force P = mg/6 is required to lower the cylinder with card making 1.25 turns around the fixed shaft. Determine the coeff. Of friction “ S” between the card and the shaft
S” between the card and the shaft
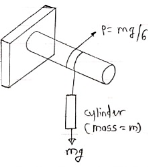 Refer the figure
Refer the figure
Answer:
Lap angle

= 2.5 radians
radians
For flat belt,
 =
= 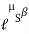
 =
= 
 6
6 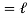
 1.79 =
1.79 = 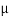 S
S 2.5
2.5
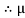 S = 0.23
S = 0.23
Question 9): Determine the range of P for the equilibrium of block of weight W as shown in fig. The coeff. Of friction between rope and pulley is 0.2
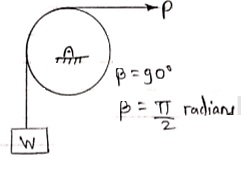
Answer:
Case I : when P is max.
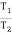 =
= 
 =
= 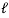
 Pmax = 1.369 w
Pmax = 1.369 w
Case II : when P is min.
 =
= 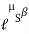
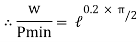
 Pmin = 0.73 w
Pmin = 0.73 w
For equilibrium P must be between 0.73  P
P 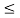 1.369 w
1.369 w
Question 10) Explain type of friction.
Answer:
Types of friction
Friction is the force that opposes motion between any surfaces that are in contact. There are four types of friction: static, sliding, rolling, and fluid friction. Static, sliding, and rolling friction occur between solid surfaces. Fluid friction occurs in liquids and gases. All four types of friction are described below.
Static Friction
Static friction acts on objects when they are resting on a surface. For example, if you are hiking in the woods, there is static friction between your shoes and the trail each time you put down your foot (see Figure below). Without this static friction, your feet would slip out from under you, making it difficult to walk. In fact, that's exactly what happens if you try to walk on ice. That's because ice is very slippery and offers very little friction.
Sliding Friction
Sliding friction is friction that acts on objects when they are sliding over a surface. Sliding friction is weaker than static friction. That's why it's easier to slide a piece of furniture over the floor after you start it moving than it is to get it moving in the first place. Sliding friction can be useful. For example, you use sliding friction when you write with a pencil. The pencil “lead” slides easily over the paper, but there's just enough friction between the pencil and paper to leave a mark.
Rolling Friction
Rolling friction is friction that acts on objects when they are rolling over a surface. Rolling friction is much weaker than sliding friction or static friction. This explains why most forms of ground transportation use wheels, including bicycles, cars, 4-wheelers, roller skates, scooters, and skateboards. Ball bearings are another use of rolling friction. You can see what they look like in the Figure below. They let parts of a wheel or other machine roll rather than slide over on another.

The ball bearings in this wheel reduce friction between the inner and outer cylinders when they turn.[Figure3]
Fluid Friction
Fluid friction is friction that acts on objects that are moving through a fluid. A fluid is a substance that can flow and take the shape of its container. Fluids include liquids and gases. If you've ever tried to push your open hand through the water in a tub or pool, then you've experienced fluid friction. You can feel the resistance of the water against your hand. Look at the skydiver in the Figure below. He's falling toward Earth with a parachute. Resistance of the air against the parachute slows his descent. The faster or larger a moving object is, the greater is the fluid friction resisting its motion. That's why there is greater air resistance against the parachute than the skydiver's body.