UNIT – 1
PHYSICAL OPTICS
Q1) Derive expression for resolving power of grating?
A1) It is defined as the capacity of a grating to form separate diffraction maxima of two wavelengths which are very close to each other
It is measured by λ/dλ where dλ is the smallest difference in two wavelengths which are just resolvable by grating and λ is the wavelength of either of them or mean wavelength.
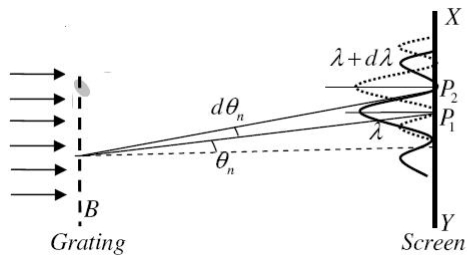
Figure: Resolving Power of Diffraction Grating
Let AB represent the surface of a plane transmission grating having grating element (e+d) and N total number of slits.
Let a beam of light having two wavelengths by λ and dλ is normally incident on the grating. Let P1 is nth primary maximum of a spectral line of wavelength λ at an angle of diffraction 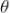 and P2 is the nth primary maximum of wavelength λ+dλ at diffracting angle θ+dθ.
and P2 is the nth primary maximum of wavelength λ+dλ at diffracting angle θ+dθ.
According to Rayleigh criterion, the two wavelengths will be resolved if the principal maximum λ+dλ of nth order in a direction θ+dθ falls over the first minimum of nth order in the same direction θ+dθ.
Let us consider the first minimum of l of nth order in the direction θ+dθ as below.
The principal maximum of 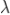 in the θ direction is given by
in the θ direction is given by
(e+d) sinθ =nλ ……………… (1)
The equation of minima is
N(e+d) sinθ = mλ ……………… (2)
Where m takes all integers except 0, N, 2N, …, nN, because for these values of m, the condition for maxima is satisfied.
Thus, first minimum adjacent to nth principal maximum in the direction θ+dθ can be obtained by substituting the value of ‘m’ as (nN+1).
Therefore, the first minimum in the direction of θ+dθ is given by
N(e+d) sin(θ+dθ) = (nN+1) λ
(e+d) sin(θ+dθ) = (n+ )λ ……………… (3)
)λ ……………… (3)
The principal maximum of λ+dλ in direction θ+dθ is given by
(e+d) sin(θ+dθ) = n(λ+dλ) ……………… (4)
Dividing eqn (3) by equation (4), we get
(n+ )λ = n(λ+dλ)
)λ = n(λ+dλ)
nλ + = nλ + ndλ
= nλ + ndλ
 = ndλ
= ndλ
 = nN
= nN
Resolving Power =  = nN ……………… (5)
= nN ……………… (5)
Thus, the resolving power is directly proportional to
(i) The order of the spectrum ‘n’
(ii) The total number of lines on the grating ‘N’.
Q2) Write a note on diffraction grating?
A2) A set of large number of parallel slits of same width and separated by opaque spaces is known as diffraction grating.
Diffraction gratings are much more effective than prisms for dispersing light of different wavelengths so they are used almost exclusively in instruments designed to detect and identify characteristic spectral lines. There is nothing mysterious about these devices.
Fraunhofer used the first grating consisting of a large number of parallel wires placed side by side very closely at regular separation. Now the gratings are constructed by ruling the equidistance parallel lines on a transparent material such as glass with fine diamond point. The ruled lines are opaque to light while the space between the two lines is transparent to light and act as a slit.

Figure: Plane Transmission Grating
Let ‘e’ be the width of line and ‘d’ be the width of the slit.
Then (e + d) is known as grating element.
If N is the number of lines per inch on the grating, then
(𝑒+𝑑) =1𝑖𝑐ℎ=2.54𝑐𝑚
(𝑒+𝑑) =2.54𝑐𝑚/𝑁
Commercial gratings are produced by taking the cost of actual grating on a transparent film like that of cellulose acetate. Solution of cellulose acetate is poured on a ruled surface and allowed to dry to form a thin film, detachable from the surface. This film of grating is kept between the two glass plates.
Q3) A parallel beam of monochromatic light of wavelength 500nm is inclined normally on a plane diffraction grating 4000 per centimetre. Calculate the angle of diffraction for first-order principal maximum.
A3) Given: number of lines per cm =4000
Grating element e+b = 1/4000 cm = 2.5 x 10-6m
λ=500 nm
Order n =1
(e+b) sinθ =nλ
sinθ = nλ / (e+b)
sinθ = 1 x 500 x 10-9 / 2.5 x 10-6
sinθ =0.2
θ =sin-1 0.2
θ = 11.5o
Q4) A grating containing 4000 slits per centimetre is illuminated with a monochromatic light and produces the second-order bright line at a 30° angle. What is the wavelength of the light used? (1 Å = 10-10 m)
A4) The distance between slits (d) = 1 / (4000 slits / cm) = 0.00025 cm = 2.5 x 10-4 cm = 2.5 x 10-6 meters
Order (n) = 2
Sin 30o = 0.5
1 Å = 10-10 m
Wavelength of the light (λ) =?

Q5) A monochromatic light with a wavelength of 2.5x10-7 m strikes a grating containing 10,000 slits/cm. Determine the angular positions of the second-order bright line.
A5) Given
The distance between slits (d) = 1 / (10,000 slits / cm) = 0.0001 cm = 1 x 10-4 cm = 1 x 10-6 m
Order (n) = 2
Wavelength (λ) = 2.5 x 10-7 m
Angle (θ)
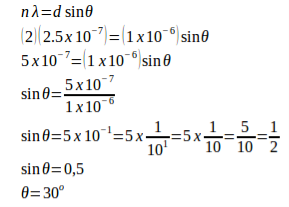
Q6) A monochromatic light with a wavelength of 500 nm (1 nm = 10-9 m) strikes a grating and produces the second-order bright line at a 30° angle. Determine the number of slits per centimetre.
A6) Wavelength (λ) = 500.10-9 m = 5.10-7 m
θ = 30o
n = 2
Distance between slits:
d sin θ = n λ
d (sin 30o) = (2) (5.10-7)
d (0.5) = 10.10-7
d = (10.10-7) / 0.5
d = 20.10-7
d = 2.10-6 m
Number of slits per centimetre:
x = 1 / d
x = 1 / 2.10-6 m
x = 0.5.106 / 1 m
x = 0.5.106 / 102 cm
x = 0.5.104 cm
x = 5.103 /cm
x = 5000 /cm
Q7) A monochromatic light with a wavelength of 500 nm (1 nano = 10-9) strikes a grating and produces the fourth-order bright line at a 30° angle. Determine the number of slits per centimetre.
A7) Wavelength (λ) = 500.10-9 m = 5.10-7 m
θ = 30o
n = 4
Distance between slits:
d sin θ = n λ
d (sin 30o) = (4) (5.10-7)
d (0.5) = 20.10-7
d = (20.10-7) / 0.5
d = 40.10-7
d = 4.10-6 m
Number of slits per centimetre:
x = 1 / 4.10-6 m
x = 0.25.106 / m
x = 0.25.106 / 102 cm
x = 0.25.104 / cm
x = 25.102 per cm
x = 2500 per cm
Q8) A grating has 1100 lines ruled on it. What is the difference between the two wavelengths that just appear resolved in the first-order spectrum in the region of wavelength λ = 660 nm?
A8) N = 1100 nm
λ = 660 x 10-9 m
n = 1
R = λ /dλ =Nn
dλ = λ /Nn
dλ = 660 x 10-9 / 1100 x 10-9 x 1
dλ = 0.6 x 10-9 m
Q9) What is the difference between Fresnel’s diffraction and Fraunhofer's diffraction?
A9)
Fresnel Diffraction | Fraunhofer diffraction |
1 If the source of light and screen is at a finite distance from the obstacle, then the diffraction is called Fresnel diffraction. | 1 If the source of light and screen is at an infinite distance from the obstacle then the diffraction is called Fraunhofer diffraction. |
2 The corresponding rays are not parallel. | 2 The corresponding rays are not parallel. |
3 The wavefronts falling on the obstacle are not plane. | 3 The wavefronts falling on the obstacle are planes. |
4 No convex lens is needed to converge spherical wavefronts. | 4 Plane diffracting wavefronts are converged by means of a convex lens to produce a diffraction pattern. |
5 Fresnel diffraction patterns on flat surfaces. | 5 Fraunhofer diffraction patterns on spherical surfaces. |
6 Diffraction patterns Change as we propagate them further ‘downstream’ of the source of scattering. | 6 Diffraction patterns Shape and intensity of a Fraunhofer diffraction pattern stay constant. |
7 To obtain Fresnel diffraction, zone plates are used. | 7 To obtain Fraunhofer diffraction, the single-double plane diffraction grafting is used. |
8 Diffraction pattern moves along the corresponding shift in the object. | 8 Diffraction pattern remains in a fixed position. |
Q10) What is dispersive power for grating?
A10) The dispersive power of a grating is defined as the ratio of the difference in the angle of diffraction of any two neighbouring spectral lines to the difference in the wavelength between the two spectral lines.
Or
It can also be defined as the diffraction in the angle of diffraction per unit change in wavelength.
The diffraction of the nth order principal maximum for a wavelength λ is given by the equation,
(a + b) sin θ = nλ (1)
Differentiating this equation with respect to θ and λ
a + b is constant and n is constant in a given order.
(a + b) cos θ dθ = n dλ
 (2)
(2)
In equation (2) dθ/dλ is the dispersive power,
n is the order of the spectrum,
N’ is the number of lines per cm of the grating surface and
θ is the angle of diffraction for the nth order principal maximum of wavelength λ.
From equation (2), it is clear, that the dispersive power of the grating is directly proportional to the number of lines per cm and inversely proportional to cos θ.
Thus, the angular spacing of any two spectral lines is double in the second-order spectrum in comparison to the first order. Secondly, the angular dispersion of the lines is more with a grating having a larger number of lines per cm. thirdly, the angular dispersion is minimum when θ = 0. If the value of θ is not large the value of cosθ can be taken as unity approximately and the influence of the factor cosθ in the equation (2) can be neglected.
Neglecting the influence of cosθ, it is clear that the angular dispersion of any two spectral lines (in a particular order) is directly proportional to the difference in wavelength between the two spectral lines. A spectrum of this type is called a normal spectrum.
If the linear spacing of two spectral lines of wavelength λ and λ + dλ is dx in the focal plane of the telescope objective or the photographic plate, then,
dx = fdθ
Where ƒ is the focal length of the objective. The linear dispersion

The linear dispersion is useful in studying the photographs of a spectrum.
Q11) Discuss construction and working Laurent’s half shade Polarimeter?
A11) Construction

Figure: Laurent’s half shade polarimeter
Working
To understand the working of this device we have to know the need of a Laurent half shade device. For this we assume that half shade device is not present.
In this basic setup (without the half-shade A) you are looking for the maximum and minimum brightness, which then tells you that the analyzer A is precisely aligned with the output rotation.
When tube is empty placed the analyzer in such a position that the view of field is completely dark. Note the position of the analyzer with the help of circular scale.
Now filled the tube with optically active solution and it is set in its proper position. The optically active solution rotates the plane of polarization of the light emerging out of the polariser P by some angle. So, the light is transmitted by analyzer A and the field of view of telescope becomes bright.
Now the analyzer is rotated by a finite angle so that the field of view of telescope again become dark. This will happen only when the analyzer is rotated by the same angle by which plane of polarization of light is rotated by optically active solution.
The position of analyzer is again noted. The difference of the two readings will give you angle of rotation of plane of polarization.
But here the difficulty associated with this procedure that when analyzer is rotated for the total darkness, then it is attained gradually and hence it is difficult to find the exact position correctly for which complete darkness is obtained.

Figure: Laboratory Set Up of Polarimeter
To overcome above difficulty half shade device is introduced between polariser P and glass tube T.
Half Shade Device

Figure: Half Shade Device
Now if the principal plane of the analysing Nicol is parallel to OP, then the light will pass through glass half passable. Hence glass half will be brighter than quartz half or we can say that glass half will be bright and the quartz half will be dark. Similarly, if principal plane of analysing. Nicol is parallel to OQ then quartz half will be bright and glass half will be dark.
When the principal plane of analyzer is along AOB then both halves will be equally bright. On the other hand, if the principal plane of analyzer is along DOC. then both the halves will be equally dark.
Thus, it is clear that if the analysing Nicol is slightly disturbed from DOC, then one half becomes brighter than the other. Hence by using half shade device, one can measure angle of rotation more accurately.
Mathematically
The specific rotation is given by S=θ/lC where l is length of tube and C is concentration.
Q12) Write the point of Differences between Positive Crystal and Negative Crystal
A12) Difference between Positive Crystal and Negative Crystal
Positive Crystal | Negative Crystal |
|
|
2. The crystals in which vo>ve are termed as positive. In positive crystals E-ray travels slower than o-ray in all directions except along the optic axis. | 2. The crystals in which vo<ve are termed as negative. In negative crystals O-ray travels slower than E- ray in all directions except along the optic axis. |
3. According to Huygen's ellipse corresponding to e-ray is contained within the sphere corresponding to o-ray | 3. According to Huygen's ellipse corresponding to e-ray lies outside the sphere corresponding to O-ray |
4. Birefringence or amount of double refraction of a crystal is defined as ∆μ=μe-. μ for ∆μ is positive quality for positive crystals. | 4. ∆μ Negative for negative crystals. |
5. Example: Quartz | 5. Example: calcite |
Q13) In a Newton’s rings experiment the diameter of the 15th ring was found to be 0.59 cm and that of the 5th ring is 0.336 cm. If the radius of curvature of the lens is 100 cm, find the wave length of the light.
A13) The given data are
Diameter of Newton’s 15th ring (D15) = 0.59 cm = 0.59×10–2 m
Diameter of Newton’s 5th ring (D5) = 0.336 cm = 0.336 × 10–2 m
Radius of curvature of lens (R) = 100 cm = 1 m
Wave length of light (λ) =?
Here m is difference between rings = 15-5=10
λ = D2n+m - D2n / 4mR
λ = (0.59×10–2 )2- (0.336 × 10–2)2 / 4 x 10 x 1
λ = 0.3481 x10-4 – 0.112896 x10-4 / 40
λ =0.00588 x 10-4 m
λ =5880 x 10-10 m
λ =5880Å
Q14) Discuss Newton’s Ring and also derive the formula for diameter of bright and dark fringes?
A14) Newton’s Rings
Newton’s Rings are the circular interference pattern first discovered by physicist Sir Isaac Newton in 1704. It is cosists of concentric bright and dark rings with the point of contact of lens and the glass plate as centre,
The fringes obtained by interference of light waves by using the following arrangement
When a Plano convex lens with large radius of curvature is placed on a plane glass plate such that its curved surface faces the glass plate, a wedge air film (of gradually increasing thickness) is formed between the lens and the glass plate. The thickness of the air film is zero at the point of contact and gradually increases away from the point of contact.
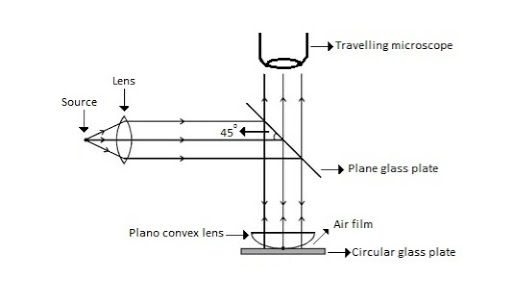
Figure: Newton Ring Assembly
If monochromatic (means light with single wavelength) light is allowed to fall normally on the lens from a source 'S', then two reflected rays R1 (reflected from upper surface of the film) and R2 (reflected from lower surface of the air film) interfere to produce circular interference pattern. This interference pattern has concentric alternate bright and dark rings around the point of contact. This pattern is observed through traveling microscope.
Mathematical analysis of Newton’s Ring
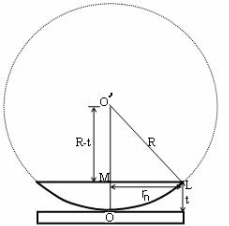
Figure: Mathematical analysis of Newton’s Ring
(OL)2 =(O’M)2-(ML)2 ………. (1)
R2=(R-t)2 +rn2
R2=R2 +t2-2Rt +rn2
Radius is large as compared to the thickness
so t2 is neglected as t2<< R2
R2=R2 +-2Rt +rn2
2Rt =rn2
Thickness of the film t =rn2 /2R ………. (2)
Theory of Fringes:
The effective path difference between the two reflected rays R1 and R2 for a wedge-shaped film from equation
∆ = 2μtcos(r+θ) +λ/2 ………. (3)
If the light is incident normally on the lens,
r = 0 and near to point of contact θ is small;
Therefore, near point of contact, (r+θ) approaches to 0 and cos(r+θ) =cos0=1
Therefore
∆ = 2μt+λ/2 ………. (4)
Also, At point of contact t = 0 therefore the effective path difference ∆ = λ/2
Which is odd multiple of λ/2 Therefore the Central fringe being dark.
Bright Fringe: Condition of Maxima
For the condition of maxima, the effective path difference
∆ = ±nλ
Using equation (4) ∆ = 2μt+λ/2 we have
2μt+λ/2= ±nλ
2μt = ± (2n-1) λ /2 ………. (5)
Diameter of Bright Rings
we know by equation (2) t =rn2 /2R substitute in equation (5) we have
2μ (rn2 /2R) = ± (2n-1) λ /2
rn2 = ± (2n-1) λR /2μ
We know diameter D=2r and for nth fringe Dn=2rn
so, we have Dn2=± 2(2n-1) λR /μ
Dn=
The medium enclosed between the lens and glass plate is if air therefore,  =1. The diameter of nth order bright fringe will be
=1. The diameter of nth order bright fringe will be
D= n=0,1,2,3,4……. ………. (6)
n=0,1,2,3,4……. ………. (6)
The diameter of bright ring is proportional to square root of odd natural numbers
Dark Fringe: Condition for Minima
For the condition of minima, The effective path difference
∆ =± (2n+1) λ /2
2μt+λ/2 =± (2n+1) λ /2
2μt= ±nλ ………. (7)
it is clear that for particular dark or bright fringe t should be constant.
Every fringe is the locus of points having equal thickness. Hence the fringes are circular in shape.
Diameter of Dark Rings
we know by equation (2) t =rn2 /2R substitute in equation (7) we have
2μ (rn2 /2R) = nλ
rn2 = nλR/ μ
We know diameter D=2r and for nth fringe Dn=2rn
so, we have Dn2= 4nλR/ μ
Dn= 
The medium enclosed between the lens and glass plate is if air therefore,  =1. The diameter of nth order bright fringe will be
=1. The diameter of nth order bright fringe will be
Dn=  n=0,1,2,3,4……. ………. (8)
n=0,1,2,3,4……. ………. (8)
The diameter of dark ring is proportional to square root of natural numbers
Q15) Newton’s rings are observed in the reflected light of wave length 5900 Å. The diameter of 10th dark ring is 0.5 cm. Find the radius of curvature of the lens used.
A15) The given data are
Wave length of light (λ) = 5900 Å= 5900 × 10–10 m
Diameter of 10th Newton’s dark ring (D10) = 0.5 cm = 0.5 × 10–2 m
Radius of curvature of lens (R) =?
Formula is D2n = 4nλR
R= D2n /4nλ
R= (0.5 × 10–2)2 /4x 10 x 5900 × 10–10
R= 0.25 x10-4 /236x10-7
R=1.059m
Q16) Show that the fringe width of newton’s rings reduces with increase in n.
or
Show that the spacing of newton’s rings gets closer with increase in n.
A16) Spacing between Fringes
The Newton’s rings are not equally spaced because the diameter of ring does not increase in the same proportion as the order of ring and rings get closer and closer as ‘n’ increases.
For example, the diameter of dark ring is given by Dn=  where n=0,1,2,3,4…….
where n=0,1,2,3,4…….
D3 - D2 = -
-  = (
= ( -
- )
) = 0.635
= 0.635
D7 – D6 = -
-  = (
= ( -
- )
) = 0.392
= 0.392
D10– D9 = -
-  = (
= ( -
- )
) = 0.324
= 0.324
From above result we conclude that the fringe width reduces with increase in n.
Q17) What happens when we use white light in newton’s rings method instead of monochromatic light?
A17) Newton’s Ring with White Light
If the monochromatic source is replaced by the white light, dark and bright fringes are not produced. Because the diameter of the rings depends upon wavelength and it is proportional to the square root of wavelength.
If example, the monochromatic source is replaced by the white light superposition of rings take place due to different wavelength. Few coloured rings are seen around dark centre later illumination is seen in the field of view. As shown in below figure.

Figure: Newton’s ring with white light
Q18) What do you mean by interference?
A18) Interference in light waves occurs whenever two or more waves overlap at a given point.
TYPES OF INTERFERENCE
Interference of light waves can be either constructive interference or destructive interference.
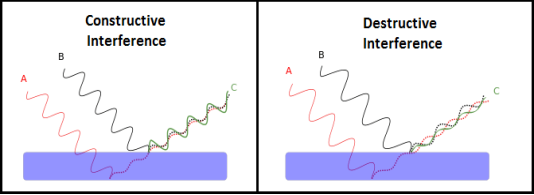
Figure: Types of interference
We know that the superposition of two mechanical waves can be constructive or destructive. In constructive interference, the amplitude of the resultant wave at a given position or time is greater than that of either individual wave, whereas in destructive interference, the resultant amplitude is less than that of either individual wave.
Light waves also interfere with each other. Fundamentally, all interference associated with light waves arises when the electromagnetic fields that constitute the individual waves combine.
If two light bulbs are placed side by side, no interference effects are observed because the light waves from one bulb are emitted independently of those from the other bulb. The emissions from the two light bulbs do not maintain a constant phase relationship with each other over time. Light waves from an ordinary source such as a light bulb undergo random phase changes in time intervals less than a nanosecond.
Therefore, the conditions for constructive interference, destructive interference, or some intermediate states are maintained only for such short time intervals. Because the eye cannot follow such rapid changes, no interference effects are observed. Such light sources are said to be incoherent.
Q19) What are the conditions to obtain sustained interference of light?
A19) In a sustained interference pattern, the position of maximum and minimum intensity regions remains constant with time. To obtain the sustained interference, the following conditions are required:
Q20) Discuss the phenomenon of interference by Division of Wavefront and Division of Amplitude
A20)The phenomenon of interference may be grouped into two categories:
Division of Wavefront
Under this category, the coherent sources are obtained by dividing the wavefront, originating from a common source, by employing mirrors, biprisms or lenses. This class of interference requires essentially a point source or a narrow-slit source. The instruments used to obtain interference by division of wavefront are the Fresnel biprism, Fresnel mirrors, Lloyd's mirror, lasers, etc.
Some of the standard arrangements used to observe two-wave interference by division of the wavefront are shown in Figure.

Figure: Two-wave interference; (a) Young’s two-slit arrangement, (b) Fresnel’s
biprism, (c) Fresnel’s two mirrors, (d) Loyd’s mirror
In Figure 9(a) The narrow slits S1 and S2 in Young’s double slit arrangement intercept portions of the spherical wavefront and act as real mutually coherent point sources, diffracting light in the forward direction. Interference among the diffracted waves can be observed anywhere in the region of overlap (shaded portion) behind the plane of the slits. Historically, the interference produced in Young’s double slit experiment was not accepted as a conclusive proof of the wave nature of light because it was thought that the fringes in Young’s experiment could arise due to some unexplained interaction of light with the edges of the slits.
In Figure 9(b) Fresnel’s biprisms arrangement, however, established the wave nature of light beyond any doubt. Fresnel’s biprism consists of two small angles (a few degrees) prisms in a manner that the incident spherical wavefront is split by the two prisms. The split wavefronts travel in different directions, eventually overlap and produce interference. S1 and S2 act as virtual but mutually coherent point sources.
Figure 9(c) shows the two-mirror arrangement, also devised by Fresnel. Here, the portions of the spherical wavefront reflected by the two mirrors overlap to produce interference. The source images S1 and S2, formed by the two mirrors, are virtual but mutually coherent.
In Figure 9(d) shows Lloyd’s single mirror arrangement, interference is produced by the portion of the wavefront reflected by the mirror and the portion which propagates directly to the region of superposition. In this case, the point source S and its virtual image S’ act as mutually coherent point sources.
Division of Amplitude
In this method, the amplitude of the incident beam is divided into two or more parts either by partial reflection or refraction. Thus, we have coherent beams produced by division of amplitude. These beams travel different paths and are finally brought together to produce interference. The effects resulting from the superposition of two beams are referred to as two beam interference and those resulting from superposition of more than two beams are referred to as multiple beam interference. The interference in thin films, Newton's rings, and Michelson's interferometer are examples of two beam interference and Fabry-Perot's interferometer is an example of multiple beam interference.
In case of division by wavefront, we considered two-wave interference when different portions of the wavefront are made to propagate in different directions and then recombined. Now, we discuss two-wave interference when a quasi-monochromatic wave is incident on a thin transparent film as shown in Figure. Here we will discuss interference by division of amplitude.
The wave is partly reflected and partly transmitted at each interface. Amplitudes of successively reflected and transmitted waves diminish rapidly for films of low reflectivity. The amplitude transmission coefficient for passage of the wave from the medium of refractive index n1 to the medium of refractive index n2 is t and t’ is the corresponding amplitude transmission coefficient for passage in the reverse direction.

Figure: Reflection and transmission across a thin film.
The amplitude reflection coefficients for the external and internal reflections are r and r’=−r, respectively. For sufficiently small r (r2 <1), only two waves need to be considered in reflection as well as in transmission, leading to two-wave interference. The amplitudes of the first two waves in reflection are comparable, but those in transmission differ considerably.
As a result, interference fringes in reflected light have higher visibility than those in transmitted light.
Q21) Explain Fraunhoffer diffraction at single slit in detail?
A21) When the light falls on the obstacle whose size is comparable with the wavelength of light then the light bends around the obstacle and enters in the geometrical shadow. This bending of light is called diffraction.
FRAUNHOFFER DIFFRACTION AT SINGLE SLIT
The adjacent figure represents a narrow-slit AB of width ‘e’. Let a plane wavefront of monochromatic light of wavelength '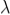 ' is incident on the slit. Let the diffracted light be focused by means of a convex lens on a screen. According to Huygen Fresnel, every point of the wavefront in the plane of the slit is a source of secondary wavelets. The secondary wavelets traveling normally to the slit i.e., along OP0 are brought to focus at -+
' is incident on the slit. Let the diffracted light be focused by means of a convex lens on a screen. According to Huygen Fresnel, every point of the wavefront in the plane of the slit is a source of secondary wavelets. The secondary wavelets traveling normally to the slit i.e., along OP0 are brought to focus at -+
az P0 by the lens. Thus, P0 is a bright central image. The secondary wavelets traveling at an angle '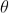 ' are focused at a point P1 on the screen.
' are focused at a point P1 on the screen.
The intensity at the point P1 is either minimum or maximum and depends upon the path difference between the secondary waves originating from the corresponding points of the wavefront.
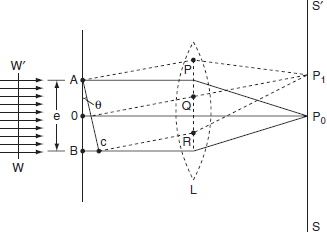
Figure: Fraunhoffer diffraction at single slit
Theory:
In order to find out the intensity at P1, draw a perpendicular AC on BR.
The path difference between secondary wavelets from A and B in direction θ is BC i.e,
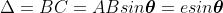
So, the phase difference,
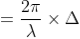
Let us consider that the width of the slit is divided into ‘n’ equal parts and the amplitude of the wave from each part is ‘a’.
So, the phase difference between two consecutive points
 ........... (1)
........... (1)
Then the resultant amplitude R is calculated by using the method of vector addition of amplitudes
Figure: Mathematical analysis of Fraunhoffer diffraction at single slit
The resultant amplitude of n number of waves having same amplitude 'a' and having common phase difference of '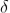 ' is
' is
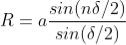 ................... (2)
................... (2)
Substituting the value of 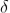 in equation (2)
in equation (2)
R = a  ................... (3)
................... (3)
Substituting =
=  .esinθ in equation (3)
.esinθ in equation (3)
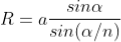
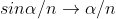
As  is small value; sin
is small value; sin 
R = n
and na = A
Therefore
R =A ................... (4)
................... (4)
Therefore, the Intensity is given by
I =R2 = A2  ................... (5)
................... (5)
Case (i): Principal Maximum:
Eqn (4) takes maximum value for
 = 0
= 0
 =
=  .esinθ = 0
.esinθ = 0
sinθ = 0 or θ=0
The condition
The condition θ=0 means that this maximum is formed by the secondary wavelets which travel normally to the slit along OP0 and focus at P0. This maximum is known as “Principal maximum”.
Intensity of Principal maxima
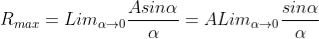
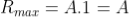
Therefore
Imax = R2max = A2
Case (ii): Minimum Intensity positions:
Equation (3) takes minimum values for sin The values of '
The values of '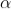 ' which satisfy sin
' which satisfy sin are
are
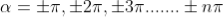
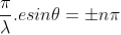
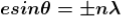 where
where 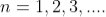 ………... (6)
………... (6)
In the above equation (6) n = 0 is not applicable because corresponds to principal maximum. Therefore, the positions according to equation (6) are on either side of the principal maximum.
Case (iii): Secondary maximum:
In addition to principal maximum at  = 0, there are weak secondary maxima between minima positions. The positions of these weak secondary maxima can be obtained with the rule of finding maxima and minima of a given function in calculus. So, differentiating equation (5) and equating to zero, we have
= 0, there are weak secondary maxima between minima positions. The positions of these weak secondary maxima can be obtained with the rule of finding maxima and minima of a given function in calculus. So, differentiating equation (5) and equating to zero, we have
 =
=  (A2
(A2  ) =0
) =0
 = 2A2
= 2A2  (
(  )(
)( =0
=0
A2  0;
0;  =0
=0
Because  =0 correspond to minima positions
=0 correspond to minima positions
 =0
=0
 ………... (7)
………... (7)
The values of '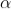 ' satisfying the equation (7) are obtained graphically by plotting the curves
' satisfying the equation (7) are obtained graphically by plotting the curves  and
and  on the same graph.
on the same graph.
The points of intersection of the two curves gives the values of  which satisfy equation (7).
which satisfy equation (7).
The points of intersections are
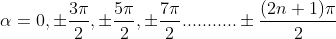
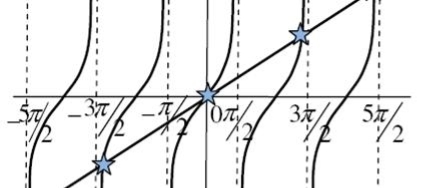
Figure: Variation of intensity
But  , gives principal maximum, substituting the values of
, gives principal maximum, substituting the values of  in equation (5), we get
in equation (5), we get
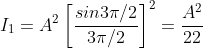

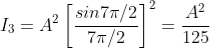
and so on.
From the above expressions, Imax, I1, I2, I3… it is evident that most of the incident light is concentrated at the principal maximum.
INTENSITY DISTRIBUTION GRAPH
A graph showing the variation of intensity with ' ' is as shown in the adjacent figure
' is as shown in the adjacent figure
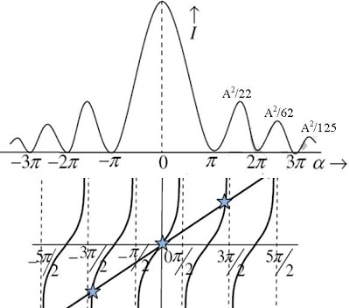
Figure: Intensity Distribution Graph