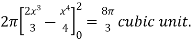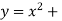Unit-2

Multivariable Calculus-II

Q1: Evaluate.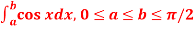

A1. Here we notice that f:x→cos x is a decreasing function on [a , b],

Therefore by the definition of the definite integrals-

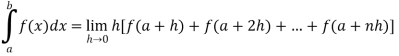
Then

Now,

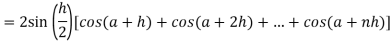
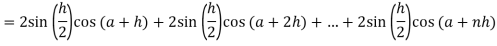
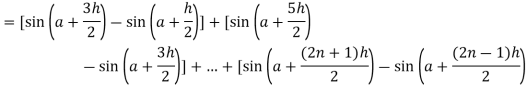
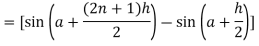
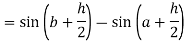
Here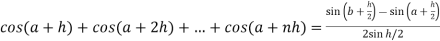

Thus

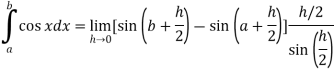
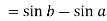
Q2: Evaluate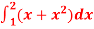

A2. Here 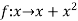is an increasing function on [1 , 2]

So that,

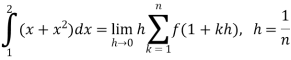
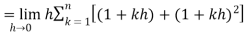
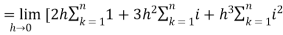  …. (1)

We know that-

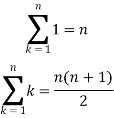
And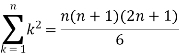
 

Then equation (1) becomes-

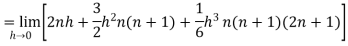
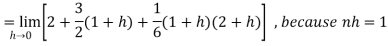
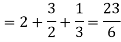
Q3: Find out the integral 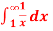is convergent or divergent. Find the value in case of convergent.

A3.  Here we will convert the integral into limit ,

=

              =

              =

              = ∞

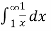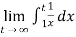
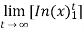
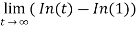
As we can see, here limit does not exist. i.e. that is infinity.

So we can say that the given integral is divergent.

Q4: Find out the integral is convergent or divergent. Find the value in case of convergent.

A4.  Follow the same process as we did above,

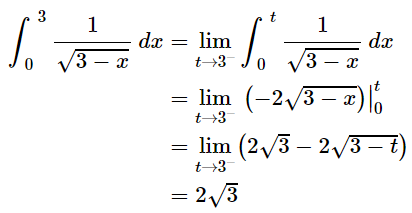
Here limit exists and finite , so that the integral is convergent. And its value is 2√3.

Q5: Evaluate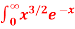 dx

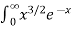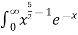A5: dx = dx

= γ(5/2)

= γ(3/2+ 1)

= 3/2 γ(3/2 )

= 3/2.  ½ γ(½ )

= 3/2. ½ π

=    ¾ π

Q6: Evaluate 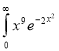  dx.

A6:   Let 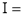 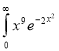 dx.

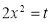Put or 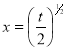 ;             4x dx = dt

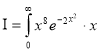dx

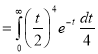
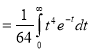
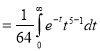
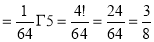
Q7: Evaluate    I   =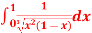  

A7: 

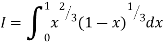
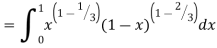
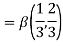
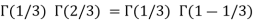
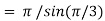
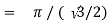
= 2 π/3

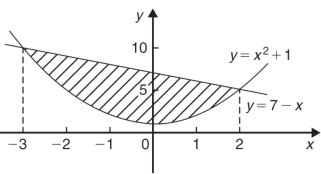
Q8: Determine the area enclosed by the curves-

A8:. We know that the curves are equal at the points of interaction, thus equating the values of y of each curve-

Which gives-

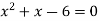
By factorization,

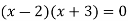
Which means,

x = 2 and x = -3

By determining the intersection points the range the values of x has been found-

And

We get the following figure by using above two tables-

Area of shaded region =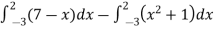

=

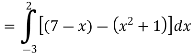
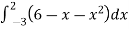
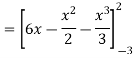
= ( 12 – 2 –  8/3 ) – (-18 – 9/2 + 9)

=

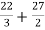
= 125/6 square unit

Q9: Find the area enclosed by the curves and if the area is rotated about the x-axis then determine the volume of the solid of revolution.

A9:  We know that, at the point of intersection the coordinates of the curve are equal. So that first we will find the point of intersection-

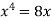
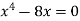
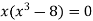
We get,

x = 0 and x = 2

The curve of the given equations will look like as follows-

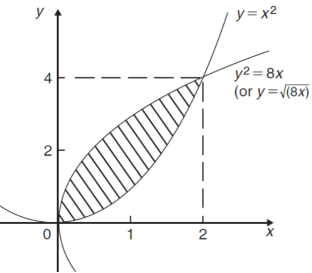
Then,

The area of the shaded region will be-

A =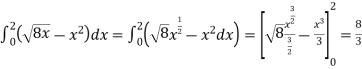

So that the area will be 8/3 square unit.

The volume will be

= (volume produced by revolving 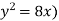– (volume produced by revolving

=

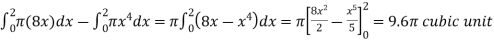
Q10: Find the volume of the solid of revolution formed by revolving R around y-axis of the function f(x) = 2x - x² over the interval [0 , 2].

A 10:. The graph of the function f(x) = 2x - x² will be-

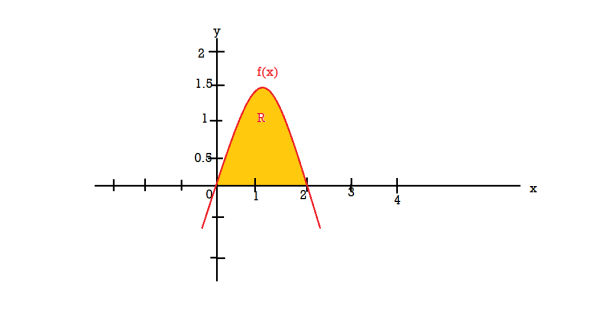
The volume of the solid is given by-

=
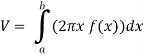
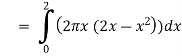
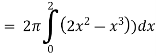