Unit - 4
Photogrammetric Survey
Q1) What are the types of Photogrammetry?
A1)
- Aerial Photogrammetry
The camera is mounted in an aircraft, usually pointed perpendicular towards the ground with the camera axis vertical or nearly so.
Many photographs are taken with the clincher – built concept. Later the processing of these photographs done using stereo-plotter.
Photos are also being used in for Digital Elevation Model (DEM) creation.
- Terrestrial Photogrammetry
Photographs are taken from a stable, usually known as, position on or near the ground and with the camera axis horizontal or nearly so.
The position and orientation of the camera are frequently measured directly at the time of exposure. Instrument used for exposing such photograph is known photo theodolite.
- Space Photogrammetry
In this type of photogrammetry, satellites are used.
Global coverage of satellite image is taken in lesser time with a high-resolution data.
- Interpretative Photogrammetry
Images are studied and identification is done for judging their significance with standard and careful analysis.
Q2) Explain in detail about Aerial Camera?
A2)
Main function of terrestrial camera and aerial camera is same. In both the camera, photographs are taken. But location of the aerial camera is different. It is located or mounted on a fast-moving airplane, therefore its requirements are different rather than terrestrial camera.
Following things are required for aerial camera:
- It requires fast lens.
- It requires high speed emulsion for the film.
- It requires a magazine so as to hold large rolls of film.
- It requires high speed and efficient shutter.
Aerial camera is used as a surveying instrument of high precision.
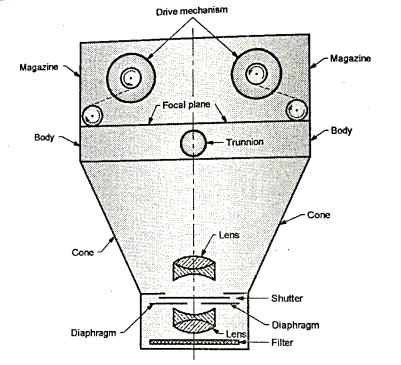
Fig.: Schematic diagram of an aerial camera
Parts of the aerial camera:
Following are the Parts of the aerial camera:
(a) Lens assembly
(1) Lenses
(2) Diaphragm
(3) Shutter
(4) Filter
(b) Camera cone
(c) Focal plane
(d) Magazine
(e) Camera body
(a) Lens assembly
It consists of four parts viz (1) lenses (2) diaphragm (3) shutter and (4) filter.
(1) Lenses
- Camera has high performance lenses manufactured by wild Heerbrugg Ltd. Wild aviotar f: 4, wild universal aviogon f: 5.6, wild super-Aviogon f: 5.6. Camera can have normal angle lenses as well as wide and super angle lenses as per the requirement of accuracy.
- The other lenses which are commonly used are:
- Bausch and Lomb metrogen f: 6.3.
- Zeiss Topogon f: 6.3,
- Goertz Aerotar f: 6.8.
- The focal plane of the aerial camera can be fixed at one location. Therefore, an aerial camera is always of a fixed focus type of camera.
(2) Diaphragm
- A diaphragm is situated between the lenses. Lens system can physically be opened by diaphragm.
- Diaphragm is made of series of leaves. Series of leaves can be rotated as per the requirement of the opening size whether increase or decrease; which restrict the size of rays to pass through lens.
- Shutter speed should be greater if the diaphragm opening is large.
(3) Shutter
- Function of camera shutter is to control the interval of time when the light is allowed to pass through lens.
- Aerial camera is mounted on aeroplane which moves with a very high speed, hence a fast speed shutter is needed so as to avoid the possibility of blurring of the image due to vibration of camera and forward motion of aeroplane. The shutter speed varies from 1/100 second to 1/1000 second.
- There are three types of shutters probably used in aerial camera.
- Types of shutters
- Focal plane type
- Louvre type
- Between-the lens type
(4) Filter:
- Piece of coloured glass is placed in front of the lens in case of filter.
- Filtration of stray light i.e., blue and violet light in the atmosphere is done by the filter and filter also protect the lens from the dust particles.
(b) Camera cone
- Complete lens assembly including filter is supported by the camera cone. Focal plane is provided at the top of the camera cone. The camera cone is made up of material whose co efficient of thermal expansion is low so that complete system will stand still in operational temperature.
(c) Focal plane
- The focal plane is exactly provided above the collimation marks. Focal plane is adjusted at such a distance from the near nodal point so that best and possible image can be obtained.
(d) Magazine
- The exposed and unexposed films is held by magazine. Magazine houses the film flattening device at the focal plane. Power is supplied to the movable parts of the magazine by drive mechanism.
(e) Camera body
- At the top of the cone, the camera body is provided and it acts as an integral part to preserve the interior orientation.
(f) Drive mechanism
- Drive mechanism is located in the camera body and can be operated automatically or manually.
- It is used for the following purposes:
- Winding and tripling of shutter is done by drive mechanism.
- It operates the vacuum system to flatten the film.
- It winds the film.
Q3) What is the Scale of vertical photograph?
A3)
- Aerial photographs do not have a uniform scale and it vary from point to point depending upon the ground profile whether tilt and tip.
- If the terrain to be photographed is flat, the photographs taken are vertical.
- Though the ground is tilt or tip and if the altitude of the camera is known, a datum can be selected and scale of the photograph can be determined for the datum. Note that if the altitude of the airplane is changed, then scale of photograph is also changed. Most of the scales lies between 1 cm= 80 m and 1 cm= 800 m.
- In Fig. The line PQ is at a height h, above the datum and photographic images of P, Q, R, S on the photographs are p, q, r and s respectively.
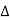 OPQ and
OPQ and 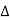 opq are two similar triangles as shown in Fig. Hence scale of the photographic image of the line pq to the ground line PQ is given by following expression,
opq are two similar triangles as shown in Fig. Hence scale of the photographic image of the line pq to the ground line PQ is given by following expression,
Scale (s) = 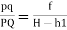 ... (by property of two similar triangle)
... (by property of two similar triangle)
- Similarly, the line RS is at height of h, above the datum and the photographic images of ground line RS is rs. Hence scale of the photographic image of the line rs to the ground line RS is given by following expression.
Scale (s)= 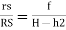 ... (by property of two similar triangle)
... (by property of two similar triangle)
- Hence, in general, the scale of a photograph for any ground height or ground elevation is given by the following expressions,
∴S =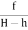
Where, S= scale
H =altitude of the aircraft above mean sea level
h = height or elevation of the ground above mean
Sea level
f = focal length of the camera
- For a vertical photograph, it is not easy to have a uniform scale; but lengths of the lines can be calculated from the ground co-ordinates say (X, Y) and photographic ordinates say (x, y) by following expression,
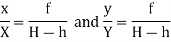
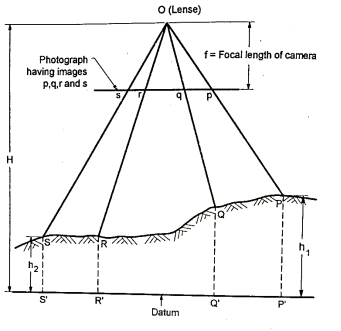
Fig.: Scale of Vertical Photograph
- Plumb point is taken as an origin in case of ground co-ordinates and co-ordinates axes are parallel to the geometrical axes of the photograph in which X-axis is in the direction of the line of flight.
To find the length of a line PQ:
- It is essential to find the length of a line PQ on the ground with the help of end point's co-ordinates p and q on photograph by using the principal point considered as an origin.
- From last Equation, we can write the co-ordinates as,
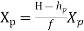 ,
,

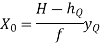
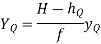
- By distance formula, we get,
True horizontal distance (PQ) = 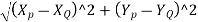
- Average photographic scale can be determined from Equation by using the average aircraft altitude for 'H' and the average height or elevation of the area for 'h'. Note that the ground distance obtained by using average scale gives only appropriate value of ground distance.
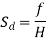
Where, Sd=datum scale
f = focal length of the camera
H=altitude of the aircraft above mean sea level.
- Note that datum scale is the scale which is effective over the entire photograph.
Q4) What is the Relief displacement of a vertical photograph and height of object from relief displacement?
A4)
- It is defined as the distance on a photograph from the image of any ground point to its fictitious image projected to a datum plane which is the image displacement due to topographic relief.
- All the objects other than the objects in the plump point and datum plane are displaced because all the photograph are perspectives.
- The relief displacements are always radial with respect to principal point on the vertical photograph.
- Refer to the Fig. For better understanding and further simplification.
- PP1 is the relief displacement as shown in Fig.
Let, R=ground distance PQ
ro =radial distance of 'p' from the principal point 'q'.
r₁ = radial distance of 'p1’ from the principal point 'q'.
In Fig. p1qO and P1 K1 O are two similar triangles.
From the property of the similar triangle, we can write as,
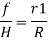
∴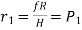 … (1)
… (1)
- A pq0 and PQ0 O are similar triangle as shown in Fig.
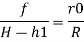
∴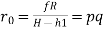 … (2)
… (2)
- Let 'd' be the relief displacement and if h, is the height of an object then,
d= qp-qp1
d=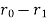 …(3)
…(3)
Substituting the value of ro from Equation 2 and r from Equation 1 in Equation 3 we get,
∴d=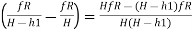
∴d=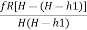
d =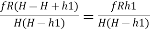
∴ d=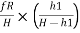
∴d = 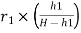
d =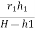 … (4)
… (4)
- Equation is used to determine the height of objects at the time of non-availability of stereoscopic instruments.
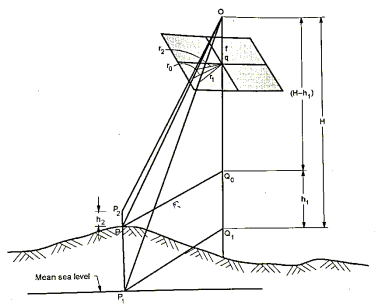
Fig.: Relief Displacement
- The height of an object is generally measured above the ground instead of taking above the datum.
- If h2 is the height of an object, then we can write as,
d =q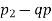
d =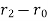 … (5)
… (5)
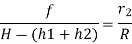
∴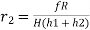 … (6)
… (6)
Substituting the value of r2 from Equation 5 and r0 from Equation 2, we get,
d = 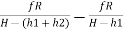
By solving the equation, we get,
∴ d =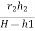 … (7)
… (7)
- It has been observed from the Equation 4 and 7. The relief displacement decrease when increase in flying height, but it increases as the distance increases form the principal point.
- Relief displacement is + ve and radially outwards for the points above the datum plane and it is - ve and radially inwards for the points below the datum plane.
- Note that the relief displacement is zero for the points lies vertically below the exposure station and also it is zero for the flat ground and in this way the vertical photograph can be used as a map.
Q5) What is the flight planning for aerial photography?
A5)
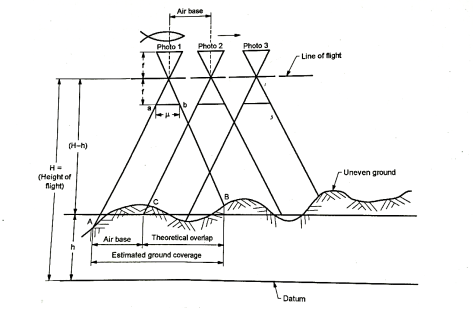
Fig.: Vertical profile through a flight line
- Flight planning consists of the following information:
(a) The area to be surveyed
(b) Focal length of the camera
(c) Scale of the photograph
(d) Longitudinal and side overlap
(e) Approximate ground speed of the aircraft in stable air
- The above information is required to find the altitude of the aircraft above datum, time interval between the exposures, the area covered by each photograph, number of photographs number of strips and air base.
- To cover the entire terrain to be surveyed, the sufficient photographs with suitable overlap should be taken.
1.Longitudinal overlap or forward overlap
- The overlap of the photographs occurs in the direction of flight line is called as longitudinal overlap or forward overlap. This type of overlap is kept at about 60%.
- Vertical profile through a flight line can be easily understood by the Fig. 7.13.1.
2. Side overlap
- The overlap between the adjacent flight lines is called as side overlap which is kept at about 30%.
- Note that, there must be some overlap of the area covered by each aerial photograph so as to ensure a complete coverage of the area to be surveyed.
- Following are the reasons for overlap:
- Overlap is provided to orient prints so that there is a continuous flight strip formation.
- Overlap is provided so as to discard the highly distorted outer portion of the photograph.
- The overlapped portion is only useful for a stereoscopic vision.
- The overlap is provided to prevent the possibility of gaps left because of the deviation of the aircraft from the flight line.
- Other reasons to provide overlap are drift and crab.
3. Drift
- The failure of the aircraft to remain stay along with the flight line is called as drift when wind correction is not applied then drift is occurred.
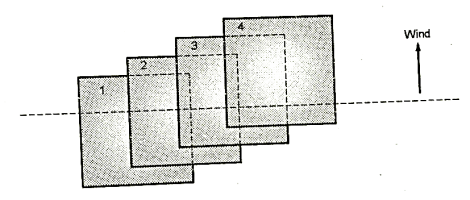
Fig.: Drift
4. Crab
- When the camera is not square with respect to the direction of the flight at the time of exposure, then it is called as crab.
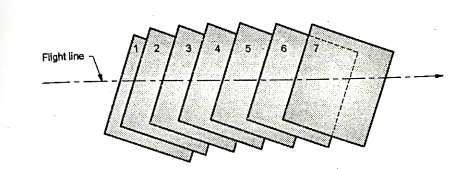
Fig.: Crab
5. Altitude of aircraft
- Flying altitude (H) deals with the scale of the photograph, C factor and the contour interval and it can be determined by the following formula
H = (Counter interval) x (C-factor)
Where, H= flying height
C-factor varies from 500 to 1500
- H can also be computed by the following formula
S=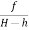
Where S= Scale of photograph:
f = Focal length of camera
H= Flying height
h =Elevation of the ground above m.s.l.
6.Area covered by one photograph
- Area covered by one photograph can be determined provided the length and the exposed portion and the scale of the photograph are known.
- Area covered by one photograph = [length x scale] [width x scale]
7. Number of photographs to cover a given area
- Net length covered by each photograph (L) = (1-P₁) Sl
Where, Pl = Longitudinal overlap
l = Length of the photograph in the direction of flight
S = Scale of a photograph
- Net width covered by each photogram (w)= (1-Pw) Sw
Where, Pw =Side overlap
S = Scale of a photograph
W=Width of the photograph normal to the direction of flight
- If the dimensions of the area A (L1 x B₁) are given, the number of photographs required is determined by calculating the number of photographs in each strip and the number of strips.
Consider,
N₁ = Number of photographs in each strip
N2 = Number of strips required
∴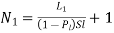
Where, L1 =Length of the area
Pl =Longitudinal overlap
S= Scale of a photograph
l= Length of the photograph in the direction of flight
∴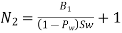
Where B1 =Width of the area
Pw = Side overlap
S =Scale of photograph
w = Width of the photograph normal to the direction of flight
Hence number of photographs required = N₁XN₂
8.Interval between exposures:
- The time interval between exposures in seconds is given by the following expression
T =
Where, T = Time interval between exposures in seconds
L = Ground distance covered between exposures along the flight line in km
V = Ground speed of the aircraft in km/h
9. Air base
- The air base is defined as the distance covered by an aircraft between any two successive exposures. Air base is shown in Fig. for better understanding.
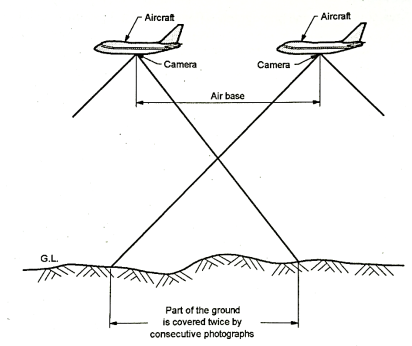
Fig.: Air base
Q6) Explain stereoscope and stereoscopic views?
A6)
- Stereoscopy is the process in which stereoscope is used to bring out the difference in elevation so that the photograph stands out in relief. In this way, the main function of a stereoscope is to accommodate the wide separation of the individual photograph of the stereopair to the fixed length of the eye base.
- Stereoscopic viewing makes it possible to measure elevations and plot the contours and planimetric features from the photographs.
- The instrument used called as stereoscope which enables the observer to see the spatial model of the area photographed.
- Stereoscopy is the significant part of aerial photogrammetric surveying which deals with the depth of perception and with the help of depth perception, the relative distance of objects is determined.
Stereoscopic Vision on Vertical Photographs:
- The process of determining relative distance of the objects from the observer by the impressions received through eyes is called as the depth perception.
- The phenomenon of stereoscopic vision makes possible to an observer to view an object more precisely and distinctly and also two different perspectives of an object so as to obtain a mental impression of the 3-dimensional image.
- The stereoscopic vision is suitable and possible upto 600 m only because parallactic angle is very small and hence it is not possible to have the vision beyond the distance of 600 m with naked eye.
Types of stereoscopic vision:
- Orthoscopic view
- Pseudoscopic view
1.Orthoscopic view
- By keeping the overlaps of the stereo pair inwards and observing under a stereoscope, the orthoscopic view can be obtained. The natural order of elevation and depth remains as it is.
2.Pseudoscopic view
- By keeping the overlaps of the stereoscopic view outwards, a pseudoscopic view can be obtained. The natural order is reversed in such case.
Requirements for stereoscopic vision:
- Following are the important requirement for stereoscopic vision:
(a) From the different points, the views should be taken.
(b) There should be the intersection of the direction vision and image points in space.
(c) Separate image should be presented simultaneously to each eye so as to have parallax.
Requirements of stereoscopic pair:
- For production of a spatial model, the two over-lapping photographs should follow the requirements as given below.
(a) At the time of exposures, the camera axes must be co-planer.
(b) The two photographs should have minimum of 60% common overlap.
(c) There should not be large convergence of the optical axes of the cameras.
(d) Difference in scale of photographs upto 15% can be considered. If the scale difference is more than 15% can be considered. If the scale difference is more than 15%, then it is very difficult to take observations and so for the measurements.
Q7) Explain Parallax equation?
A7)
- With the help of parallax measurements, the co-ordinates and elevation on the ground can be found. Refer to the Fig. For better understanding and simplification.
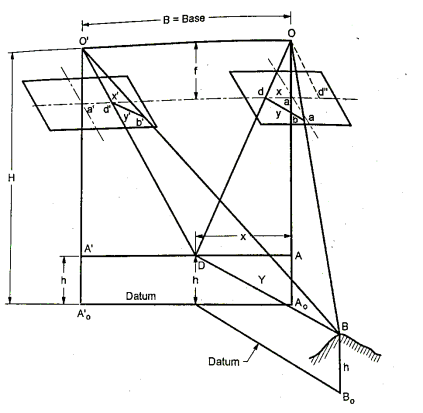
Fig: Determination of co-ordinates and elevation of ground points by parallax measurements
- Consider B point on the ground whose co-ordinates and elevation to be found out.
- Consider A which is ground position of the principal point 'a' of the right photograph.
- Let X and Y are the ground co-ordinates of B with respect to the co-ordinate’s axes of the ground. These co-ordinates are parallel to the photograph axes x and y with 'A' as origin.
- Let D is an imaginary point with same elevation as that of B and lies on the ground x-axis consider b and d are the images of B and D on right photograph.
From 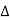 OAD and Oad,
OAD and Oad,
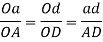
From Fig.: Oa= f and OA=H-h
∴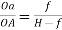
∴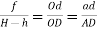
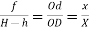 … (1) ∵ad=x and AD=X
… (1) ∵ad=x and AD=X
Similarly,
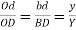 … (2) ∵bd=y and BD=Y
… (2) ∵bd=y and BD=Y
Substituting Equations (1) and (2) we get
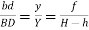 … (3)
… (3)
Similarly, from triangle O'A'D and O'a'd
∴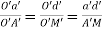
Or 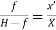 … (4)
… (4)
From triangle O'D B and O'd'a
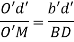
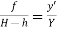 …. (5)
…. (5)
Considering Equation (3) and (5), we get
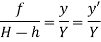
∴y=y’ … (6)
Equation (6) shows that there is no parallax in a stereoscopic pair of photographs.
In the right photograph Od" is drawn parallel to O'd' of left photograph.
Hence considering triangle Od"d and ODO', we get
Oo' is parallel to d"d
Od is parallel to OD and coincides Od" is parallel to O'd
It is observed from the above similarity that their corresponding altitudes are fand (H-h) respectively.
In this way:
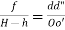 … (7)
… (7)
But dd” = ad + ad" = x-x' = P
And 00’ = b= air base
Hence Equation (7) can be written as follows;
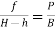 … (8)
… (8)
∴H-h=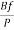 …(9)
…(9)
Equation (8) is the parallax equation for the elevation of the point 'B'.
Considering Equation (1) and (9), we get;
X=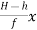
And Y=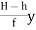
From equation (8) we have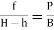
∴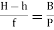
∴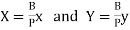 … (10)
… (10)
The equation (10) is the parallax equation for the ground co-ordinates of the point ‘B’.
Q8) What is Digital Photogrammetry?
A8)
- The digital or soft extension of the conventional hard copy photogrammetry is called as digital photogrammetry.
- In case of digital photogrammetry, there is use of digital images without using the conventional photographic images. Digital images can be obtained by using the satellite images or by scanning aerial photos of high resolution.
- In general, digital photogrammetric is the art of using computers so as to obtain the measurements of objects in a photograph. It consists of analyzing one or more existing photographs or videos with photogrammetric software to determine spatial relationships.
- Digital photogrammetry is commonly used to create or produce the topographical maps. It can also be used in the area like architecture, manufacturing, police investigations and plastic surgery.
- There are several variables involved. In digital photogrammetry. These variables can be useful for a photogrammetrist to know the information about camera such as type of camera, the focal length of the lens, the distance of the camera to the object of interest.
- Metric camera is a special type of camera which is often used to take these type of photographs Metric camera is calibrated so well that it gives precision photographs with little lens distortion.
- Another variable in digital photogrammetry to be considered is a desired dimensions of the measurements.
- Some of the projects require only 2D i.e., the dimensional measurements such as the height of a building or width of a river and these measurements can be taken from a single photograph. For 3D i.e., three-dimension measurements, the process involves 3D models from two or more photographs.
- One more variable which to be considered in digital photogrammetry is the type of photographs used. Photogrammetrists usually use either still digital photographs or video. In such case, film photographs and video tape should be scanned into a computer in order to use in digital photogrammetry.
- There are two main categories of photographs
- Aerial photographs
- Close-range photographs
- Aerial photogrammetry often used in topographical mapping begins with digital photographs or video taken from a camera mounted on the bottom of an airplane.
Q9) What are the Advantages of Digital Photogrammetry?
A9)
Following are the various advantages:
- It is used in digital terrain modeling.
- It gives appropriate display and measurements.
- It is used for two-dimensional and three-dimensional plain mapping.
- It can also be used for stream mapping.
- It can produce superior quality plainmetric and topographic data.
- It can also be used for highway mapping and data compilation.
Q10) The scale of aerial photograph is 1: 25000, effective at an average elevation of terrain of 335 m. The size of aerial photograph is 230 mm x 230 mm. Focal length of camera lens is 200 mm. Speed of aircraft is 270 km/h, longitudinal overlap is 65% and side overlap is 28%. Determine the number of photographs required to cover an area of 150 km x 105 km.
A10)
Step 1: To find the number of photographs required to cover an area of 150 km x 105 km
L₁ = 150 km = 150 x 1000 150000 m
P₁=longitudinal overlap = 65 %=65 /100 = =0.65
Scale of photographs = 1:25000
Flight map is on a scale of 1:25000 or 1 cm = 250 m
∴S=250
l= length of photographs in the direction of flight= 230 mm = 23 cm
∴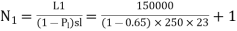
∴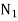 =74.53+1=7.53=76
=74.53+1=7.53=76
L2=105Km=105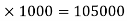
Now Pw = side overlap = 28% = 28/100=0.28
w = width of photograph normal to the direction of = 230 mm= 23 cm

=25.3+1=26.3=23cm
Number of photographs = N₁ x N₂=76×26
∴Number of photographs = 1976
Q11) A line AB 2000 m long, lying at an elevation of 500 m measures 8.65 cm on a vertical photography for which focal length is 20 cm. Determine the scale of the photograph in an area the average elevation of which is about 800 m.
A11)
Given: Map distance = 8.65 cm, Ground distance = 2000 m, f = focal length = 20 cm, h = Elevation = 500 m, Average elevation = 800 m
To find the flying height (H):
S= Scale= Map distance/Ground distance = 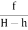
∴
H-500 = 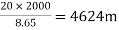
∴H-500 == 4624 m
H = 4624+500=5124 m
To determine the scale of photograph at an average elevation of 800 m:
S800 = Scale of photograph at average elevation of 800 m
∴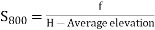
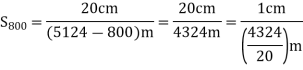
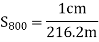
Hence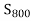 is 1cm=216.2 m
is 1cm=216.2 m
Q12) A camera having focal length of 200 mm is to be used to take a vertical photograph of a terrain having an average elevation of 2000 m. At what height above datum, the air craft should fly to have a photograph at a scale of 1:5000?
A12)
Step 1:
Given: Focal length of camera = f = 200 mm
Average elevation of a terrain = 2000 m
Scale of photograph = 1:5000
Step 2: To find flying height:
Scale of photograph=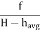
∴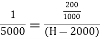
∴(H-2000) =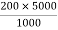
∴H=flying height=3000m