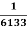UNIT 1
Introduction
Q1: Find the critical water depth for a specific energy head of E1 = 1.5 m in the following channels:
a) Rectangular channel, B = 2.0 m.
b) Triangular channel, m = 1.5.
c) Trapezoidal channel, B = 2.0 m and m = 1.0.
Solution:
a) Rectangular channel
Ec =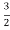 yc = 1.5m
yc = 1.5m
Yc = 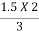 = 1.00 m
= 1.00 m
b) Triangular channel
Ec =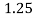 yc = 1.5m
yc = 1.5m
Yc =  = 1.20 m
= 1.20 m
c) Trapezoidal channel
Ec = yc +
yc + 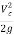
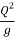 =
= 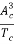
Ec = yc +
yc + 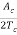

Yc = 1.10 m (By trial and error method)
Q2: A rectangular channel has a width of 2.0 m and carries a discharge of 4.80 m3 /sec with a depth of 1.60 m. At a certain cross-section a small, smooth hump with a flat top and a height 0.10 m is proposed to be built. Calculate the likely change in the water surface. Neglect the energy loss.
Solution: Let the suffixes 1 and 2 refer to the upstream and downstream sections respectively.
q = 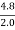 = 2.4 m3/sec/m
= 2.4 m3/sec/m
V1 = 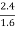 = 1.5 m/sec
= 1.5 m/sec
 =
= 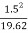 =0.115 m
=0.115 m
Fr1 = 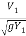 =
= 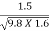 =0.38
=0.38
The upstream flow is subcritical and the hump will cause a drop in the water surface elevation. The specific energy at section 1 is,
E1 = 1.6 + 0.115 =1.715 m
At section 2
E2 = E1 - 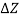 = 1.715 – 0.10 = 1.615 m
= 1.715 – 0.10 = 1.615 m
Yc = 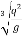 =
= 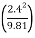 1/3 = 0.837 m
1/3 = 0.837 m
Ec = 1.5 Yc = 1.5 X 0.837 = 1.26 m
The minimum specific energy at section 2 is Ec2 = 1.26 m < E2 = 1.615 m. Hence y2 > yc and the upstream depth y1 will remain unchanged. The depth y2 is calculated by solving the specific energy equation,
E2 = y2 +
y2 + 
1.615 = y2 + 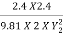
Solving by trial and error gives, Y2 = 1.48 m
The drop at water surface elevation is,
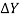 = 1.60 – 1.48 - 0.10 = 0.02 m
= 1.60 – 1.48 - 0.10 = 0.02 m
Q3: A long rectangular channel of width 5 m has a slope of 1:5000 and a Manning’s roughness coefficient 𝑛 of 0.02 m–1/3 s. The total discharge is 10 m3 s –1. The channel narrows to a width of 1.2 m over a short length.
(a) Determine the normal depth at this flow rate in the 5 m-wide channel.
(b) Show that critical conditions occur at the narrow section.
(c) Determine the depth just upstream of the narrowed section, where the width is 5 m.
(d) Determine the distance upstream to where the depth is 5% greater than the normal depth, using two steps in the gradually-varied-flow equation.
Solution:
Given: b = 5 m (bmin = 1.2 m)
So = 0.0002
n = 0.02 m-1/3Sec
Q = 10 m3/sec
For the normal depth,
Q = VA
Where, V =  Rh2/3 S1/2
Rh2/3 S1/2
Rh = 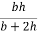 =
= 
A = bh
Q = b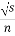 X
X 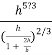
Rearranging as an iterative formula for ℎ to find the normal depth at the channel slope So:
h = (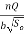 )3/5 X
)3/5 X 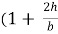 )2/5
)2/5
Here, with lengths in meters:
h = 1.866 (1 + 0.4h)2/5
Iteration (from, e.g., h = 1.866) gives normal depth:
hn = 2.453 m
Q4: Find the velocity of flow and rate of flow of water through a rectangular channel of 6 m wide and 3 m deep, when it is running full. The channel is having bed slope as 14 in 2000. Take Chezy’s constant C = 55.
Solution: Given:
Width of rectangular channel, b = 6 m
Depth of channel, d = 3 m
Bed slope, I = 1 in 2000 = 
Chezy’s constant, C = 55
Perimeter, P = b + 2d = 6 + 2 X 3 = 12 m
: Hydraulic mean depth, m =  =
= 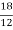 = 1.5 m
= 1.5 m
Velocity of flow is given by equation
V = C 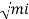 = 55
= 55 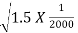 = 1.506 m/s.
= 1.506 m/s.
Rate of flow, Q = V X A = 1.506 X 18 = 27.108 m3/s.
Q5: Find the discharge through a rectangular channel of width 2 m, having a bed slope of 4 in 8000. The depth of flow is 1.5 m and takes the value of N in Manning’s formula as 0.012.
Solution:
Given
Width of channel, b = 2 m
Depth of flow, d = 1.5 m
Area of flow, A = b X d = 2 X 1.5 = 3.0 m2
Wetted perimeter, P = b + d + d = 2 + 1.5 + 1.5 =5.0 m
Hydraulic mean depth, = m =  =
=  = 0.6
= 0.6
Bed Slope, i = 4 in 8000 = 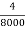 =
= 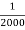
Value of N
Using Manning’s formula, given by equation
C =  m1/6 =
m1/6 = 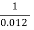 X 0.61/6 = 76.54
X 0.61/6 = 76.54
Discharge, Q is given by equation
Q = AC 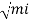 = 3.0 X 76.54 X
= 3.0 X 76.54 X 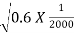
=3.977m3/s
Q6: Determine the maximum discharge of water through a circular channel of diameter1.5 m when the bed slope of the channel is 1 in 1000. Take C = 60.
Solution:
Diameter of channel, D = 1.5 m
R = 1.5/2 = 0.75 m
Bed slope, i = 1/2000
C = 60
Foe maximum discharge, 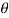 = 154
= 154 or
or 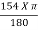 = 206878 radians
= 206878 radians
Wetted perimeter for a circular channel is given by
P = 2R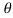 = 2 X D/2 X 2.6878
= 2 X D/2 X 2.6878
= 2 X 1.5/2 X 2.6878 = 4.0317 m
Wetted area A is given by equation
A = R2 (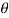 - sin2
- sin2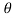 /2)
/2)
= 0.752 (2.6878 – Sin (2X 154 )/2)
)/2)
= 1.7335
Hydraulic mean depth, m =  =
= 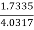 = 04229
= 04229
Maximum discharge if given by Q = AC 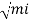 = 1.7335 X 60 X
= 1.7335 X 60 X 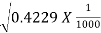
Q = 2.1565 m3/s
Q7: The specific energy for a 3m wide channel is to be 3 kg-m/kg. What would be the maximum possible discharge?
Solution:
Width of channel b = 3 m
Specific energy, E = 3 kg-m/kg =3 m
For the given value of specific energy, the discharge will be maximum, when the depth of flow is critical. Hence from equation of maximum discharge,
hc= h =  E =
E =  X 3.0 = 2.0 m
X 3.0 = 2.0 m
Maximum discharge, Qmax is given by
Qmax = Area X Velocity = b X (depth of flow) X velocity
= (b X hc) X Vc (At critical depth, velocity will be critical
Where, Vc is critical velocity and it is given by equation
Vc = 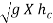 =
= 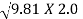 = 4.4249m/s
= 4.4249m/s
Qmax = (b X hc) X Vc = (3 X 2) X 4.4249
= 26.576 m3/s.
Q8: The specific energy for a 5 m wide rectangular channel is to be 4 N-m/N. If the rate of flow of water through the channel is 20m3/s, determine the alternate depths of flow.
Solution:
Width of channel, b = 5m
Specific energy, E = 4 N-m/N = 4 m
Discharge, Q = 20 m3/s
The specific energy (E) is given by
E = h + 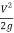
Where, V = 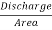 =
= 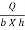 =
= 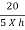 =
= 
Specific energy, E = h + 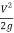 = h + (
= h + ( ) 2 X
) 2 X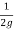
= h + 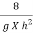
But, E = 4.0
Equating two values of E = 4 = h + 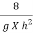
h3 – 4h2 + 0.1855 = 0
By trial and error method
h = 3.93m and 0.48m
Q9: A flow of water of 1000 litres per second flows down in a rectangular flume of width 600 mm and having adjustable bottom slope. If Chezy’s constant C is 56, find the bottom slope necessary for uniform flow with a depth of flow of 300 mm.
Solution:
Discharge, Q = 100 litres/s =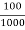 =0.10 m3/s
=0.10 m3/s
Width of channel, b = 600 mm = 0.60 m
Depth of flow, d = 300 mm =0.30 m
Area of flow, A = b X d = 0.6 X 0.3 = 0.18m2
Chezy’s constant, C = 56
Let the bed slope of bed = i
Hydraulic mean depth, m = 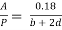 =
= 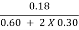 =
= = 0.15 m
= 0.15 m
Using equation, we have Q = AC 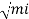
0.10 = 0.18 X 56 X 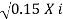
Squaring both sides, we have 0.15i = ( 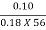 ) 2 = 0.000098418
) 2 = 0.000098418
i = 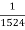
So, slope of bed is 1 in 1524.
Q10: Find the bed slope of trapezoidal channel of bed width 6 m, depth of water 3 m and side slope of 3 horizontal to 4 vertical, when the discharge through the channel is 30m3/s. Take Chezy’s constant, C = 70.
Solution:
Bed width, b = 6.0 m
Depth of flow, d = 3.0 m
Side slope = 3 horizontal to 4 vertical
Discharge, Q = 30 m3/s
Chezy’s constant, C = 70
Distance, BE = 3 x ¾ = 2.25 m
Top width = 6 + 2 X 2.25 = 10.50 m
Wetted perimeter, P = Sum of all the sides
= 6 + 2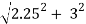 = 13.5 m
= 13.5 m
Area of flow, A = Area of trapezoid ABCD
= 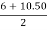 X 3.0 = 24.75 m2
X 3.0 = 24.75 m2
Hydraulic mean depth, m = =
= 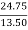 = 1.833
= 1.833
Using Equation, Q = AC 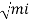
30.0 = 24.75 X 70 X 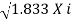
i = 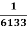
UNIT 1
Introduction
Q1: Find the critical water depth for a specific energy head of E1 = 1.5 m in the following channels:
a) Rectangular channel, B = 2.0 m.
b) Triangular channel, m = 1.5.
c) Trapezoidal channel, B = 2.0 m and m = 1.0.
Solution:
a) Rectangular channel
Ec =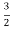 yc = 1.5m
yc = 1.5m
Yc = 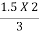 = 1.00 m
= 1.00 m
b) Triangular channel
Ec =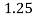 yc = 1.5m
yc = 1.5m
Yc = 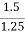 = 1.20 m
= 1.20 m
c) Trapezoidal channel
Ec = yc +
yc + 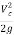
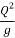 =
= 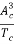
Ec = yc +
yc + 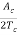

Yc = 1.10 m (By trial and error method)
Q2: A rectangular channel has a width of 2.0 m and carries a discharge of 4.80 m3 /sec with a depth of 1.60 m. At a certain cross-section a small, smooth hump with a flat top and a height 0.10 m is proposed to be built. Calculate the likely change in the water surface. Neglect the energy loss.
Solution: Let the suffixes 1 and 2 refer to the upstream and downstream sections respectively.
q = 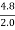 = 2.4 m3/sec/m
= 2.4 m3/sec/m
V1 = 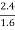 = 1.5 m/sec
= 1.5 m/sec
 =
= 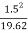 =0.115 m
=0.115 m
Fr1 = 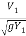 =
= 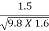 =0.38
=0.38
The upstream flow is subcritical and the hump will cause a drop in the water surface elevation. The specific energy at section 1 is,
E1 = 1.6 + 0.115 =1.715 m
At section 2
E2 = E1 - 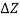 = 1.715 – 0.10 = 1.615 m
= 1.715 – 0.10 = 1.615 m
Yc = 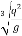 =
= 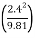 1/3 = 0.837 m
1/3 = 0.837 m
Ec = 1.5 Yc = 1.5 X 0.837 = 1.26 m
The minimum specific energy at section 2 is Ec2 = 1.26 m < E2 = 1.615 m. Hence y2 > yc and the upstream depth y1 will remain unchanged. The depth y2 is calculated by solving the specific energy equation,
E2 = y2 +
y2 + 
1.615 = y2 + 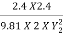
Solving by trial and error gives, Y2 = 1.48 m
The drop at water surface elevation is,
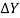 = 1.60 – 1.48 - 0.10 = 0.02 m
= 1.60 – 1.48 - 0.10 = 0.02 m
Q3: A long rectangular channel of width 5 m has a slope of 1:5000 and a Manning’s roughness coefficient 𝑛 of 0.02 m–1/3 s. The total discharge is 10 m3 s –1. The channel narrows to a width of 1.2 m over a short length.
(a) Determine the normal depth at this flow rate in the 5 m-wide channel.
(b) Show that critical conditions occur at the narrow section.
(c) Determine the depth just upstream of the narrowed section, where the width is 5 m.
(d) Determine the distance upstream to where the depth is 5% greater than the normal depth, using two steps in the gradually-varied-flow equation.
Solution:
Given: b = 5 m (bmin = 1.2 m)
So = 0.0002
n = 0.02 m-1/3Sec
Q = 10 m3/sec
For the normal depth,
Q = VA
Where, V =  Rh2/3 S1/2
Rh2/3 S1/2
Rh = 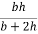 =
= 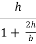
A = bh
Q = b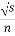 X
X 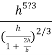
Rearranging as an iterative formula for ℎ to find the normal depth at the channel slope So:
h = (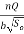 )3/5 X
)3/5 X 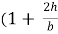 )2/5
)2/5
Here, with lengths in meters:
h = 1.866 (1 + 0.4h)2/5
Iteration (from, e.g., h = 1.866) gives normal depth:
hn = 2.453 m
Q4: Find the velocity of flow and rate of flow of water through a rectangular channel of 6 m wide and 3 m deep, when it is running full. The channel is having bed slope as 14 in 2000. Take Chezy’s constant C = 55.
Solution: Given:
Width of rectangular channel, b = 6 m
Depth of channel, d = 3 m
Bed slope, I = 1 in 2000 = 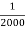
Chezy’s constant, C = 55
Perimeter, P = b + 2d = 6 + 2 X 3 = 12 m
: Hydraulic mean depth, m =  =
= 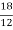 = 1.5 m
= 1.5 m
Velocity of flow is given by equation
V = C 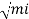 = 55
= 55 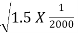 = 1.506 m/s.
= 1.506 m/s.
Rate of flow, Q = V X A = 1.506 X 18 = 27.108 m3/s.
Q5: Find the discharge through a rectangular channel of width 2 m, having a bed slope of 4 in 8000. The depth of flow is 1.5 m and takes the value of N in Manning’s formula as 0.012.
Solution:
Given
Width of channel, b = 2 m
Depth of flow, d = 1.5 m
Area of flow, A = b X d = 2 X 1.5 = 3.0 m2
Wetted perimeter, P = b + d + d = 2 + 1.5 + 1.5 =5.0 m
Hydraulic mean depth, = m =  =
=  = 0.6
= 0.6
Bed Slope, i = 4 in 8000 = 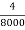 =
= 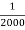
Value of N
Using Manning’s formula, given by equation
C =  m1/6 =
m1/6 = 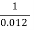 X 0.61/6 = 76.54
X 0.61/6 = 76.54
Discharge, Q is given by equation
Q = AC 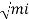 = 3.0 X 76.54 X
= 3.0 X 76.54 X 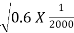
=3.977m3/s
Q6: Determine the maximum discharge of water through a circular channel of diameter1.5 m when the bed slope of the channel is 1 in 1000. Take C = 60.
Solution:
Diameter of channel, D = 1.5 m
R = 1.5/2 = 0.75 m
Bed slope, i = 1/2000
C = 60
Foe maximum discharge, 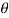 = 154
= 154 or
or 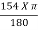 = 206878 radians
= 206878 radians
Wetted perimeter for a circular channel is given by
P = 2R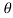 = 2 X D/2 X 2.6878
= 2 X D/2 X 2.6878
= 2 X 1.5/2 X 2.6878 = 4.0317 m
Wetted area A is given by equation
A = R2 ( - sin2
- sin2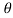 /2)
/2)
= 0.752 (2.6878 – Sin (2X 154 )/2)
)/2)
= 1.7335
Hydraulic mean depth, m =  =
= 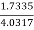 = 04229
= 04229
Maximum discharge if given by Q = AC 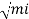 = 1.7335 X 60 X
= 1.7335 X 60 X 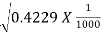
Q = 2.1565 m3/s
Q7: The specific energy for a 3m wide channel is to be 3 kg-m/kg. What would be the maximum possible discharge?
Solution:
Width of channel b = 3 m
Specific energy, E = 3 kg-m/kg =3 m
For the given value of specific energy, the discharge will be maximum, when the depth of flow is critical. Hence from equation of maximum discharge,
hc= h =  E =
E =  X 3.0 = 2.0 m
X 3.0 = 2.0 m
Maximum discharge, Qmax is given by
Qmax = Area X Velocity = b X (depth of flow) X velocity
= (b X hc) X Vc (At critical depth, velocity will be critical
Where, Vc is critical velocity and it is given by equation
Vc = 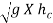 =
= 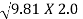 = 4.4249m/s
= 4.4249m/s
Qmax = (b X hc) X Vc = (3 X 2) X 4.4249
= 26.576 m3/s.
Q8: The specific energy for a 5 m wide rectangular channel is to be 4 N-m/N. If the rate of flow of water through the channel is 20m3/s, determine the alternate depths of flow.
Solution:
Width of channel, b = 5m
Specific energy, E = 4 N-m/N = 4 m
Discharge, Q = 20 m3/s
The specific energy (E) is given by
E = h + 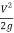
Where, V = 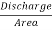 =
= 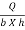 =
= 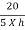 =
= 
Specific energy, E = h + 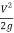 = h + (
= h + ( ) 2 X
) 2 X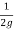
= h + 
But, E = 4.0
Equating two values of E = 4 = h + 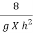
h3 – 4h2 + 0.1855 = 0
By trial and error method
h = 3.93m and 0.48m
Q9: A flow of water of 1000 litres per second flows down in a rectangular flume of width 600 mm and having adjustable bottom slope. If Chezy’s constant C is 56, find the bottom slope necessary for uniform flow with a depth of flow of 300 mm.
Solution:
Discharge, Q = 100 litres/s = =0.10 m3/s
=0.10 m3/s
Width of channel, b = 600 mm = 0.60 m
Depth of flow, d = 300 mm =0.30 m
Area of flow, A = b X d = 0.6 X 0.3 = 0.18m2
Chezy’s constant, C = 56
Let the bed slope of bed = i
Hydraulic mean depth, m = 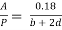 =
= 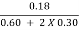 =
=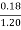 = 0.15 m
= 0.15 m
Using equation, we have Q = AC 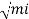
0.10 = 0.18 X 56 X 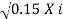
Squaring both sides, we have 0.15i = ( 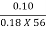 ) 2 = 0.000098418
) 2 = 0.000098418
i = 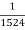
So, slope of bed is 1 in 1524.
Q10: Find the bed slope of trapezoidal channel of bed width 6 m, depth of water 3 m and side slope of 3 horizontal to 4 vertical, when the discharge through the channel is 30m3/s. Take Chezy’s constant, C = 70.
Solution:
Bed width, b = 6.0 m
Depth of flow, d = 3.0 m
Side slope = 3 horizontal to 4 vertical
Discharge, Q = 30 m3/s
Chezy’s constant, C = 70
Distance, BE = 3 x ¾ = 2.25 m
Top width = 6 + 2 X 2.25 = 10.50 m
Wetted perimeter, P = Sum of all the sides
= 6 + 2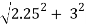 = 13.5 m
= 13.5 m
Area of flow, A = Area of trapezoid ABCD
= 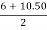 X 3.0 = 24.75 m2
X 3.0 = 24.75 m2
Hydraulic mean depth, m = =
= 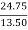 = 1.833
= 1.833
Using Equation, Q = AC 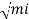
30.0 = 24.75 X 70 X 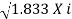
i =