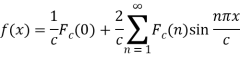Module-2
Integral Transform
Question-1: Using complex form, find the Fourier series of the function
f(x) = sinx = 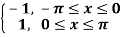
Sol.
We calculate the coefficients 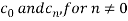
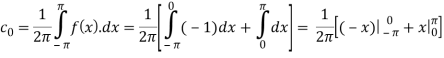
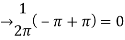
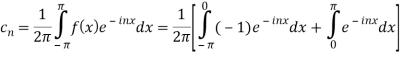
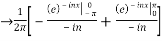 =
= 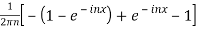
=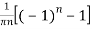
Hence the Fourier series of the function in complex form is
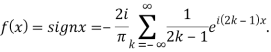
We can transform the series and write it in the real form by renaming it as
n=2k-1,n=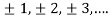
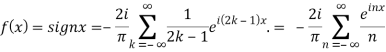
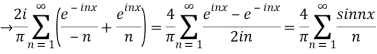
= 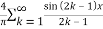
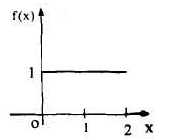
Question-2: Find the Fourier integral representation of the function
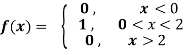
Sol.
The graph of the function is shown in the below figure satisfies the hypothesis of
Theorem -1. Hence from Eqn,(5) and (6), we have
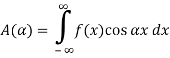
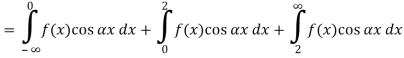
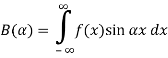
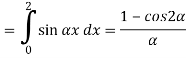
Substituting these coefficients in Eqn. (4) we obtain
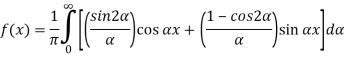
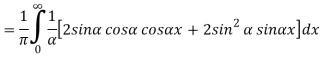
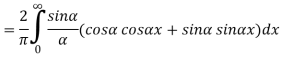
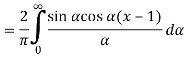
This is the Fourier integral representation of the given function.
Question-3: Find the Fourier integral representation of the function
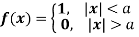
Sol.
The graph of the given function is shown in the below figure. Clearly, the given function f(x) is an even function. We represent f(x) by the Fourier cosine integral. We obtain
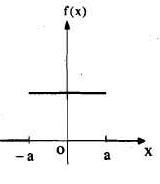
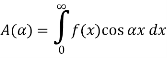

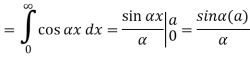
And thus,
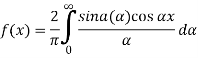
Question-4: Find the half –range sine series of the function
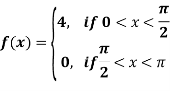
Sol. xx
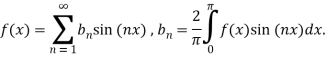
Where
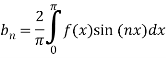
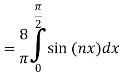
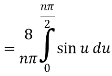
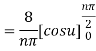
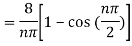
Question-5: Find the Fourier transform of-
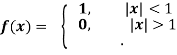
Hence evaluate 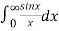
Sol. As we know that the Fourier transform of f(x) will be-

So that-
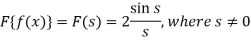
For s = 0, we get- F(s) = 2
Hence by the inverse formula, we get-

Putting x = 0, we get
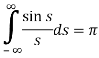
So-
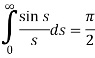
Question-6: Find the Fourier transform of 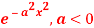
Sol. As we know that the Fourier transform of f(x) will be-
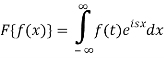
So that-
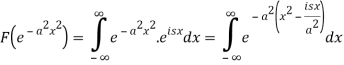
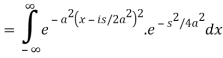
Now put 
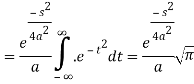
So that-
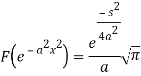
Question-7: Find the Fourier sine transform of 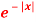
Sol. Here x being positive in the interval (0, ∞)
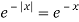
Fourier sine transform of  will be-
will be-
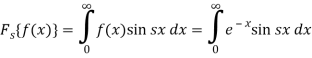
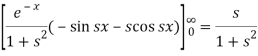
Question-8: Find the Fourier sine transform of 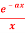
Sol. Let 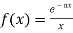
Then the Fourier sine transform will be-
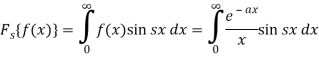
Now suppose,

Differentiate both sides with respect to x, we get-
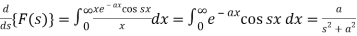 ……. (1)
……. (1)
On integrating (1), we get-
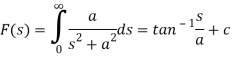
Question-9: Solve the equation-
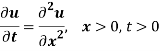
Subject to the conditions-
- u = 0 when x = 0, t>0
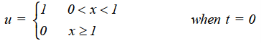
- U(x,t) is bounded.
Sol.
Here we apply Fourier sine transform-
We get-
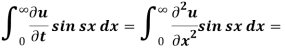
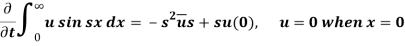
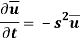
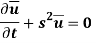
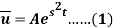
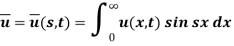
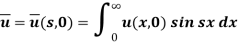
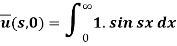
 ...... (2)
...... (2)
Put the value of 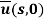 in equation (1), we get
in equation (1), we get
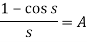
So that-
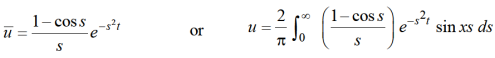
Question-10: Find Z-transform of the following functions-
(i) 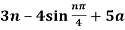
(ii) 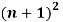
Sol. (i) 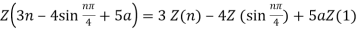
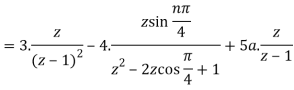
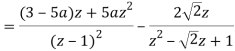
(ii) 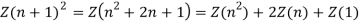
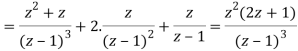
Question-11: Find the z-transformation of the following left-sided sequence
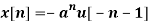
Sol.
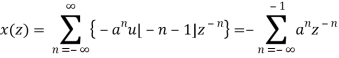
= 
= 1- 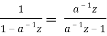
= 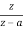
If 
Question-12: Solve the differential equation  by the z-transformation method.
by the z-transformation method.
Sol.
Given,
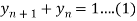
Let y(z) be the z-transform of 
Taking z-transforms of both sides of eq(1) we get,
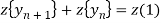
Ie. 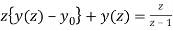
Using the given condition, it reduces to
(z+1)y(z) = 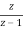
Ie.
Y(z) = 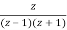
Or Y(Z) = 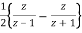
On taking inverse Z-transforms, we obtain
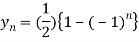
Question-13: Solve the following by using Z-transform
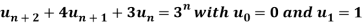
Sol. If  then
then 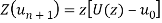
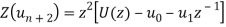
And
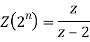
Now taking the Z-transform of both sides, we get
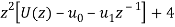 z[
z[
It becomes-
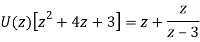
So,
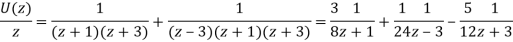
Now-
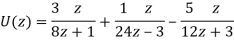
On inversion, we get-
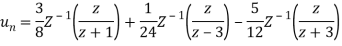

Question-14: Define finite Fourier sine and cosine transforms.
Sol.
Finite Fourier sine and cosine transforms-
The finite Fourier sine transform of f(x), in 0<x<c, is given by-
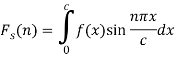
Where n is an integer.
And the function f(x) is called the inverse finite Fourier sine transform of  which is defined by-
which is defined by-
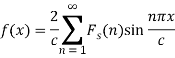
The finite Fourier cosine transform of f(x), in 0<x<c, is given by-
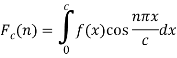
Where n is an integer.
And the function f(x) is called the inverse finite Fourier cosine transform of 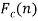 which is defined by-
which is defined by-
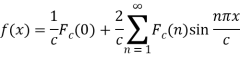
Module-2
Module-2
Module-2
Integral Transform
Question-1: Using complex form, find the Fourier series of the function
f(x) = sinx = 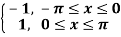
Sol.
We calculate the coefficients 
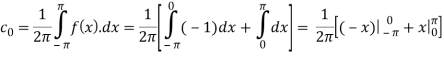
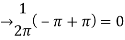

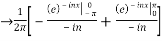 =
= 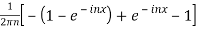
=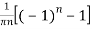
Hence the Fourier series of the function in complex form is
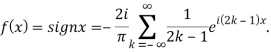
We can transform the series and write it in the real form by renaming it as
n=2k-1,n=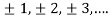
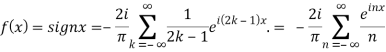
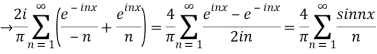
= 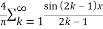
Question-2: Find the Fourier integral representation of the function
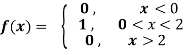
Sol.
The graph of the function is shown in the below figure satisfies the hypothesis of
Theorem -1. Hence from Eqn,(5) and (6), we have
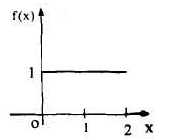
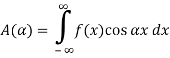
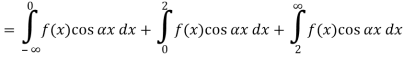

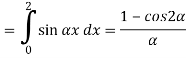
Substituting these coefficients in Eqn. (4) we obtain
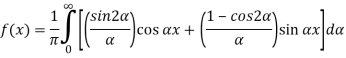
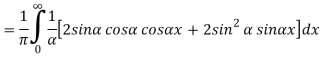
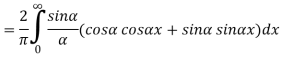
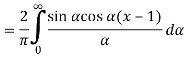
This is the Fourier integral representation of the given function.
Question-3: Find the Fourier integral representation of the function
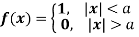
Sol.
The graph of the given function is shown in the below figure. Clearly, the given function f(x) is an even function. We represent f(x) by the Fourier cosine integral. We obtain
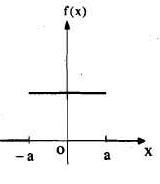
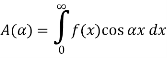
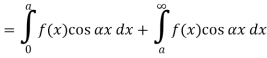
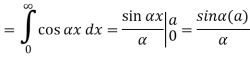
And thus,
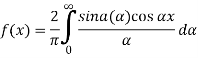
Question-4: Find the half –range sine series of the function
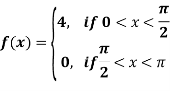
Sol. xx
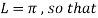
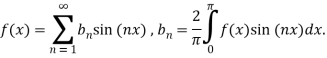
Where
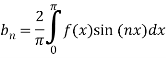

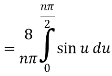

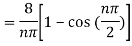
Question-5: Find the Fourier transform of-

Hence evaluate 
Sol. As we know that the Fourier transform of f(x) will be-

So that-
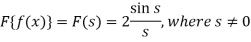
For s = 0, we get- F(s) = 2
Hence by the inverse formula, we get-
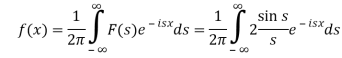
Putting x = 0, we get
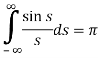
So-
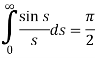
Question-6: Find the Fourier transform of 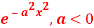
Sol. As we know that the Fourier transform of f(x) will be-

So that-
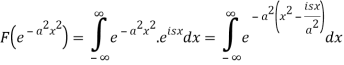
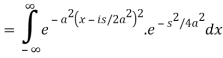
Now put 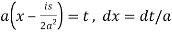

So that-
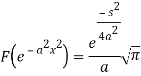
Question-7: Find the Fourier sine transform of 
Sol. Here x being positive in the interval (0, ∞)
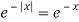
Fourier sine transform of 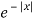 will be-
will be-
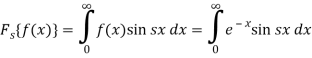
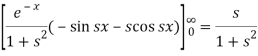
Question-8: Find the Fourier sine transform of 
Sol. Let 
Then the Fourier sine transform will be-
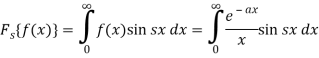
Now suppose,
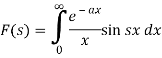
Differentiate both sides with respect to x, we get-
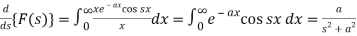 ……. (1)
……. (1)
On integrating (1), we get-
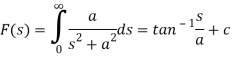
Question-9: Solve the equation-
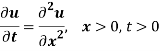
Subject to the conditions-
- u = 0 when x = 0, t>0
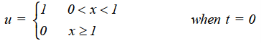
- U(x,t) is bounded.
Sol.
Here we apply Fourier sine transform-
We get-
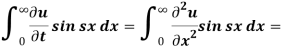

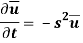
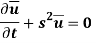
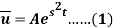
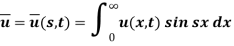
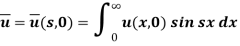
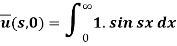
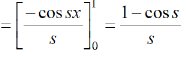 ...... (2)
...... (2)
Put the value of 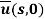 in equation (1), we get
in equation (1), we get
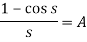
So that-
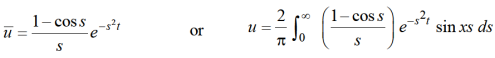
Question-10: Find Z-transform of the following functions-
(i) 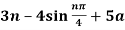
(ii) 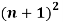
Sol. (i) 
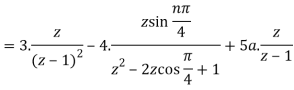

(ii) 
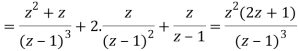
Question-11: Find the z-transformation of the following left-sided sequence
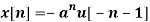
Sol.
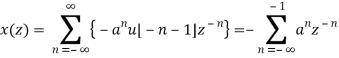
= 
= 1- 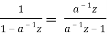
= 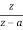
If 
Question-12: Solve the differential equation  by the z-transformation method.
by the z-transformation method.
Sol.
Given,
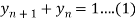
Let y(z) be the z-transform of 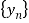
Taking z-transforms of both sides of eq(1) we get,
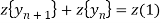
Ie. 
Using the given condition, it reduces to
(z+1)y(z) = 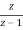
Ie.
Y(z) = 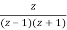
Or Y(Z) = 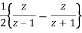
On taking inverse Z-transforms, we obtain
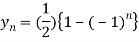
Question-13: Solve the following by using Z-transform
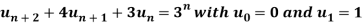
Sol. If  then
then 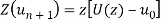
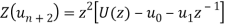
And
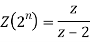
Now taking the Z-transform of both sides, we get
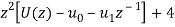 z[
z[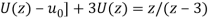
It becomes-
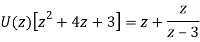
So,
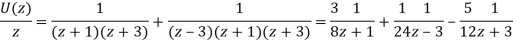
Now-

On inversion, we get-
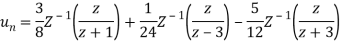
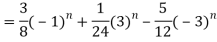
Question-14: Define finite Fourier sine and cosine transforms.
Sol.
Finite Fourier sine and cosine transforms-
The finite Fourier sine transform of f(x), in 0<x<c, is given by-
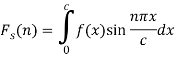
Where n is an integer.
And the function f(x) is called the inverse finite Fourier sine transform of 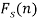 which is defined by-
which is defined by-
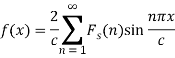
The finite Fourier cosine transform of f(x), in 0<x<c, is given by-
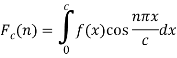
Where n is an integer.
And the function f(x) is called the inverse finite Fourier cosine transform of  which is defined by-
which is defined by-