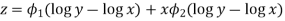Unit - 1

Partial differential equation

Q1) Form a partial differential equation from-

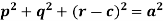
A1)

Here we have-

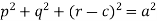
It contains two arbitrary constants a and c

Differentiate the equation with respect to p, we get-

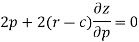
Or

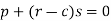
Now differentiate the equation with respect to q, we get-

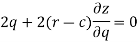
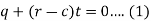
Now eliminate ‘c’,

We get

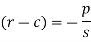
Now put z-c in (1), we get-

Or

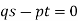
Q2) Solve the differential equation-

Given the boundary condition that-

At x = 0,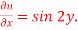

A2)

Here we have-

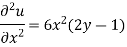
On integrating partially with respect to x, we get-

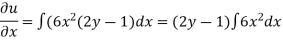
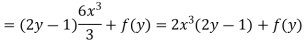
Here f(y) is an arbitrary constant.

Now form the boundary condition-

When x = 0,

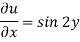
Hence-

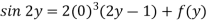
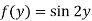
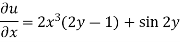
On integrating partially w.r.t.x, we get-

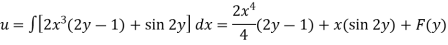
Q3) Solve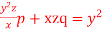

A3)

Rewriting the given equation as

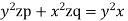
The subsidiary equations are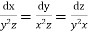

The first two fractions give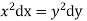

Integrating we get 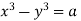   n    (i)

Again the first and third fraction give xdx = zdz

Integrating, we get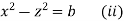

Hence from (i) and (ii), the complete solution is

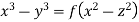
Q4) Solve-

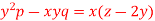
A4)

We have-

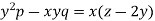
Then the auxiliary equations are-

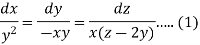
Consider the first two equations only-

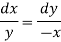
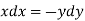
On integrating

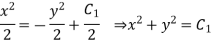
Now consider the last two equations-

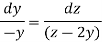
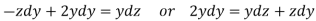
On integrating we get-

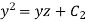
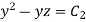…………… (3)

From equation (2) and (3)-

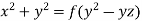
Q5) Solve-

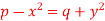
A5)

Let-

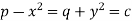
That means-

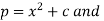
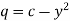
Put these values of p and q in

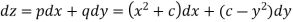
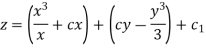
Q6) Solve-

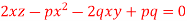
A6)

Let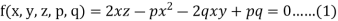
 

Charpit’s subsidiary equations are-

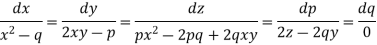
So that- dq = 0 or q = a

On putting q = a in (1) we get-

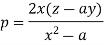
Such that-

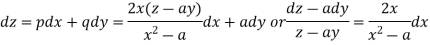
Integrating

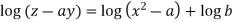
Or

Which is the required solution.

Q7) Find the characteristics of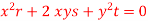

A7)

Here we have-

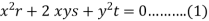
Now comparing (1) with-

Rr + Ss + Tt + f(x, y, z, p, q) = 0

We get-

R =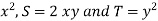

Now we see that-

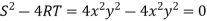
Hence the equation is parabolic.

The 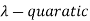is given by-

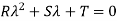
Putting the values of R, S, and T in this, we get-

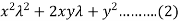
Now simplifying (2), we get-

Solving it we get the repeated roots given by

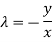
Therefore we get only ne family of the characteristic of (1).

The characteristic equation of (1) is-

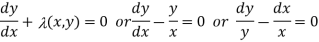
On integrating, we get-

Log y – log x = log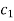

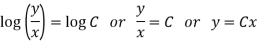
Which is the required family of characteristics and it represents a family of straight lines passing through the origin.

Q8) Solve

A8)

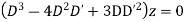
Its auxiliary equation is

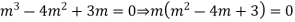
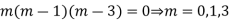
The required solution is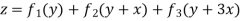

Q9) Solve

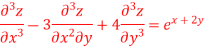
A9)

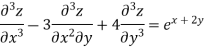
Given equation in symbolic form is

(D3-3D2 D'+4D'3)z=ex+2y

It’s A.E. Is 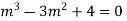where m = -1,2, 2

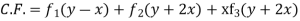
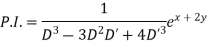
Put, D=1,D’=2 

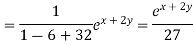
Hence the complete solution is

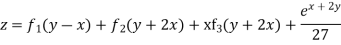
Q10) Solve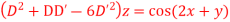

A10)

A.E. Is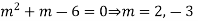

C.F. =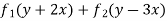

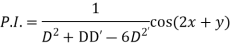
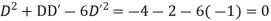
It is a case of failure.

Now,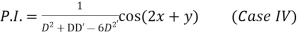

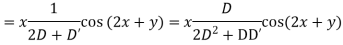
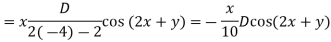
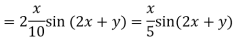
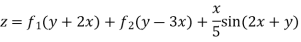
Q11) Solve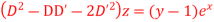

A11)

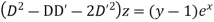
A.E. Is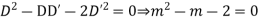

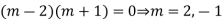
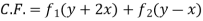
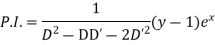
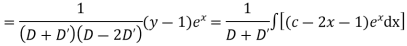
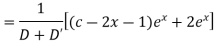
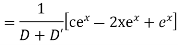
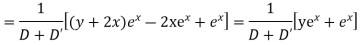
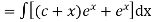
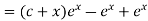
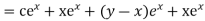
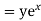
Hence the complete solution is

Q12) Solve

A12)

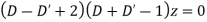
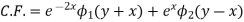
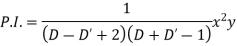
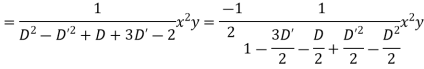
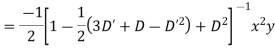
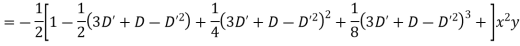
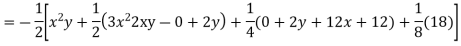
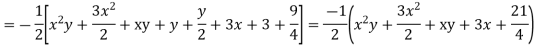
Hence the complete solution is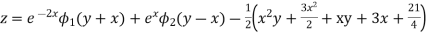

Q13) Solve-

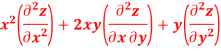
A13)

The above PDE can be written as-

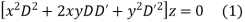
Now put

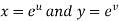
Which gives-

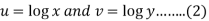
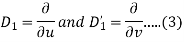
Then the equation (1) can be written as-

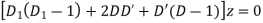
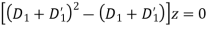
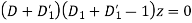
Hence the required solution will be-