Unit – 2
Continuum & Free Molecular Flows
Q1) Define Knudsen No. And state its significance.
A1) The controlling non-dimensional parameter for continuum prediction is the Knudsen number, which is defined as the ratio of the mean free path to the object's characteristic length. In this context, mean free path refers to the average distance travelled by a molecule between two sequential collisions with other molecules.
Significance
- In some cases, the continuum theory is inapplicable. Such conditions exist when the distance between molecules, or, more precisely, the mean free path that they take between collisions with other molecules, is comparable to some physical dimension of the flow channel's container, d (
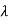 /d -1).
/d -1). - For most of the relations or governing equations, the derived Knudsen number should be close to zero or less than 0.3 for continuum to hold
- The use of governing equations in their standard form remains intact at the first critical value of Knudsen number (0.3), but the nature of boundary conditions changes due to the presence of velocity and temperature slip on the wall.
- This occurs most frequently when the gas density is low (leading to a high mean free path) and the gas interacts with solid surfaces that have a small-scale structure, such as a porous solid or a capillary tube. In such cases, the gas molecules may interact with the solid surface as frequently as they do with other molecules, resulting in a transition regime. As a result, for the Knudsen number range of 0.3 to 1, commonly known as the transitional regime, slip wall boundary conditions should be applied in addition to the conventional governing equations based on the continuum assumption.
- When the density of the gas is reduced further, molecule-to-wall collisions entirely dominate the processes, and the free-molecule or Knudsen regime is reached when
 /d >> 1. The Knudsen Number (Kn) is the ratio
/d >> 1. The Knudsen Number (Kn) is the ratio 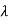 /d. This flow is independent of the gas's viscosity and density, and it is proportional to the third power of the radius of the tube, as opposed to viscous flow, which is proportional to the fourth power.
/d. This flow is independent of the gas's viscosity and density, and it is proportional to the third power of the radius of the tube, as opposed to viscous flow, which is proportional to the fourth power. - The presence of free molecular flow can be guaranteed if the Knudsen number exceeds 1. If the Knudsen number exceeds its second critical value, kinetic theory of gases and related equations are often mandated. This state persists beyond 150 km from the earth's surface, where air density is extremely low.
Q2) Explain types of Flows with Examples.
A2) Steady flow –
- The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
- Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Unsteady flow:
- It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
- E.g.: the flow in a pipe whose value is being opened or closed gradually.
Uniform flow –
- The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
- E.g.: Flow through a straight prismatic conduit.
Non-uniform flow –
- It is that type of flow in which the velocity at any given time changes with respect to space.
- E.g.: Flow through non-prismatic conduit.
Laminar Flow –
- A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
- E.g.: Flow of blood in veins and arteries.
Turbulent Flow –
- A turbulent flow is that flow in which fluid particles move in a zig zag way.
- E.g.: High velocity flows in conduit of large size.
Rotational Flow –
- A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
- E.g.; Motion of liquid in a rotating tank.
Irrotational flow –
- A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.
Compressible Flow –
- It is that type of Flow in Which the density () of the fluid changes from point to point.
- E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
- Mathematically,
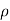 not = to constant
not = to constant
Incompressible Flow –
- It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
- E.g.: subsonic aerodynamics.
One dimensional flow –
- It is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
- E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Two-dimensional Flow –
- The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
- E.g.: Flow between parallel plates of infinite extent.
Three-dimensional flow –
- It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
- E.g.: Flow in a converging or diverging pipe or channel.
Q3) For steady flow ( =0) incompressible fluids (
=0) incompressible fluids ( = constant) the derive the continuity equation in three dimension.
= constant) the derive the continuity equation in three dimension.
A3) Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.
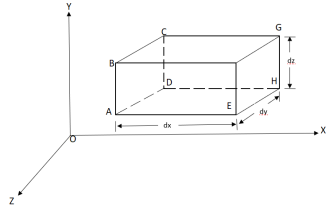
Fig: continuity equation
Let, 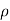 = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 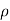 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=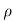 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
=  u dy dz +
u dy dz + 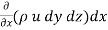
:. Mass accelerated per unit time, due to flow in x-direction
= 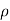 u dy dz – [
u dy dz – [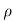 u+
u+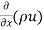 dx] dydz
dx] dydz
= - - (
( u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
= 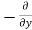 (
(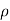 v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
=  (
( w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= - [ (
( u) +
u) +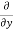 (
(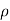 v) +
v) + 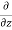 (
(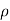 w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
=  (
(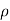 d x, d y d z) …………………... 2
d x, d y d z) …………………... 2
From Equation 1& 2
- [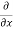 (
(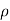 u) +
u) +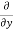 (
(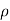 v) +
v) +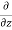 (
(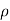 w)] dx dy dz =
w)] dx dy dz = 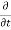 (
(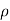 dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
 (
(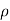 u) +
u) + 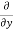 (
(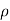 v) +
v) + 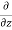 (
( w) +
w) + 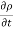 =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow (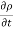 =0) incompressible fluids (
=0) incompressible fluids (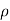 = constant) the equation reduces to
= constant) the equation reduces to
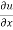 +
+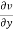 +
+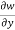 =0
=0
Q4) Define Froude's number and its significance.
A4) Froude's number is defined as the square root of the ratio of inertia force and gravitational force is called Froude's number. It is denoted by F
∴Fr= =
=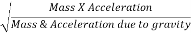 =
=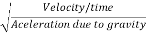
=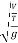 ∵V=
∵V= =T=
=T= =
=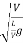 =
=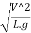 =
=
Significance
- When Froude number is less than one or V>
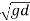 ,the flow is said to be subcritical flow or tranquil or streaming flow. Fr <1
,the flow is said to be subcritical flow or tranquil or streaming flow. Fr <1 - When Froude number is less than one or V=
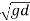 ,the flow is said to be Critical flow. Fr =1
,the flow is said to be Critical flow. Fr =1 - When the Froude number is greater than one or V>
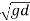 , the flow is said to be super critical flow or Rapid flow or Shooting flow. Fr >1
, the flow is said to be super critical flow or Rapid flow or Shooting flow. Fr >1
Q5) Define Reynolds number and Euler number with applications.
A5) Reynolds’s Number:
The ratio of inertia force to the viscous force is called as Reynold's number. It is denoted by Re.
=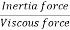
=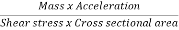
=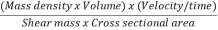
=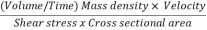
=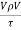 =
=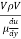
= =
=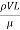 =
= =
=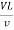 ∵v=
∵v=
Applications of Reynolds number:
Motion of completely submerged bodies like submarine, aero-planes .Incompressible flow through pipes, flow through low-speed turbo machine in which viscous forces are predominant.
Euler's Number:
The square root of the ratio of inertia force to the pressure force is called as Euler's ratio.
= =
=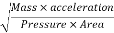
=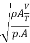 =
=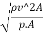 =
=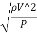
=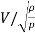 ∵A/T=Q=V.A
∵A/T=Q=V.A
Application:
It is applied in cavitation study where pressure force is predominant.
Q6) What is circulation, stream function velocity potential?
A6) Circulation
- In rotational motion, circulation is very useful concept.
- Circulation around a closed curve C is define as the integrate of the tangential component of the velocity taken around a closed curve C, taken positive in anticlockwise direction. The flow along a closed curve called as circulation.
- E.g., Flow in eddies and vortices
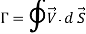
Stream function
- It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
- It is denoted by Ψ (psi) and defined only for two-dimensional flow.
- Mathematically, for ready flow it is defined as Ψ = f (x, y) such that
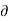 Ψ /
Ψ / 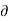 x= v
x= v
And 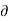 Ψ /
Ψ / 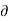 y = -u
y = -u
Velocity potential
- The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
- It is denoted by Ø (phi)
- Thus, mathematically the velocity potential is defined as
Ø = f (x, y, z, t)
And Ø = f (x, y, z)
u = -  Ø /
Ø / 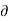 x
x
v = - 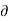 Ø /
Ø / 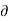 y
y
w = - 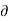 Ø /
Ø / 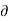 z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
- The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q7) Write a note on Buckingham’s Pi theorem.
A7)
- When a large number of physical variables are involved Rayleigh’s method of dimensional analysis becomes increasingly laborious and cumbersome.
- Buckingham’s method is an improvement over the Rayleigh’s method.
- Buckingham’s designated the dimensionless group by the letter Π. It is therefore often called Buckingham Π-Method.
- The Buckingham’s Π-Method states as follows:
“If there are n variables in a dimensionally homogeneous equation and if these variables contain fundamental dimensions (such as M, L, T, etc.) then the variables are arranged into (n-m) dimensionless terms.
- These dimensionless terms are called Π– terms.”
Mathematically, if any variable X1, depends on independent variables, X2, X3, X4,…… Xn; the functional equation may be written as
 -------- 1
-------- 1
Equation 1 can also be written as
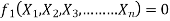 -------- 2
-------- 2
It is dimensionally homogeneous equation and contains n variables.
If there are m fundamental dimensions, then according to Buckingham’s Π – theorem, it can be written in terms of number of Π – terms in which number of Π terms is equal to (n-m).
Hence equation 2 becomes as
 --------3
--------3
Each dimensionless 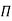 – term is formed by combining m variables out of the total n variables with one of the remaining (n-m) variable.
– term is formed by combining m variables out of the total n variables with one of the remaining (n-m) variable.
These m variables which appear repeated in each of 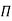 – terms are consequently called repeating variables and are chosen from among the variables such that they together involve all the fundamental dimensions and they themselves do not form a dimensionless parameter.
– terms are consequently called repeating variables and are chosen from among the variables such that they together involve all the fundamental dimensions and they themselves do not form a dimensionless parameter.
Let in the above case X2, X3 and X4 are the repeating variables if the fundamental dimensions m (M, L, T) = 3, then each term is written as:
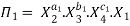
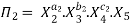
.
.
.
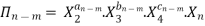 -------- 4
-------- 4
Where a1, b1, c1, a2, b2, c2 etc. are the constants, which are determined by considering dimensional homogeneity. These values are substituted in equation 4 and values of 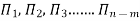 are obtained. These values of Π’s are substituted in equation 3.
are obtained. These values of Π’s are substituted in equation 3.
The final general equation for the phenomenon may then be obtained by expressing anyone of the Π – terms as a function of the other as:
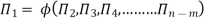
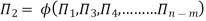
Q8) Define stream line, Stagnation point, path line and streak line.
A8) Stream line
A stream line is an imaginary curve created through a flowing fluid in such a way that the tangent to it at any location reveals the direction of the flow's instantaneous velocity (V) at that place.
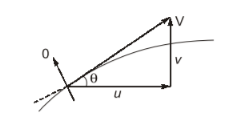
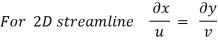
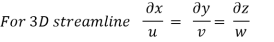
Because velocity is always tangential to the stream line, there is no component of velocity perpendicular to the stream line; thus, any discharge trapped between two stream lines remains trapped there; thus, as the gap between stream lines increases, velocity decreases; and as the gap decreases, velocity increases.
Stagnation point: In a fluid flow system, the point at which the velocity of flow becomes zero is referred to as the stagnation point.
Path Line: The actual path taken by every individual fluid particle over a given time period is referred to as the path line, and path line is a lagrangian concept. These are the actual lines, not fictitious ones. These lines may intersect.
Streak Line: It is the location of all fluid particles that have passed through the same point at the same time.
Q9) The velocity potential for a two dimensional flow is Փ = x (2y-1) Determine the velocity at the point P(4, 5). Also obtain the value of stream function at P. (2019-20)
A9) Velocity
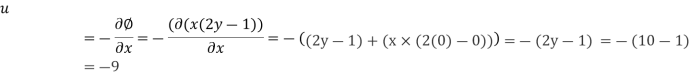
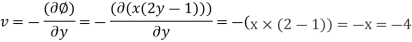
The magnitude of velocity at point P (4,5) is
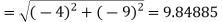
Q10) If the velocity field is given by u = x + y and v = x3 – y Find the circulation around a closed contour defined by x = 1, y = 0, y = 1 and x = 0.
A10) Contours Corners at A(0, 0), B(1, 0), C(1, 1), D(0, 1)
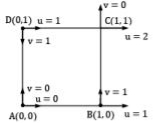
Circulation
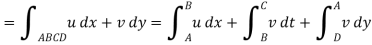

Based on shape of contour y = 0 for 1st integral x = 1 for 2nd integral y = 1 for
3rd integral x = 0 for 4th integral
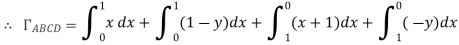

If we check  in this equation
in this equation
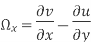
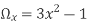
i.e. vorticity is non-uniform and thus the method mentioned in question 14 cannot be used directly.
Q11) If stream function for steady flow is given by ψ = x2-y2, determine whether the flow is rotational or irrotational. Find the potential function, if the flow is irrotational and vorticity, if it is rotational.
A11) Solution
Stream function ψ is given by,
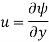
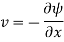
Thus, the velocity components become
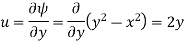
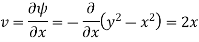
The rotation is given by
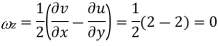
The rotation is zero, the flow is irrotational that implies the velocity potential exists.
For irrotational flow, the velocity potential (φ)
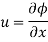
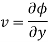
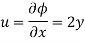
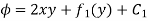
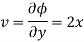
Or
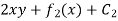 (2)
(2)
From equation 1 and 2
f1(y) =0 and f2(x)=0
So velocity potential for the flow is φ = 2xy + C
Q12) For velocity components are given by u =x –4y and v=-y-4x. Check the following
- Compressible or incompressible
- Rotational or irrotational
A12)
- Compressible or incompressible
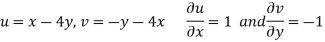
That is
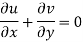
The above velocity field fulfills the incompressible flow continuity equation. As a result, the flow is incompressible.
2. Rotational or irrotational
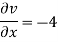
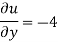
The rotation is given by
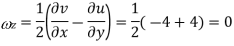
The flow is irrotational since the rotation is zero.
Q13) If the flow is incompressible, irrotational and velocity components are given by u =x –4y and v=-y-4x. Find stream function ψ and the velocity potential (φ).
A13)
1. Stream function ψ is given by
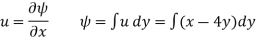
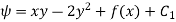 (1)
(1)
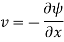
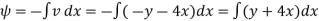
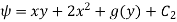 (2)
(2)
From equation 1 and 2
f(x) =2x3 and g(y)=-2y2
So Stream function is given by
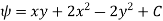
2. The velocity potential (φ) is given by

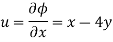
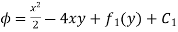 (1)
(1)
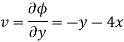
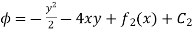 (2)
(2)
From equation 1 and 2
f1(y) = and f2(x) = =
and f2(x) = =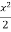
So velocity potential for the flow is
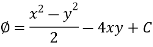
Q14) The pressure difference Δp in a pipe of diameter D and length l due to viscous flow depends on the velocity V, viscosity µ and , density ρ. Using Buckingham π-theorem, obtain the expression for Δp.
A14)
| Variables. | Dimensions |
1 | The pressure difference Δp | MLT-2 |
2 | Diameter D | L |
3 | Velocity V | LT-1 |
4 | Viscosity µ | ML-1T-1 |
5 | Density ρ. | ML-3 |
6 | Length l | L |
No of variables, n =6
No of independent dimensions (M, L, T), m=3
No of non-dimensional group Πs =n-m= 6-3 =3
Choosing dimensionally independent variable, geometric (D), Time Dependent(Kinematic) (V), mass-dependent (Dynamic) (ρ)
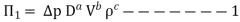
Considering dimension on both side
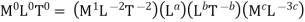
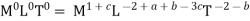
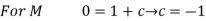
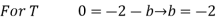
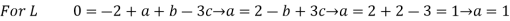
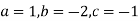
Substituting values in 1

By inspection,



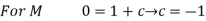
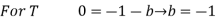
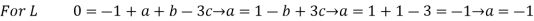
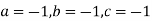
Substituting values in 4

Non dimensional relationship
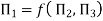
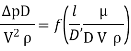
Also
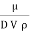 is reciprocal of Reynolds no.
is reciprocal of Reynolds no.
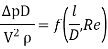

Also
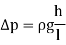
Substituting in 6
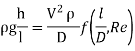
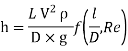
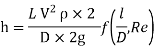
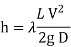
This is Darcy-Weisbach equation where λ is a function of relative roughness l/D and Reynolds number Re