BEE
UNIT – 2Magnetic Circuit & Single-Phase Transformer Q1) A 2500/200 V transformer draws a no-load primary current of 0.5 A and absorbs 400 W. find magnetising and loss currents.A 1)Iron-loss current = No load input(W) / Primary voltage= 400/2500 = 0.16 AI20 = I2w + I2µ
Iµ = √I20 – I2w= √ (0.5)2 – (0.16)2Iµ = 0.473 A Q2) A 1-φ transformer has 1000 turns on primary and 200 on secondary. The no load current is 4 amp at p.f of 0.2 lagging. Find primary current and pf when secondary current is 280 A at pf of 0.6 lagging.A 2)cos-1 0.6 = 53.130 (sin φ = 0.8)I2 = 280/-53.130AΦ = cos-1 0.2 = 78.50Sin φ = 0.98I1 = I0 + I’2I’2 = (I2/K) ( -53.130K = N1/N2 = 1000/200 = 5I’2 = 280/5 (-53.130I’2 = 56(-53.130I1 = I0 + I’2= 4(0.20 – j0.98) + 56(0.6 – j0.8)= 0.80 – j3.92 + 33.6 – j44.8I1 = 34.4 – j48.72I1 = 59.64 ( -54.770I lags supply voltage by 54.770Q3) A 1- φ transformer with ratio of 440/110-V takes a no-load current of 6 A at 0.3 pf lagging. If secondary supplies 120 A at pf of 0.8 lagging. Find current taken by primary.
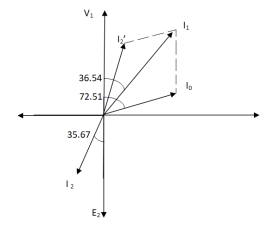
A 3) Cos φ2 = 0.8Φ2 = 36.540Cos φ0 = 0.3Φ0 = 72.540K = V2/V1 = 110/440 = ¼I’2 = KI2 = 120 x ¼ = 30 AI0 = 6AAngle between I0 & I’2= 72.54 – 36.54= 35.670From vector diagram,I1 = √(62 + 302 + 2 x 6 x 30 cos 35.67)I1 = 35.05 A Q4) A – 100 KVA transformer has 500 turns on primary and 80 turns on secondary. The primary and secondary resistances are 0.3 and 0.01 Ω respectively and the corresponding leakage reactances are 1.1 and 0.035 Ω. The supply voltage is 2400 V. Find (i). Equivalent impedance referred to primary(ii). Voltage regulation and the secondary terminal voltage for full load having pf 0.8 lagging?A 4)Equivalent impedance referred to primaryZ01 = √R201 + X201 = R01 + jX01R01 = R1 + R2/K2 = 0.3 + 0.01/K2 = 0.69 ΩK = 80/500 = 4/25X01 = X1 + X2/K2 = 1.1 + 0.035/(0.16)2 = 2.467 ΩZ01 = 0.69 + j2.46 (ii). Secondary terminal voltage Z02 = K2 Z01Z02 = 0.018 + j 0.063= 0.065 ( 74.050 No-load secondary voltage = KV1= 0.16 x 2400 = 384 VI2 = 100 x 103/384 = 260.42 A Full load voltage drop referred to secondary = I2 (R02 cosφ – X02 Sinφ)Cosφ = 0.8Φ = 36.860Sinφ = 0.6= 260.42(0.018 x 0.8 – 0.063 x 0.6)= - 6.094 V% regn = -6.094/384 x 100= -1.587 Secondary terminal voltage on-load= 384 – (-6.094)= 390.09 V Q5) In a 50 KVA, 2200/200 V, 1-φ transformer, the iron and full-load copper losses are 400 W and 450 W respectively. Calculate n at unity power factor on (i). Full load (ii). half-full load? A5) (i). Total loss = 400 + 450 = 850 WF.L output at unity power factor = 50 x 1= 50 KVAn = 50 / 50 + .850 = 50/50.850 = 0.98 = 98% (ii). half full load, unity pf= 50 KVA/2 = 25 KVACu loss = 400 x (1/2)2 = 100 WIron loss is same = 450 WTotal loss = 100 + 450 = 550 Wn = 25/25 + 0.55 = 25/25.55 = 0.978 = 97.8 % Q6) A 40 KVA 440/220 V, 1- φ, 50 Hz transformer has iron loss of 300 W. The cu loss is found to be 100 W when delivering half full-load current. Determine (i) n when delivering full load current at 0.8 lagging pf (ii) the percentage of full-load when the efficiency will be max.A 6)Full load efficiency at 0.8 pf= 40 x 0.8/(40 x 0.8) + lossesFull load cu loss = (440/220)2 x 100= 400 WIron loss = 400 + 300= 700 Wn = 40 x 0.8/(40 x 0.8) + 0.7 = 97.8 % (ii). KVA for maximum / F.L KVA = √ iron loss / F.L cu loss = √300/400 = 0.866 Q7) An auto transformer suppliers load of 4KW at 100v at unity pf. IF the applied primary voltage is 220v. calculating power transferred to load (a) Inductively (b) conductively.A 7) Power transferred inductively = Input(1-k) Power transferred conductively = K* Input K=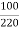 
=
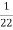Input= Output =4KWInductively transferred power =4(
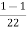)=3.82KWConductively transferred power = 
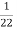*4= 0.182 kw Q8) The core of a 110 KVA, 10,000/500v, 50 Hz, 1-Φ core type transformer has a cross section of 18 cm x 18 cm. Find the number of HV and LV turns per phase and the emf per turn if the maximum core density does not exceed 1.3 tesla. Assume a stacking factor pf 0.9.A 8) Bm= 1.3TArea = (0.18 x 0.18) = 0.032m2Emf induced in primaryE1 = 4.44 fN1BmA10000=4.44 x 50 x N1 x 1.3 x 0.032N1=1082.8emf induced in secondaryE2 = 4.44 fN2BmA500= 4.44 x 50 x N2 x 1.3 x 0.032N2= 54.14 The number of turns is N1=1082.8 and N2= 54.14 Emf per turn = E1/E2 = N1/N2 = K =10000/1082=9.24V or 500/54.14=9.23V Q9) A 1-Φ transformer has 400 turns in primary and 110 turns in the secondary. The cross-sectional area of the secondary. The cross-sectional area of the core is 80cm2. If the primary winding is connected to the 50 Hz supply at 500V. Calculate peak flux density in core.A 9) As we know Emf induced in primaryE1 = 4.44 fN1BmA500 = 4.44 x 50 x 400 x Bm x (80x10‑4)Bm=0.704Wb/m2 Q10) A 2000/200v transformer draws a no-load primary current of 0.6A and absorbs 360 watts find the magnetising and iron loss currents.A 10)Iron loss current =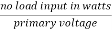
= 360/2000=0.18AI20 = I2w + I2µMagnetising component Iµ = √I20 – I2w= √ (0.6)2 – (0.18)2Iµ = 0.57 A Q11) A 2200/250 V transformer takes 0.7A at a p.f of 0.4 0n open circuit. Find the magnetising and working component of no load primary current?A11) I0= 0.7Acosφ0=0.4Iw= I0 cosφ0Iw=0.7 x 0.4=0.28AMagnetising component Iµ = √I20 – I2w= √ (0.7)2 – (0.280)2Iµ = 0.64 A Q12) A single phase transformer has 400 turns in primary and 1000 turns in secondary. The cross-sectional area is 80 cm2. If primary is connected to 50hz at 500V. Voltage induced in secondary?A 12)As we know Emf induced in primaryE1 = 4.44 fN1BmA500= 4.44x50x1000xBmx(80x10-4)Bm= 0.28Wb/m2The voltage induced in secondary is given asE1/E2 = N1/N2 = KE2=1000x500/400=1250V Q13) A 200 KVA, 1200/200v, 50 Hz, 1-Φ transformer has a leakage impedance of (0.1 + 0.30) Ω for the HV winding and (0.005 + 0.015) Ω for the LV winding. Find the equivalent winding resistance, reactance and impedance referred to the HV and LV side.A 13) The Turn ratio is given as E1/E2 = N1/N2 = KK=6Referring to High Voltage SideResistance = R1+K2R2= 0.1+ 62 x 0.005 =0.28ohmReactance = X1+K2X2= 0.30+ 62 x0.015 = 0.84ohmImpedance = √0.282 +0.842 =0.880hmReferring to Low voltage sidesResistance= R1/ K2+R2= (0.1/62) + 0.005=0.007ohmReactance = Reactance HV side/ K2=0.84/62=0.013ohmImpedance = Impedance referring HV/ K2= 0.88/62 = 0.024ohm Q14) When a transformer is connected to a 1200v,50Hz supply the core loss is 900 W, of which 600 is hysteresis and 350 W is eddy current loss. If the applied voltage is raised to 2000V and frequency to 100 Hz. Find new core losses.A 14) Hysteresis Loss (Wh) α 
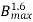f =P 
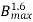fEddy current Loss (We) α P
f2 = Q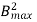 
fThe emf equation of transformer is given as E= 4.44fNBmaxABmax α E/fSo, the above equations become Wh = P (E/f)2 f = P E1.6 f-0.6600= P x 12001.6 x 50-0.6P=0.074The eddy current loss We= Q (E/f)2 f2 = QE2 350=Q x 12002Q=0.243 x 10-3Now the applied voltage is raised to 2000V so finding new losses with above found P and QWh = P E1.6 f-0.6 = 0.074 x 20001.6 x 100-0.6 = 893.06WWe= QE2 = 0.243 x 10-3 x 20002 = 972 W
|
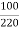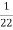=Input= Output =4KWInductively transferred power =4(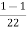)=3.82KWConductively transferred power = 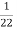*4= 0.182 kw Q8) The core of a 110 KVA, 10,000/500v, 50 Hz, 1-Φ core type transformer has a cross section of 18 cm x 18 cm. Find the number of HV and LV turns per phase and the emf per turn if the maximum core density does not exceed 1.3 tesla. Assume a stacking factor pf 0.9.A 8) Bm= 1.3TArea = (0.18 x 0.18) = 0.032m2Emf induced in primaryE1 = 4.44 fN1BmA10000=4.44 x 50 x N1 x 1.3 x 0.032N1=1082.8emf induced in secondaryE2 = 4.44 fN2BmA500= 4.44 x 50 x N2 x 1.3 x 0.032N2= 54.14 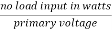= 360/2000=0.18AI20 = I2w + I2µMagnetising component Iµ = √I20 – I2w= √ (0.6)2 – (0.18)2Iµ = 0.57 A Q11) A 2200/250 V transformer takes 0.7A at a p.f of 0.4 0n open circuit. Find the magnetising and working component of no load primary current?A11) I0= 0.7Acosφ0=0.4Iw= I0 cosφ0Iw=0.7 x 0.4=0.28AMagnetising component Iµ = √I20 – I2w= √ (0.7)2 – (0.280)2Iµ = 0.64 A Q12) A single phase transformer has 400 turns in primary and 1000 turns in secondary. The cross-sectional area is 80 cm2. If primary is connected to 50hz at 500V. Voltage induced in secondary?A 12)As we know Emf induced in primaryE1 = 4.44 fN1BmA500= 4.44x50x1000xBmx(80x10-4)Bm= 0.28Wb/m2The voltage induced in secondary is given asE1/E2 = N1/N2 = KE2=1000x500/400=1250V Q13) A 200 KVA, 1200/200v, 50 Hz, 1-Φ transformer has a leakage impedance of (0.1 + 0.30) Ω for the HV winding and (0.005 + 0.015) Ω for the LV winding. Find the equivalent winding resistance, reactance and impedance referred to the HV and LV side.A 13) The Turn ratio is given as E1/E2 = N1/N2 = KK=6Referring to High Voltage SideResistance = R1+K2R2= 0.1+ 62 x 0.005 =0.28ohmReactance = X1+K2X2= 0.30+ 62 x0.015 = 0.84ohmImpedance = √0.282 +0.842 =0.880hmReferring to Low voltage sidesResistance= R1/ K2+R2= (0.1/62) + 0.005=0.007ohmReactance = Reactance HV side/ K2=0.84/62=0.013ohmImpedance = Impedance referring HV/ K2= 0.88/62 = 0.024ohm Q14) When a transformer is connected to a 1200v,50Hz supply the core loss is 900 W, of which 600 is hysteresis and 350 W is eddy current loss. If the applied voltage is raised to 2000V and frequency to 100 Hz. Find new core losses.A 14) Hysteresis Loss (Wh) α 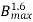f =P 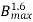fEddy current Loss (We) α P f2 = Q 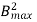fThe emf equation of transformer is given as E= 4.44fNBmaxABmax α E/fSo, the above equations become Wh = P (E/f)2 f = P E1.6 f-0.6600= P x 12001.6 x 50-0.6P=0.074The eddy current loss We= Q (E/f)2 f2 = QE2 350=Q x 12002Q=0.243 x 10-3Now the applied voltage is raised to 2000V so finding new losses with above found P and QWh = P E1.6 f-0.6 = 0.074 x 20001.6 x 100-0.6 = 893.06WWe= QE2 = 0.243 x 10-3 x 20002 = 972 W 
0 matching results found