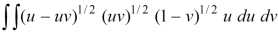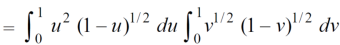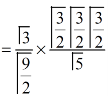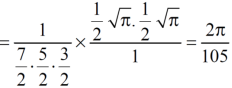Question Bank
Unit- 5
Question-1:: Evaluate
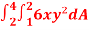
Sol. Let , I = 
= 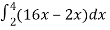
= 
= 
= 84 sq. Unit.
Which is the required area.
Question-2: Evaluate 
Sol. Let us suppose the integral is I,
I = 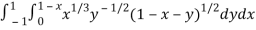
Put c = 1 – x in I, we get
I = 
Suppose , y = ct
Thendy = c
Now we get,
I = 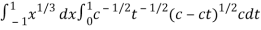
I = 
I = 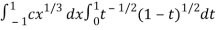
I = 
I =
As we know that by beta function,
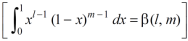
Which gives,
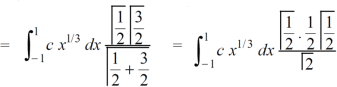
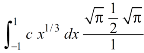
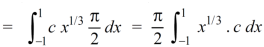
Now put the value of c, we get
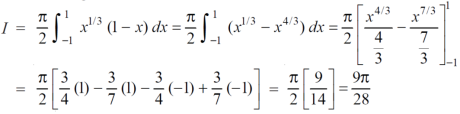
Qjuestion-3: Evaluate  ey/x dy dx.
ey/x dy dx.
Soln. :
Given :I =  ey/x dy dx
ey/x dy dx
Here limits of inner integral are functions of y therefore integrate w.r.t y,
I = 
 dx
dx
= 
= 
I = 
= =
 ey/xdy dx=
ey/xdy dx=
Question – 4: Evaluate 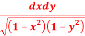
Soln. :
Let,I =
Here limits for both x and y are constants, the integral can be evaluated first w.r.t any of the variables x or y.
I = dy
I= 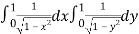
= 
= 
= 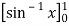
=
= 
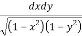 =
=
Question-5: Evaluate e–x2 (1 + y2) x dx dy.
Soln. :
Let I = e–x2 (1 + y2) x dy =dy e–x2 (1 + y2) x dy
= dy e– x2 (1 + y2)  dx
dx
=dy [∵ f (x) ef(x) dx = ef(x) ]
= (–1) dy (∵ e– = 0)
= = =
e–x2 (1 + y2) xdxdy =
Question-6: Evaluate y dx dy over the area bounded by x= 0 y =  and x + y = 2 in the first quadrant
and x + y = 2 in the first quadrant
Soln. :
The area bounded by y = x2 (parabola) and x + y = 2 is as shown in Fig.6.2
The point of intersection of y = x2 and x + y = 2.
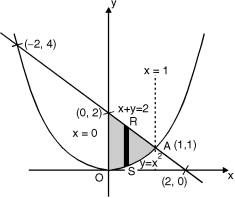
x + x2 = 2 x2 + x – 2 = 0
x =1, – 2
At x = 1, y = 1 and at x = –2, y = 4
Fig. 6.2
(1, 1) is the point of intersection in Ist quadrant. Take a vertical strip SR, Along SR x constant and y varies from S to R i.e. y = x2 to y = 2 – x.
Now slide strip SR, keeping IIel to y-axis, therefore y constant and x varies from x = 0 to x = 1.
I = 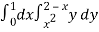
= 
= 
= (4 – 4x +  –
–  ) dx
) dx
= =
I = 16/15
Question-7:Evaluate  over x 1, y
over x 1, y 
Soln. :
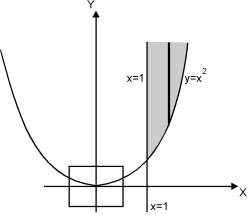 Let I=
Let I= over x 1, y
over x 1, y 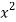
The region bounded by x 1 and y 
Is as shown in Fig. 6.3.
Fig. 6.3
Take a vertical strip along strip x constant and y varies from y = 
To y = . Now slide strip throughout region keeping parallel to y-axis. Therefore y constant and x varies from x = 1 to x = .
I= 
= 
=  [ ∵
[ ∵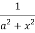 dx = tan–1 (x/a)]
dx = tan–1 (x/a)]
=  =
=
=– = (0 – 1)
I =
Question-8: Evaluate (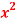 +
+ 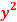 ) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
Soln. :
Let I = ( +
+  ) dx dy
) dx dy
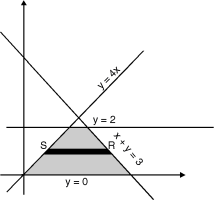 The area enclosed by the curves y = 4x, x + y =3, y = 0 and y = 2 is as shown in Fig. 6.4.
The area enclosed by the curves y = 4x, x + y =3, y = 0 and y = 2 is as shown in Fig. 6.4.
(find the point of intersection of x + y = 3 and y = 4x)
Fig. 6.4
Take a horizontal strip SR, along SR y constant and x varies from x = to x = 3 – y. Now slide strip keeping IIel to x axis therefore x constant and y varies from y = 0 to y = 2.
I = dy(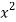 +
+  ) dx
) dx
= 
= 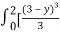 +
+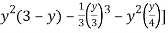 dy
dy
I=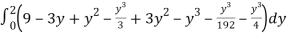
= 
= 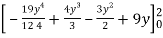
= + – 6 + 18
I=
Question-9:Calculate the volume generated by the revolution of a cardioid,
r = a ( 1 – cosθ) about its axis
Sol. Here, r = a ( 1 – cosθ)
Volume =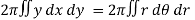

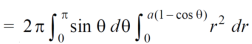
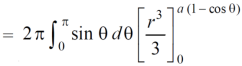
= 
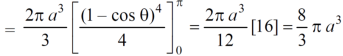
Which is the volume of generated by cardioid.
Question-10:Find the volume generated by revolving a circle x ² + y² = 4 about the line x= 3.
Sol. We know that,
Volume = 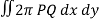
Here , PQ = 3 – x,
= 
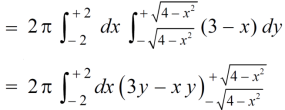
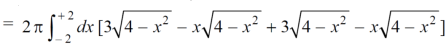
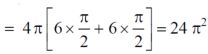
The volume is 24π².
Question-11:Calculate the volume under the surface z = 3 + x² - 2y over the region R defined as 0≤ x ≤ 1 and -x ≤ y ≤ x
Sol. The is a double integral of z = 3 + x² - 2y over the region R .
Volume will be,
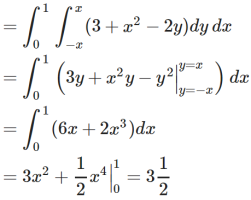
Question-12: Change the order of integration for the integral  and evaluate the same with reversed order of integration.
and evaluate the same with reversed order of integration.
Soln. :
Given, I =  …(1)
…(1)
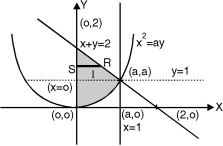 In the given integration, limits are
In the given integration, limits are
y= , y = 2a – x and x = 0, x = a
, y = 2a – x and x = 0, x = a
The region bounded by x2 = ay, x + y = 2a Fig.6.5
And x = 0, x = a is as shown in Fig. 6.5
Here we have to change order of Integration. Given the strip is vertical.
Now take horizontal strip SR.
To take total region, Divide region into two parts by taking line y = a.
1 stRegion :
Along strip, y constant and x varies from x = 0 to x = 2a – y. Slide strip IIel to x-axis therefore y varies from y = a to y = 2a.
I1=dyxy dx…(2)
2nd Region :
Along strip, y constant and x varies from x = 0 to x = . Slide strip IIel to x-axis therefore x-varies from y = 0 to y = a.
I2=dyxy dx…(3)
From Equation (1), (2) and (3),
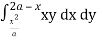 = dyxy dx + dyxy dx
= dyxy dx + dyxy dx
=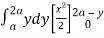 + y dy
+ y dy
= dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2dy
dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2dy
= (4a2 y – 4ay2 + y3) dy + y2dy
=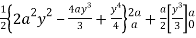
=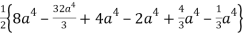 +
+ 
= a4
Question-13: Express as single integral and evaluate dy dx + dy dx.
Soln. :
Given :I = dy dx + dy dx
I = I1 + I2
The limits of region of integration I1 are
x = – ; x = and y = 0, y = 1 and I2 are x = – 1,
x = 1 and y = 1, y = 3.
The region of integration are as shown in Fig. 6.7
To consider the complete region take a vertical strip SR along the strip y varies from y = x2 to
y = 3 and x varies from x = –1 to x = 1.Fig. 6.7

I = 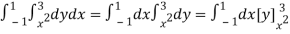
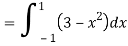
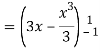
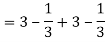
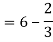
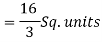
Question-14:Evaluate
Soln. :
The region of integration bounded by
y = 0, y =  and x = 0, x = 1
and x = 0, x = 1
y = 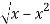 x2 + y2 = x
x2 + y2 = x
The region bounded by these is as shown in Fig. 6.8.
Convert the integration in polar co-ordinates by using x = r cos, y = r sin and dx dy = r dr d
x2 + y2= x becomes r = cos
y = 0 becomes r sin = 0 = 0
x = 0 becomes r cos = 0 =
And x = 1 becomes r = sec Fig. 6.8
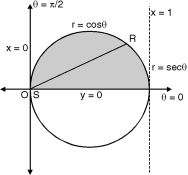
Take a radial strip SR with angular thickness , Along strip constant and r varies from r = 0 to r = cos. Turning strip throughout region therefore varies from = 0 to =
I = 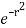 r dr d
r dr d
= 4 cos sin d r 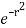 dr
dr
= 4 cos sin d [–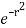 ]
]
= – 2 cos sin [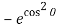 +1] d
+1] d
= – 2 [cos sin  – cos sin ] d
– cos sin ] d
= –2  + 2 cos sin d
+ 2 cos sin d
= – + 2
= + 1 =
Question-15: Evaluate r4 cos3dr d over the interior of the circle r = 2a cos
Soln. :
 The region of the integration is as shown in Fig. 6.10.Take a radial strip SR, along strip constant and r varies from r = 0 to r = 2a cos. Now turning this strip throughout region therefore varies from = to =
The region of the integration is as shown in Fig. 6.10.Take a radial strip SR, along strip constant and r varies from r = 0 to r = 2a cos. Now turning this strip throughout region therefore varies from = to =
I = r4 cos3dr d
= cos3 d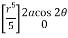
= 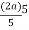 cos3 cos5 d
cos3 cos5 d
=  cos8 dFig. 6.10
cos8 dFig. 6.10
= 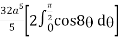
= 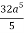 2
2
I = 
r4 cos3dr d= 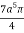
Question-16: Evaluate r sin dr d over the cardioid r = a (1 – cos) above the initial line.
Soln. :
The cardioid r = a (1 – cos) is as shown in Fig. 6.11. The region of the integration is above the initial line.
Take a radial strip SR, along strip constant and r Varies from r = 0 to r = a (1 – cos).
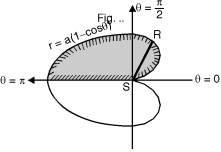 New turning the strip throughout region therefore varies from = 0 to = .
New turning the strip throughout region therefore varies from = 0 to = .
I= r sin dr d
= sin d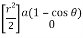
Fig.6.11
=sin [a2 (1 – cos)2]
= 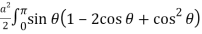
I=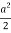 (sin – 2 sin cos + sin
(sin – 2 sin cos + sin  ) d
) d
= 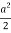 2 (sin – sin2 + sin
2 (sin – sin2 + sin  ) d
) d
=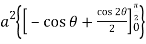 +
+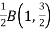
I= a2= a2
I= 
Question-17: Show that the area of curve 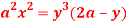 is
is 
Solution: The curve is symmetrical about Y - axis passing through origin and there exists a cusp at origin. It intersects Y-axis at (0 , 0) , (0 , 2a) .
Also 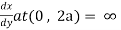 .
.
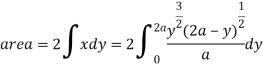
Putting 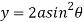
 .
.
Question-18: : Find the Area included between the two cardioids 
Solution: - Area = 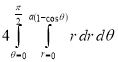
= 
= 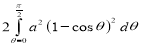
= 
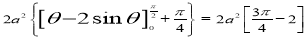
Question-19: Find the Area common to the two circle 
Solution: - By converting the given circle into polar form we get
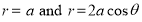
Area = 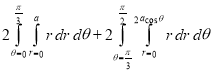
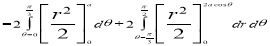
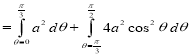
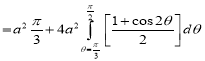
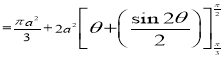
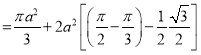
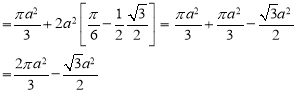
Question-20: : Evaluate the following by changing to polar coordinates,
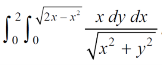
Sol. In this problem, the limits for y are 0 to 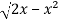 and the limits for are 0 to 2.
and the limits for are 0 to 2.
Suppose,
y = 
Squaring both sides,
y² = 2x - x²
x² + y² = 2x
But in polar coordinates,
We have,
r² = 2r cosθ
r = 2 cosθ
From the region of integration, r lies from 0 to 2 cosθ and θ varies from 0 to π / 2.
As we know in case of polar coordinates,
Replace x by r cosθ and y by r sinθ ,dy dx by r drdθ,
We get,
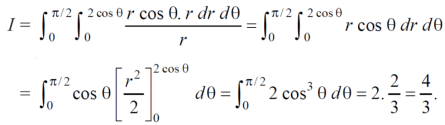
Question-21: Evaluate the following integral by converting into polar coordinates.
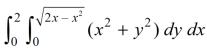
Sol. Here limits of y ,
y = 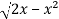
y² = 2x - x²
x² + y² = 2x
x² + y² - 2x = 0 ………………(1)

Eq. (1) represent a circle whose radius is 1 and centre is ( 1, 0)
Lower limit of y is zero.
Region of integration in upper hapf circle,
First we will covert into polar coordinates,
By putting
x by r cosθ and y by r sinθ , dy dx by r drdθ,
Limits of r are 0 to 2 cosθ and limits of θ are from 0 to π / 2.
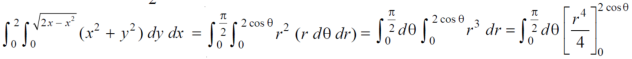
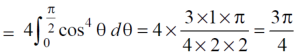
Question-22: Evaluate 
Sol. Let the integral,
I = 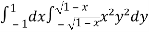
=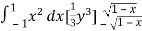

Put x = sinθ
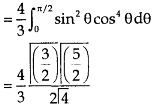
= π / 24 ans.
Question-23:Find the area between the parabola y ² = 4ax and another parabola x² = 4ay.
Sol. Let,
y² = 4ax ………………..(1)
And
x² = 4ay…………………..(2)
Then if we solve these equations , we get the values of points where these two curves intersect
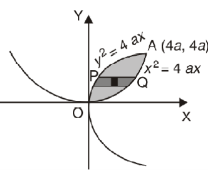
x varies from y²/4a to 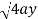 and y varies from o to 4a,
and y varies from o to 4a,
Now using the conceot of double integral,
Area = 
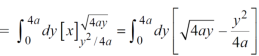
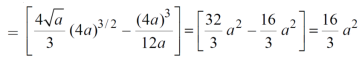
Question-24: Find the are lying inside a cardioid r = 1 + cosθ and ouside the parabola r(1 + cosθ) = 1.
Sol. Let,
r = 1 + cosθ ……………………..(1)
r(1 + cosθ) = 1……………………..(2)
Solving these equestions , we get
(1 + cosθ )(1 + cosθ ) = 1
(1 + cosθ )² = 1
1 + cosθ = 1
Cosθ = 0
θ = ±π / 2
So that, limits of r are,
1 + cosθ and 1 / 1 + cosθ
The area can be founded as below,

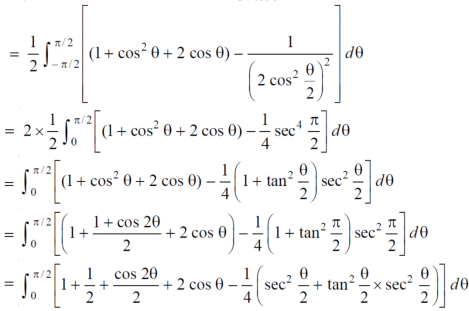
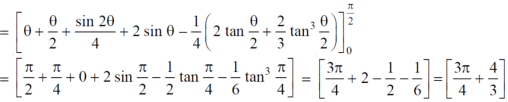
Question-25: Evaluate
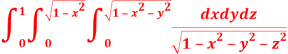
Solution : Let
I = 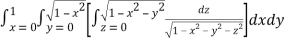
= 
(Assuming m = 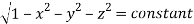 )
)
= 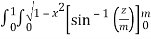 dxdy
dxdy
= 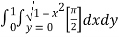
=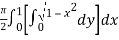
=  dx
dx
= 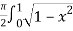 dx
dx
= 
=
I =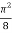
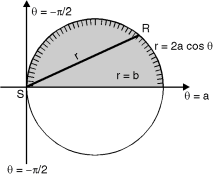
Question-26: : Evaluate 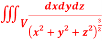 Where V is annulus between the spheres
Where V is annulus between the spheres 
And (
(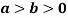 )
)
Solution: It is convenient to transform the triple integral into spherical polar co-ordinate by putting
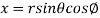 ,
,  ,
, 
 , dxdydz=
, dxdydz=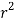 sin
sin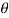 drd
drd d
d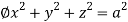 ,
,
 and
and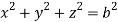
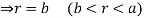
For the positive octant, r varies from r =b to r =a , 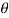 varies from
varies from 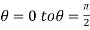
And varies from
varies from 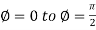
I= 
= 8
=8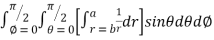
=8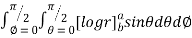
=8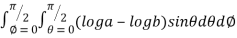
=8 log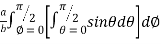
= 8 log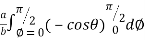
I= 8 log I = 4 log
I = 4 log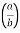
Question-27: Evaluate 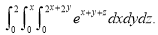
Solution:-
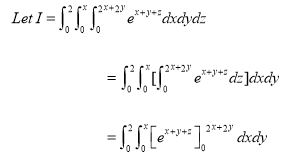
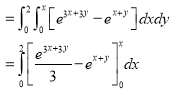
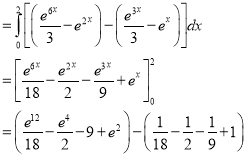
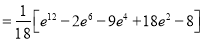
Question-28:Evaluate
Solution:- 

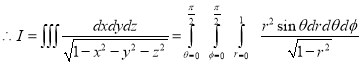

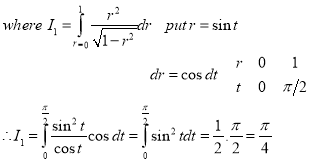


Question-29: : Find Volume of the tetrahedron bounded by the co-ordinates planes and the plane
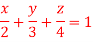
Solution: Volume =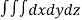 ………. (1)
………. (1)
Put 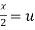 ,
, 
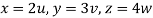
From equation (1) we have
V = 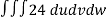
=24
=24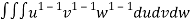 (u+v+w=1) ByDirichlet’s theorem.
(u+v+w=1) ByDirichlet’s theorem.
=24 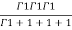
=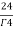 =
= = 4
= 4
Volume =4
Question-30: Evaluate
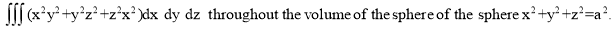
Solution:-
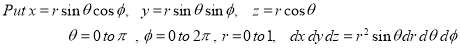
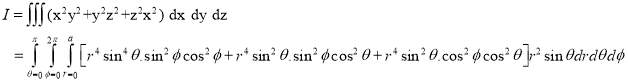
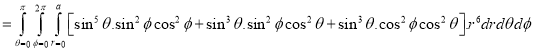
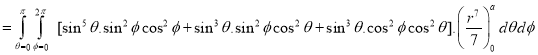
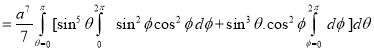
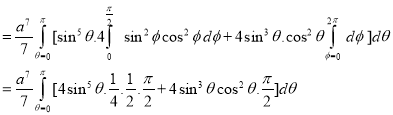
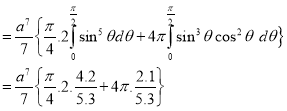
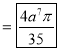
Question-31:Find mean value and R.M.S. Value of the ordinate of cycloid
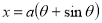 ,
,  over the range
over the range  to
to  .
.
Sol Let P(x,y) be any point on the cycloid . Its ordinate is y.
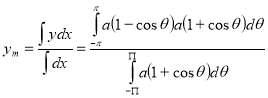
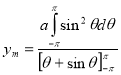
= 
=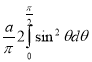
=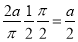
R.M.S.Value= 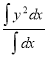
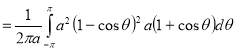
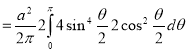
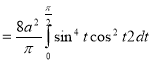

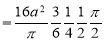
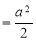
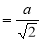
Question-32: Find The Mean Value of Over the positive octant of the
Over the positive octant of the
Ellipsoid 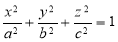
Sol: M.V.=
Since 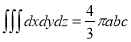
Put 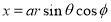
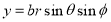
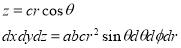
M.V.=

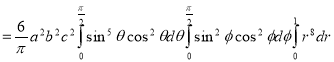
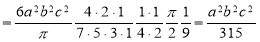
Question-33: Evaluate the triple integral 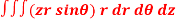 , where the limits of r ,
, where the limits of r , , z are 0≤ r ≤ 2 , 0 ≤ θ ≤ π / 2 and 0 ≤ z ≤ 4.
, z are 0≤ r ≤ 2 , 0 ≤ θ ≤ π / 2 and 0 ≤ z ≤ 4.
Sol. We can use the method of triple integration as follows,
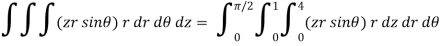
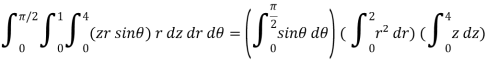
On solving the above integral, it gives
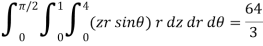
Therefore the value of the integral is 64/3
Question-34: ; Using the transformation x + y = u and y = uv, find the value of the following double integral , taken over the area of the triangle bounded by the lines x = 0 and y = 0 , x + y = 1.
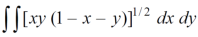
Sol. It is given that,
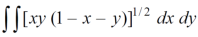
We have,
x + y = u or x = u – yor x = u – uv
 =
= 
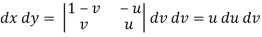
x = 0 ,
u ( 1 – v) = 0
u = 0 , v = 0
y = 0
Uv = 0
u = 0 , v = 0
x+y = 1
u = 1
Therefore the limit of u is from 0 to1 and the limit of v are from 0 to 1.
On putting x = u – uv , y = uv , dx dy = u du dv , we get