GUJARAT TECHNOLOGICAL UNIVERSITY

WAVES, MOTION AND ACOUSTICS

MODULE 2

QUESTION BANK

1 Question: A cinema hall has a volume of 7500m3. It is required to have reverberation time of 1.5 sec? What should be the absorption in the hall?

Solution:

Given

Volume = 7500m3

Reverberation time T = 1.5 sec

We know that reverberation time is given by

T =   0.165 V/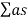

T =   0.165 V/ A

Where V-Volume of hall in m3

A - Absorption coefficient

1.5 =  0.165 x 7500/A

A= 825 O.W.S

2 Question: A volume of room is 1200m3. The wall area of room is 200 m3. The floor wall area of room is 120m3 and ceiling area is 120m3 and. The average the sound absorption coefficient (i) for walls is 0.03 (ii) for ceiling is 0.80 (iii) for the floor is 0.06. Calculate the average absorption coefficient and reverberation time?

Solution:

Given

Volume = 1200m3

The average absorption coefficient is

A = a1s1 +a2s2 +a3s3 / s1 +s2 +s3

A = (0.03 x 200 + 0.80 x120 +0.06 x 120) / (200+120+120)

A = 0.2389 =0.24 (approx.)

Now the total absorption of room = As =0.24 x460 =110.40 O.W.S.

Reverberation Time T =   0.165 V/AS = 0.165 x 1200/ 109.2

= 1.80 seconds

3 Question: For an empty hall of size 20x15x10 m3 the reverberation time is 3.5 sec. Calculate the average absorption coefficient. What area of the wall should be covered by the curtain so as to reduce reverberation time 2.5 sec. Given the absorption coefficient of curtain clothe is 0.5

Solution:

A =as = (0.165) x (20x15x10)/ 3.5 = 138 m2

When wall are covered with the curtain clothe

2.5 = (0.165) x (20x15x10)/ 0.5x s

Therefore area of the wall covered by the curtain

s = 483- (2.5 x 138)  /2.5 x 0.5

110.4 m2

4 Question: What is the relationship between Velocity, Frequency and Wavelength of a Wave?

Solution:

Velocity = Distance travelled/ Time taken
Let v = λ / T
Where T = time taken by one wave.
v = f X λ
This formula is known as wave equation.
Where v = velocity of the wave
f = frequency
λ = wavelength
Velocity of a wave = Frequency X Wavelength

This applies to all the waves like transverse waves like water waves, longitudinal waves like sound waves and  the electromagnetic waves like light waves and radio              waves

5 Question: List the requirement for good acoustics?

Solution:

Acoustics, the science concerned with the production, control, transmission, reception, and effects of sound. The term is derived from the Greek akoustos, meaning heard.

Acoustics in architecture means improving sound in environments. Although it is a complex science, understanding the basics - and making efficient and effective decisions - is much easier than you might think. The first step is to understand that there are two technical categories used in acoustics: soundproofing and acoustical treatment. Soundproofing means "less noise" and acoustical treatment, "better sound”.

According to classic acoustics theory there are five requirements which, when met, result in good acoustics:

• An appropriate reverberation time
• Uniform sound distribution
• An appropriate sound level
• An appropriately low background noise
• No echo or flutter echo

Acoustic requirement for good auditorium is as follows-

• The initial sound should be of adequate intensity.
• The sound should be evenly distributed throughout the hall.
• The successive nodes should be clear & distinct.
• Noise has to be taken care of.
• The size & the shape of the ball have also to be taken care.

These requirements can be achieved by following ways-

Site/location:-Before construction the first important factor to be considered is the location. For best acoustical quality of the hall, it should be far from railway tracks, industrial areas, airports, & highways, etc.

Size: - The size of the hall should be optimum, neither big nor small. It is small uneven distribution of sound will take place due to the formations of stationary waves. If size is too big reverberation time will be more that results in confusion & unpleasant sound.

Shape: - Instead of parallel walls spade walls are preferred, curved surfaces should be built with proper care.

Reverberation: - Reverberation time (T) should be neither too small nor too large. If it is small intensity will be weak. If large sound will be unpleasant. Thick carpets curtains, upholstered chairs, audience take care of reverberation. For lecture halls the reverberation time is approximately 0.5sec, for music concerts hall-1.5sec, for cinema theatres-2sec

Absorption: - Use of proper absorbent material enhances the quality of sound.

Echelon effect: - The regular intervals/space between staircase or railings give repeated echo, this makes the sound unpleasant, so thick carpets take care of this & wide gaps between staircases are generally preferred. No echo or flutter echoes must occur for the acoustics to be good. It is easy to prevent echo by installing a little sound-absorbing material on the wall. 
 

6 Question: Define absorption coefficient?

Solution:

The coefficient of absorption of material is defined as the ratio of the sound energy absorbed by the surface to that of the total incident sound energy on the surface.

Absorption Coefficient =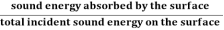

As all sound waves falling on an open window pass through, it can be assumed that an open window behaves as perfect absorber of sound and hence the standard of absorp­tion is taken as the unit area of an open window as a standard unit of absorption.

Thus, the absorption coefficient of a material is defined as the rate of the sound energy absorbed by a certain area of the surface to that of open window of same area.

The absorption coefficient of a surface is defined as the reciprocal of its area which absorbs the same sound energy absorbed at a unit area of an open window.

7 Question: What is Reverberation and Time of Reverberation?

Solution:

When a sound is produced in a building, it lasts too long after its production. It reaches to the listener a number of times. Once it reaches directly from the source and subsequently after reflection from the walls, windows, ceiling and flour of hall. The listener therefore receives series of sounds of diminishing intensity. By reverberation is meant the prolonged reflection of sound from the walls, floor and ceiling of a room.

The reverberation is defined as the persistence of audible sound after the source has stopped to emit sound. The duration for which the sound is stopped is called reverberation time. This time is measured from the instant the source stops emitting sound.

The time of reverberation is defined as the time taken by the sound to fall below the minimum audibility measured from the instant when the source stopped emitting sound.

According to Prof. W. C. Sabine, the standard reverberation time is defined as the time taken by sound to fall to one millionth of its intensity just before the sound is cut off.

8 Question: State Sabine’s formula for reverberation time ?

Solution:

According to W. C. Sabine, the time of reverberation depends up on

1. Size of hall,

2. Loudness of the sound,

3. Kind of music or sound for which hall is to be used.

Acoustics and Ultrasonic Reverberation Time

T =   0.165 V/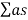

T =   0.165 V/ A

Where V-Volume of hall in m3

a - Absorption coefficient

S - Area of reflecting surface in a square meter

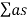Absorption of hall.

9 Question: Discuss the factors affecting acoustics of buildings and their remedies?

Solution:

Acoustically good hall we mean that in which every syllable or musical note reaches an audible level of loudness  at every point of the hall and then quickly dies away to make room ready for the next syllable or group of notes. Following are the factors affecting architectural acoustics.

1. REVERBERATION

In a hall, if the reverberation is large there is of successive sounds which result in loss of clarity in hearing. However if the reverberation is very small, the loudness is inadequate Thus the time of reverberation for a hall should neither too large nor too small. The preferred value of the time of reverberation is called optimum the reverberation time. According to W. C. Sabine standard reverberation time is given by:

T =   0.165 V/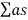

T =   0.165 V/ A

Where V-Volume of hall in m3

a - Absorption coefficient

S - Area of reflecting surface in a square meter

Absorption of hall

The reverberation can be controlled by the following factors:

• By providing windows and ventilators which can be opened and closed to make the optimum time of reverberation
• Decorating the walls by pictures and maps
• Using heavy curtains with folds
• The walls are lined with absorbent material such as felt, fibreboard, glass wool etc.
• Having full capacity of audience
• By covering floor with carpet
• By providing acoustics tiles

2.     ADEQUATE LOUDNESS

With large absorption the time of reverberation will be smaller which will minimize the chances of confusion and may go below the level of intelligibility of hearing. Hence suffi­cient loudness in every portion of the hall is an important factor for satisfactory hearing.

The loudness can he maintained at desired level by:

Using large sounding boards behind the speaker and facing the audience.

Large polished wooden reflecting surfaces immediately above the speakers.

Low ceiling are also useful in reflecting the sound energy towards the audience.

By providing additional sound energy using more number of speakers

3.     FOCUSING DUE TO WALLS AND CEILINGS

If there are focusing surfaces like concave, spherical, cylindrical or parabolic etc. on the walls or ceiling or the floor of the hall, they produce concentration of the sound in to particular region, while in some other parts no sound reaches at all. Thus there will be non-­ uniformity in the distribution of sound energy in the hall.

For uniform distribution of sound in the hall:

• There should be no curved surfaces. If such surfaces are present, they should be covered with absorbent material.
• Ceiling should be low. 
• Arrange speaker at the focus of parabolic reflecting surface. This will helpful to reflect beam of sound in the hall.

4.     ECHOES

An echo is heard, when direct and reflected sound waves coming from the same source reach the listener with time interval of about 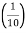th second. It should be avoided as far as possible by absorption.

Echoes can he avoided by:

• Covering long distant walls with curtain or absorbent material
• Covering high ceiling with absorbent material

5.     ECHELON EFFECT

A set of railings, pillars or any regular spacing of reflected surfaces may produce a musical note due to regular succession of the echoes of the original sound to the listener. This makes the original sound confused.

This can be avoided by:

• Covering steps with carpet
• Covering flour with carpet
• Avoid pillars in the hall

6.     BALCONIES

There are chances of reflection of sound from the railing of balcony. This may lead to the problem like echelon effect or echoes.

This can be eliminated by:

• Adjust height to depth ratio as 2: 1
• Use grills and bars for railings instead of bricks

7.     SEATING ARRANGEMENT

This is one of the factors to be taken care at the time of arranging the seats.

It pre­ferred to arrange:

• Seats perpendicular to the direction of sound for better audibility
• Seats must be gradually elevated to take care of absorption of sound energy by human body.

8.     EXTRANEOUS NOISE AND SOUND INSULATION

In a good hall no noise should reach from outside. Noise may be defined as unwanted sound such as:

Outside Noise: street traffic, hammering, drilling, operating machinery, moving of furni­ture, electrical generator etc.

Inside Noise: machinery, typewriters, telephone, mobiles, projector etc.

This extraneous noise can be avoided by:

• Avoiding openings for pipes and ventilators
• Allotting suitable locations for doors and windows
• Using heavy glasses to doors and windows
• By providing double wall construction with air space between them
• By interposing layers of some acoustical insulators
• Use of soft floor finish e.g. Carpet, rubber etc.
• Insulating machines like refrigerators, lifts, typewriters, projector etc.
• Constructing small sound proof cabin for machine and office staff
• Making hall sound proof

9.     FREEDOM FROM RESONANCE

If the frequency of the created sound is equal to original sound, then the original music will be reinforced. Due to the interference between original sounds is distorted. Enclosed air in the hall also causes resonance.

This can be avoided by:

• Using absorbing material on reflecting surfaces
• Providing decoration which include holes in the design on interior wall
• Using ventilators whenever necessary

10 Question: Derive differential equation for mechanical simple harmonic oscillators?

Solution:

The figure-1 depicts simple mechanical oscillator having mass attached to the spring. This structure is similar to Pendulum. It is also known as harmonic oscillator.

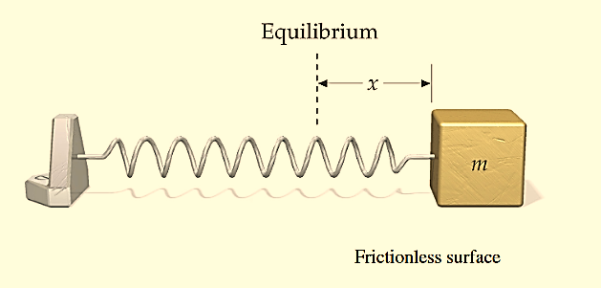
Figure: 1
 

If a body attached to a spring is displaced from its equilibrium position, the spring exerts a restoring force on it, which tends to restore the object to the equilibrium position. This force causes oscillation of the system, or periodic motion.
When the restoring force is directly proportional to the displacement from equilibrium, the resulting motion is known as simple harmonic motion and the oscillator is known as Harmonic Oscillator.

In this type of system body itself changes its position.

For mechanical oscillation two things are especially responsible i.e. Inertia & Restoring force.

Differential Equation

A particle is said to be execute simple harmonic oscillation is the restoring force is directed towards the equilibrium position and its magnitude is directly proportional to the magnitude and displacement from the equilibrium position. If F is the restoring force on the oscillator when its displacement from the equilibrium position is x, then

F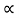–x

Here, the negative sign implies that the direction of restoring force is opposite to that of displacement of body i.e. towards equilibrium position.

F= -kx ............. (1)

Where, k= proportionality constant called force constant.

An example of such a simple system is the mass m, attached to a spring of stiffness k.

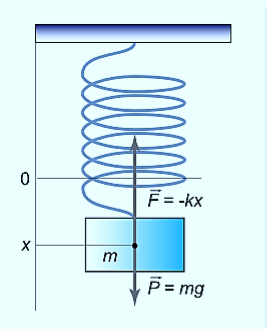
Figure: 2
 

In the case of a mass on a spring, the restoring force for small oscillations obeys Hooke’s law:

F=−kx,

Where k is the stiffness of the spring. Here the coordinate x=0 corresponds to the point of equilibrium, in which the force of gravity is balanced by the initial tension of the spring.

Then, according to Newton’s second law, the movement of the mass will be described by the differential equation

Mx′′=−kx,

⇒x′′+ x=0.

Solution of differential equation is

x= Acosωt

Thus, the mass on the spring will perform undamped harmonic oscillations with the circular frequency

ω=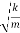

The period of oscillation, respectively, will be equal to

T= 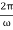= 2π

A similar analysis of other oscillatory system – a simple (mathematical) pendulum – leads to the following formula for the oscillation period:

T=2π

Where L is the length of the pendulum, g is the acceleration of gravity.

In the case of a compound or physical pendulum, the period of oscillation is given by

T=2π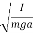

Where I is the moment of inertia of the pendulum about the pivot point, m is the mass of the pendulum, a is the distance between the pivot point and the center of mass of the pendulum.

Example: oscillation of mass spring system, oscillation of fluid-column in a U-tube, oscillation of simple pendulum, rotation of earth around the sun, oscillation of body dropped in a tunnel along earth diameter, oscillation of floating cylinder, oscillation of a circular ring suspended on a nail, oscillation of atoms and ions of solids, vibration of swings etc.

11 Question: Discuss heavy, critical and light damping?

Solution:

In real systems, there is always a resistance or friction, which leads to a gradual damping of the oscillations. In many cases, the resistance force (denoted by FC) is proportional to the velocity of the body, that is

FC=−cx′.

Then, taking into account the force of resistance, the differential equation for the “mass-spring” system is written as

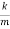Mx′′+cx′+kx=0, ⇒ x′′+ x′+x=0

We introduce the following notations: =2β, 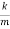= . Here ω0 is the natural frequency of the undamped oscillator (previously, we denoted it as ω), β is the damping coefficient. In the new notations, the differential equation looks like

x′′+2βx′+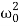x=0.

We will seek the solution of this equation as a function

x(t)=Aeλt.

The derivatives are given by

x′(t)=Aλeλt,x′′(t)=Aλ2eλt.

Substituting this into the differential equation, we obtain the algebraic characteristic equation:

Aλ2eλt+2βAλeλt+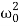Aeλt=0,

⇒λ2+2βλ+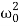 =0.

The roots of this equation are

D=4β2−4,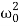

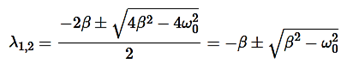
It can be seen that depending on the sign of the radicand β2−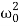, there may be three different types of solutions.

Case 1. Overdamping or Heavy Damping: β>ω0

When resistance to motion is.very strong, the system is said to be heavily damped. Can you name a heavily damped system of practical interest? Springs joining wagons of a train constitute the most important heavily damped system. In your physics laboratory, vibrations of a pendulum in a viscous medium such as thick oil and motion of the coil of a dead beat galvanometer are heavily damped systems.

In this case (the case of strong damping), the radicand is positive: β2>. The roots of the characteristic equation are real and negative. The general solution of the differential equation has the form

x(t)=C1eλ1t+C2eλ2t,

Where the coefficients C1, C2, as usual, depend on the initial conditions.

It follows from this expression that there are no oscillations and the system returns to equilibrium exponentially, i.e. aperiodically (Figure 9).

Figure 9

Case 2. Critical Damping: β=ω0

You may have observed that on hitting an isolated road bump, a car bounces up and down and the occupants feel uncomfortable. To minimise this discomfort, the bouncing caused by the road bumps must be damped very rapidly and the automobile is restored to equilibrium quickly. For this we use critically damped shock absorbers. Critical damping is also useful in recording instruments such as a galvanometer (pointer type as well as suspended coil type) which experience sudden impulses. We require the pointer to move to the correct position in minimum time and stay there without executing oscillations. Similarly, a ballistic galvanometer coil is required to return to zero displacement immediately.

In the limiting case when β=ω0, the roots of the characteristic equation are real and coincide:

λ1=λ2=−β=−ω0.

Here the solution is given by the formula

x(t)=(C1t+C2)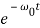

In this mode, the value of x(t) may even increase at the beginning of the process because of the linear factor C1t+C2. But in the end the deflection x(t) decreases rapidly due to the exponential decay with a characteristic time τ=2π/ω0.

Note that in this critical mode the relaxation occurs faster than in the case of the aperiodic damping (Case 1). Indeed, in this mode the relaxation time will be determined by the smaller (in absolute value) root λ1, and will be given by the formula

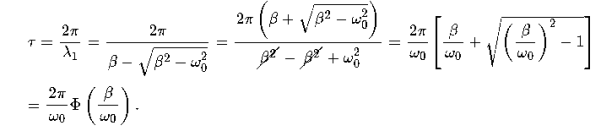
The function Φ(β/ω0) included in this expression is monotonically increasing. It is always greater than or equal to 1, as shown in Figure 10

Figure 10

In the critical case (Case 2) the ratio βω0 is 1, and βω0>1 in the case of the aperiodic damping (Case 1). Therefore for the aperiodic damping mode, we can write

τ= Φ() >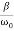

Thus, the critical damping mode provides the fastest possible return of the system to equilibrium. This is often used, for example, in door closing mechanisms.

Case 3. UnderdampingB  or Light Damping β<ω0

Here the roots of the characteristic equation are complex conjugate:

λ1,2 =−β±i√

The general solution of the differential equation is oscillatory in nature and can be written as

x(t)=e−βt[C1cos(ω1t)+C2sin(ω1t)]

Where the oscillation frequency ω1 is equal to

ω1=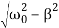

The resulting formula can be written in a somewhat different form:

x(t)=Ae−βtcos(ω1t+φ0),

Where φ0 is the initial phase of the oscillations and Acosφ0 is the initial amplitude of the oscillations. We see that classical damped oscillations occur in this mode. Here the oscillation frequency ω1 is less than the harmonic frequency ω0, and the oscillation amplitude decreases exponentially with e−βt.

12 Question: Define vibration? What is the difference between free and forced Vibrations?

Solution:

Vibration is periodic back-and-forth motion of the particles of an elastic body or medium, commonly resulting when almost any physical system is displaced from its equilibrium condition and allowed to respond to the forces that tend to restore equilibrium.

Free Vibrations

Definition- A system is said to execute free vibrations if on being disturbed from its position of equilibrium, it vibrates itself without outside interference

Free vibrations occur when the system is disturbed momentarily and then allowed to move without restraint. A classic example is provided by a weight suspended from a spring. In equilibrium, the system has minimum energy and the weight is at rest. If the weight is pulled down and released, the system will respond by vibrating vertically.

A free vibration is where there is no externally applied vibration forcing. The solution to a free vibration is usually roughly sinusoidal. Of course, vibrations can occur across a whole spectrum of frequencies. There will usually be one or more frequencies where there is growing amplitude. At the limit of stability, a free vibration has constant amplitude. It neither grows nor decays.

The vibrations of a spring are of a particularly simple kind known as simple harmonic motion (SHM). This occurs whenever the disturbance to the system is countered by a restoring force that is exactly proportional to the degree of disturbance. In this case, the restoring force is the tension or compression in the spring, which (according to Hooke’s law) is proportional to the displacement of the spring. In simple harmonic motion, the periodic oscillations are of the mathematical form called sinusoidal. One characteristic of SHM is that the period of the vibration is independent of its amplitude.

Forced vibrations

Definition- A system is said to execute forced vibrations when it is compelled or forced to vibrate with a frequency other than its natural frequency.

Forced vibrations occur if a system is continuously driven by an external agency. A simple example is a child’s swing that is pushed on each downswing. Of special interest are systems undergoing SHM and driven by sinusoidal forcing.

We have learnt that the amplitude of the oscillations of a system or a body executing damped simple harmonic oscillator goes on decreasing and ultimately vanish with the passage of time. The oscillation of such a body can be maintained by applying an external periodic force. 

When a body vibrates by being influenced by an external periodic force, it is called forced vibrations Here, the amplitude of vibrations, experiences damping but remains constant due to the external energy supplied to the system.

Sinusoidal vibration forcing of a stable system produces steady vibration amplitude. However, if the system is forced with a frequency at the limit of stability, vibration amplitude will grow to infinity or until limited by non-linearity. We may therefore consider limiting stability to be unstable in practice.

For example – A child’s swing comes to rest unless external force applied at proper and regular interval of time to make the swing keep on swinging with a large amplitude. The same is the case with a pendulum or a vibrating mass attached to a spring. The applied periodic force is called the Driving Force and the oscillating system is called the Driven Oscillator.

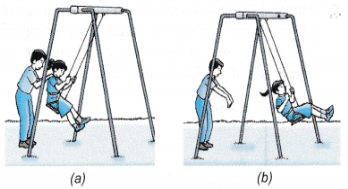
Figure: 3
 

13 Question: What do you meant by Resonance?

Solution:

Resonance occurs when:-

Frequency of the external periodic force = Natural frequency of the system.

Definition:- The phenomena of producing vibratory motion in a system by the influence of an external periodic force having the same frequency as that of natural frequency of the system is called Resonance.

Free vibrations are oscillations where the total energy stays the same over time. This means that the amplitude of the vibration stays the same. This is a theoretical idea because in real systems the energy is dissipated to the surroundings over time and the amplitude decays away to zero, this dissipation of energy is called damping.

Example:- Resonance causes disaster during earthquake. When the natural frequency of the building becomes equal to the frequency of the periodic vibration present in the earth during earthquake, then the building starts vibrating with large amplitude and hence it collapses.

14 Question: Difference between Undamped Vibration and Damped Vibration?

Solution:

Undamped Vibration

Undamped Vibration refers to vibrations where energy of the vibrating object does not get dissipated to surroundings over time.

If there is no external force applied on the system, f(t) =0, the system will experience free vibration. Motion of the system will be established by an initial disturbance (i.e. initial conditions).

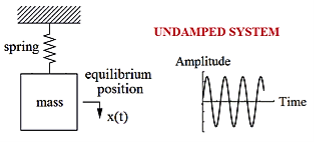
Figure: 4
 

Furthermore, if there is no resistance or damping in the system, the oscillatory motion will continue forever with constant amplitude. Such a system is termed undamped.

 Damped Vibration

When the energy of a vibrating system is gradually dissipated by friction and other resistances, the vibrations are said to be damped. The vibrations gradually reduce or change in frequency or intensity or cease and the system rests in its equilibrium position.

Damped vibration refers to vibrations where the vibrating object loses its energy to the surroundings.

Damping, in physics, restraining of vibratory motion, such as mechanical oscillations, noise, and alternating electric currents, by dissipation of energy. Unless a child keeps pumping a swing, its motion dies down because of damping. Shock absorbers in automobiles and carpet pads are examples of damping devices.

A system may be so damped that it cannot vibrate. Critical damping just prevents vibration or is just sufficient to allow the object to return to its rest position in the shortest period of time.

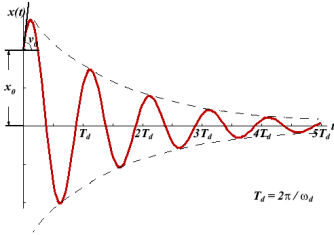
Figure: 5

There are many types of mechanical damping. Friction, also called in this context dry, or Coulomb, damping, arises chiefly from the electrostatic forces of attraction between the sliding surfaces and converts mechanical energy of motion, or kinetic energy, into heat. The motion of a vibrating body is also checked by its friction with the gas or liquid through which it moves.

15 Question: Discuss force vibration and amplitude resonance, velocity resonance and energy intake?

Solution:

Forced vibration without damping

Force Applied to Mass. When the sinusoidal force F=F0sinωt is applied to the mass of the undamped single degree-of-freedom system shown in Figure 8.

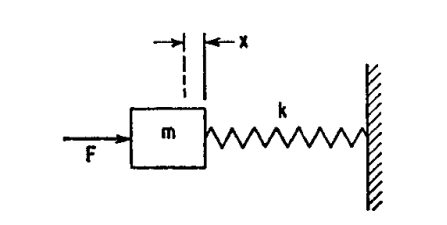
Figure 8: Undamped single degree-of-freedom system excited in forced vibration by force acting on mass.

The differential equation of motion is

m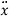+kx=F0sinωt..………..(1)

The solution of this equation is

X = Asinωnt + Bcosωnt+ 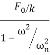sinωt..………..(2)

Where ωn=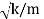

The first two terms represent an oscillation at the undamped natural frequency ωn. The coefficient B is the value of x at time t=0, and the coefficient A may be found from the velocity at time t=0.

Differentiating Equation (2) and setting t=0,

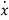  (0)=Aωn+ 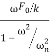…………..(3)

The value of A is found from Equation (3).

The oscillation at the natural frequency ωn gradually decays to zero in physical systems because of damping. The steady-state oscillation at forcing frequency ω is

x= sinωt……………(4)

This oscillation exists after a condition of equilibrium has been established by decay of the oscillation at the natural frequency ωn and persists as long as the force F is applied.

The force transmitted to the foundation is directly proportional to the spring deflection: Ft=kx. Substituting x from Equation (4) and defining transmissibility T=Ft/F,

T= ……….…(5)

If the mass is initially at rest in the equilibrium position of the system (i.e., x=0 and 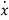=0) at time t=0, the ensuing motion at time t>0 is

x= (sinωt− 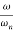sinωnt)…………(6)

For large values of time, the second term disappears because of the damping inherent in any physical system, and Equation (6) becomes identical to Equation (4).

When the forcing frequency coincides with the natural frequency, ω=ωn and a condition of resonance exists. Then Eq. (6) is indeterminate and the expression for x may be written as

X =− 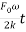cos ωt+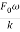sin ωt……………(7)

According to Equation (7), the amplitude x increases continuously with time, reaching an infinitely great value only after an infinitely great time.

Motion of Foundation

The differential equation of motion for the system of Figure 9 excited by a continuing motion u=u0sinωt of the foundation is

m = −k(x−u0sin ωt)

The solution of this equation is

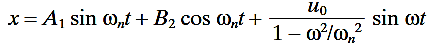
Where ωn=k/m and the coefficients A1,B1 are determined by the velocity and displacement of the mass, respectively, at time t=0. The terms representing oscillation at the natural frequency are damped out ultimately, and the ratio of amplitudes is defined in terms of transmissibility T:

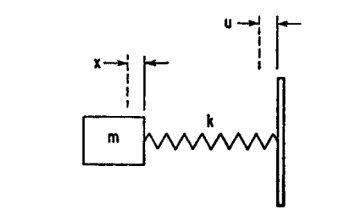
Figure 9: Undamped single degree-of-freedom system excited in forced vibration by motion of foundation.

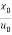= T = T=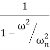

Where x=x0sinωt.Thus, in the forced vibration of an undamped single degree-of-freedom system, the motion response, the force transmissibility, and the motion transmissibility are numerically equal.

Forced vibration with viscous damping

Force Applied to Mass.

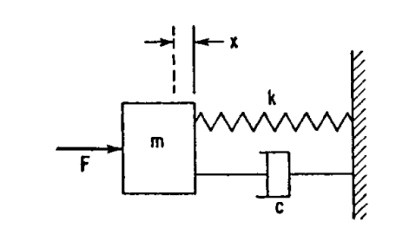
Figure 10: Single degree-of-freedom sys-tem with viscous damping, excited in forced vibration by force acting on mass

The differential equation of motion for the single degree-of-freedom system with viscous damping shown in Figure 10, when the excitation is a force F=F0sinωt applied to the mass, is

m+c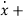kx=F0sinωt..………..(8)

Equation (8) corresponds to Equation (1) for forced vibration of an un-damped system; its solution would correspond to Eq. (2) in that it includes terms representing oscillation at the natural frequency. In a damped system, however, these terms are damped out rapidly and only the steady-state solution usually is considered. The resulting motion occurs at the forcing frequency ω; when the damping coefficient c is greater than zero, the phase between the force and resulting motion is different than zero. Thus, the response may be written

x=Rsin(ωt−θ) =A1sin ωt+B1cos ωt..………..(9)

Substituting this relation in Eq. (8), the following result is obtained:

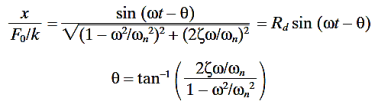..………..(10)

And Rd is a dimensionless response factor giving the ratio of the amplitude of the vibratory displacement to the spring displacement that would occur if the force F were applied statically. At very low frequencies Rd is approximately equal to 1; it rises to a peak near ωn and approaches zero as ω becomes very large. The displacement response is defined at these frequency conditions as follows:

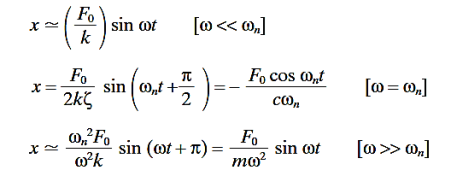..………..(11)

For the above three frequency conditions, the vibrating system is sometimes described as spring-controlled, damper-controlled, and mass-controlled, respectively, depending on which element is primarily responsible for the system behaviour. Curves showing the dimensionless response factor Rd as a function of the frequency ratio ω/ωn are plotted in Figure 11 on the coordinate lines having a positive 45° slope. Curves of the phase angle θ are plotted in Figure 12. A phase angle between 180 and 360°cannot exist in this case since this would mean that the damper is furnishing energy to the system rather than dissipating it. An alternative form of Equations (10) and (11) is

…………..(12)

This shows the components of the response which are in phase [(Rd)xsin ωt] and 90°out of phase [(Rd)Rcosωt] with the force. Curves of (Rd)x and (Rd)R are plotted as a function of the frequency ratio ω/ωn in Figures 13 and 14.

Velocity and Acceleration Response

The shape of the response curves changes distinctly if velocity ̇x or acceleration ̈x is plotted instead of displacement x. Differentiating Equation (10),

………………(13)

The acceleration response is obtained by differentiating Equation (13)

……………….(14)

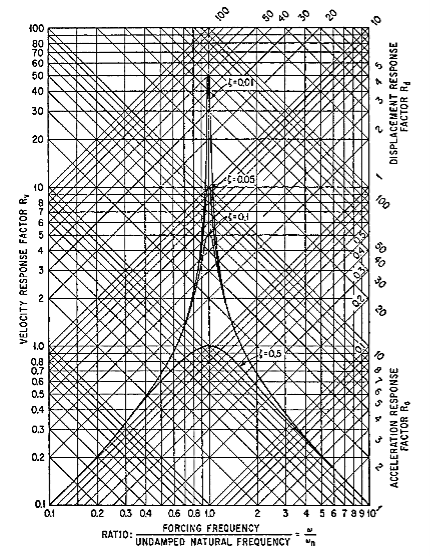
Figure: 11

Response factors for a viscous-damped single degree-of-freedom system excited in forced vibration by force acting on the mass. The velocity response factor shown by horizontal lines is defined by Eq. (13); the displacement response factor shown by diagonal lines of positive slope is defined by Eq. (10); and the acceleration response factor shown by diagonal lines of negative slope is defined by Equation (14).

The velocity and acceleration response factors defined by Equations. (13) and (14) are shown graphically in Figure 11, the former to the horizontal coordinates and the latter to the coordinates having a negative 45°slope. Note that the velocity response factor approaches zero as ω→0 and ω→∞, whereas the acceleration response factor approaches 0 as ω→0 and approaches unity as ω→∞

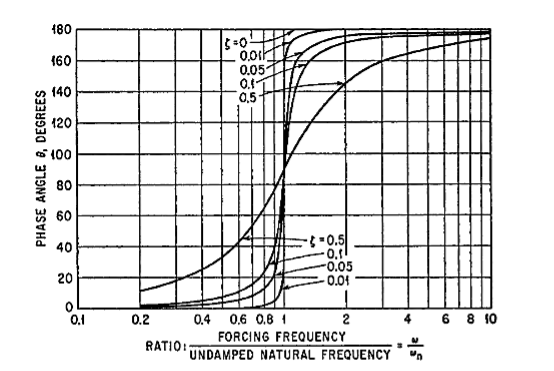
Figure 12:

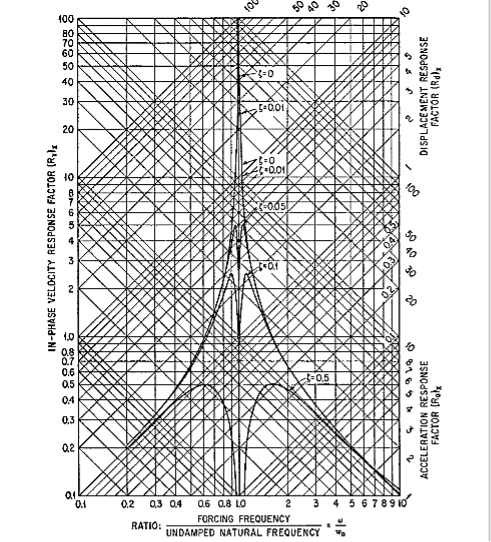
Figure 13:  In-phase component of response factor of a viscous-damped system in forced vibration. All values of the response factor for ω/ωn>1 are negative but are plotted without regard for sign. The fraction of critical damping is denoted by ζ.

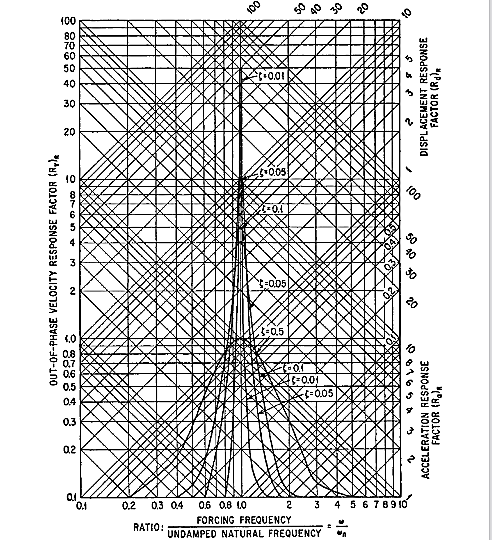
Figure 14: Out-of-phase component of response factor of a viscous-damped system in forced vibration. The fraction of critical damping is denoted by ζ.

Resonance Frequencies

The peak values of the displacement, velocity, and acceleration response of a system undergoing forced, steady-state vibration occur at slightly different forcing frequencies. Since a resonance frequency is defined as the frequency for which the response is a maximum, a simple system has three resonance frequencies if defined only generally. The natural frequency is different from any of the resonance frequencies. The relations among the several resonance frequencies, the damped natural frequency, and the undamped natural frequency ωn are:

Displacement resonance frequency: ωn(1 −2ζ2)1/2

Velocity resonance frequency: ωn

Acceleration resonance frequency: ωn/(1 −2ζ2)1/2

Damped natural frequency: ωn(1 −ζ2)1/2

For the degree of damping usually embodied in physical systems, the difference among the three resonance frequencies is negligible

Energy Intake

When the viscous damped, single degree-of-freedom system shown in Figure 10 undergoes vibration defined by

x=x0sin ωt……………(15)

The net force exerted on the mass by the spring and damper is

F=kx0sin ωt+cωx0cos ωt……………(16)

Equations (15) and (16) define the relation between F and x; this relation is the ellipse shown in Figure 15.

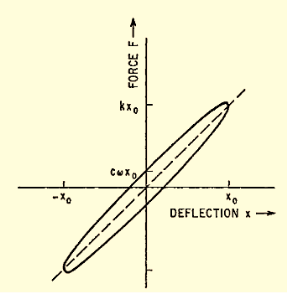
Figure 15: Hysteresis curve for a spring and viscous damper in parallel.

The energy dissipated in one cycle of oscillation is

W =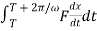 =πcω

16 Question: Discuss Longitudinal waves and Transverse waves?

Solution:

There are two types of waves:

• Longitudinal waves
• Transverse waves

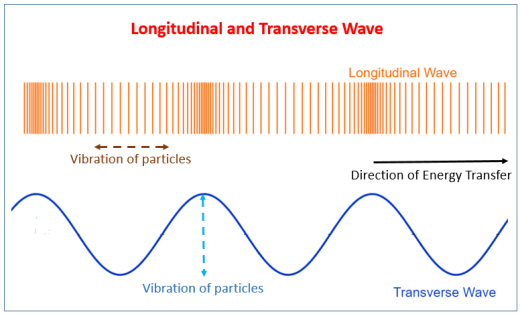
Figure: 16

Longitudinal Waves: A wave in which the particles of the medium vibrate back and forth in the ‘same direction’ in which the wave is moving.  Medium can be solid, liquid or gases. Therefore, sound waves are longitudinal waves. These waves travel in the form of compressions and rarefactions.

Longitudinal waves have the same direction of vibration as their direction of travel. This means that the movement of the medium is in the same direction as the motion of the wave. Some longitudinal waves are also called compressional waves or compression waves. An easy experiment for observing longitudinal waves involves taking a Slinky and holding both ends. After compressing and releasing one end of the Slinky (while still holding onto the end), a pulse of more concentrated coils will travel to the end of the Slinky. In the example of the Slinky, each coil will oscillate at a point but will not travel the length of the Slinky. It is important to remember that energy, in this case in the form of a pulse, is being transmitted and not the displaced mass.

Longitudinal waves can sometimes also be conceptualized as pressure waves. The most common pressure wave is the sound wave. Sound waves are created by the compression of a medium usually air. Longitudinal sound waves are waves of alternating pressure deviations from the equilibrium pressure, causing local regions of compression and rarefaction. Matter in the medium is periodically displaced by a sound wave, and thus oscillates. When people make a sound, whether it is through speaking or hitting something, they are compressing the air particles to some significant amount. By doing so, they create transverse waves. When people hear sounds, their ears are sensitive to the pressure differences and interpret the waves as different tones.

Transverse Waves: A wave in which the particles of the medium vibrate up and down ‘at right angles’ to the direction in which the wave is moving. These waves are produced only in a solids and liquids but not in gases. These waves travel in the form of crests and troughs. If a transverse wave is moving in the positive x-direction, its oscillations are in up and down directions that lie in the y–z plane. Light is an example of a transverse wave. For transverse waves in matter, the displacement of the medium is perpendicular to the direction of propagation of the wave. A ripple on a pond and a wave on a string are easily visualized transverse waves.

Transverse waves are waves that are oscillating perpendicularly to the direction of propagation