Module 3

Intermolecular forces and Potential Energy Surfaces

Question Bank

1. Explain the iconic interactions?

Ionic interactions are the interactions between charged molecules or atoms (ions).Positively, charged ions, are Na (+), Li (+), and Ca (+), they are called as Cations, on the other hand the negatively charged ions are Cl (-), Br (-), Ho (-) they are Anions. The attractive forces between ions that are oppositely charged is described under the Coulomb’s Law, as the law says the force increases with charge and decreases as the distance between these ions increases. The highly polarised or charged nature of the ionic molecules is reflected in the nature of their melting point. For e.g. NaCl has a melting point of 801oc. The ionic molecules also show the property of high solubility in water.

2.                 Explain H2 bonding with an example?

Hydrogen bonding occurs in molecules that are highly electromagnetic in nature, elements like F, O or N are directly bound to hydrogen. As hydrogen has an electronegativity of 2.2, they are not as polarized as ionic bonds and have some covalent character. But still the hydrogen bond is polarized and possess a dipole. Hydrogen bonding is an attractive interaction that occurs when the dipole of one molecule can align with the dipole from another molecule. Since the molecular motion of these molecules are rapid in a solution, these bonds are short lived therefore HO and NH are bonding molecules containing these functional groups that tend to have high boiling point.

3.                 What do you understand by dipole and van der waals interaction?

Other groups other than hydrogen can be involved in covalent bonding with strong electronegative atoms. For example, in the figure given below each of the molecules contain a dipole: Dipoles are created by differences in electronegativities between nearby atoms

• Interactions between opposite-charged dipoles are attractive
• Similar in origin to hydrogen bonding, but since the differences in electronegativities are smaller
• Carbon is more electronegative (2.5) than hydrogen (2.2): the magnitudes of the dipoles are smaller, and these interactions are weaker.

These dipoles interact with each other in a very unique and attractive manner and thereby increase the boiling point. But the main aspect is the bonding depends on the electronegativity differences. The electronegativity of carbon =2.5, but that of Oxygen and Nitrogen is less than hydrogen whose electronegativity is=2.2, therefore the polar interaction is not so very strong. So, on an average these forces tend to be weaker than in hydrogen bonding

To sum up, boiling points are a measure of intermolecular forces and boiling point increases with molecular weight and surface area. The intermolecular forces increase with the increase in polarization of bonds.

4.                 Define real gases?

A real gas is a gas that does not behave as an ideal gas due to interactions between gas molecules. A real gas is also known as a nonideal gas because the behaviour of a real gas in only approximated by the ideal gas law.

5.                 Explain two difference between real gases and ideal gases?

Ideal gases do not have intermolecular forces and the gas molecules considered as point particles. In contrast real gas molecules have a size and a volume. Further they have intermolecular forces.

Ideal gases cannot be found in reality. But gases behave in this manner at certain temperatures and pressures.

Gases tend to behave as real gases in high pressures and low temperatures. Real gases behave as ideal gases at low pressures and high temperatures.

Ideal gases can be related to the PV=nRT=NkT equation, whereas real gases cannot. For determining real gases, there are much more complicated equations.

6.                 Mention the factors that deviate from the ideal behavior?

The behavior of real gases usually agrees with the assumptions of the ideal gas equation to within 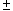5% at normal temperatures and pressures. At low temperatures or high pressures, real gases deviate significantly from ideal gas behaviour. In 1873, while searching for a way to link the behaviour of liquids and gases, the Dutch physicist Johannes van der Waals developed an explanation for these deviations and an equation that was able to fit the behaviour of real gases over a much wider range of pressures. Van der Waals stated the fact that the volume of a real gas is too large at high pressures by deletinga term from the volume of the real gas before we substitute or add it into the ideal gas equation.

7.                 Derive the van der waals gas equation?

In a real gas the molecular volume is not negligible, also cohesive or repulsive intermolecular forces mean that the pressure applied on the containing vessel is less than that of an Ideal gas. Therefore, the equation of state needs that the pressure p is increased by a quantity proportional to the density or, by the quantity inversely proportional to the volume. The van der Waals equation has played an important role in describing fluids, i.e. both liquids and gases.

Van der Waals equation is also known as Van der Waals equation of state for real gases and is not applicable or follows ideal gas law. According to the state of ideal gas law, PV = nRT where P is the pressure, V is the volume, n is the number of moles, T is the temperature and R is the universal gas constant. The Van der Waals Equation derivation is explained below.

Derivation of Van der Waals equation

For the state of real gas, using Van der Waals equation, the volume of a real gas is given as (Vm – b), where b is volume occupied by per mole.

Therefore, ideal gas law when substituted with V = Vm – b is given as:

P(Vm−b) =nRT

Because of intermolecular attraction P was modified as below

(P+a/Vm2) (Vm−b) =RT 

(P+an2/V2) (V−nb) =nRT

Where,

Vm: molar volume of the gas

R: universal gas constant

T: temperature

P: pressure

V: volume

Thus, Van der Waals equation can be reduced to ideal gas law as PVm = RT.

8.                 Explain the reasons for using constants in van der waals gas equation?

The Van der Waal equation for real gas is:

[P+an^2/V^2][V-nb]

Here a and b are Van der Waal constant….

Volume and pressure correction are done because of following reasons:

• In a real gas the molecular volume cannot be ignored. Here we take the volume of the container but we know that gas can be compressed easily.
• So, there should be some excluded gas. Hence, for a real gas of n no. Of moles the volume excluded is (V- nb ).
• Now for pressure correction
  • Assume that real gas exerts the pressure'P' . The molecule that exert the force on the container will get attracted by molecule
  • It can be seen that the pressure of real exert would be less than the pressure of ideal gas.
  • Therefore, a real gas exerts a pressure 'P' then an ideal gas would exert a pressure [ P+ an^2/V^2]

9.                 What is the significance of van der waals gas equation?

The van der Waals equation is a state equation for real gases that modifies the ideal gas equation (PV = north) in order to consider intermolecular interactions. These interactions result from molecules attracting each other when they are approaching, and repelling each other when they are colliding.

10.             What are the limitations of van der waals equation?

(I) The value of ‘b’ is not constant but varies with pressure and temperature.

(ii) The value of 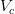 is not equal to 3b, but actually it is equal to, in some case; and in other cases, 2b.

(iii)  The value of 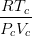 is not equal to 8/3but it is usually more than 3 for most of the gases.

11.  What do you understand by Potential energy surfaces explain with an example?

In a molecule, atoms are held together by chemical bonds, stretching or compressing occurs in bonds when bonds are distorted, thereby increasing the potential energy of its system. The molecule however remains stationary when a new geometry is formed. Therefore, the position of the atoms (potential)plays an important role in proving that the energy of the system is not caused by the kinetic energy, the position of the nuclei directly corresponds to the Energy of a molecule. The electrons however readjust quickly When nuclei show movement.  Thus, the relationship between this molecular energy and molecular geometry (position) is mapped out with potential energy surface.

Three-dimensional PES is used for systems that have three atoms; in this case the collision reaction of an atom is substituted a diatomic molecule. The very first study done on this topic is H3H3 atom. The reaction is

H+H2→H2+H(2)(2)H+H2→H2+H

In this case, the triatomic molecule is the H-H-H transition state. There occurs a barrier on the potential energy surface at this point as it may have a higher energy than the product and reactants. Analysis such as the Quantum mechanical and quasiclassical trajectories calculations are required for the PES of H3 system. The PES however does not dependent on the masses of the atoms, therefore, the H3 surface in this reaction can be used for any isotopic variant. As three nuclei (ABC) are involved, the PES depends on three coordinates. Therefore, one coordinate needs to be fixed in order to plot the PES as a function of the remaining two.