UNIT-4
Multiple Integrals
Question-1: Evaluate  , where dA is the small area in xy-plane.
, where dA is the small area in xy-plane.
Sol. Let,
I = 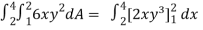
= 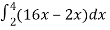
= 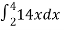
= 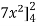
= 84 sq. Unit.
Which is the required area.
Question-2: Evaluate 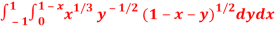
Sol. Let us suppose the integral is I,
I = 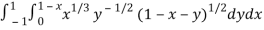
Put c = 1 – x in I, we get
I = 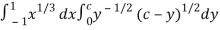
Suppose, y = ct
Then dy = c
Now we get,
I = 
I = 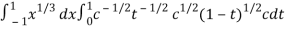
I = 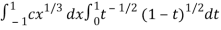
I = 
I = 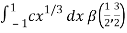
As we know that by beta function,
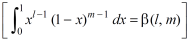
Which gives,

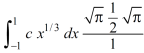
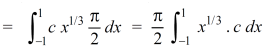
Now put the value of c, we get
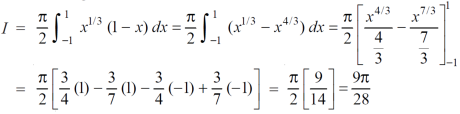
Question-3: Evaluate 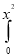 ey/x dy dx.
ey/x dy dx.
Soln. :
Given : I =  ey/x dy dx
ey/x dy dx
Here limits of inner integral are functions of y therefore integrate w.r.t y,
I = 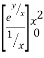
 dx
dx
= 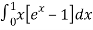
= 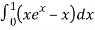
I = 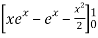
= =
 ey/x dy dx=
ey/x dy dx=
Question-4: Evaluate 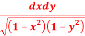
Soln.:
Let,I =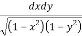
Here limits for both x and y are constants, the integral can be evaluated first w.r.t any of the variables x or y.
I = dy 
I= 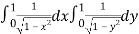
= 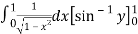
= 
= 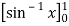
=
= 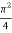
 =
=
Question-5: Evaluate
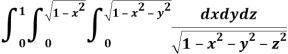
Solution:
Let
I = 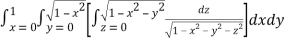
= 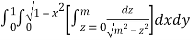
(Assuming m = 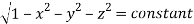 )
)
= 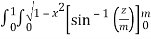 dxdy
dxdy
= 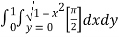
=
= 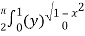 dx
dx
= 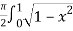 dx
dx
= 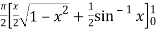
=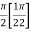
I =
Question-6:Evaluate 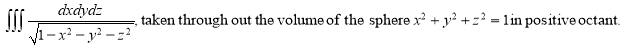
Solution:- 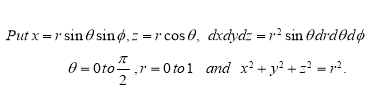
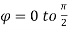

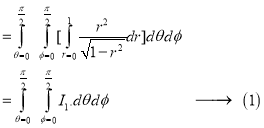
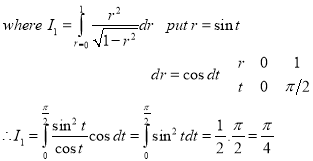
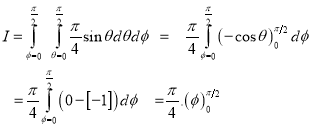
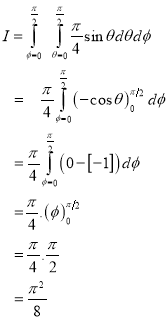
Question-7: Find volume common to the cylinders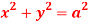 ,
,  .
.
Solution: For given cylinders,
 ,
,  .
.
Z varies from
Z=-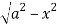 to z =
to z = 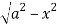
Y varies from
y= -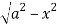 to y =
to y = 
x varies from x= -a to x = a
By symmetry,
Required volume= 8 (volume in the first octant)
=8 
=8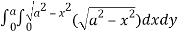
= 8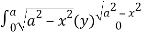 dx
dx
=8
=8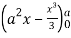
=8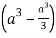
Volume = 16
Question-8: Change the order of integration in the double integral-
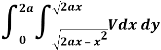
Sol.
Limits are given-
x = 0, x = 2a
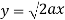
And
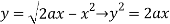
And
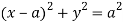
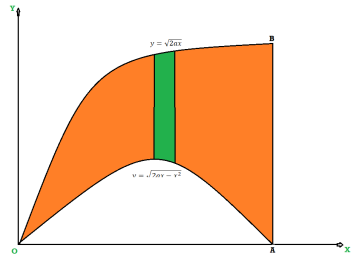
The area of integration is the shaded portion of OAB. On changing the order of integration first we will integrate with respect to x, the area of integration has three portions BCE, ODE and ACD,
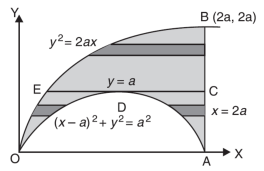
Now-
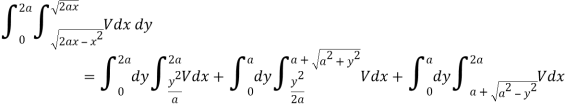
Which is the required answer.
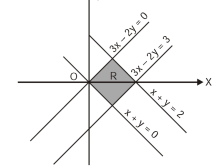
Question-9: Evaluate-
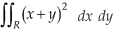
Where-
R is the region bounded by a parallelogram- x + y = 0, x + y = 2, 3x – 2y = 0, 3x – 2y = 3.
Sol.
By changing the variables x, y to the new variables u and v, by the substitution x + y = u, 3x – 2y = v, the given parallelolgram R reduces to a rectangle 
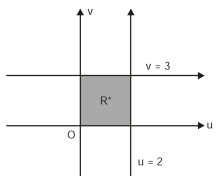

The required Jacobian is-
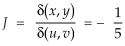
Since u = x + y and u = x + y = 2, here u varies from 0 to 2 while v varies from 0 to 3.
Since-
3x – 2y = v = 0, 3x – 2y = v = 3
Therefore the integral will be in new variables-
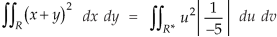
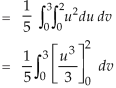
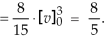
Which is the required answer.
Question-10: Evaluate 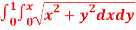 the transformation is x = v and y = uv.
the transformation is x = v and y = uv.
Sol.
Here the region of integration R is the triangle which is bounded by y = 0, x = 1 and y = x
Here put,
X = u and y = uv, we get-
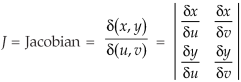
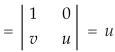
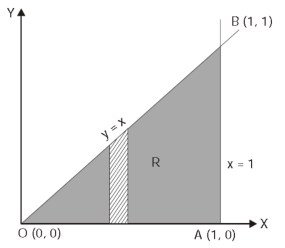
Here x varies from 0 to 1 while y varies from 0 to x.
Since u = x so u varies from 0 to 1
Here, similarly, since  , so that v varies from 0 to 1. Thus-
, so that v varies from 0 to 1. Thus-
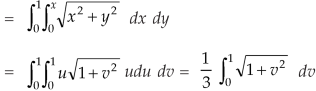
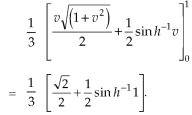
Question-11: Find the area between the parabola y ² = 4ax and another parabola x² = 4ay.
Sol. Let,
y ² = 4ax ………………..(1)
And
x² = 4ay…………………..(2)
Then if we solve these equations , w e get the values of points where these two curves intersect
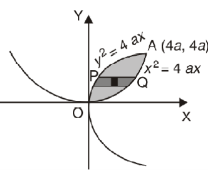
x varies from y²/4a to 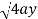 and y varies from o to 4a,
and y varies from o to 4a,
Now using the conceot of double integral,
Area = 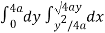

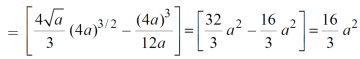
Question-12: Find Volume of the tetrahedron bounded by the co-ordinates planes and the plane
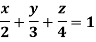
Solution: Volume =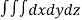 ………. (1)
………. (1)
Put  ,
, 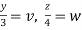
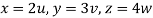
From equation (1) we have
V = 
=24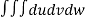
=24 (u+v+w=1) By Dirichlet’s theorem.
(u+v+w=1) By Dirichlet’s theorem.
=24 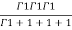
=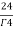 =
=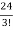 = 4
= 4
Volume =4
Question-13: Evaluate 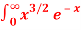 dx
dx
Solution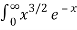 dx =
dx =  dx
dx
= γ(5/2)
= γ (3/2+ 1)
= 3/2 γ(3/2 )
= 3/2. ½ γ (½ )
= 3/2. ½ π
= ¾ π
Question-14: Evaluate 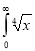
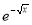 dx.
dx.
Solution: Let 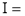
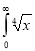
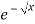 dx
dx
X | 0 | 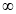 |
t | 0 | 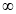 |
Put 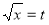 or
or 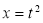 ;dx =2t dt .
;dx =2t dt .
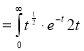 dt
dt
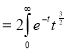 dt
dt
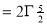
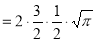
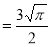
Question-15: Evaluate the integral-
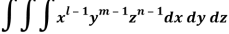
Where x, y and z are positive with conditions-
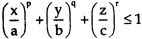
Sol. Let us put-
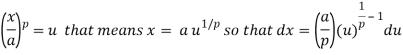
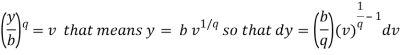
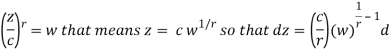
So that the required integral will be-
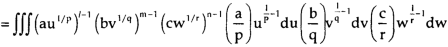

Where u + v + w ≤ 1
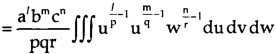
By Dirichlet’s integral-

Question-16: Show that-
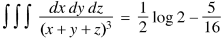
The conditions are given x + y = 0, y = 0, z = 0, x + y + z = 1.
Sol.
By Lioville’s theorem when 0 < x + y + z < 1
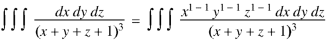

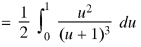
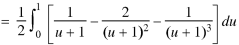
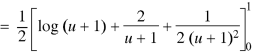
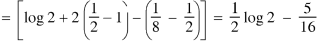
Proved.