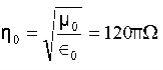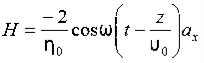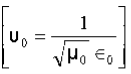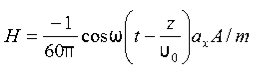IIMT UNIVERSITY
IIMT UNIVERSITY
UNIT- 2
ELECTROMAGNETIC
QUESTION BANK
1. Question: Calculate the skin depth for a frequency of 1010 Hz for silver. Given that  =2 x 107 Sm-1 and 4π x 10-7 m-1
=2 x 107 Sm-1 and 4π x 10-7 m-1
Solution:
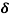 =
= 
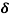 =
= 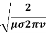
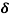 =
=
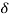 =1.125
=1.125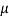 m.
m.
2. Question: Find the skin depth of copper if  =5.8 x 107 Sm-1 and
=5.8 x 107 Sm-1 and 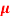 = 1.26
= 1.26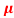 H per meter and n =50 Hz.
H per meter and n =50 Hz.
Solution:
Given 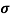 = 5.8 x 107 Sm-1
= 5.8 x 107 Sm-1
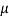 = 1.26
= 1.26 x 10-6 Hm-1
x 10-6 Hm-1
n =50 Hz
ω = 2πn =2 x 3.14 x 50 = 314.3
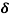 =
= 
 =
=  =
= = 9.33mm
= 9.33mm
3. Question: Solar Constant At the upper surface of the Earth’s atmosphere, the time-averaged magnitude of the Poynting vector, <S> = 1.35x103 W/m2, is referred to as the solar constant.
(a) Assuming that the Sun’s electromagnetic radiation is a plane sinusoidal wave, what are the magnitudes of the electric and magnetic fields?
(b)What is the total time-averaged power radiated by the Sun? The mean Sun-Earth distance is R= 1.50x1011m
Solution:
(a)The time-averaged Poynting vector is related to the amplitude of the electric field by
<S> = = 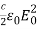
The amplitude of electric field is therefore
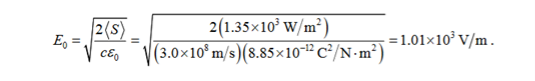
The amplitude of magnetic field can be obtained by
B0 = E0/ c
= 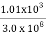 = 3.4 x 10-6 T
= 3.4 x 10-6 T
The associated magnetic field is one tenth of the earth’s magnetic field.
(b) The total time averaged power radiated by the Sun at the distance R is
<P> =<S>A =<S> 4πR2
=1.35x103 4 x 3.14 x (1.50x1011)2
= 3.8 x 1026 W
The type of wave discussed in the example above is a spherical wave, which originates from a “point-like” source. The intensity at a distance r from the source is which decreases is .
I =<S> = <P> /4πR2
Which decreases as 1/r2, On the other hand, the intensity of a plane wave remains constant and there is no spreading in its energy.
4. Question: Discuss continuity equation for current density
Solution:
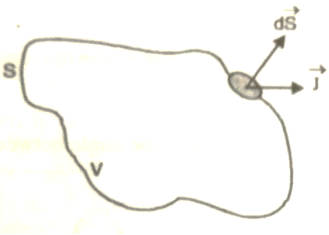
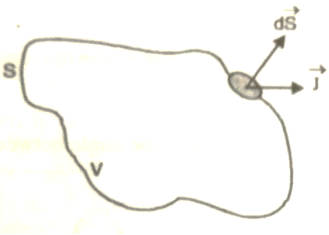
Consider a closed surface S enclosing a Volume V through which currents are flowing. Let I be the current passing through the surface at any time t. Consider a small area  of the closed surface. The outward current flowing through
of the closed surface. The outward current flowing through  at a time t is
at a time t is
DI = 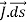 …………..(1)
…………..(1)
The total current flowing out through the closed surface S at time t is
I = 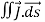 …………..(2)
…………..(2)
Suppose is ρ the Volume Charge density of charges inside the surface S. Then the total charge inside the Volume V is
q =  …………..(3)
…………..(3)
Since the current is flowing outward is it mean that charge within the enclosed surface is decreasing with time. The time rate of decrease of charge is
 = -
= -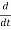
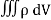 …………..(4)
…………..(4)
The negative sign shows that the charge inside the surface S is decreasing with time. The above equation can be written as
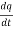 = -
= - 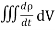 …………..(5)
…………..(5)
Since the charge is conserved, the current flowing outward through the closed surface must be equal to the rate of decrease of charge. Thus
I = 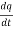
Using equation (2) and (5), it becomes
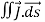 = -
= - 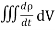 …………..(6)
…………..(6)
According to divergence theorem
 =
= 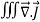 dV
dV
Using this result in equation (6) we obtain
 dV = -
dV = - 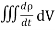
This equation holds good for arbitrary volumes. Therefore the integrands must be equal. Hence
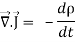
 =0 …………..(7)
=0 …………..(7)
This equation is called equation of continuity. It is the mathematical statement of law of conservation of charge.
SPECIAL CASE
Consider a conductor AB through which a steady current I is flowing. The current I is said to be steady if charge is flowing per unit time through any two any sections C and D is the same. This means that the total amount of charge entering the volume V through C is equal to the charge leaving the volume V through D. Thus there is no change in the volume charge density in the volume V with time i.e.
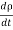 =0
=0
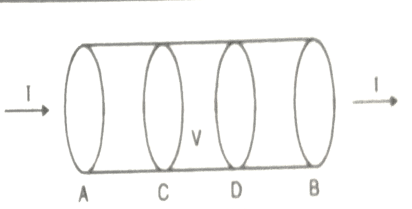
We may define steady current as that current in which the charge density is independent of time.
Using equation (7) we obtain
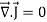
This is known as the equation of continuity for steady currents.
5. Question: Write a note on displacement current?
Solution:
Displacement current is the rate of change of electric displacement field.
In electromagnetism, displacement current is a quantity appearing in Maxwell's equations that is defined in terms of the rate of change of electric displacement field.
Displacement current has the units of electric current density, and it has an associated magnetic field just as actual currents do.
It is mathematically represented as
ID = JD S = S 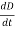
Where
S = Area of the plate of the capacitor
ID = Displacement current
JD = Displacement current density
D = εE where ε is permittivity of the medium
During the charging and discharging process of the capacitor, the electric current flows through the wires of the circuit. However, no current flow between the plates of the capacitor.
According to Ampere's law, the magnetic field should not present between the plates as there is no current, but in reality, the magnetic field exists there. Maxwell formulated this limitation of Ampere's law by adding a term in the equation of Ampere's law to solve the issue.
Maxwell predicted that the magnetic field will still exist even in the absence of conduction current, and the magnetic field may be associated with the changing electric field. This theory of Maxwell was experimentally proved.
Since the magnetic field is associated with the electric field, the general displacement current formula is given by,
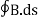 = μ0 ( I +ε0
= μ0 ( I +ε0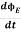 )
)
This equation is the generalized formula of Maxwell-Ampere law.
Displacement Current Definition
The displacement current (ID) is the part which Maxwell has added to the Ampere's law.
ID = ε0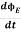
ε0 = Permittivity of free space
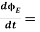 Derivative of electric flux
Derivative of electric flux
Electric flux is the time rate change of flow of the electric field through a surface. If we take the derivative of electric flux, we get the rate of change of the electric field of a given area concerning time.
6. Question: Write a note on skin depth?
Solution:
We know that amplitude of the em wave decreases exponentially with distance of penetration of the wave. Suppose, the amplitude at a depth x is denoted by Ex.
Then Ex = E0e-kx………….(1)
Where k = [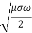 ] ………….(2)
] ………….(2)
The decrease in amplitude or attenuation of the field vector is quantitatively expressed in terms of a quantity called skin depth.
It is defined as the distance beyond the surface of the conductor inside it at which the amplitude of the field vector is reduced to 1/e of its value at the surface.
Let the skin depth is denoted by 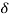 , Then:
, Then:
Eδ =  E0………….(3)
E0………….(3)
Where E0 = amplitude vector at the surface of the conductor
Eδ = amplitude of electric vector at depth 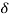
So equation (3) can be written as
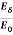 =
=  = e-1
= e-1
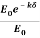 = e-1
= e-1
 e-1
e-1
k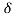 =1
=1
 =
= 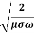
It shows that the skin depth varies inversely as the square root of conductivity of the medium and frequency of em wave.
7. Question: Derive Maxwell’s equations in vacuum and in non-conducting medium?
Solution:
Maxwell’s four equations are given by
∇·E = ρ/ε0(1)
∇×E = −∂B/∂t(2)
∇×H = J + ∂D/∂t(3)
∇·B = 0(4)
These equations illustrate the unique coexistence in nature of the electric field and the magnetic field. The first two equations give the value of the given flux through a closed surface, and the second two equations give the value of a line integral around a loop. In this notation,
∇=(∂/∂x, ∂/∂y, ∂/∂z)
E is the electric vector
B is the magnetic induction
ρ is the electric charge density
j is the electric current density
ε0 is the permittivity of free space
c is the speed of light.
In addition to Maxwell equations, the following identities are useful:
J = σE(5)
D = εE(6)
B = μH(7)
Here,
D is the electric displacement
H is the magnetic vector
σ is the specific conductivity
ε is the dielectric constant (or permittivity)
μ is the magnetic permeability
Free space or non-conducting medium. We know that non conducting medium means no current so conductivity is zero i.e. σ=0
So current density J=σE will also become zero as σ=0 Also free space means no charges which leads to ρ=0. These points mentioned below.
(a) No condition current i.e σ=0, thus J=0 ( J=σE)
(b) No charges (i.e ρ=0)
For the case of no charges or currents, that is, j = 0 and ρ = 0, and a homogeneous medium. Using these the Maxwell equation can rewritten as
∇.D=0 or ∇.E=0 as ρ=0 (12)
∇ x E= -dB/dt or ∇ x E= -μ dH/dt because B = μH (13)
∇ x H=d D/dt or ∇ x H = ε dE/dt (J=0) and D = εE (14)
∇.B=0 (15)
Now taking curl of second Maxwell’s equation (13) ,we get
∇ x(∇ x E)=- μ d/dt (∇ x H)
Applying standard vector identity, that is [∇ *(∇*E)=∇(∇.E)-∇2E] on left hand side of above equation, we get
∇ (∇ .E)-∇2E= -μ d/dt (∇ x H) (16)
Substituting equations (13) and (14) in equations (16) ,we get
-∇2E= – με d/dt (dE/dt)
Or ∇2E=με d 2 E/dT2 (17)
Equation (17) is the required wave equation in terms of electric field intensity, E for free space . This is the law that E must obey.
Wave Equation In Terms Of Magnetic Field Intensity, H
Take curl of fourth Maxwell’s equation(14) ,we get
∇x(∇xH)=ε d/dt(∇xE)
Applying standard vector identity that is
[∇*(∇*H)=∇ (∇.H)-∇2H]
On left side of above equation , we get
∇(∇.H)-∇2H= ε d/dt(∇xE) (18)
Substituting equations (14) and (13) in equation(18) ,we get
-∇2H= – μεd/dt(dH/dt)
Or
∇2H=με d2H/dt2 (19)
Equations (19) is the required wave equation in terms of magnetic field intensity, H and this is the law that H must obey
For vacuum μ=μ0 and ε=ε0, equations (17) and (19) will become
∇2 E=μ0ε0 d2E/dt2 (20)
And ∇2H= μ0ε0 d2H/dt2 (21)
This leads to an expression for the velocity of propagation
From equation both equations (20) and (21) have the form of the general wave equation for a wave
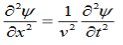
(x,t) traveling in the x direction with speed v. Equating the speed with the coefficients, we derive the speed of electric and magnetic waves, which is a constant that we symbolize with “c”
It is useful to note that in vacuum
c2=1/ε0μ0
Where μ0 is the permeability of free space
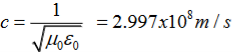
8. Question: Derive Maxwell’s equations in conducting medium?
Solution:
We will consider a plane electromagnetic wave travelling in a linear dielectric medium such as air along the z direction and being incident at a conducting interface. The medium will be taken to be a linear medium. So that one can describe the electrodynamics using only the E and H vectors. We wish to investigate the propagation of the wave in the conducting medium.
As the medium is linear and the propagation takes place in the infinite medium, the vectors 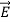 ,
, 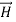 and
and  are still mutually perpendicular. We take the electric field along the x direction, the magnetic field along the y-direction and the propagation to take place in the z direction. Further, we will take the conductivity to be finite and the conductor to obey Ohm’s law,
are still mutually perpendicular. We take the electric field along the x direction, the magnetic field along the y-direction and the propagation to take place in the z direction. Further, we will take the conductivity to be finite and the conductor to obey Ohm’s law, 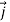 =𝜎
=𝜎 . Consider the pair of curl equations of Maxwell.
. Consider the pair of curl equations of Maxwell.
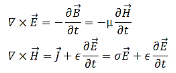
Let us take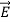 ,
, 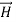 and
and  to be respectively in x, y and z direction. We then have,
to be respectively in x, y and z direction. We then have,
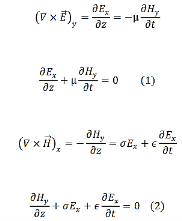
We take the time variation to be harmonic ( ~𝑒𝑖ωt ) so that the time derivative is equivalent to a multiplication by 𝑖ω. The pair of equations (1) and (2) can then be written as
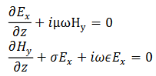
We can solve this pair of coupled equations by taking a derivative of either of the equations with respect to z and substituting the other into it,
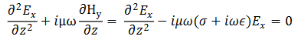
Define, a complex constant γ through
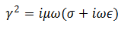
In terms of which we have,
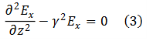
Similarly, we get
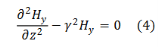
Solutions of (3) and (4) are well known and are expressed in terms of hyperbolic functions,

Where A, B, C and D are constants to be determined. If the values of the electric field at z=0 is 𝐸0 and that of the magnetic field at z=0 is 𝐻0, we have 𝐴 =𝐸0 and 𝐶 = 𝐻0.
In order to determine the constants B and D, let us return back to the original first order equations (1) and (2)
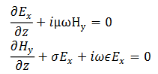
Substituting the solutions for E and H
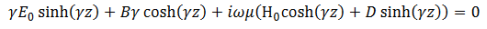
This equation must remain valid for all values of z, which is possible if the coefficients of sinh and cosh terms are separately equated to zero,
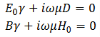
The former gives,
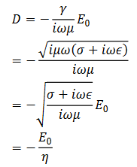
Where
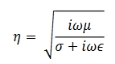
Likewise, we get,
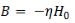
Substituting these, our solutions for the E and H become,
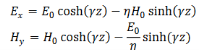
The wave is propagating in the z direction. Let us evaluate the fields when the wave has reached z = 𝑙,
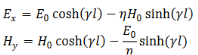
If 𝑙 is large, we can approximate
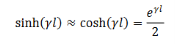
We then have,
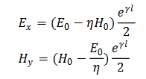
The ratio of the magnitudes of the electric field to magnetic field is defined as the “characteristic impedance” of the wave

Suppose we have lossless medium, σ=0, i.e. for a perfect conductor, the characteristic impedance is
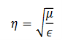
If the medium is vacuum, μ = μ0 = 4π x 10-7 N/A2 and 𝜖 = 𝜖0 = 8.85 × 10-12 C2/N - m2 gives 𝜂 ≈ 377 𝛺. The characteristic impedance has the dimension of resistance.
In this case, 𝛾 = 𝑖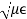 .
.
Let us look at the full three dimensional version of the propagation in a conductor. Once again, we start with the two curl equations,

Take a curl of both sides of the first equation,
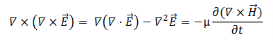
As there are no charges or currents, we ignore the divergence term and substitute for the curl of H from the second equation,
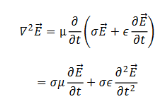
We take the propagating solutions to be
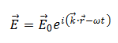
So that the above equation becomes,
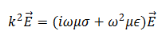
So that we have, the complex propagation constant to be given by
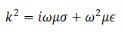
So that
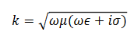
k is complex and its real and imaginary parts can be separated by standard algebra,
We have
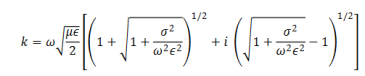
Thus the propagation vector β and the attenuation factor α are given by
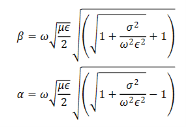
The ration 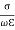 determines whether a material is a good conductor or otherwise. Consider a good conductor for which 𝜎 ≫ ω𝜖. For this case, we have,
determines whether a material is a good conductor or otherwise. Consider a good conductor for which 𝜎 ≫ ω𝜖. For this case, we have,
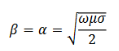
The speed of electromagnetic wave is given by
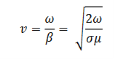
The electric field amplitude diminishes with distance as 𝑒-az. The distance to which the field penetrates before its amplitude diminishes be a factor 𝑒−1 is known as the “skin depth” , which is given by
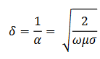
The wave does not penetrate much inside a conductor. Consider electromagnetic wave of frequency 1 MHz for copper which has a conductivity of approximately 6 × 107𝛺-1m-1. Substituting these values, one gets the skin depth in Cu to be about 0.067 mm. For comparison, the skin depth in sea water which is conducting because of salinity, is about 25 cm while that for fresh water is nearly 7m. Because of small skin depth in conductors, any current that arises in the metal because of the electromagnetic wave is confined within a thin layer of the surface.
9. Question: Derive Poynting Theorem?
Solution:
When electromagnetic wave travels in space, it carries energy and energy density is always associated with electric fields and magnetic fields.
The rate of energy travelled through per unit area i.e. the amount of energy flowing through per unit area in the perpendicular direction to the incident energy per unit time is called Poynting vector.
Mathematically Poynting vector is represented as
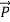 =
=  =(
=(  )(i)
)(i)
The direction of Poynting vector is perpendicular to the plane containing 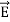 and
and 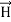 . Poynting vector is also called as instantaneous energy flux density. Here rate of energy transfer
. Poynting vector is also called as instantaneous energy flux density. Here rate of energy transfer  is perpendicular to both
is perpendicular to both  and
and . It represents the rate of energy transfer per unit area.
. It represents the rate of energy transfer per unit area.
UNIT
Its unit is W/m2.
Poynting Theorem
Poynting theorem states that the net power flowing out of a given volume V is equal to the time rate of decrease of stored electromagnetic energy in that volume decreased by the conduction losses.
i.e. total power leaving the volume =Rate of decrease of stored electromagnetic energy – ohmic power dissipated due to motion of charge
Proof
The energy density carried by the electromagnetic wave can be calculated using Maxwell's equations
Div 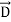 = 0 ...(i)
= 0 ...(i)
Div 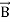 =0 ...(ii)
=0 ...(ii)
Curl 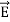 = -
= - 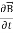 …(iii)
…(iii)
Curl  =
=  +
+ 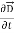 …(iv)
…(iv)
Taking scalar product of (3) with H and (4) with 
i.e.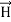 curl
curl 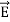 = -
= - 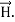
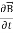 ……(v)
……(v)
And 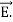 curl
curl 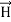 =
= 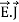 +
+ 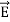 .
. 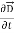 …..(vi)
…..(vi)
Doing (vi) – (v) i.e.
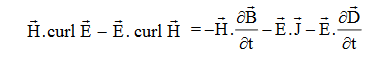
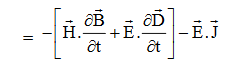
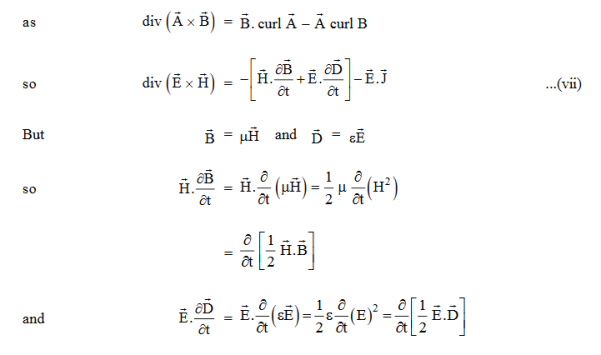
So from equation (vii)
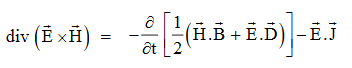
Or
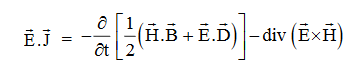
Integrating equation (viii) over a volume V enclosed by a Surface S
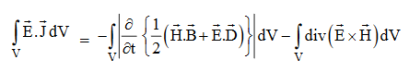
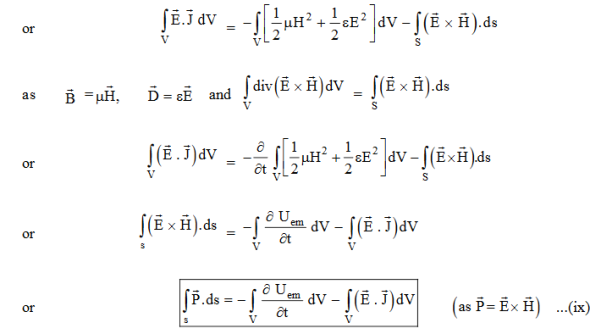
Total power leaving the volume = rate of decrease of stored e.m. Energy -ohmic power dissipated due to charge motion
This equation (ix) represents the Poynting theorem according to which the net power flowing out of a given volume is equal to the rate of decrease of stored electromagnetic energy in that volume minus the conduction losses.
In equation (ix) 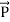 .ds represents the amount of electromagnetic energy crossing the closed surface per second or the rate of flow of outward energy through the surface S enclosing volume V i.e. it is Poynting vector.
.ds represents the amount of electromagnetic energy crossing the closed surface per second or the rate of flow of outward energy through the surface S enclosing volume V i.e. it is Poynting vector.
The term represent
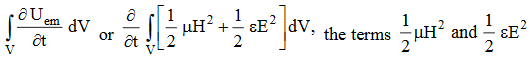
The energy stored in electric and magnetic fields respectively and their sum denotes the total energy stored in electromagnetic field. So total terms gives the rate of decrease of energy stored in volume V due to electric and magnetic fields.
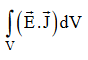
Gives the rate of energy transferred into the electromagnetic field.
This is also known as work-energy theorem. This is also called as the energy conservation law in electromagnetism.
10. Question: Define Gauss’s law?
Solution:
The law was published posthumously in 1867 as part of a collection of work by the famous German mathematician Carl Friedrich Gauss.
Gauss’s law for the electric field describes the static electric field generated by a distribution of electric charges.
It states that the electric flux through any closed surface is proportional to the total electric charge enclosed by this surface.
Let’s calculate the electric flux through a spherical surface around a positive point charge q, since we already know the electric field in such a situation. Recall that when we place the point charge at the origin of a coordinate system, the electric field at a point P that is at a distance r
From the charge at the origin is given by
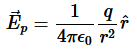
 is the radial vector from the charge at the origin to the point P. We can use this electric field to find the flux through the spherical surface of radius r, as shown in Figure 2
is the radial vector from the charge at the origin to the point P. We can use this electric field to find the flux through the spherical surface of radius r, as shown in Figure 2
.
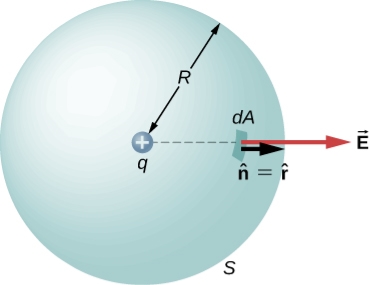
Figure 1: A closed spherical surface surrounding a point charge q.
Then we apply Φ=∫S  ⋅
⋅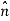 dA
dA
To this system and substitute known values. On the sphere,  and r=R
and r=R
So for an infinitesimal area dA,
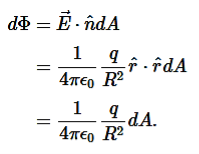
We now find the net flux by integrating this flux over the surface of the sphere
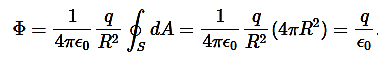
Where the total surface area of the spherical surface is 4πR2. This gives the flux through the closed spherical surface at radius r as
Φ=q/ϵ0
A remarkable fact about this equation is that the flux is independent of the size of the spherical surface. This can be directly attributed to the fact that the electric field of a point charge decreases as 1/r2 with distance, which just cancels the r2 rate of increase of the surface area.
11. Question: Write a short note on Maxwell’s equations?
Solution:
Maxwell equations are of fundamental importance since they describe the whole of classical electromagnetic phenomena.
Maxwell's Equations are a set of 4 complicated equations that describe the world of electromagnetics. These equations describe how electric and magnetic fields propagate, interact, and how they are influenced by objects.
Maxwell was one of the first to determine the speed of propagation of electromagnetic (EM) waves was the same as the speed of light - and hence to conclude that EM waves and visible light were really the same thing.
These equations are rules the universe uses to govern the behaviour of electric and magnetic fields. A flow of electric current will produce a magnetic field. If the current flow varies with time (as in any wave or periodic signal), the magnetic field will also give rise to an electric field. Maxwell's Equations shows that separated charge (positive and negative) gives rise to an electric field - and if this is varying in time as well will give rise to a propagating electric field, further giving rise to a propagating magnetic field.
From a classical perspective, light can be described as waves of electromagnetic radiation. As such, Maxwell equations are very useful to illustrate a number of the characteristics of light including polarization.
We are just to stating these equations without derivation. Since our goal is simply to apply them, the usual approach will be followed.
Maxwell’s four equations are given by
∇·E = ρ/ε0(1)
∇·B = 0(2)
∇×E = −∂B/∂t(3)
∇×H = J + ∂D/∂t(4)
These equations illustrate the unique coexistence in nature of the electric field and the magnetic field. The first two equations give the value of the given flux through a closed surface, and the second two equations give the value of a line integral around a loop. In this notation,
∇=(∂/∂x, ∂/∂y, ∂/∂z)
E is the electric vector
B is the magnetic induction
ρ is the electric charge density
j is the electric current density
ε0 is the permittivity of free space
c is the speed of light.
In addition to Maxwell equations, the following identities are useful:
J = σE(5)
D = εE(6)
B = μH(7)
Here,
D is the electric displacement
H is the magnetic vector
σ is the specific conductivity
ε is the dielectric constant (or permittivity)
μ is the magnetic permeability
12. Question: Explain Maxwell’s first equation in differential and integral form??
Solution:
Maxwell’s first equation (Gauss' Law for Electric Fields)
Gauss' Law is the first of Maxwell's Equations which dictates how the Electric Field behaves around electric charges. Gauss' Law can be written in terms of the Electric Flux Density and the Electric Charge Density as:
∇·E = ρ/ε0
0r
∇·D = ρ
Hence, Gauss' law is a mathematical statement that the total Electric Flux exiting any volume is equal to the total charge inside. Hence, if the volume in question has no charge within it, the net flow of Electric Flux out of that region is zero. If there is positive charge within a volume, then there exists a positive amount of Electric Flux exiting any volume that surrounds the charge. If there is negative charge within a volume, then there exists a negative amount of Electric Flux exiting (i.e. the Electric Flux enters the volume).
The Gauss’s law states that flux passing through any closed surface is equal to 1/ε0 times the total charge enclosed by that surface.
Integral form of Maxwell’s 1st equation
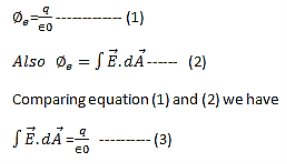
It is the integral form of Maxwell’s 1st equation.
Maxwell’s first equation in differential form
It is called the differential form of Maxwell’s 1st equation.

13. Question: Explain Maxwell’s second equation in differential and integral form?
Solution:
The Second Maxwell’s equation (Gauss’s law for magnetism)
The Gauss’s law for magnetism states that net flux of the magnetic field through a closed surface is zero because monopoles of a magnet do not exist.
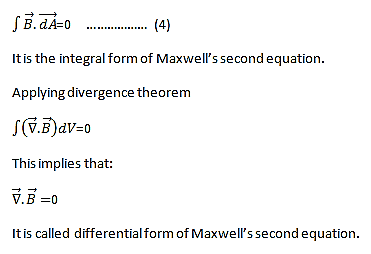
We know that Gauss' Law for Electric Fields states that the divergence of the Electric Flux Density D is equal to the volume electric charge density. But the second equation, Gauss' Magnetism law states that the divergence of the Magnetic Flux Density (B) is zero.
Why? Why isn't the divergence of B equal to the magnetic charge density?
Well - it is. But it just so happens that no one has ever found magnetic charge - not in a laboratory or on the street or on the subway. And therefore, until this hypothetical magnetic charge is found, we set the right side of Gauss' Law for Magnetic Fields to zero:
∇·B = 0
Now, you may have played with magnets when you were little, and these magnetic objects attracted other magnets similar to how electric charges repel or attract like electric charges. However, there is something special about these magnets - they always have a positive and negative end. This means every magnetic object is a magnetic dipole, with a north and South Pole. No matter how many times you break the magnetic in half, it will just form more magnetic dipoles. Gauss' Law for Magnetism states that magnetic monopoles do not exist - or at least we haven't found them yet.
14. Question: Explain Maxwell’s third equation in differential and integral form?
Solution:
Maxwell’s Third equation (Faraday's Law)
Faraday figured out that a changing Magnetic Flux within a circuit (or closed loop of wire) produced an induced EMF, or voltage within the circuit. Maxwell gives his second equation from this.
Faraday's Law tells us that a magnetic field that is changing in time will give rise to a circulating E-field. This means we have two ways of generating E-fields - from Electric Charges (or flowing electric charge, current) or from a magnetic field that is changing.
∇×E = −∂B/∂t
According to Faraday’s law of electromagnetic induction

It is the differential form of Maxwell’s third equation.
15. Question: Explain Maxwell’s fourth equation in differential and integral form?
Solution:
Maxwell’s fourth equation (Ampere's Law)
Ampere's Law tells us that a flowing electric current gives rise to a magnetic field that circles the wire. In addition to this, it also says that an Electric Field that is changing in time gives rise to a magnetic field that encircles the E-field - this is the Displacement Current term that Maxwell himself introduced.
This means there are 2 ways to generate a solenoidal (circulating) H-field - a flowing electric current or a changing Electric Field. Both give rise to the same phenomenon.
Modification of Ampere’s circuital law. Maxwell modified Ampere’s law by giving the concept of displacement current D and so the concept of displacement current density Jd for time varying fields.
For time varying fields should be written as
∇ xH=J+jd (7)
By taking divergence of equation(7) , we get
∇ .( ∇ xH)= ∇.J+ ∇.Jd
As divergence of the curl of a vector is always zero,therefore
∇ .( ∇ x H)=0
It means, ∇ .(J+ .Jd)=0
Or ∇. J= – ∇.Jd
But from equation of continuity for time varying fields,
∇.J= – dρ/ dt
By comparing above two equations of .j ,we get
∇ .jd =d(∇ .D)/dt (8)
Because from Maxwell’s first equation ∇ .D=ρ
As the divergence of two vectors is equal only if the vectors are equal.
Thus Jd= dD/dt
Substituting above equation in equation (7), we get
∇ xH=J+dD/dt (9)
Here ,dD/dt= Jd=Displacement current density
J=conduction current density
D= displacement current
The equation (9) is the Differential form of Maxwell’s fourth equation or Modified Ampere’s circuital law.
Intergal form
Taking surface integral of equation (9) on both sides, we get
∫( ∇xH).dS=∫(J+ dD/dt).dS
Apply stoke’s theorem to L.H.S. Of above equation, we get
∫( ∇xH).dS=∫ H.dL
Comparing the above two equations ,we get
∫H.dL=∫(J+dD/dt).dS
Statement of modified Ampere’s circuital Law
The line integral of the Magnetic field H around any closed path or circuit is equal to the conductions current plus the time derivative of electric displacement through any surface bounded by the path.
Equation (9) is the integral form of Maxwell’s fourth equation.
The modified form of Ampere's Law s given by Maxwell’s fourth equation in differential form
∇×H = J + ∂D/∂t
Maxwell’s third equation in integral form
∫( ∇xH).dS=∫(J+ dD/dt).dS
16 Question: If the electric field strength of a radio broadcast signal at a TV receiver is given by
E = 5.0 cos (t-y) az, V/m,
Determine the displacement current density. If the same field exists in a medium whose conductivity is given by 2.0 x 103(mho)/cm, find the conduction current density.
Solution:
E at a TV receiver in free space= 5.0 cos (t-y) az, V/m
Electric flux density
D =0E = 50cos (t-y) az, V/m
The displacement current density
Jd = 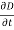
= 
Jd=-50sin (t-y) az, V/m2
The conduction current density,
Jc =E
=2.0 x 103(mho) /cm
= 2 x 105mho /m
Jc= 2 x 105x 5 cos (t-y) az
Jc= 106cos (t-y) az V/m2
17 Question: Given E = 10 sin (t-y) ay V/m, in free space, determine D, B and H.
Solution:
E = 10 sin (t-y) ay, V/m
D =0E,
0= 8.854 x 10-12 F/m
D = 100sin (t-y) ay, C/m2
Second Maxwell’s equation is
x E = -B
That is,
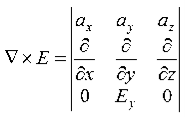
Or
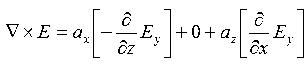
As Ey= 10 sin (t-z) V/m
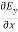 = 0
= 0
Now,x E becomes
x E = - 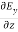 ax
ax
= 10 cos (t-z) ax
= - 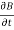
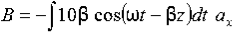
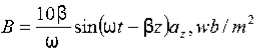
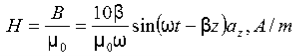
18 Question: If the electric field strength, E of an electromagnetic wave in free space is given by
E = 2 cos (t - 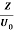 ) ay V/m,
) ay V/m,
Find the magnetic field, H
Solution:
We have
B/t =-x E
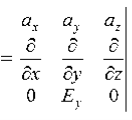
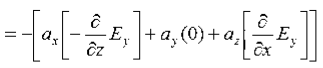
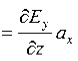
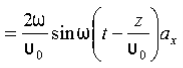
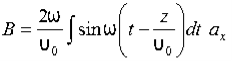
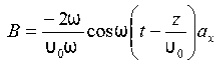
Thus
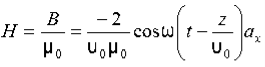
Also
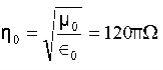
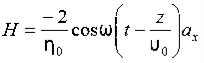
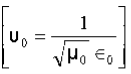
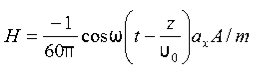
IIMT UNIVERSITY
UNIT- 2
ELECTROMAGNETIC
QUESTION BANK
1. Question: Calculate the skin depth for a frequency of 1010 Hz for silver. Given that  =2 x 107 Sm-1 and 4π x 10-7 m-1
=2 x 107 Sm-1 and 4π x 10-7 m-1
Solution:
 =
= 
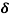 =
= 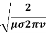
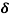 =
=
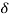 =1.125
=1.125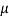 m.
m.
2. Question: Find the skin depth of copper if  =5.8 x 107 Sm-1 and
=5.8 x 107 Sm-1 and  = 1.26
= 1.26 H per meter and n =50 Hz.
H per meter and n =50 Hz.
Solution:
Given 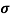 = 5.8 x 107 Sm-1
= 5.8 x 107 Sm-1
 = 1.26
= 1.26 x 10-6 Hm-1
x 10-6 Hm-1
n =50 Hz
ω = 2πn =2 x 3.14 x 50 = 314.3
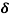 =
= 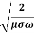
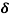 =
=  =
= = 9.33mm
= 9.33mm
3. Question: Solar Constant At the upper surface of the Earth’s atmosphere, the time-averaged magnitude of the Poynting vector, <S> = 1.35x103 W/m2, is referred to as the solar constant.
(a) Assuming that the Sun’s electromagnetic radiation is a plane sinusoidal wave, what are the magnitudes of the electric and magnetic fields?
(b)What is the total time-averaged power radiated by the Sun? The mean Sun-Earth distance is R= 1.50x1011m
Solution:
(a)The time-averaged Poynting vector is related to the amplitude of the electric field by
<S> = = 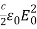
The amplitude of electric field is therefore
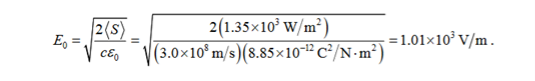
The amplitude of magnetic field can be obtained by
B0 = E0/ c
= 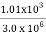 = 3.4 x 10-6 T
= 3.4 x 10-6 T
The associated magnetic field is one tenth of the earth’s magnetic field.
(b) The total time averaged power radiated by the Sun at the distance R is
<P> =<S>A =<S> 4πR2
=1.35x103 4 x 3.14 x (1.50x1011)2
= 3.8 x 1026 W
The type of wave discussed in the example above is a spherical wave, which originates from a “point-like” source. The intensity at a distance r from the source is which decreases is .
I =<S> = <P> /4πR2
Which decreases as 1/r2, On the other hand, the intensity of a plane wave remains constant and there is no spreading in its energy.
4. Question: Discuss continuity equation for current density
Solution:
Consider a closed surface S enclosing a Volume V through which currents are flowing. Let I be the current passing through the surface at any time t. Consider a small area 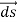 of the closed surface. The outward current flowing through
of the closed surface. The outward current flowing through 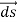 at a time t is
at a time t is
DI = 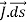 …………..(1)
…………..(1)
The total current flowing out through the closed surface S at time t is
I =  …………..(2)
…………..(2)
Suppose is ρ the Volume Charge density of charges inside the surface S. Then the total charge inside the Volume V is
q = 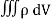 …………..(3)
…………..(3)
Since the current is flowing outward is it mean that charge within the enclosed surface is decreasing with time. The time rate of decrease of charge is
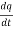 = -
= -
 …………..(4)
…………..(4)
The negative sign shows that the charge inside the surface S is decreasing with time. The above equation can be written as
 = -
= - 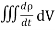 …………..(5)
…………..(5)
Since the charge is conserved, the current flowing outward through the closed surface must be equal to the rate of decrease of charge. Thus
I = 
Using equation (2) and (5), it becomes
 = -
= -  …………..(6)
…………..(6)
According to divergence theorem
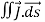 =
= 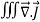 dV
dV
Using this result in equation (6) we obtain
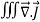 dV = -
dV = - 
This equation holds good for arbitrary volumes. Therefore the integrands must be equal. Hence
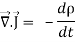
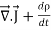 =0 …………..(7)
=0 …………..(7)
This equation is called equation of continuity. It is the mathematical statement of law of conservation of charge.
SPECIAL CASE
Consider a conductor AB through which a steady current I is flowing. The current I is said to be steady if charge is flowing per unit time through any two any sections C and D is the same. This means that the total amount of charge entering the volume V through C is equal to the charge leaving the volume V through D. Thus there is no change in the volume charge density in the volume V with time i.e.
 =0
=0

We may define steady current as that current in which the charge density is independent of time.
Using equation (7) we obtain
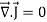
This is known as the equation of continuity for steady currents.
5. Question: Write a note on displacement current?
Solution:
Displacement current is the rate of change of electric displacement field.
In electromagnetism, displacement current is a quantity appearing in Maxwell's equations that is defined in terms of the rate of change of electric displacement field.
Displacement current has the units of electric current density, and it has an associated magnetic field just as actual currents do.
It is mathematically represented as
ID = JD S = S 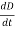
Where
S = Area of the plate of the capacitor
ID = Displacement current
JD = Displacement current density
D = εE where ε is permittivity of the medium
During the charging and discharging process of the capacitor, the electric current flows through the wires of the circuit. However, no current flow between the plates of the capacitor.
According to Ampere's law, the magnetic field should not present between the plates as there is no current, but in reality, the magnetic field exists there. Maxwell formulated this limitation of Ampere's law by adding a term in the equation of Ampere's law to solve the issue.
Maxwell predicted that the magnetic field will still exist even in the absence of conduction current, and the magnetic field may be associated with the changing electric field. This theory of Maxwell was experimentally proved.
Since the magnetic field is associated with the electric field, the general displacement current formula is given by,
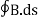 = μ0 ( I +ε0
= μ0 ( I +ε0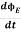 )
)
This equation is the generalized formula of Maxwell-Ampere law.
Displacement Current Definition
The displacement current (ID) is the part which Maxwell has added to the Ampere's law.
ID = ε0
ε0 = Permittivity of free space
 Derivative of electric flux
Derivative of electric flux
Electric flux is the time rate change of flow of the electric field through a surface. If we take the derivative of electric flux, we get the rate of change of the electric field of a given area concerning time.
6. Question: Write a note on skin depth?
Solution:
We know that amplitude of the em wave decreases exponentially with distance of penetration of the wave. Suppose, the amplitude at a depth x is denoted by Ex.
Then Ex = E0e-kx………….(1)
Where k = [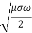 ] ………….(2)
] ………….(2)
The decrease in amplitude or attenuation of the field vector is quantitatively expressed in terms of a quantity called skin depth.
It is defined as the distance beyond the surface of the conductor inside it at which the amplitude of the field vector is reduced to 1/e of its value at the surface.
Let the skin depth is denoted by 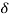 , Then:
, Then:
Eδ =  E0………….(3)
E0………….(3)
Where E0 = amplitude vector at the surface of the conductor
Eδ = amplitude of electric vector at depth 
So equation (3) can be written as
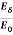 =
=  = e-1
= e-1
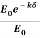 = e-1
= e-1
 e-1
e-1
k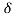 =1
=1
 =
= 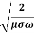
It shows that the skin depth varies inversely as the square root of conductivity of the medium and frequency of em wave.
7. Question: Derive Maxwell’s equations in vacuum and in non-conducting medium?
Solution:
Maxwell’s four equations are given by
∇·E = ρ/ε0(1)
∇×E = −∂B/∂t(2)
∇×H = J + ∂D/∂t(3)
∇·B = 0(4)
These equations illustrate the unique coexistence in nature of the electric field and the magnetic field. The first two equations give the value of the given flux through a closed surface, and the second two equations give the value of a line integral around a loop. In this notation,
∇=(∂/∂x, ∂/∂y, ∂/∂z)
E is the electric vector
B is the magnetic induction
ρ is the electric charge density
j is the electric current density
ε0 is the permittivity of free space
c is the speed of light.
In addition to Maxwell equations, the following identities are useful:
J = σE(5)
D = εE(6)
B = μH(7)
Here,
D is the electric displacement
H is the magnetic vector
σ is the specific conductivity
ε is the dielectric constant (or permittivity)
μ is the magnetic permeability
Free space or non-conducting medium. We know that non conducting medium means no current so conductivity is zero i.e. σ=0
So current density J=σE will also become zero as σ=0 Also free space means no charges which leads to ρ=0. These points mentioned below.
(a) No condition current i.e σ=0, thus J=0 ( J=σE)
(b) No charges (i.e ρ=0)
For the case of no charges or currents, that is, j = 0 and ρ = 0, and a homogeneous medium. Using these the Maxwell equation can rewritten as
∇.D=0 or ∇.E=0 as ρ=0 (12)
∇ x E= -dB/dt or ∇ x E= -μ dH/dt because B = μH (13)
∇ x H=d D/dt or ∇ x H = ε dE/dt (J=0) and D = εE (14)
∇.B=0 (15)
Now taking curl of second Maxwell’s equation (13) ,we get
∇ x(∇ x E)=- μ d/dt (∇ x H)
Applying standard vector identity, that is [∇ *(∇*E)=∇(∇.E)-∇2E] on left hand side of above equation, we get
∇ (∇ .E)-∇2E= -μ d/dt (∇ x H) (16)
Substituting equations (13) and (14) in equations (16) ,we get
-∇2E= – με d/dt (dE/dt)
Or ∇2E=με d 2 E/dT2 (17)
Equation (17) is the required wave equation in terms of electric field intensity, E for free space . This is the law that E must obey.
Wave Equation In Terms Of Magnetic Field Intensity, H
Take curl of fourth Maxwell’s equation(14) ,we get
∇x(∇xH)=ε d/dt(∇xE)
Applying standard vector identity that is
[∇*(∇*H)=∇ (∇.H)-∇2H]
On left side of above equation , we get
∇(∇.H)-∇2H= ε d/dt(∇xE) (18)
Substituting equations (14) and (13) in equation(18) ,we get
-∇2H= – μεd/dt(dH/dt)
Or
∇2H=με d2H/dt2 (19)
Equations (19) is the required wave equation in terms of magnetic field intensity, H and this is the law that H must obey
For vacuum μ=μ0 and ε=ε0, equations (17) and (19) will become
∇2 E=μ0ε0 d2E/dt2 (20)
And ∇2H= μ0ε0 d2H/dt2 (21)
This leads to an expression for the velocity of propagation
From equation both equations (20) and (21) have the form of the general wave equation for a wave
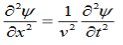
(x,t) traveling in the x direction with speed v. Equating the speed with the coefficients, we derive the speed of electric and magnetic waves, which is a constant that we symbolize with “c”
It is useful to note that in vacuum
c2=1/ε0μ0
Where μ0 is the permeability of free space
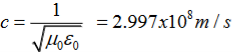
8. Question: Derive Maxwell’s equations in conducting medium?
Solution:
We will consider a plane electromagnetic wave travelling in a linear dielectric medium such as air along the z direction and being incident at a conducting interface. The medium will be taken to be a linear medium. So that one can describe the electrodynamics using only the E and H vectors. We wish to investigate the propagation of the wave in the conducting medium.
As the medium is linear and the propagation takes place in the infinite medium, the vectors  ,
, 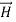 and
and 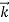 are still mutually perpendicular. We take the electric field along the x direction, the magnetic field along the y-direction and the propagation to take place in the z direction. Further, we will take the conductivity to be finite and the conductor to obey Ohm’s law,
are still mutually perpendicular. We take the electric field along the x direction, the magnetic field along the y-direction and the propagation to take place in the z direction. Further, we will take the conductivity to be finite and the conductor to obey Ohm’s law,  =𝜎
=𝜎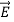 . Consider the pair of curl equations of Maxwell.
. Consider the pair of curl equations of Maxwell.
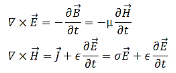
Let us take ,
,  and
and 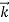 to be respectively in x, y and z direction. We then have,
to be respectively in x, y and z direction. We then have,
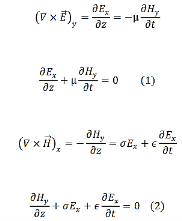
We take the time variation to be harmonic ( ~𝑒𝑖ωt ) so that the time derivative is equivalent to a multiplication by 𝑖ω. The pair of equations (1) and (2) can then be written as
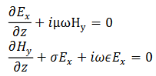
We can solve this pair of coupled equations by taking a derivative of either of the equations with respect to z and substituting the other into it,

Define, a complex constant γ through

In terms of which we have,
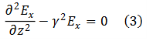
Similarly, we get

Solutions of (3) and (4) are well known and are expressed in terms of hyperbolic functions,
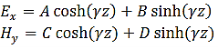
Where A, B, C and D are constants to be determined. If the values of the electric field at z=0 is 𝐸0 and that of the magnetic field at z=0 is 𝐻0, we have 𝐴 =𝐸0 and 𝐶 = 𝐻0.
In order to determine the constants B and D, let us return back to the original first order equations (1) and (2)
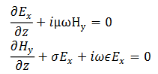
Substituting the solutions for E and H
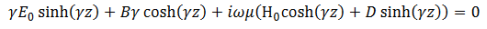
This equation must remain valid for all values of z, which is possible if the coefficients of sinh and cosh terms are separately equated to zero,
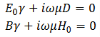
The former gives,
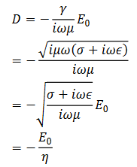
Where
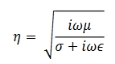
Likewise, we get,
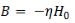
Substituting these, our solutions for the E and H become,
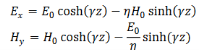
The wave is propagating in the z direction. Let us evaluate the fields when the wave has reached z = 𝑙,
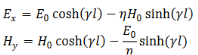
If 𝑙 is large, we can approximate
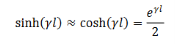
We then have,
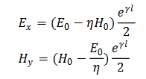
The ratio of the magnitudes of the electric field to magnetic field is defined as the “characteristic impedance” of the wave
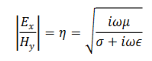
Suppose we have lossless medium, σ=0, i.e. for a perfect conductor, the characteristic impedance is
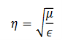
If the medium is vacuum, μ = μ0 = 4π x 10-7 N/A2 and 𝜖 = 𝜖0 = 8.85 × 10-12 C2/N - m2 gives 𝜂 ≈ 377 𝛺. The characteristic impedance has the dimension of resistance.
In this case, 𝛾 = 𝑖 .
.
Let us look at the full three dimensional version of the propagation in a conductor. Once again, we start with the two curl equations,
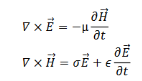
Take a curl of both sides of the first equation,
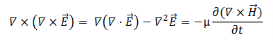
As there are no charges or currents, we ignore the divergence term and substitute for the curl of H from the second equation,
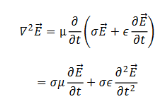
We take the propagating solutions to be

So that the above equation becomes,
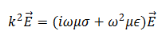
So that we have, the complex propagation constant to be given by
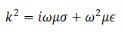
So that
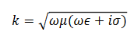
k is complex and its real and imaginary parts can be separated by standard algebra,
We have
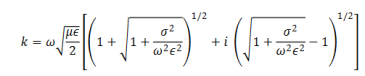
Thus the propagation vector β and the attenuation factor α are given by
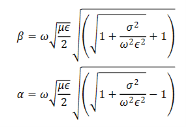
The ration 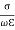 determines whether a material is a good conductor or otherwise. Consider a good conductor for which 𝜎 ≫ ω𝜖. For this case, we have,
determines whether a material is a good conductor or otherwise. Consider a good conductor for which 𝜎 ≫ ω𝜖. For this case, we have,
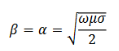
The speed of electromagnetic wave is given by
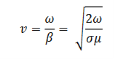
The electric field amplitude diminishes with distance as 𝑒-az. The distance to which the field penetrates before its amplitude diminishes be a factor 𝑒−1 is known as the “skin depth” , which is given by
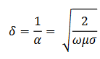
The wave does not penetrate much inside a conductor. Consider electromagnetic wave of frequency 1 MHz for copper which has a conductivity of approximately 6 × 107𝛺-1m-1. Substituting these values, one gets the skin depth in Cu to be about 0.067 mm. For comparison, the skin depth in sea water which is conducting because of salinity, is about 25 cm while that for fresh water is nearly 7m. Because of small skin depth in conductors, any current that arises in the metal because of the electromagnetic wave is confined within a thin layer of the surface.
9. Question: Derive Poynting Theorem?
Solution:
When electromagnetic wave travels in space, it carries energy and energy density is always associated with electric fields and magnetic fields.
The rate of energy travelled through per unit area i.e. the amount of energy flowing through per unit area in the perpendicular direction to the incident energy per unit time is called Poynting vector.
Mathematically Poynting vector is represented as
 =
=  =(
=( 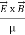 )(i)
)(i)
The direction of Poynting vector is perpendicular to the plane containing 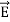 and
and 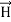 . Poynting vector is also called as instantaneous energy flux density. Here rate of energy transfer
. Poynting vector is also called as instantaneous energy flux density. Here rate of energy transfer  is perpendicular to both
is perpendicular to both  and
and . It represents the rate of energy transfer per unit area.
. It represents the rate of energy transfer per unit area.
UNIT
Its unit is W/m2.
Poynting Theorem
Poynting theorem states that the net power flowing out of a given volume V is equal to the time rate of decrease of stored electromagnetic energy in that volume decreased by the conduction losses.
i.e. total power leaving the volume =Rate of decrease of stored electromagnetic energy – ohmic power dissipated due to motion of charge
Proof
The energy density carried by the electromagnetic wave can be calculated using Maxwell's equations
Div 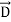 = 0 ...(i)
= 0 ...(i)
Div 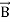 =0 ...(ii)
=0 ...(ii)
Curl 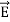 = -
= - 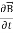 …(iii)
…(iii)
Curl 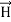 =
=  +
+  …(iv)
…(iv)
Taking scalar product of (3) with H and (4) with 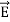
i.e. curl
curl 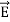 = -
= - 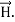
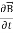 ……(v)
……(v)
And 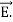 curl
curl 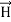 =
=  +
+ 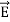 .
. 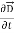 …..(vi)
…..(vi)
Doing (vi) – (v) i.e.
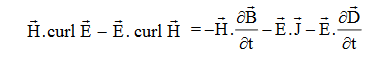
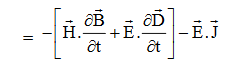
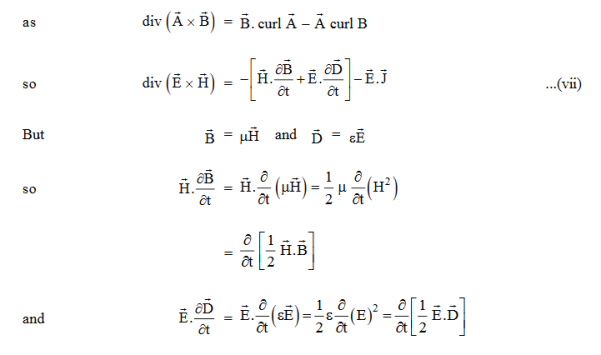
So from equation (vii)
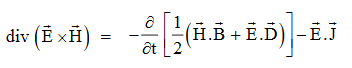
Or
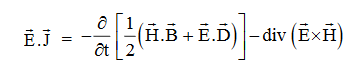
Integrating equation (viii) over a volume V enclosed by a Surface S
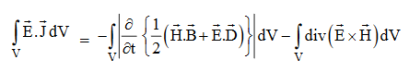
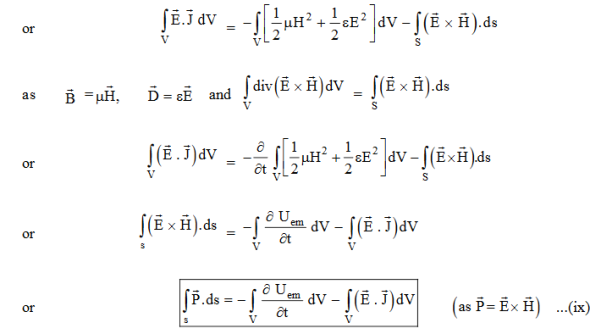
Total power leaving the volume = rate of decrease of stored e.m. Energy -ohmic power dissipated due to charge motion
This equation (ix) represents the Poynting theorem according to which the net power flowing out of a given volume is equal to the rate of decrease of stored electromagnetic energy in that volume minus the conduction losses.
In equation (ix)  .ds represents the amount of electromagnetic energy crossing the closed surface per second or the rate of flow of outward energy through the surface S enclosing volume V i.e. it is Poynting vector.
.ds represents the amount of electromagnetic energy crossing the closed surface per second or the rate of flow of outward energy through the surface S enclosing volume V i.e. it is Poynting vector.
The term represent
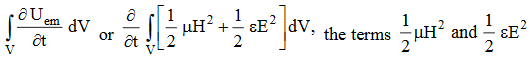
The energy stored in electric and magnetic fields respectively and their sum denotes the total energy stored in electromagnetic field. So total terms gives the rate of decrease of energy stored in volume V due to electric and magnetic fields.
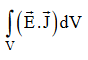
Gives the rate of energy transferred into the electromagnetic field.
This is also known as work-energy theorem. This is also called as the energy conservation law in electromagnetism.
10. Question: Define Gauss’s law?
Solution:
The law was published posthumously in 1867 as part of a collection of work by the famous German mathematician Carl Friedrich Gauss.
Gauss’s law for the electric field describes the static electric field generated by a distribution of electric charges.
It states that the electric flux through any closed surface is proportional to the total electric charge enclosed by this surface.
Let’s calculate the electric flux through a spherical surface around a positive point charge q, since we already know the electric field in such a situation. Recall that when we place the point charge at the origin of a coordinate system, the electric field at a point P that is at a distance r
From the charge at the origin is given by
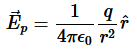
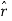 is the radial vector from the charge at the origin to the point P. We can use this electric field to find the flux through the spherical surface of radius r, as shown in Figure 2
is the radial vector from the charge at the origin to the point P. We can use this electric field to find the flux through the spherical surface of radius r, as shown in Figure 2
.
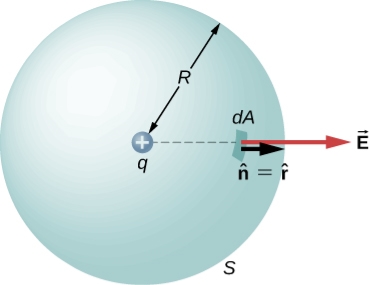
Figure 1: A closed spherical surface surrounding a point charge q.
Then we apply Φ=∫S 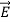 ⋅
⋅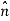 dA
dA
To this system and substitute known values. On the sphere, 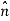 and r=R
and r=R
So for an infinitesimal area dA,
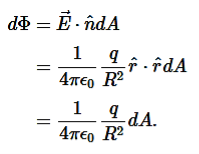
We now find the net flux by integrating this flux over the surface of the sphere
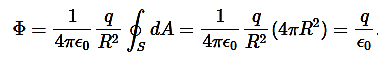
Where the total surface area of the spherical surface is 4πR2. This gives the flux through the closed spherical surface at radius r as
Φ=q/ϵ0
A remarkable fact about this equation is that the flux is independent of the size of the spherical surface. This can be directly attributed to the fact that the electric field of a point charge decreases as 1/r2 with distance, which just cancels the r2 rate of increase of the surface area.
11. Question: Write a short note on Maxwell’s equations?
Solution:
Maxwell equations are of fundamental importance since they describe the whole of classical electromagnetic phenomena.
Maxwell's Equations are a set of 4 complicated equations that describe the world of electromagnetics. These equations describe how electric and magnetic fields propagate, interact, and how they are influenced by objects.
Maxwell was one of the first to determine the speed of propagation of electromagnetic (EM) waves was the same as the speed of light - and hence to conclude that EM waves and visible light were really the same thing.
These equations are rules the universe uses to govern the behaviour of electric and magnetic fields. A flow of electric current will produce a magnetic field. If the current flow varies with time (as in any wave or periodic signal), the magnetic field will also give rise to an electric field. Maxwell's Equations shows that separated charge (positive and negative) gives rise to an electric field - and if this is varying in time as well will give rise to a propagating electric field, further giving rise to a propagating magnetic field.
From a classical perspective, light can be described as waves of electromagnetic radiation. As such, Maxwell equations are very useful to illustrate a number of the characteristics of light including polarization.
We are just to stating these equations without derivation. Since our goal is simply to apply them, the usual approach will be followed.
Maxwell’s four equations are given by
∇·E = ρ/ε0(1)
∇·B = 0(2)
∇×E = −∂B/∂t(3)
∇×H = J + ∂D/∂t(4)
These equations illustrate the unique coexistence in nature of the electric field and the magnetic field. The first two equations give the value of the given flux through a closed surface, and the second two equations give the value of a line integral around a loop. In this notation,
∇=(∂/∂x, ∂/∂y, ∂/∂z)
E is the electric vector
B is the magnetic induction
ρ is the electric charge density
j is the electric current density
ε0 is the permittivity of free space
c is the speed of light.
In addition to Maxwell equations, the following identities are useful:
J = σE(5)
D = εE(6)
B = μH(7)
Here,
D is the electric displacement
H is the magnetic vector
σ is the specific conductivity
ε is the dielectric constant (or permittivity)
μ is the magnetic permeability
12. Question: Explain Maxwell’s first equation in differential and integral form??
Solution:
Maxwell’s first equation (Gauss' Law for Electric Fields)
Gauss' Law is the first of Maxwell's Equations which dictates how the Electric Field behaves around electric charges. Gauss' Law can be written in terms of the Electric Flux Density and the Electric Charge Density as:
∇·E = ρ/ε0
0r
∇·D = ρ
Hence, Gauss' law is a mathematical statement that the total Electric Flux exiting any volume is equal to the total charge inside. Hence, if the volume in question has no charge within it, the net flow of Electric Flux out of that region is zero. If there is positive charge within a volume, then there exists a positive amount of Electric Flux exiting any volume that surrounds the charge. If there is negative charge within a volume, then there exists a negative amount of Electric Flux exiting (i.e. the Electric Flux enters the volume).
The Gauss’s law states that flux passing through any closed surface is equal to 1/ε0 times the total charge enclosed by that surface.
Integral form of Maxwell’s 1st equation
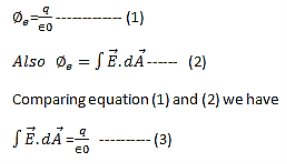
It is the integral form of Maxwell’s 1st equation.
Maxwell’s first equation in differential form
It is called the differential form of Maxwell’s 1st equation.

13. Question: Explain Maxwell’s second equation in differential and integral form?
Solution:
The Second Maxwell’s equation (Gauss’s law for magnetism)
The Gauss’s law for magnetism states that net flux of the magnetic field through a closed surface is zero because monopoles of a magnet do not exist.
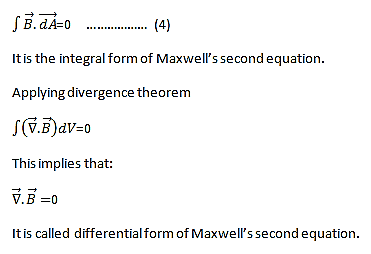
We know that Gauss' Law for Electric Fields states that the divergence of the Electric Flux Density D is equal to the volume electric charge density. But the second equation, Gauss' Magnetism law states that the divergence of the Magnetic Flux Density (B) is zero.
Why? Why isn't the divergence of B equal to the magnetic charge density?
Well - it is. But it just so happens that no one has ever found magnetic charge - not in a laboratory or on the street or on the subway. And therefore, until this hypothetical magnetic charge is found, we set the right side of Gauss' Law for Magnetic Fields to zero:
∇·B = 0
Now, you may have played with magnets when you were little, and these magnetic objects attracted other magnets similar to how electric charges repel or attract like electric charges. However, there is something special about these magnets - they always have a positive and negative end. This means every magnetic object is a magnetic dipole, with a north and South Pole. No matter how many times you break the magnetic in half, it will just form more magnetic dipoles. Gauss' Law for Magnetism states that magnetic monopoles do not exist - or at least we haven't found them yet.
14. Question: Explain Maxwell’s third equation in differential and integral form?
Solution:
Maxwell’s Third equation (Faraday's Law)
Faraday figured out that a changing Magnetic Flux within a circuit (or closed loop of wire) produced an induced EMF, or voltage within the circuit. Maxwell gives his second equation from this.
Faraday's Law tells us that a magnetic field that is changing in time will give rise to a circulating E-field. This means we have two ways of generating E-fields - from Electric Charges (or flowing electric charge, current) or from a magnetic field that is changing.
∇×E = −∂B/∂t
According to Faraday’s law of electromagnetic induction

It is the differential form of Maxwell’s third equation.
15. Question: Explain Maxwell’s fourth equation in differential and integral form?
Solution:
Maxwell’s fourth equation (Ampere's Law)
Ampere's Law tells us that a flowing electric current gives rise to a magnetic field that circles the wire. In addition to this, it also says that an Electric Field that is changing in time gives rise to a magnetic field that encircles the E-field - this is the Displacement Current term that Maxwell himself introduced.
This means there are 2 ways to generate a solenoidal (circulating) H-field - a flowing electric current or a changing Electric Field. Both give rise to the same phenomenon.
Modification of Ampere’s circuital law. Maxwell modified Ampere’s law by giving the concept of displacement current D and so the concept of displacement current density Jd for time varying fields.
For time varying fields should be written as
∇ xH=J+jd (7)
By taking divergence of equation(7) , we get
∇ .( ∇ xH)= ∇.J+ ∇.Jd
As divergence of the curl of a vector is always zero,therefore
∇ .( ∇ x H)=0
It means, ∇ .(J+ .Jd)=0
Or ∇. J= – ∇.Jd
But from equation of continuity for time varying fields,
∇.J= – dρ/ dt
By comparing above two equations of .j ,we get
∇ .jd =d(∇ .D)/dt (8)
Because from Maxwell’s first equation ∇ .D=ρ
As the divergence of two vectors is equal only if the vectors are equal.
Thus Jd= dD/dt
Substituting above equation in equation (7), we get
∇ xH=J+dD/dt (9)
Here ,dD/dt= Jd=Displacement current density
J=conduction current density
D= displacement current
The equation (9) is the Differential form of Maxwell’s fourth equation or Modified Ampere’s circuital law.
Intergal form
Taking surface integral of equation (9) on both sides, we get
∫( ∇xH).dS=∫(J+ dD/dt).dS
Apply stoke’s theorem to L.H.S. Of above equation, we get
∫( ∇xH).dS=∫ H.dL
Comparing the above two equations ,we get
∫H.dL=∫(J+dD/dt).dS
Statement of modified Ampere’s circuital Law
The line integral of the Magnetic field H around any closed path or circuit is equal to the conductions current plus the time derivative of electric displacement through any surface bounded by the path.
Equation (9) is the integral form of Maxwell’s fourth equation.
The modified form of Ampere's Law s given by Maxwell’s fourth equation in differential form
∇×H = J + ∂D/∂t
Maxwell’s third equation in integral form
∫( ∇xH).dS=∫(J+ dD/dt).dS
16 Question: If the electric field strength of a radio broadcast signal at a TV receiver is given by
E = 5.0 cos (t-y) az, V/m,
Determine the displacement current density. If the same field exists in a medium whose conductivity is given by 2.0 x 103(mho)/cm, find the conduction current density.
Solution:
E at a TV receiver in free space= 5.0 cos (t-y) az, V/m
Electric flux density
D =0E = 50cos (t-y) az, V/m
The displacement current density
Jd = 
= 
Jd=-50sin (t-y) az, V/m2
The conduction current density,
Jc =E
=2.0 x 103(mho) /cm
= 2 x 105mho /m
Jc= 2 x 105x 5 cos (t-y) az
Jc= 106cos (t-y) az V/m2
17 Question: Given E = 10 sin (t-y) ay V/m, in free space, determine D, B and H.
Solution:
E = 10 sin (t-y) ay, V/m
D =0E,
0= 8.854 x 10-12 F/m
D = 100sin (t-y) ay, C/m2
Second Maxwell’s equation is
x E = -B
That is,
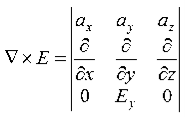
Or
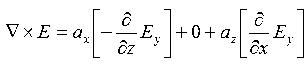
As Ey= 10 sin (t-z) V/m
 = 0
= 0
Now,x E becomes
x E = - 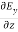 ax
ax
= 10 cos (t-z) ax
= - 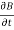
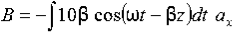
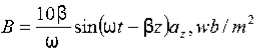
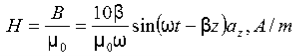
18 Question: If the electric field strength, E of an electromagnetic wave in free space is given by
E = 2 cos (t - 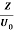 ) ay V/m,
) ay V/m,
Find the magnetic field, H
Solution:
We have
B/t =-x E
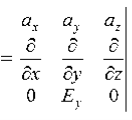

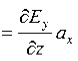
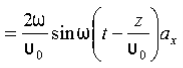
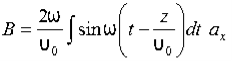
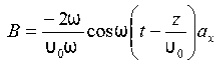
Thus
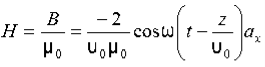
Also