UNIT 1

Introduction of Engineering Drawing

1. Explain the profile of number of objects consists of various types of curves.

1. Conic sections:

The section obtained by intersection of a right circular cone by a plane in different positions relative to the axis of the cone are called conics.

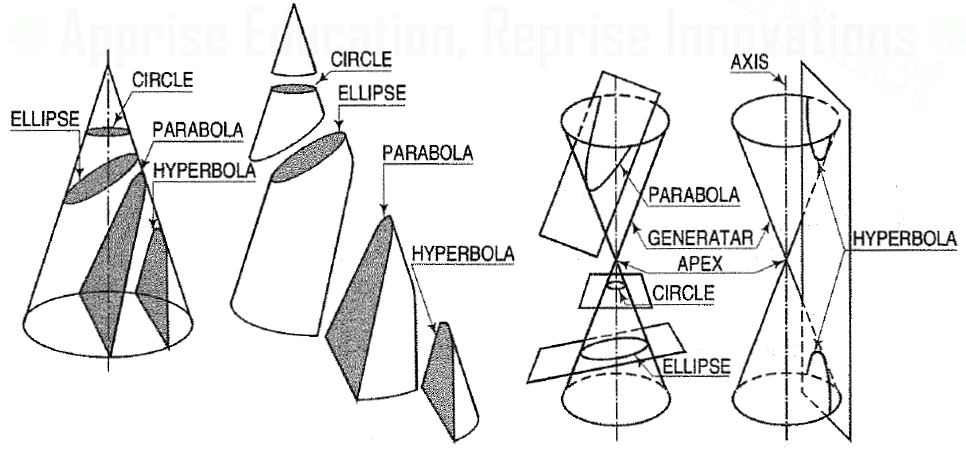
Figure 1

(i) When the section plane is inclined to the axis and cuts all the generators on one side of the apex, the section is an ellipse [fig. 1].

(ii) When the section plane is inclined to the axis and is parallel to one of the generators, the section is a parabola [fig. 1].

(iii) A hyperbola is a plane curve having two separate parts or branches, formed when two cones that point towards one another are intersected by a plane that is parallel to the axes of the cones.

Conic sections are always "smooth". More precisely, they never contain any inflection points.  This is important for many applications, such as aerodynamics, civil engineering, mechanical engineering, etc.

The conic may be defined as the locus of a point in a plane, wherein the ratio of its distance from a fixed point and a fixed straight line is always constant. The fixed point is called the focus and the fixed line, the directrix.

2. Define eccentricity.

The ratio 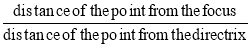is called eccentricity and is denoted by ‘e’. It is always less the 1 for ellipse, equal to 1 for parabola and greater than 1 for hyperbola i.e.

(i) ellipse: e < 1

(ii) parabola: e = 1

(iii) hyperbola: e > 1

3. Explain the role of Engineering Drawing.

The ability to read drawing is the most important requirement of all technical people in any profession. As compared to verbal or written description, this method is brief and clearer. Some of the applications are: building drawing for civil engineers, machine drawing for mechanical engineers, circuit diagrams for electrical and electronics engineers, computer graphics for one and all.

The subject in general is designed to impart the following skills.

1. Ability to read and prepare engineering drawings.

2. Ability to make free - hand sketching of objects.

3. Power to imagine, analyze and communicate, and

4. Capacity to understand other subjects.

4. Explain cycloid with construction.

Cycloid is a curve generated by a point on the circumference of a circle which rolls along a straight line. It can be described by an equation,

y = a (1 – cosθ) or x = a (θ - sinθ)

To construct a cycloid, given the diameter of the generating circle.

(i) With centre C and given radius R, draw a circle. Let P be the generating point.

(ii) Draw a line PA tangential to and equal to the circumference of the circle.

(iii) Divide the circle and the line PA into the same number of equal parts, say 12,and mark the division-points as shown.

(iv) Through C, draw a line CB parallel and equal to PA.

(v) Draw perpendiculars at points 1, 2 etc. cutting CB at points C1, C2 etc.

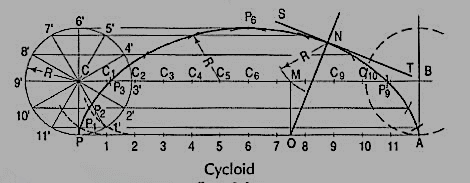
Assume that the circle starts rolling to the right. When point 1' coincides with 1, centre C will move to C1. In this position of the circle, the generating point P will have moved to position P1 on the circle, at a distance equal to P'1 from point 1. It is evident that P1 lies on the horizontal line through 1' and at a distance R from C1. Similarly, P2 will lie on the horizontal line through 2' and at the distance R from C2.

Construction:

(vi) Through the points 1 ', 2' etc. draw lines parallel to PA.

(vii) With centres C1, C2 etc. and radius equal to R, radius of generating circle, draw arcs cutting the lines through 1 ', 2' etc. at points P1, P2 etc. respectively. Draw a smooth curve through points P, P1, P2 …. A. This curve is the required cycloid.

Normal and tangent to a cycloid curve: The rule for drawing a normal to all cycloid curves:

The normal at any point on a cycloid curve will pass through the corresponding point of contact between the generating circle and the directing line or circle. The tangent at any point is perpendicular to the normal at that point.

5. Explain: Involute, spiral and Archemedian spiral.

Use of parabolic curves is made in arches, bridges, sound reflectors, light reflectors, etc. Mathematically it can be described by an equation 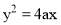or.

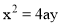Involute:

The involute is a curve traced out by an end of a piece of thread unwound from a circle or a polygon, the thread being kept tight. It may also be defined as a curve traced out by a point in a straight line which rolls without slipping along a circle or a polygon.

It is used as teeth profile of gear wheel.

Mathematically it can be described as by 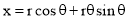, , where “r” is the radius of the circle.

Spiral:

If a line rotates in a plane about one of its ends and if at the same time, a point moves along the line continuously in one direction, the curve traced out by the moving point is called a spiral. The point about which the line rotates is called a pole.

The line joining any point on the curve with the pole is called the radius vector and the angle between this line and the line in its initial position is called the vectorial angle.

Archemedian spiral:

It is a curve traced out by a point moving in such a way that its movement towards and away from the pole is uniform with the increase of the vectorial angle from the starting line.

The use of this curve is made in teeth profiles of helical gears, profile of cams, etc.

6. Trace the paths of the ends of a straight-line AP, 100 mm long, when it rolls, without slipping, on a semi-circle having its diameter AB, 75 mm long. (Assume the line AP to be tangent to the semi-circle in the starting position.)

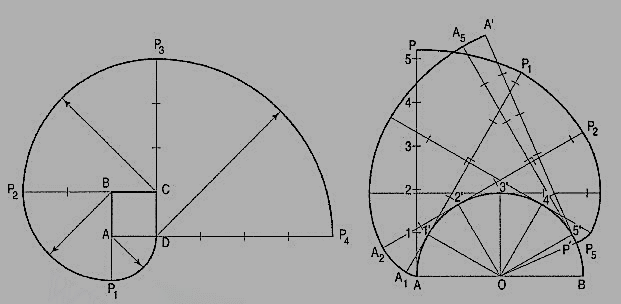
(i) Draw the semi-circle and divide it into six equal parts.

(ii) Draw the line AP and mark points 1, 2 etc. such that A1 = arc A1 ', A2 = arc A2' etc. The last division SP will be of a shorter length. On the semi-circle, mark a point P' such that S'P' = SP.

(iii) At points 1 ', 2' etc. draw tangents and on them, mark points P1, P2 etc. such that 1' P1 = 1P, 2' P2 =2P .... And 5' P5 = 5' P6 =SP. Similarly, mark pointsA1, A2 etc. such that A11'= A1, A22' = A2 .... And A'p'= AP. Draw the required curves through points P,P1 .... And P', and through points A, A1 .... And A'.

If AP is an inelastic string with the end A attached to the semicircle, the end P will trace out the same curve PP' when the string is wound round the semi-circle.

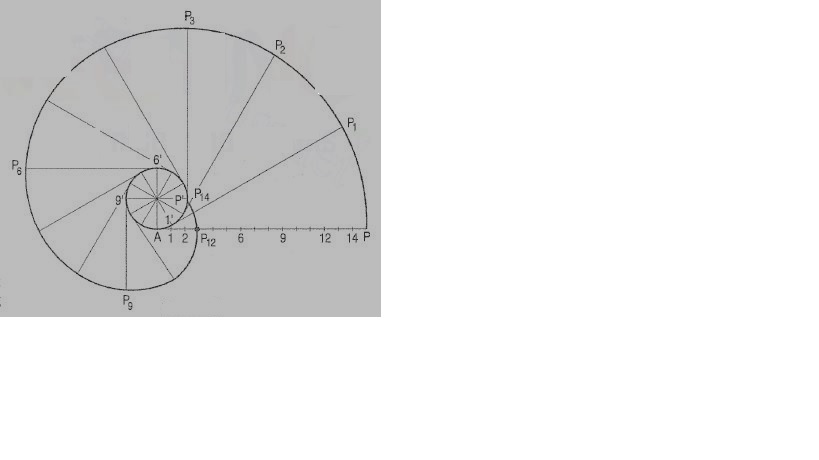
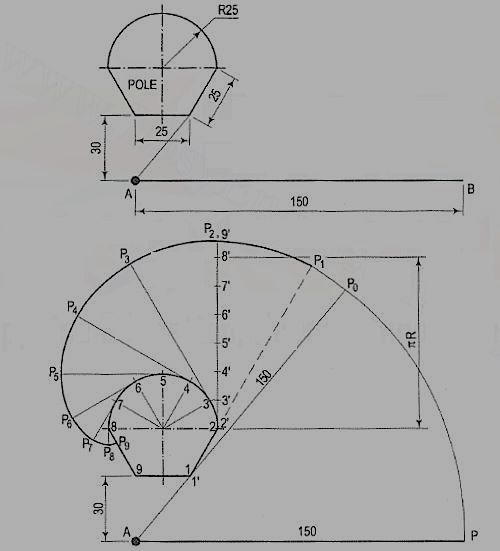
7. AP is a rope 1.50-metre-long, tied to peg at A as shown in fig. 24. Keeping it always tight, the rope is wound round the pole. Draw the curve traced out by the end P. Use scale 1 :20.

Draw given figure to the scale.

(ii) From A, draw a line passing through 1. A as centre and AP as radius, draw the arc intersecting extended line A1' at P0. Extend the side 1-2, 1 as centre and 1 'Po as radius, draw the arc to intersect extended line 1-2 at P1.

(iii) Divide the circumference of the semicircle into six equal parts and label it as 2, 3, 4, 5, 6, 7 and 8.

(iv) Draw a tangent to semicircle from 2 such that 2'-P1 = 2'-P2 . Mark 8' on this tangent such that 2'-8' = nR. Divide 2'-8' into six equal parts.

(v) Similarly draw tangents at 3, 4, 5, 6, 7 and 8 in anti-clockwise direction such that 3-P3 = 3'-9', 4-P4 = 4'-9', 5-P5 = 5'-9', 6-P6 = 6'-9', 7-P7 = 7'-9', 8-P8 = 8'-9' and 8-P9 = 8'-9' respectively.

(vi) Join P1, P2, ....• , P9 with smooth curve.

8. What is a scale and why it is needed?

A scale is defined as the ratio of the linear dimensions of the object as represented in a drawing to the actual dimensions of the same.

Necessity

• Drawings drawn with the same size as the objects are called full sized drawing.
• It is not convenient, always, to draw drawings of the object to its actual size. e.g. Buildings, Heavy machines, Bridges, Watches, Electronic devices etc.
• Hence scales are used to prepare drawing at

      Full size

      Reduced size

      Enlarged size