Unit - 4
Complex Variable - Differentiation
Q1) Find-
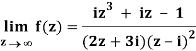
A1)
Here we have-
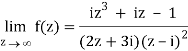
Divide numerator and denominator by z3, we get-
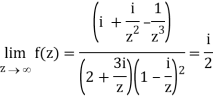
Q2) If f(z) is a complex function given below, then discuss dz/dz at z = 0
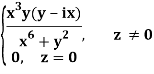
A2)
If z→0 along radius vector y = mx
= 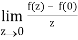
= 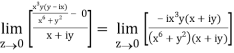
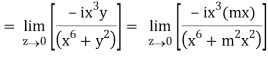
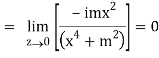
But along v = x3,
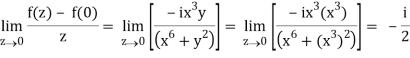
In different paths we get different value of df/dz that means 0 and –i/2, in that case the function is not differentiable at z = 0.
Q3) Prove: The necessary condition for a function  to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
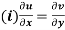 (ii)
(ii) 
Provided, 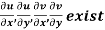
A3)
Let  be an analytic function in region R.
be an analytic function in region R.
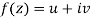
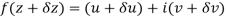

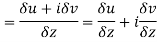
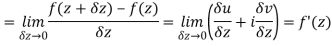
Along real axis
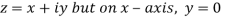
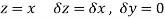
Then f’(z), becomes-
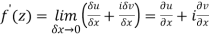 ………… (1)
………… (1)
Along imaginary axis
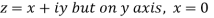
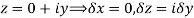
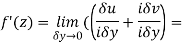
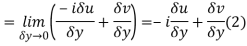
From equation (1) and (2)
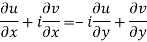
Equating real and imaginary parts
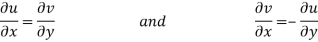
Therefore-
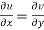 and
and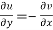
These are called Cauchy Riemann Equations.
Q4) What is Analytic function and write is necessary conditions?
A4)
A function 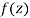 is said to be analytic at a point
is said to be analytic at a point 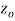 if f is differentiable not only at
if f is differentiable not only at  but every point of some neighborhood at
but every point of some neighborhood at  .
.
Note-
1. A point at which the function is not differentiable is called singular point.
2. A function which is analytic everywhere is called an entire function.
3. An entire function is always analytic, differentiable and continuous function. (converse is not true)
4. Analytic function is always differentiable and continuous but converse is not true.
5. A differentiable function is always continuous but converse is not true.
The necessary condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1.  …………. (1)
…………. (1)
2. 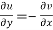 ……...…. (2)
……...…. (2)
Provided 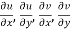 exists
exists
Equation (1) and (2) are known as Cauchy-Riemann equations.
The sufficient condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 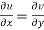 …………. (1)
…………. (1)
2. 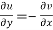 ……...…. (2)
……...…. (2)
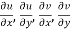 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Q5) State and prove sufficient condition for analytic functions
A5)
Statement – The sufficient condition for a function 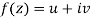 to be analytic at all points in a region R are
to be analytic at all points in a region R are
1. 
2. 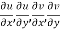 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Proof:- Let f(z) be a simple valued function having  at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
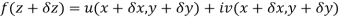
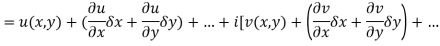
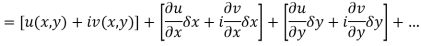
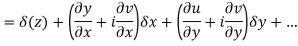
Ignoring the terms of second power and higher power
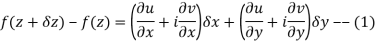
We know C-R equation
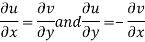
Replacing 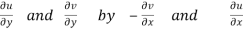
Respectively in (1) we get
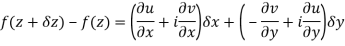
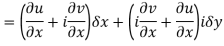
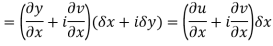
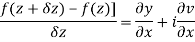
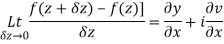
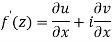
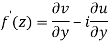
Q6) Show that  is analytic at
is analytic at 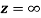
A6)
The function f(z) is analytic at 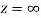 if the function
if the function  is analytic at z=0
is analytic at z=0
Since 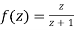
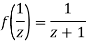
Now 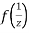 is differentiable at z=0 and at all points in its neighbourhood Hence the function
is differentiable at z=0 and at all points in its neighbourhood Hence the function  is analytic at z=0 and in turn f(z) is analytic at
is analytic at z=0 and in turn f(z) is analytic at 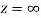
Q7) If w = log z, then find  . Also determine where w is non-analytic.
. Also determine where w is non-analytic.
A7)
Here we have 
Therefore-
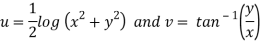
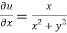 and
and
Again-
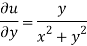
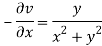
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0).
So that w is analytic everywhere but not at z = 0
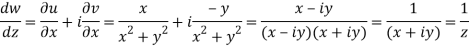
Q8) Prove that the function  is an analytical function.
is an analytical function.
A8)
Let 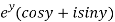 =u+iv
=u+iv
Let  =u and
=u and  =v
=v
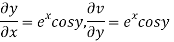
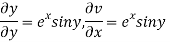

Hence C-R-Equation satisfied.
Q9) Prove that 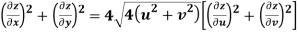
A9)
Given that
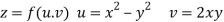
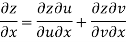
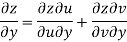
Since 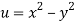

V=2xy

Now 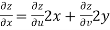
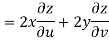
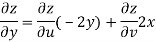
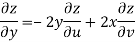
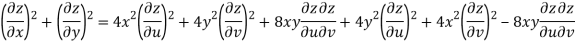
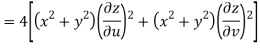
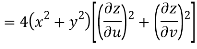
But 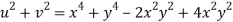

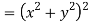
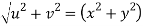
Hence 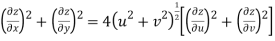
Q10) Show that polar form of C-R equations are-
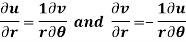
A10)
z = x + iy = 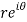
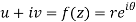
U and v are expressed in terms of r and θ.
Differentiate it partially w.r.t. r and θ, we get-
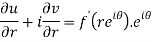
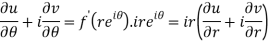
By equating real and imaginary parts, we get-

Q11) Prove Harmonic function theorem.
A11)
A function which satisfies the Laplace equation is known as a harmonic function.
Theorem- if f(z) = u + iv is an analytic function, then u and v are both harmonic functions.
Proof:
Suppose f(z) = u + iv, be an analytic function, then we have
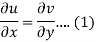
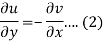
Differentiate (1) with respect to x, we get
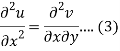
Differentiate (2) with respect to y, we get
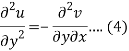
Add 3 and 4-
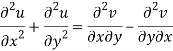
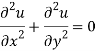
Similarly-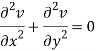
So that u and v are harmonic functions.
Q12) Prove that 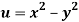 and
and  are harmonic functions of (x, y).
are harmonic functions of (x, y).
A12)
We have 
Now
u/x = 2x, 2u/x2 = 2, u/y = -2y, 2u/y2 = -2
2u/x2 + 2u/y2 = 2 – 2 = 0
Here it satisfies Laplace equation so that u (x, y) is harmonic.
Now-
v = y/(x2 + y2), v/x = - 2xy/(x2 + y2)2
2v/x2 = 
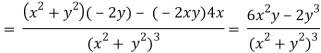
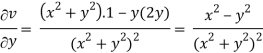
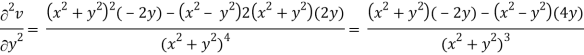
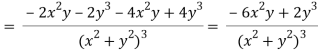
On adding the above results-
We get-
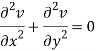
So that v(x, y) is also a harmonic function.
Q13) Find the harmonic conjugate function of the function U (x, y) = 2x (1 – y).
A13)
We have,
U(x, y) = 2x (1 – y)
Let V is the harmonic conjugate of U.
So that by total differentiation,
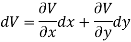
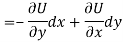
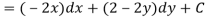
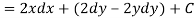
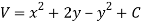
Hence the harmonic conjugate of U is 
Q14) Find the conformal transformation of 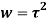 .
.
A14)
Let 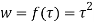
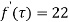
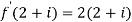
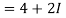
Q15) Show that the mapping  is conformal in the whole of the z plane.
is conformal in the whole of the z plane.
A15)
Let z=x+iy
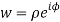
Then 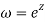
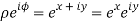
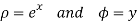
Consider the mapping of the straight line x=a in z plane the w plane which gives 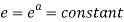 which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into 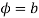 which is a radius vector in the w plane.
which is a radius vector in the w plane.
The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector 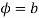 is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping 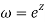 is conformal.
is conformal.
Q160 Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
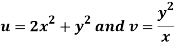
A16)
Let  …………. (1)
…………. (1)
Differentiate (1), we get-
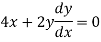
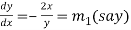 …………… (2)
…………… (2)
Now-
 …………….. (3)
…………….. (3)
Differentiate (3), we get-
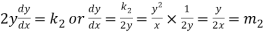 ………. (4)
………. (4)
As we know that for the condition for orthogonallity, from (2) and (4)
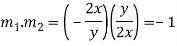
So that these two curves cut orthogonally.
Here,

And
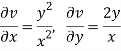
Here the C-R equation is not satisfied so that the function u + iv is not analytic.
Hence the transformation is not conformal.
Q16) Find the bi-linear transformation which aps points z=2,1,0ontpo the points w=1,0,i
A17)
Let 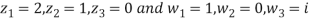

Thus we have
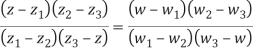
=
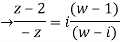
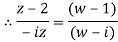
Q17) How that the bilinear transformation w=  transforms
transforms 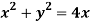 in the z-plane to 4u+3=0 in w-plane.
in the z-plane to 4u+3=0 in w-plane.
A18)
Consider  the circle in z-plane
the circle in z-plane
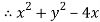 = 0
= 0
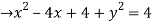
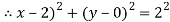
Thus, centre of the circle is (h,k)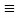 c(2,0) and radius r=2.
c(2,0) and radius r=2.
Thus in z-plane it is given as 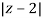 =2....(1)
=2....(1)
Consider w= 
W(z-4) = 2z+3
Wz-4w=2z+3
Wz-2z=4w+3
Z(w-2) = (4w+3)
 z =
z = 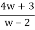
z-2 = 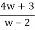 - 2
- 2
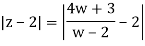
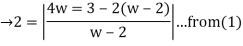
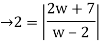
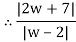
Q18) Theorem: If W=f(z) represents a conformal transformation of a domain D in the z-plane into a domain D of the W plane then f(z) is an analytic function of z in D. Prove this theorem.
A19)
Proof: We have u+iv=u(x,y)+iv(x,y)
So that u=u(x, y) and v=v(x,y)
Let ds and 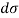 denote elementary arc length in the z-plane and w-plane respectively Then
denote elementary arc length in the z-plane and w-plane respectively Then
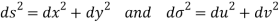
Now 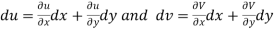
Hence 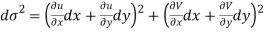
Or 
Where 
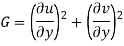
Now 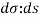 is independent of direction if
is independent of direction if
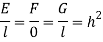
Where h depends on x and y only and is not zero. Thus the conditions for an Isogonal transformation
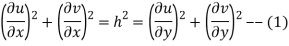
And 
The equation are satisfied if we get
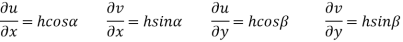
Then substituting these values in 2 we get
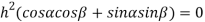
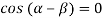
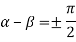
Taking 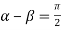 i.e.
i.e. 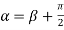
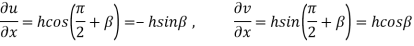
Also 
Hence 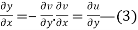
Similarly  i.e.
i.e. 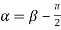
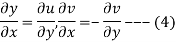
The equation (4) are the well-known Cauchy -Reimann
Unit - 4
Complex Variable - Differentiation
Q1) Find-
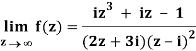
A1)
Here we have-
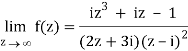
Divide numerator and denominator by z3, we get-
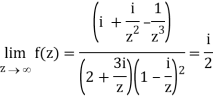
Q2) If f(z) is a complex function given below, then discuss dz/dz at z = 0
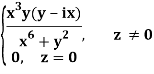
A2)
If z→0 along radius vector y = mx
= 
= 
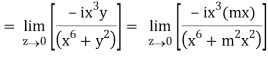
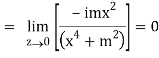
But along v = x3,
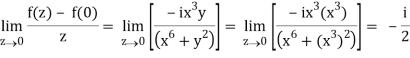
In different paths we get different value of df/dz that means 0 and –i/2, in that case the function is not differentiable at z = 0.
Q3) Prove: The necessary condition for a function  to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
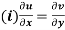 (ii)
(ii) 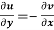
Provided, 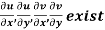
A3)
Let  be an analytic function in region R.
be an analytic function in region R.
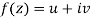
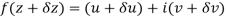
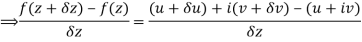
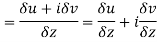
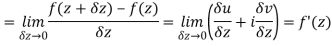
Along real axis
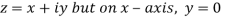
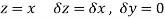
Then f’(z), becomes-
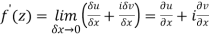 ………… (1)
………… (1)
Along imaginary axis
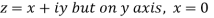
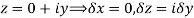
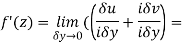
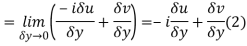
From equation (1) and (2)

Equating real and imaginary parts
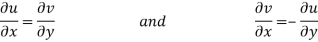
Therefore-
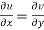 and
and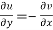
These are called Cauchy Riemann Equations.
Q4) What is Analytic function and write is necessary conditions?
A4)
A function 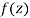 is said to be analytic at a point
is said to be analytic at a point 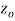 if f is differentiable not only at
if f is differentiable not only at  but every point of some neighborhood at
but every point of some neighborhood at 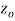 .
.
Note-
1. A point at which the function is not differentiable is called singular point.
2. A function which is analytic everywhere is called an entire function.
3. An entire function is always analytic, differentiable and continuous function. (converse is not true)
4. Analytic function is always differentiable and continuous but converse is not true.
5. A differentiable function is always continuous but converse is not true.
The necessary condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 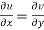 …………. (1)
…………. (1)
2. 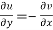 ……...…. (2)
……...…. (2)
Provided 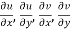 exists
exists
Equation (1) and (2) are known as Cauchy-Riemann equations.
The sufficient condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 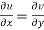 …………. (1)
…………. (1)
2. 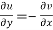 ……...…. (2)
……...…. (2)
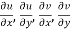 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Q5) State and prove sufficient condition for analytic functions
A5)
Statement – The sufficient condition for a function 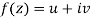 to be analytic at all points in a region R are
to be analytic at all points in a region R are
1. 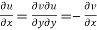
2. 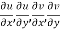 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Proof:- Let f(z) be a simple valued function having  at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
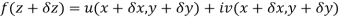
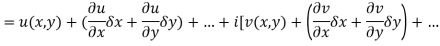
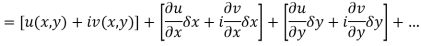
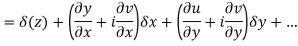
Ignoring the terms of second power and higher power
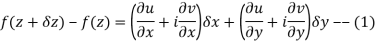
We know C-R equation
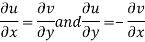
Replacing 
Respectively in (1) we get
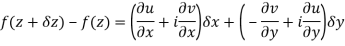
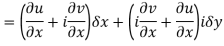
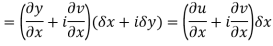
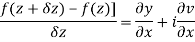
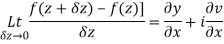
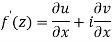
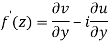
Q6) Show that 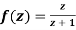 is analytic at
is analytic at 
A6)
The function f(z) is analytic at 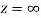 if the function
if the function 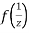 is analytic at z=0
is analytic at z=0
Since 
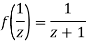
Now 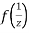 is differentiable at z=0 and at all points in its neighbourhood Hence the function
is differentiable at z=0 and at all points in its neighbourhood Hence the function  is analytic at z=0 and in turn f(z) is analytic at
is analytic at z=0 and in turn f(z) is analytic at 
Q7) If w = log z, then find  . Also determine where w is non-analytic.
. Also determine where w is non-analytic.
A7)
Here we have 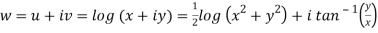
Therefore-
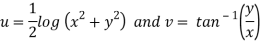
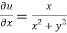 and
and
Again-
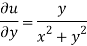
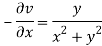
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0).
So that w is analytic everywhere but not at z = 0
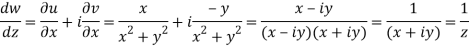
Q8) Prove that the function 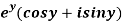 is an analytical function.
is an analytical function.
A8)
Let 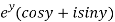 =u+iv
=u+iv
Let 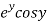 =u and
=u and 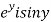 =v
=v
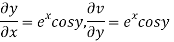
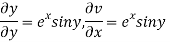
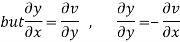
Hence C-R-Equation satisfied.
Q9) Prove that 
A9)
Given that
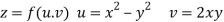
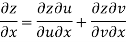
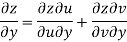
Since 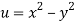

V=2xy

Now 
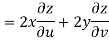
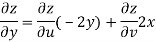

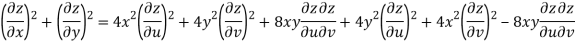
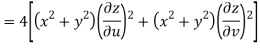
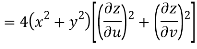
But 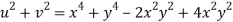
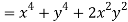
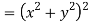
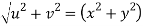
Hence 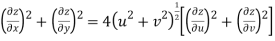
Q10) Show that polar form of C-R equations are-
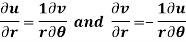
A10)
z = x + iy = 
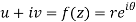
U and v are expressed in terms of r and θ.
Differentiate it partially w.r.t. r and θ, we get-
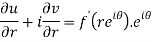
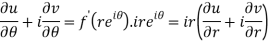
By equating real and imaginary parts, we get-
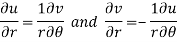
Q11) Prove Harmonic function theorem.
A11)
A function which satisfies the Laplace equation is known as a harmonic function.
Theorem- if f(z) = u + iv is an analytic function, then u and v are both harmonic functions.
Proof:
Suppose f(z) = u + iv, be an analytic function, then we have
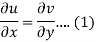
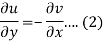
Differentiate (1) with respect to x, we get
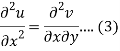
Differentiate (2) with respect to y, we get
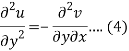
Add 3 and 4-
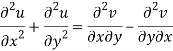
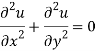
Similarly-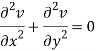
So that u and v are harmonic functions.
Q12) Prove that 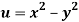 and
and 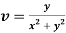 are harmonic functions of (x, y).
are harmonic functions of (x, y).
A12)
We have 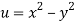
Now
u/x = 2x, 2u/x2 = 2, u/y = -2y, 2u/y2 = -2
2u/x2 + 2u/y2 = 2 – 2 = 0
Here it satisfies Laplace equation so that u (x, y) is harmonic.
Now-
v = y/(x2 + y2), v/x = - 2xy/(x2 + y2)2
2v/x2 = 
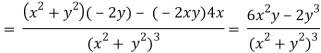
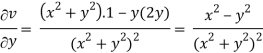
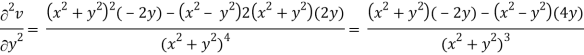
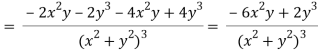
On adding the above results-
We get-
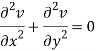
So that v(x, y) is also a harmonic function.
Q13) Find the harmonic conjugate function of the function U (x, y) = 2x (1 – y).
A13)
We have,
U(x, y) = 2x (1 – y)
Let V is the harmonic conjugate of U.
So that by total differentiation,
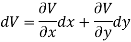

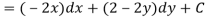
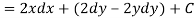
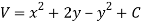
Hence the harmonic conjugate of U is 
Q14) Find the conformal transformation of  .
.
A14)
Let 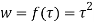
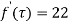
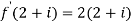

Q15) Show that the mapping 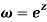 is conformal in the whole of the z plane.
is conformal in the whole of the z plane.
A15)
Let z=x+iy
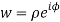
Then 
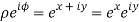

Consider the mapping of the straight line x=a in z plane the w plane which gives 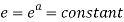 which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into  which is a radius vector in the w plane.
which is a radius vector in the w plane.
The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector 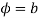 is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping 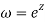 is conformal.
is conformal.
Q160 Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
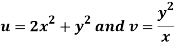
A16)
Let  …………. (1)
…………. (1)
Differentiate (1), we get-
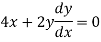
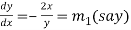 …………… (2)
…………… (2)
Now-
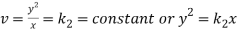 …………….. (3)
…………….. (3)
Differentiate (3), we get-
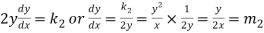 ………. (4)
………. (4)
As we know that for the condition for orthogonallity, from (2) and (4)
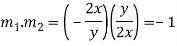
So that these two curves cut orthogonally.
Here,
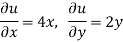
And
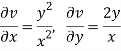
Here the C-R equation is not satisfied so that the function u + iv is not analytic.
Hence the transformation is not conformal.
Q16) Find the bi-linear transformation which aps points z=2,1,0ontpo the points w=1,0,i
A17)
Let 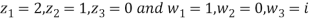
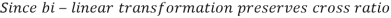
Thus we have
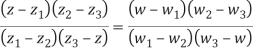
=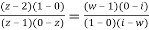
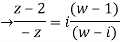
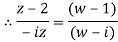
Q17) How that the bilinear transformation w= 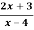 transforms
transforms  in the z-plane to 4u+3=0 in w-plane.
in the z-plane to 4u+3=0 in w-plane.
A18)
Consider 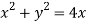 the circle in z-plane
the circle in z-plane
 = 0
= 0
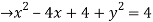
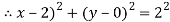
Thus, centre of the circle is (h,k)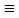 c(2,0) and radius r=2.
c(2,0) and radius r=2.
Thus in z-plane it is given as 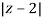 =2....(1)
=2....(1)
Consider w= 
W(z-4) = 2z+3
Wz-4w=2z+3
Wz-2z=4w+3
Z(w-2) = (4w+3)
 z =
z = 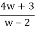
z-2 =  - 2
- 2
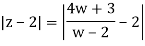
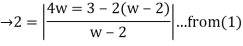

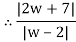
Q18) Theorem: If W=f(z) represents a conformal transformation of a domain D in the z-plane into a domain D of the W plane then f(z) is an analytic function of z in D. Prove this theorem.
A19)
Proof: We have u+iv=u(x,y)+iv(x,y)
So that u=u(x, y) and v=v(x,y)
Let ds and 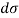 denote elementary arc length in the z-plane and w-plane respectively Then
denote elementary arc length in the z-plane and w-plane respectively Then
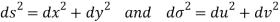
Now 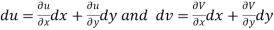
Hence 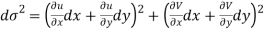
Or 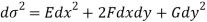
Where 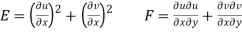
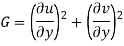
Now 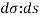 is independent of direction if
is independent of direction if
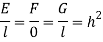
Where h depends on x and y only and is not zero. Thus the conditions for an Isogonal transformation

And 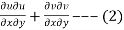
The equation are satisfied if we get
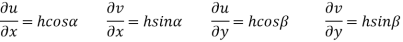
Then substituting these values in 2 we get
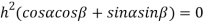
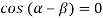

Taking 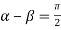 i.e.
i.e. 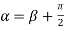
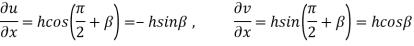
Also 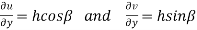
Hence 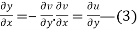
Similarly 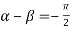 i.e.
i.e. 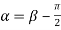
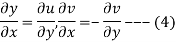
The equation (4) are the well-known Cauchy -Reimann
Unit - 4
Complex Variable - Differentiation
Q1) Find-

A1)
Here we have-
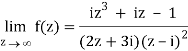
Divide numerator and denominator by z3, we get-
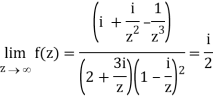
Q2) If f(z) is a complex function given below, then discuss dz/dz at z = 0

A2)
If z→0 along radius vector y = mx
= 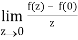
= 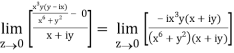
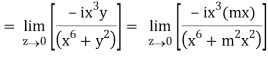

But along v = x3,
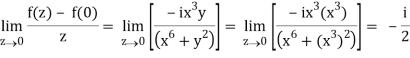
In different paths we get different value of df/dz that means 0 and –i/2, in that case the function is not differentiable at z = 0.
Q3) Prove: The necessary condition for a function 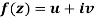 to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
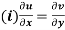 (ii)
(ii) 
Provided, 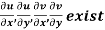
A3)
Let 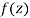 be an analytic function in region R.
be an analytic function in region R.
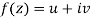
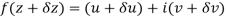
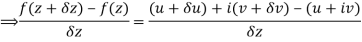
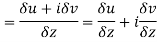
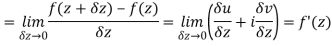
Along real axis
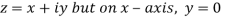
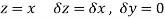
Then f’(z), becomes-
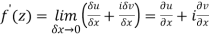 ………… (1)
………… (1)
Along imaginary axis
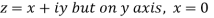
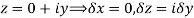
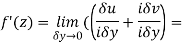
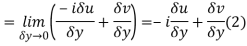
From equation (1) and (2)
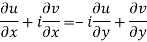
Equating real and imaginary parts
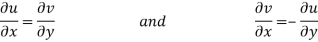
Therefore-
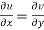 and
and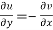
These are called Cauchy Riemann Equations.
Q4) What is Analytic function and write is necessary conditions?
A4)
A function 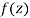 is said to be analytic at a point
is said to be analytic at a point 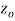 if f is differentiable not only at
if f is differentiable not only at 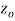 but every point of some neighborhood at
but every point of some neighborhood at 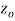 .
.
Note-
1. A point at which the function is not differentiable is called singular point.
2. A function which is analytic everywhere is called an entire function.
3. An entire function is always analytic, differentiable and continuous function. (converse is not true)
4. Analytic function is always differentiable and continuous but converse is not true.
5. A differentiable function is always continuous but converse is not true.
The necessary condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 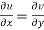 …………. (1)
…………. (1)
2. 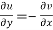 ……...…. (2)
……...…. (2)
Provided  exists
exists
Equation (1) and (2) are known as Cauchy-Riemann equations.
The sufficient condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 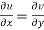 …………. (1)
…………. (1)
2. 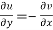 ……...…. (2)
……...…. (2)
 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Q5) State and prove sufficient condition for analytic functions
A5)
Statement – The sufficient condition for a function 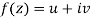 to be analytic at all points in a region R are
to be analytic at all points in a region R are
1. 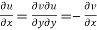
2.  are continuous function of x and y in region R.
are continuous function of x and y in region R.
Proof:- Let f(z) be a simple valued function having  at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
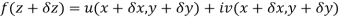
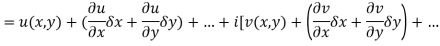
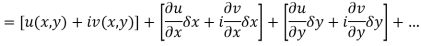
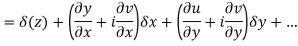
Ignoring the terms of second power and higher power

We know C-R equation
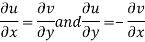
Replacing 
Respectively in (1) we get
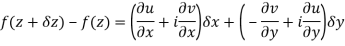
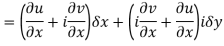
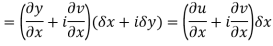

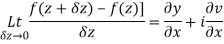
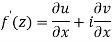
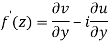
Q6) Show that 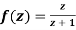 is analytic at
is analytic at 
A6)
The function f(z) is analytic at 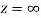 if the function
if the function  is analytic at z=0
is analytic at z=0
Since 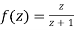
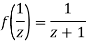
Now 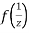 is differentiable at z=0 and at all points in its neighbourhood Hence the function
is differentiable at z=0 and at all points in its neighbourhood Hence the function 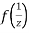 is analytic at z=0 and in turn f(z) is analytic at
is analytic at z=0 and in turn f(z) is analytic at 
Q7) If w = log z, then find 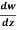 . Also determine where w is non-analytic.
. Also determine where w is non-analytic.
A7)
Here we have 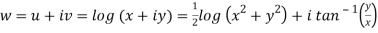
Therefore-
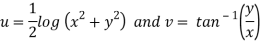
 and
and
Again-
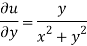
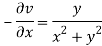
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0).
So that w is analytic everywhere but not at z = 0

Q8) Prove that the function 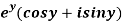 is an analytical function.
is an analytical function.
A8)
Let 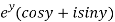 =u+iv
=u+iv
Let 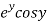 =u and
=u and 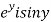 =v
=v
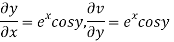
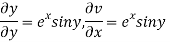
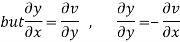
Hence C-R-Equation satisfied.
Q9) Prove that 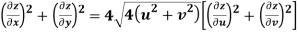
A9)
Given that
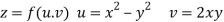
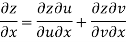
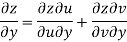
Since 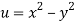

V=2xy

Now 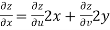
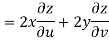
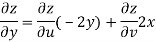
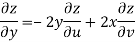
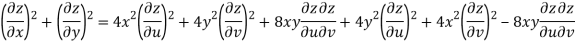
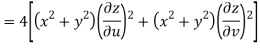
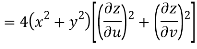
But 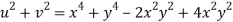

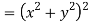
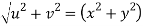
Hence 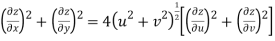
Q10) Show that polar form of C-R equations are-
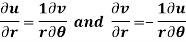
A10)
z = x + iy = 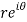
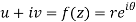
U and v are expressed in terms of r and θ.
Differentiate it partially w.r.t. r and θ, we get-
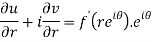

By equating real and imaginary parts, we get-
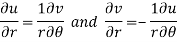
Q11) Prove Harmonic function theorem.
A11)
A function which satisfies the Laplace equation is known as a harmonic function.
Theorem- if f(z) = u + iv is an analytic function, then u and v are both harmonic functions.
Proof:
Suppose f(z) = u + iv, be an analytic function, then we have
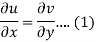
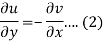
Differentiate (1) with respect to x, we get
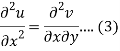
Differentiate (2) with respect to y, we get
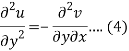
Add 3 and 4-
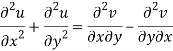
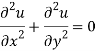
Similarly-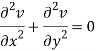
So that u and v are harmonic functions.
Q12) Prove that  and
and  are harmonic functions of (x, y).
are harmonic functions of (x, y).
A12)
We have 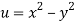
Now
u/x = 2x, 2u/x2 = 2, u/y = -2y, 2u/y2 = -2
2u/x2 + 2u/y2 = 2 – 2 = 0
Here it satisfies Laplace equation so that u (x, y) is harmonic.
Now-
v = y/(x2 + y2), v/x = - 2xy/(x2 + y2)2
2v/x2 = 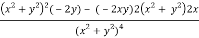
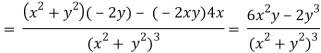
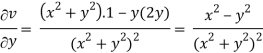
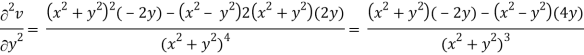
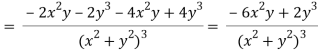
On adding the above results-
We get-
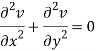
So that v(x, y) is also a harmonic function.
Q13) Find the harmonic conjugate function of the function U (x, y) = 2x (1 – y).
A13)
We have,
U(x, y) = 2x (1 – y)
Let V is the harmonic conjugate of U.
So that by total differentiation,
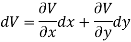
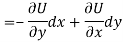
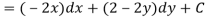
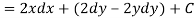
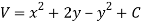
Hence the harmonic conjugate of U is 
Q14) Find the conformal transformation of 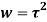 .
.
A14)
Let 
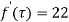
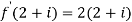
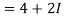
Q15) Show that the mapping  is conformal in the whole of the z plane.
is conformal in the whole of the z plane.
A15)
Let z=x+iy
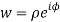
Then 
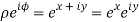
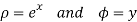
Consider the mapping of the straight line x=a in z plane the w plane which gives 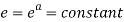 which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into 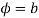 which is a radius vector in the w plane.
which is a radius vector in the w plane.
The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector 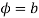 is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping 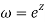 is conformal.
is conformal.
Q160 Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
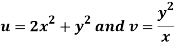
A16)
Let 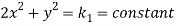 …………. (1)
…………. (1)
Differentiate (1), we get-
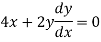
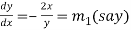 …………… (2)
…………… (2)
Now-
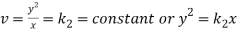 …………….. (3)
…………….. (3)
Differentiate (3), we get-
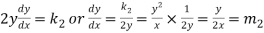 ………. (4)
………. (4)
As we know that for the condition for orthogonallity, from (2) and (4)
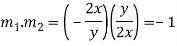
So that these two curves cut orthogonally.
Here,
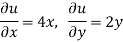
And
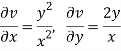
Here the C-R equation is not satisfied so that the function u + iv is not analytic.
Hence the transformation is not conformal.
Q16) Find the bi-linear transformation which aps points z=2,1,0ontpo the points w=1,0,i
A17)
Let 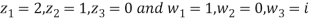
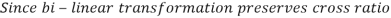
Thus we have
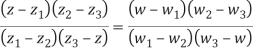
=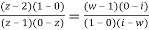
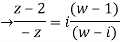
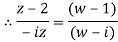
Q17) How that the bilinear transformation w= 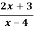 transforms
transforms 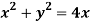 in the z-plane to 4u+3=0 in w-plane.
in the z-plane to 4u+3=0 in w-plane.
A18)
Consider  the circle in z-plane
the circle in z-plane
 = 0
= 0

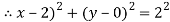
Thus, centre of the circle is (h,k)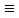 c(2,0) and radius r=2.
c(2,0) and radius r=2.
Thus in z-plane it is given as 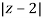 =2....(1)
=2....(1)
Consider w= 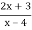
W(z-4) = 2z+3
Wz-4w=2z+3
Wz-2z=4w+3
Z(w-2) = (4w+3)
 z =
z = 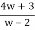
z-2 = 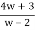 - 2
- 2
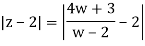
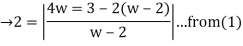
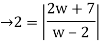
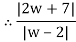
Q18) Theorem: If W=f(z) represents a conformal transformation of a domain D in the z-plane into a domain D of the W plane then f(z) is an analytic function of z in D. Prove this theorem.
A19)
Proof: We have u+iv=u(x,y)+iv(x,y)
So that u=u(x, y) and v=v(x,y)
Let ds and 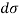 denote elementary arc length in the z-plane and w-plane respectively Then
denote elementary arc length in the z-plane and w-plane respectively Then
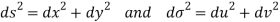
Now 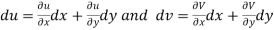
Hence 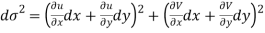
Or 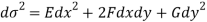
Where 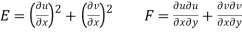
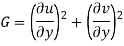
Now  is independent of direction if
is independent of direction if

Where h depends on x and y only and is not zero. Thus the conditions for an Isogonal transformation
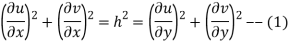
And 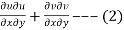
The equation are satisfied if we get
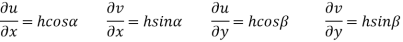
Then substituting these values in 2 we get
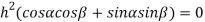

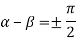
Taking  i.e.
i.e. 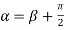
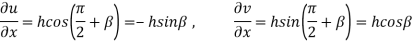
Also 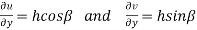
Hence 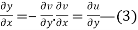
Similarly  i.e.
i.e. 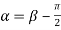
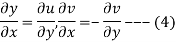
The equation (4) are the well-known Cauchy -Reimann
Unit - 4
Complex Variable - Differentiation
Q1) Find-
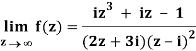
A1)
Here we have-
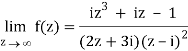
Divide numerator and denominator by z3, we get-
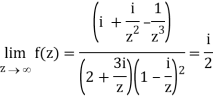
Q2) If f(z) is a complex function given below, then discuss dz/dz at z = 0
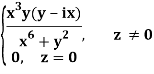
A2)
If z→0 along radius vector y = mx
= 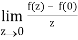
= 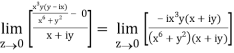
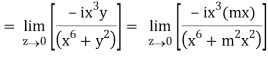
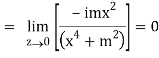
But along v = x3,

In different paths we get different value of df/dz that means 0 and –i/2, in that case the function is not differentiable at z = 0.
Q3) Prove: The necessary condition for a function 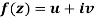 to be analytic at all the points in a region R are
to be analytic at all the points in a region R are
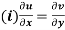 (ii)
(ii) 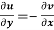
Provided, 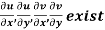
A3)
Let 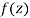 be an analytic function in region R.
be an analytic function in region R.
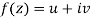

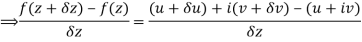
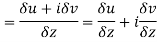
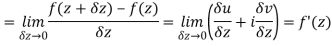
Along real axis
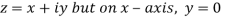
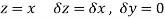
Then f’(z), becomes-
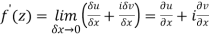 ………… (1)
………… (1)
Along imaginary axis
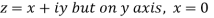
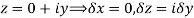
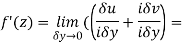
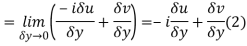
From equation (1) and (2)
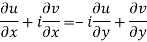
Equating real and imaginary parts
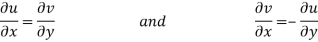
Therefore-
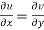 and
and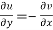
These are called Cauchy Riemann Equations.
Q4) What is Analytic function and write is necessary conditions?
A4)
A function 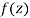 is said to be analytic at a point
is said to be analytic at a point  if f is differentiable not only at
if f is differentiable not only at  but every point of some neighborhood at
but every point of some neighborhood at 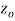 .
.
Note-
1. A point at which the function is not differentiable is called singular point.
2. A function which is analytic everywhere is called an entire function.
3. An entire function is always analytic, differentiable and continuous function. (converse is not true)
4. Analytic function is always differentiable and continuous but converse is not true.
5. A differentiable function is always continuous but converse is not true.
The necessary condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1.  …………. (1)
…………. (1)
2. 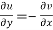 ……...…. (2)
……...…. (2)
Provided 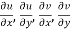 exists
exists
Equation (1) and (2) are known as Cauchy-Riemann equations.
The sufficient condition for f(z) to be analytic-
f(z) = u + i(v) is to be analytic at all the points in a region R are-
1. 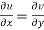 …………. (1)
…………. (1)
2. 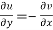 ……...…. (2)
……...…. (2)
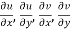 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Q5) State and prove sufficient condition for analytic functions
A5)
Statement – The sufficient condition for a function 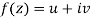 to be analytic at all points in a region R are
to be analytic at all points in a region R are
1. 
2. 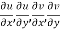 are continuous function of x and y in region R.
are continuous function of x and y in region R.
Proof:- Let f(z) be a simple valued function having 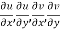 at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
at each point in the region R. Then Cauchy-Reimann equation are satisfied by Taylor’s Theorem
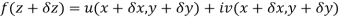
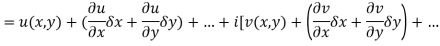
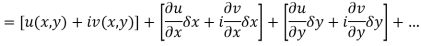
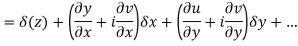
Ignoring the terms of second power and higher power
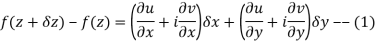
We know C-R equation
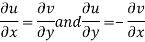
Replacing 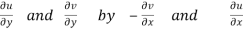
Respectively in (1) we get
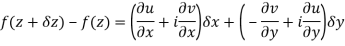
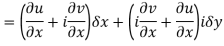
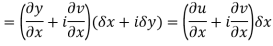

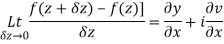
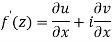
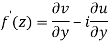
Q6) Show that 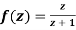 is analytic at
is analytic at 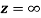
A6)
The function f(z) is analytic at 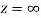 if the function
if the function  is analytic at z=0
is analytic at z=0
Since 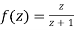
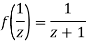
Now 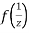 is differentiable at z=0 and at all points in its neighbourhood Hence the function
is differentiable at z=0 and at all points in its neighbourhood Hence the function  is analytic at z=0 and in turn f(z) is analytic at
is analytic at z=0 and in turn f(z) is analytic at 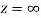
Q7) If w = log z, then find  . Also determine where w is non-analytic.
. Also determine where w is non-analytic.
A7)
Here we have 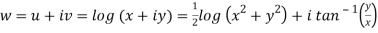
Therefore-
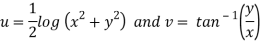
 and
and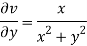
Again-
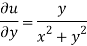
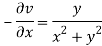
Hence the C-R conditions are satisfied also the partial derivatives are continuous except at (0 , 0).
So that w is analytic everywhere but not at z = 0
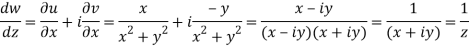
Q8) Prove that the function 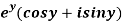 is an analytical function.
is an analytical function.
A8)
Let  =u+iv
=u+iv
Let 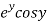 =u and
=u and 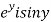 =v
=v
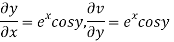
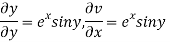
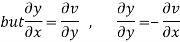
Hence C-R-Equation satisfied.
Q9) Prove that 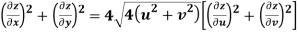
A9)
Given that
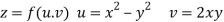
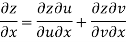

Since 

V=2xy

Now 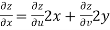
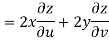
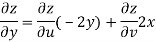
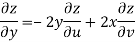
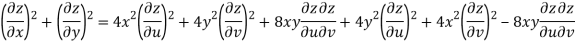
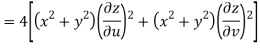
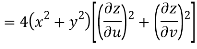
But 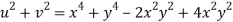
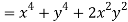
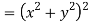
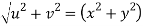
Hence 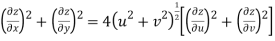
Q10) Show that polar form of C-R equations are-
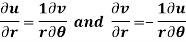
A10)
z = x + iy = 
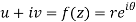
U and v are expressed in terms of r and θ.
Differentiate it partially w.r.t. r and θ, we get-
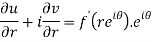
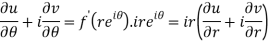
By equating real and imaginary parts, we get-
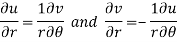
Q11) Prove Harmonic function theorem.
A11)
A function which satisfies the Laplace equation is known as a harmonic function.
Theorem- if f(z) = u + iv is an analytic function, then u and v are both harmonic functions.
Proof:
Suppose f(z) = u + iv, be an analytic function, then we have
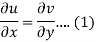
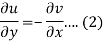
Differentiate (1) with respect to x, we get
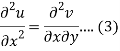
Differentiate (2) with respect to y, we get
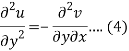
Add 3 and 4-
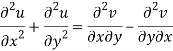
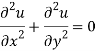
Similarly-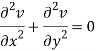
So that u and v are harmonic functions.
Q12) Prove that 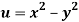 and
and 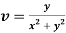 are harmonic functions of (x, y).
are harmonic functions of (x, y).
A12)
We have 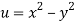
Now
u/x = 2x, 2u/x2 = 2, u/y = -2y, 2u/y2 = -2
2u/x2 + 2u/y2 = 2 – 2 = 0
Here it satisfies Laplace equation so that u (x, y) is harmonic.
Now-
v = y/(x2 + y2), v/x = - 2xy/(x2 + y2)2
2v/x2 = 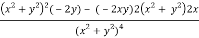
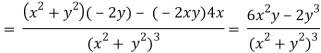
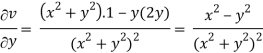
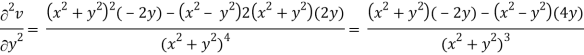
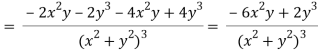
On adding the above results-
We get-
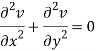
So that v(x, y) is also a harmonic function.
Q13) Find the harmonic conjugate function of the function U (x, y) = 2x (1 – y).
A13)
We have,
U(x, y) = 2x (1 – y)
Let V is the harmonic conjugate of U.
So that by total differentiation,
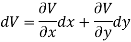
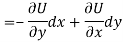
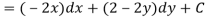
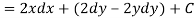
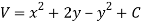
Hence the harmonic conjugate of U is 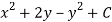
Q14) Find the conformal transformation of 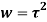 .
.
A14)
Let 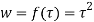
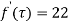
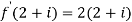
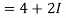
Q15) Show that the mapping 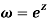 is conformal in the whole of the z plane.
is conformal in the whole of the z plane.
A15)
Let z=x+iy
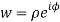
Then 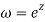
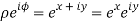
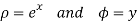
Consider the mapping of the straight line x=a in z plane the w plane which gives 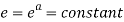 which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into
which is a circle in the w plane in the anticlockwise direction similarly the straight line y=b is mapped into 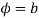 which is a radius vector in the w plane.
which is a radius vector in the w plane.
The angle between the line x=a and y=b in the z plane is a right angle. The corresponding angle in the w plane between the circle e = constant and the radius vector  is also a right angle which establishes that the mapping
is also a right angle which establishes that the mapping  is conformal.
is conformal.
Q160 Show that the curve u = constant and v = constant cut orthogonally at all intersections but the transformation w = u + iv is not conformal. Where-
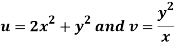
A16)
Let 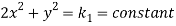 …………. (1)
…………. (1)
Differentiate (1), we get-
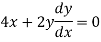
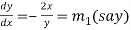 …………… (2)
…………… (2)
Now-
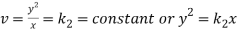 …………….. (3)
…………….. (3)
Differentiate (3), we get-
 ………. (4)
………. (4)
As we know that for the condition for orthogonallity, from (2) and (4)
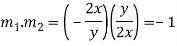
So that these two curves cut orthogonally.
Here,
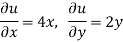
And
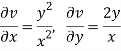
Here the C-R equation is not satisfied so that the function u + iv is not analytic.
Hence the transformation is not conformal.
Q16) Find the bi-linear transformation which aps points z=2,1,0ontpo the points w=1,0,i
A17)
Let 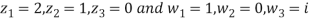
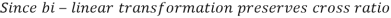
Thus we have
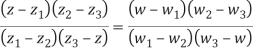
=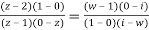
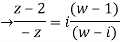
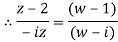
Q17) How that the bilinear transformation w= 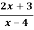 transforms
transforms  in the z-plane to 4u+3=0 in w-plane.
in the z-plane to 4u+3=0 in w-plane.
A18)
Consider  the circle in z-plane
the circle in z-plane
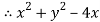 = 0
= 0
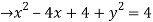
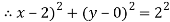
Thus, centre of the circle is (h,k) c(2,0) and radius r=2.
c(2,0) and radius r=2.
Thus in z-plane it is given as  =2....(1)
=2....(1)
Consider w= 
W(z-4) = 2z+3
Wz-4w=2z+3
Wz-2z=4w+3
Z(w-2) = (4w+3)
 z =
z = 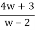
z-2 = 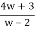 - 2
- 2
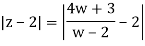

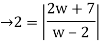
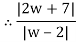
Q18) Theorem: If W=f(z) represents a conformal transformation of a domain D in the z-plane into a domain D of the W plane then f(z) is an analytic function of z in D. Prove this theorem.
A19)
Proof: We have u+iv=u(x,y)+iv(x,y)
So that u=u(x, y) and v=v(x,y)
Let ds and 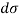 denote elementary arc length in the z-plane and w-plane respectively Then
denote elementary arc length in the z-plane and w-plane respectively Then
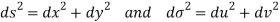
Now 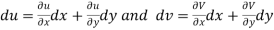
Hence 
Or 
Where 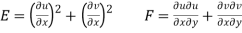
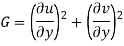
Now 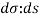 is independent of direction if
is independent of direction if
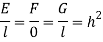
Where h depends on x and y only and is not zero. Thus the conditions for an Isogonal transformation
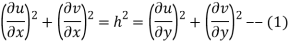
And 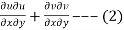
The equation are satisfied if we get
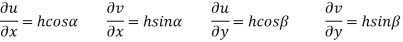
Then substituting these values in 2 we get
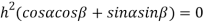
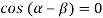
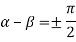
Taking 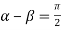 i.e.
i.e. 
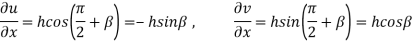
Also 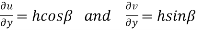
Hence 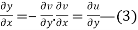
Similarly  i.e.
i.e. 
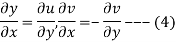
The equation (4) are the well-known Cauchy -Reimann