Unit - 3
Fluid Kinematics
Q1) Explain the terms a) Steady and unsteady flow b) Uniform and non uniform flow?
A1)
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically, 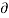 u/
u/  t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
Unsteady flow: It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically, 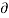 u/
u/  t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,
t not = to 0 , w/ t not = to 0 , p/ t not = to 0 , 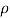 / t not = to 0
/ t not = to 0

Fig no 1 Steady and unsteady flow
Uniform And Non-Uniform Flow
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically, 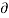 V /
V / 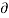 s = 0 when t = constant
s = 0 when t = constant
Non-uniform flow – It is that type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow through non-prismatic conduit.
Mathematically, 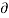 V/
V/  s not = to 0 when t = constant
s not = to 0 when t = constant
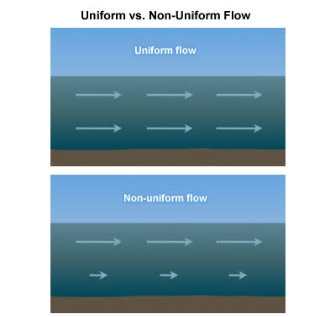
Fig no 2 Uniform and non-uniform flow
Q2) Explain the terms 1) laminar and turbulent flow 2) rotational and irrotational?
A2)
Laminar and Turbulent Flow
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig zag way.
E.g.: High velocity flows in conduit of large size.
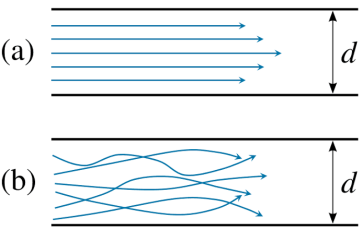
Fig no 3 Laminar and turbulent flow
Rotational and Irrotational Flow
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.
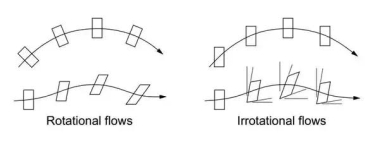
Fig no 4Rotational and irrotational flow
Q3) Explain the terms
a) compressible and incompressible flow
b) ideal and real fluid
A3)
Compressible and Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density () of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically, 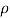 not = to constant
not = to constant
Incompressible Flow – It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically,  = constant
= constant
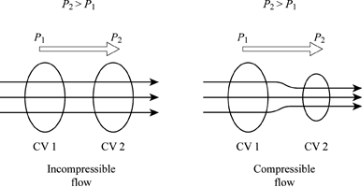
Fig no 5 Compressible and incompressible flow
Ideal and Real Fluid Flow
Ideal Fluid
- Ideal fluid is a fluid that is unmistakable and has no internal resistance to flow (zero viscosity). In addition the proper particles of fluid do not change according to their (thoughtless) center of gravity.
- Fine fluid can travel in a circular pattern, but the particles of each body do not think. Real fluids reflect all of these properties to some degree, but we will usually imitate the fluid as suitable for measuring the performance of a real liquid.
- In doing so, one must be very careful in applying the effects associated with a good liquid to a bad liquid.
- Liquids without viscosity, soil incompatibility, shear strength, and pressure are called fine liquids or complete liquids.
- The right liquid is all fluid that is considered and does not naturally contain those structures. However, a few liquids such as air and water have a very low viscosity, soil thickness, and high resistance to pressure are considered suitable water for all purposes as their thinking helps to simplify statistical analysis.
Real liquid
Liquids with viscosity, earth thickness, shear strength, and pressure are called real liquids. All liquids, in fact, are real liquids and provide a certain amount of resistance during liquid movements. The actual liquid is slightly separated as given below.
1. Newtonian fluid
The actual fluid, where the shear pressure is directly proportional to the shear strain level or velocity gradient is known as the Newtonian fluid as shown in Fig. 1.1. Examples of Newtonian liquids are water, air, paraffin, body oils, and glycerine, etc. These liquids follow Newton's law of viscosity.
2. Non-Newtonian fluid
The actual fluid where the shear pressure does not match the degree of shear hardness or velocity gradient is known as Non-Newtonian fluid. These liquids do not obey Newton's law of viscosity.
3. Ideal plastic or Bingham liquid
Liquids where shear pressure exceeds yield pressure and shear pressure is equal to the level of the shear machine or velocity gradient are more commonly known as the appropriate plastic fluid. Examples of plastic fluids suitable for the suspension of water with clay and fly ash.
4. Thiotropotropic fluid
These are non-Newtonian liquids with a non-linear relationship between stock pressure and angular fluctuations above a certain yield pressure. Examples of thixotropic liquids with crude oil, Bentonitic liquid drilling fluid and printer ink,etc.
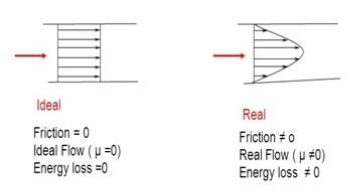
Fig no 6 Ideal and real fluid flow
Q4) Explain one, two and three dimensional flows?
A4)
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x, y) v = f2(x, y) & w = 0
Three-dimensional flow – It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x, y, z) v = f2(x, y, z) & w = f3(x, y, z)
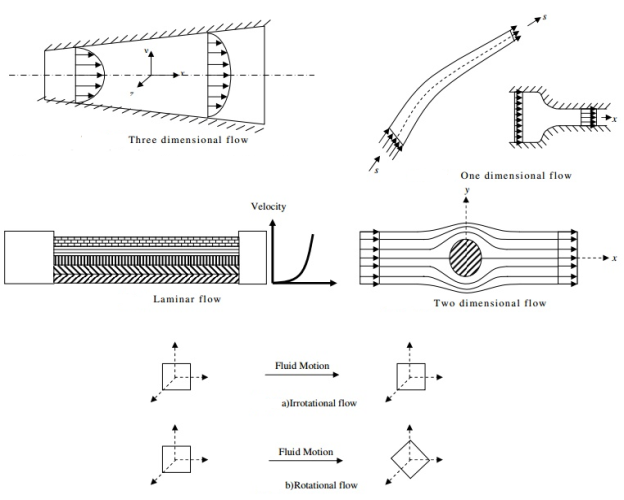
Fig no 7 One two and three dimensional flow
Q5) Explain stream line?
A5)
- A streamline may be defined on as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
- An important concept in aerodynamics research touches on the concept of simple methods. The arrangement is the process followed by the weightless particles as they flow and flow.
- It is easier to visualize a streamline when moving through the body (unlike walking and flow).
- The integrated broadcast around the airfoil and near the cylinder. In both cases, we move with the object and the flow continues from left to right.
- As the streamline is followed by moving particles, at all points along the path the velocity is moving along the path. Since there is no standard object on the road, the magnitude cannot cross a straight line.
- The complexity contained between any two streams remains the same throughout the flow. We can use Bernoulli's figure to associate the pressure and speed associated with easy movement.
- Since there is no weight passing over the airfoil (or cylinder), the surface of the object is the easiest way.
- The planes fly through almost dry air, but we build and test them using air channels, where the model of the plane is stopped and the wind is blown through that model.
- The idea of keeping the airfoil fixed and that the airflow passing through the airfoil can be a little confusing. However you experience the same kind of thing every day
- If you stand on a corner and watch the car pass by, the atmosphere is a little quieter. As the car moves in the air there is aerodynamic force present.
- Now if you were in a car and you put your hand out the window, you could feel the aerodynamic force pushing your hand. It sounds like air is passing through your hand as fast as the car is moving.
- The power of the car is the same, whether you are standing in a corner or riding in a car. The same is true of airplanes. Whether the plane is moving in the air, or the wind is being pushed past the plane, the force is exactly the same.
- It is usually simple, inexpensive, and (in some cases) harmless to check planes in the air tunnel before attempting to fly.
- Streamlines are a family of curves that quickly turn into velocity vector flow. This indicates the direction in which the waterless object will move at any time.
- Stream line, In Liquid Mechanics, a method of synthetic particles suspended in a liquid and traveled with it.
- With a constant flow, the fluid flows but straight lines are arranged. Where the dispersion meets, the velocity of the liquid is very high; when they open, the liquid is quiet. See also laminar flow, turbulent flow.

Fig no 8 Stream line
Q6) Explain path lines?
A6)
- Path lines are the trajectories that are followed by the particles of each body. This can be thought of as "recording" the method of a liquid object in a certain flow of time. The direction of the path to be determined will be determined by the fluid flow of the liquid at each time point.
- Path Lines - A line path is a line followed by water particles as they travel over a period of time. Thus the line of motion indicates the direction of the velocity of the same particle of liquid at successive times. While the formulation shows the speed direction of many liquid particles at the same time.
- The liquid particle always moves the tangent to the grid, so, with a constant flow, the lines of direction and direction are the same.

Fig no 9 Path lines
Q7) Explain streak line and stream tube?
A7)

Fig no 10 Streak line
Redirect is a line that positively connects velocity vectors over a period of time. In other words, the flow diagram presented in simple lines is similar to the abstract flow at the same time.
Fig no 11 Stream line example
A streak line is a curved line formed by a string of liquid particles that pass through a certain area. An example of a streak line is the smoke path from the chimney.
Fig no 12 Streak line example
Path line is the process followed by liquid particles. One example of a path line is a method described as a balloon floating in the air.

Fig no 13 Path line example
With constant flow, streamline, streak line, and path line are the same line. The constant flow of time is called the steady flow. On the other hand, the flow that varies with time is called transient flow. In the short-term flow, streamline, streak line, and path line are all different lines.
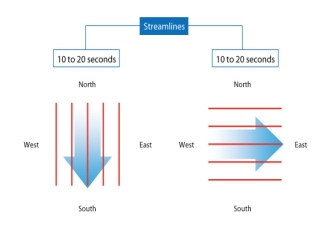
Fig no 14 Stream line direction
The streak line represents all the points that have passed in one place. In this case, where the streak line can be compared to represent the chimney from the chimney, the streak line goes south within the first ten seconds because all the smoke goes south. Then, when the wind blows eastward, all the smoke particles that start to move south (released by Time <10 seconds) begin to be placed eastward. New smoke particles (released Time> 10 seconds) are oriented eastward. After 20 seconds the streak line is in the right corner as shown on the right
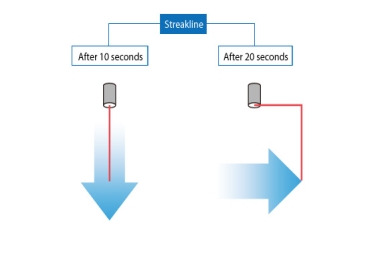
Fig no 15 Streak line direction
The path line goes south within the first 10 seconds as a streak line. The path line can be thought of as a process followed by a balloon floating in the air. When the wind shifts eastward, the balloon begins to move eastward. The path line goes east from where the direction of the wind is changed. As a result, after 20 seconds the path line bends at the right angle as shown on the right
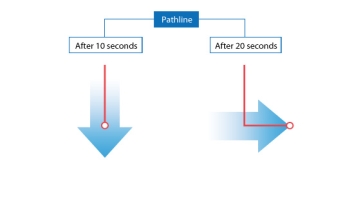
Fig no 16 Path line direction
As can be seen in this chapter, the results of the temporal flow analysis will vary depending on the method used to detect the flow. Understanding these differences is important when looking at analysis and test results.
Q8) Explain stream function?
A8)
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow it is defined as Ψ = f(x, y) such that
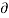 Ψ /
Ψ / 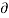 x= v
x= v
And  Ψ /
Ψ /  y = -u
y = -u
Q9) Explain velocity potential function?
A9)
The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y, z, t)
AndØ = f(x, y, z)
u = -  Ø /
Ø / 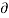 x
x
v = -  Ø /
Ø / 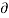 y
y
w = -  Ø /
Ø / 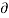 z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q10) Explain one, two and three dimensional continuity equation in Cartesian coordinate?
A10)
One dimensional
The continuity equation is based on the principle of conservation of mass. It states as follows
If no fluid is added or removed from the pipe in any length then the mass passing across different sections shall be same.
Consider two cross-sections of a pipe as shown in fig.
Let, A1&A2 = Areas of the pipe at section 1-1 & section 2-2 respectively.
V1 & V2 = Velocities of the fluid at section 1-1 & section 2-2 respectively.
1 &2 = Densities of the fluid at section 1-1 & section 2-2 respectively.
Total quantity of fluid passing through the section 1-1 = 1A1V1
Total quantity of fluid passing through the section 2-2 = 2A2V2
From the law of conservation of matter
1 A1 V1 = 2 A2 V2
In case of incompressible fluids 1 =2
A1 V1 = A2 V2
Three dimensional
Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.

Fig no 17 3d continuity equation
Let, 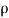 = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
=  X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=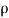 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
=  u dy dz +
u dy dz + 
:. Mass accelerated per unit time, due to flow in x-direction
= 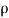 u dydz–[
u dydz–[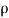 u+
u+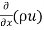 dx]dydz
dx]dydz
= - -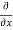 (
(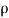 u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
= 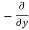 (
(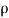 v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
= 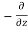 (
(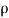 w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[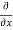 (
(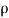 u) +
u) + (
(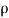 v) +
v) + 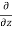 (
( w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
=  (
(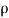 d x, d y d z) ………………….. 2
d x, d y d z) ………………….. 2
From Equation 1& 2
-[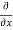 (
(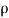 u) +
u) +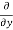 (
( v) +
v) +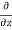 (
(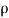 w)] dx dy dz =
w)] dx dy dz = 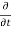 (
(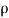 dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
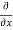 (
(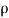 u) +
u) + 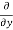 (
(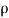 v) +
v) + 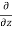 (
( w) +
w) +  =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow (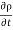 =0) incompressible fluids (
=0) incompressible fluids (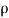 = constant) the equation reduces to
= constant) the equation reduces to
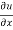 +
+ +
+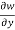 =0
=0
For two dimensional flow eq. Reduce to
Q11) The velocity of a flow is given by v = -x i + 2y j + (5 - 2) k. Derive the equation of streamline passing through a point (2, 1, 1).
A11)
u = -x, v = 2y and w = 5-z
The streamline, put the value of u, v and w
put the value of u, v and w
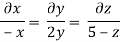
Consider,
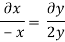
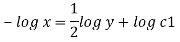
 Stream line passing through x=2, y=1, z= 1
Stream line passing through x=2, y=1, z= 1
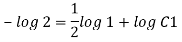



Now consider,


Stream line passing through x=2, z=1


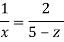

Q12) For the three-dimensional flow, the velocity distribution is given by u-x, v-4-2y, w z-2. What is the stream line equation passing through (1.-2, 3).
A12)
The streamline,
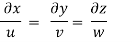
Put the value of u, v and w

Consider 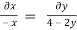
By integrating

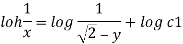
Stream line passing through x=1 y= =-2
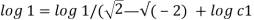
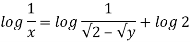
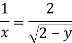
Now considering
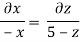

From equation

Q13) The velocity component in a steady flow are u=2kx, v= 2ky, w = - 4kz. What is the through the point (1, 0, 1).
A13)
The streamline,
Put the value of u, v and w
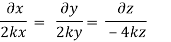
Now considering

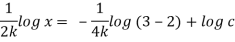
Stream line passing through x = 1, z=3,


This is the equation of streamline passing through the point (1,0,1)
Q14) The velocity field for a two-dimensional flow is given by V= 4x³i-12x²yj. What is the equation of streamlines?
A14)
Velocity vector v = 4x'i-12x²yj
Velocity component u = 4x², v = -12x²y
Stream line
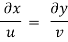
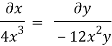
By integrating,
3 log x = -log y + log C
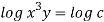

The curve defined by x³y = C₁, C₂, C, ...etc.
Q15) A stream function is given by  check if the flow is rotational and satisfies continuity equation
check if the flow is rotational and satisfies continuity equation
A15)
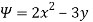
By definition
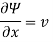
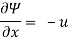
Velocity

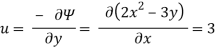
1) Irritational flow
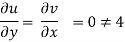
2) Continuity equation
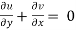
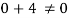
The flow is not continuous
Q16) Determine whether the following specified flows are rotational or otherwise. Determine the expression for the velocity potential in case of irrotational flow
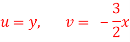
A16)
For rotational flow
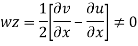
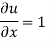

Flow is rotational.
Q17) the velocity potential function is given by an expression 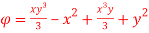
1) Find the velocity components in x and y direction
2) Show that  represents a possible case of flow
represents a possible case of flow
A17)
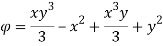
Case I) the partial derivation of 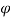 w.r.t x and y are
w.r.t x and y are

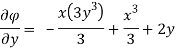
The velocity components u and v
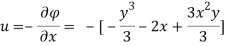
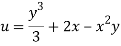

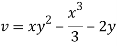
Case II) The value of 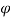 represents a possible case of flow if it satisfy the laplace equation
represents a possible case of flow if it satisfy the laplace equation

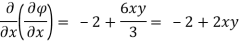
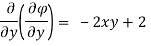

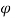 Represents possible case of flow
Represents possible case of flow
Q18) The velocity potential function for a two dimensional flow is  at a point P (4, 5) determine
at a point P (4, 5) determine
1) The velocity
A18)
Velocity potential
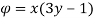
Differential w.r.t x
Hence
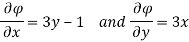
By definition of 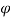
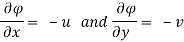
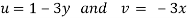
Velocity at P (4, 5)

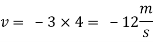
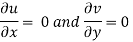

Flow is continuous
Q19) The velocity components for a two-dimensional incompressible flow is given by
1) u = 4 xy + y². v = 6 xy + 3x
2) 
Check whether the flow satisfies continuity?
A19)
(i) u = 4 xy + y², v = 6 xy + 3x
Differentiating

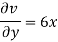
For two dimensional flow continuous equation is
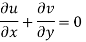
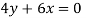
Velocity components does not satisfy the continuity equation
Ii) 
Differentiating
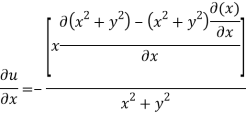

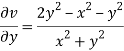
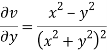
For two dimensional flow continuous equation is
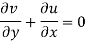
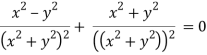
Satisfy the continuous equation.
Q20) The velocity components for a three dimensional incompressible flow is given by: 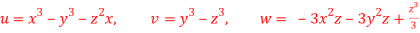
Check whether the flow satisfies continuity?
A20)
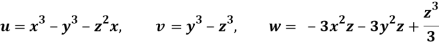
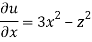
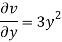
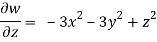
Foe three dimensional equation continuous equation is
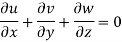


The flow is satisfy
Q21) The velocity field is given by 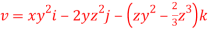 check whether the flow satisfies continuity?
check whether the flow satisfies continuity?
A21)
Velocity field
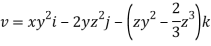
Velocity component
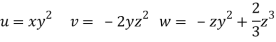
For three dimensional flow
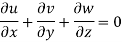
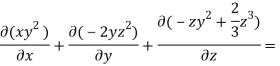
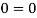
Steady incompressible fluid flow is possible.
Unit - 3
Fluid Kinematics
Q1) Explain the terms a) Steady and unsteady flow b) Uniform and non uniform flow?
A1)
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically, 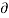 u/
u/ 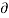 t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
Unsteady flow: It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically,  u/
u/ 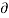 t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,
t not = to 0 , w/ t not = to 0 , p/ t not = to 0 , 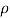 / t not = to 0
/ t not = to 0

Fig no 1 Steady and unsteady flow
Uniform And Non-Uniform Flow
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically,  V /
V /  s = 0 when t = constant
s = 0 when t = constant
Non-uniform flow – It is that type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow through non-prismatic conduit.
Mathematically,  V/
V/  s not = to 0 when t = constant
s not = to 0 when t = constant
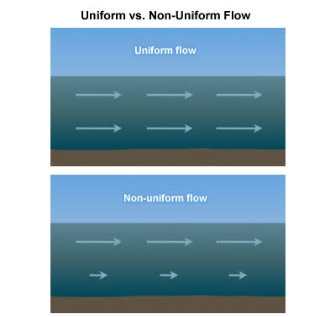
Fig no 2 Uniform and non-uniform flow
Q2) Explain the terms 1) laminar and turbulent flow 2) rotational and irrotational?
A2)
Laminar and Turbulent Flow
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig zag way.
E.g.: High velocity flows in conduit of large size.

Fig no 3 Laminar and turbulent flow
Rotational and Irrotational Flow
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.

Fig no 4Rotational and irrotational flow
Q3) Explain the terms
a) compressible and incompressible flow
b) ideal and real fluid
A3)
Compressible and Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density () of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically,  not = to constant
not = to constant
Incompressible Flow – It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically,  = constant
= constant
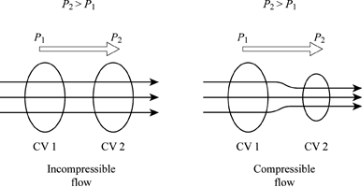
Fig no 5 Compressible and incompressible flow
Ideal and Real Fluid Flow
Ideal Fluid
- Ideal fluid is a fluid that is unmistakable and has no internal resistance to flow (zero viscosity). In addition the proper particles of fluid do not change according to their (thoughtless) center of gravity.
- Fine fluid can travel in a circular pattern, but the particles of each body do not think. Real fluids reflect all of these properties to some degree, but we will usually imitate the fluid as suitable for measuring the performance of a real liquid.
- In doing so, one must be very careful in applying the effects associated with a good liquid to a bad liquid.
- Liquids without viscosity, soil incompatibility, shear strength, and pressure are called fine liquids or complete liquids.
- The right liquid is all fluid that is considered and does not naturally contain those structures. However, a few liquids such as air and water have a very low viscosity, soil thickness, and high resistance to pressure are considered suitable water for all purposes as their thinking helps to simplify statistical analysis.
Real liquid
Liquids with viscosity, earth thickness, shear strength, and pressure are called real liquids. All liquids, in fact, are real liquids and provide a certain amount of resistance during liquid movements. The actual liquid is slightly separated as given below.
1. Newtonian fluid
The actual fluid, where the shear pressure is directly proportional to the shear strain level or velocity gradient is known as the Newtonian fluid as shown in Fig. 1.1. Examples of Newtonian liquids are water, air, paraffin, body oils, and glycerine, etc. These liquids follow Newton's law of viscosity.
2. Non-Newtonian fluid
The actual fluid where the shear pressure does not match the degree of shear hardness or velocity gradient is known as Non-Newtonian fluid. These liquids do not obey Newton's law of viscosity.
3. Ideal plastic or Bingham liquid
Liquids where shear pressure exceeds yield pressure and shear pressure is equal to the level of the shear machine or velocity gradient are more commonly known as the appropriate plastic fluid. Examples of plastic fluids suitable for the suspension of water with clay and fly ash.
4. Thiotropotropic fluid
These are non-Newtonian liquids with a non-linear relationship between stock pressure and angular fluctuations above a certain yield pressure. Examples of thixotropic liquids with crude oil, Bentonitic liquid drilling fluid and printer ink,etc.
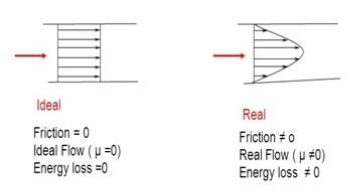
Fig no 6 Ideal and real fluid flow
Q4) Explain one, two and three dimensional flows?
A4)
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x, y) v = f2(x, y) & w = 0
Three-dimensional flow – It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x, y, z) v = f2(x, y, z) & w = f3(x, y, z)
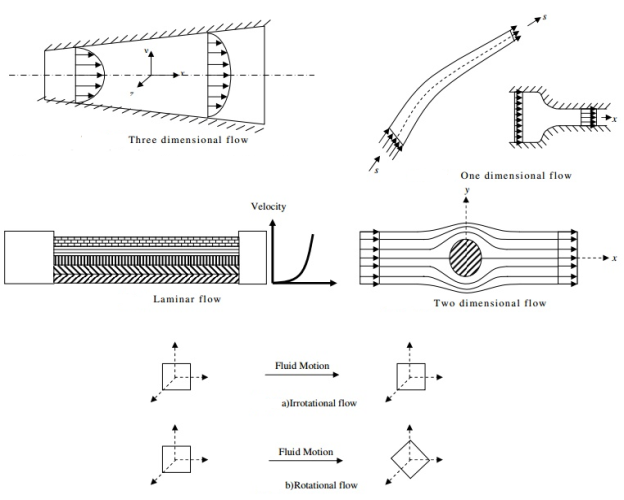
Fig no 7 One two and three dimensional flow
Q5) Explain stream line?
A5)
- A streamline may be defined on as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
- An important concept in aerodynamics research touches on the concept of simple methods. The arrangement is the process followed by the weightless particles as they flow and flow.
- It is easier to visualize a streamline when moving through the body (unlike walking and flow).
- The integrated broadcast around the airfoil and near the cylinder. In both cases, we move with the object and the flow continues from left to right.
- As the streamline is followed by moving particles, at all points along the path the velocity is moving along the path. Since there is no standard object on the road, the magnitude cannot cross a straight line.
- The complexity contained between any two streams remains the same throughout the flow. We can use Bernoulli's figure to associate the pressure and speed associated with easy movement.
- Since there is no weight passing over the airfoil (or cylinder), the surface of the object is the easiest way.
- The planes fly through almost dry air, but we build and test them using air channels, where the model of the plane is stopped and the wind is blown through that model.
- The idea of keeping the airfoil fixed and that the airflow passing through the airfoil can be a little confusing. However you experience the same kind of thing every day
- If you stand on a corner and watch the car pass by, the atmosphere is a little quieter. As the car moves in the air there is aerodynamic force present.
- Now if you were in a car and you put your hand out the window, you could feel the aerodynamic force pushing your hand. It sounds like air is passing through your hand as fast as the car is moving.
- The power of the car is the same, whether you are standing in a corner or riding in a car. The same is true of airplanes. Whether the plane is moving in the air, or the wind is being pushed past the plane, the force is exactly the same.
- It is usually simple, inexpensive, and (in some cases) harmless to check planes in the air tunnel before attempting to fly.
- Streamlines are a family of curves that quickly turn into velocity vector flow. This indicates the direction in which the waterless object will move at any time.
- Stream line, In Liquid Mechanics, a method of synthetic particles suspended in a liquid and traveled with it.
- With a constant flow, the fluid flows but straight lines are arranged. Where the dispersion meets, the velocity of the liquid is very high; when they open, the liquid is quiet. See also laminar flow, turbulent flow.

Fig no 8 Stream line
Q6) Explain path lines?
A6)
- Path lines are the trajectories that are followed by the particles of each body. This can be thought of as "recording" the method of a liquid object in a certain flow of time. The direction of the path to be determined will be determined by the fluid flow of the liquid at each time point.
- Path Lines - A line path is a line followed by water particles as they travel over a period of time. Thus the line of motion indicates the direction of the velocity of the same particle of liquid at successive times. While the formulation shows the speed direction of many liquid particles at the same time.
- The liquid particle always moves the tangent to the grid, so, with a constant flow, the lines of direction and direction are the same.
Fig no 9 Path lines
Q7) Explain streak line and stream tube?
A7)

Fig no 10 Streak line
Redirect is a line that positively connects velocity vectors over a period of time. In other words, the flow diagram presented in simple lines is similar to the abstract flow at the same time.

Fig no 11 Stream line example
A streak line is a curved line formed by a string of liquid particles that pass through a certain area. An example of a streak line is the smoke path from the chimney.

Fig no 12 Streak line example
Path line is the process followed by liquid particles. One example of a path line is a method described as a balloon floating in the air.
Fig no 13 Path line example
With constant flow, streamline, streak line, and path line are the same line. The constant flow of time is called the steady flow. On the other hand, the flow that varies with time is called transient flow. In the short-term flow, streamline, streak line, and path line are all different lines.
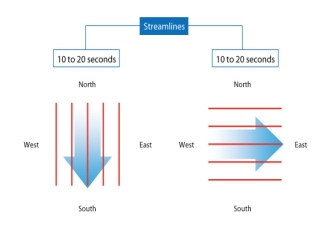
Fig no 14 Stream line direction
The streak line represents all the points that have passed in one place. In this case, where the streak line can be compared to represent the chimney from the chimney, the streak line goes south within the first ten seconds because all the smoke goes south. Then, when the wind blows eastward, all the smoke particles that start to move south (released by Time <10 seconds) begin to be placed eastward. New smoke particles (released Time> 10 seconds) are oriented eastward. After 20 seconds the streak line is in the right corner as shown on the right
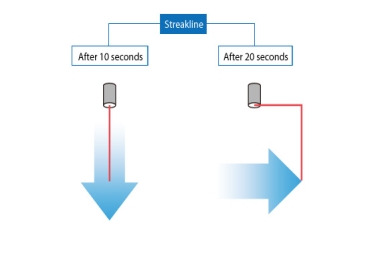
Fig no 15 Streak line direction
The path line goes south within the first 10 seconds as a streak line. The path line can be thought of as a process followed by a balloon floating in the air. When the wind shifts eastward, the balloon begins to move eastward. The path line goes east from where the direction of the wind is changed. As a result, after 20 seconds the path line bends at the right angle as shown on the right
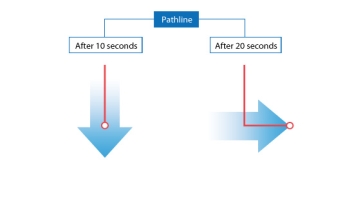
Fig no 16 Path line direction
As can be seen in this chapter, the results of the temporal flow analysis will vary depending on the method used to detect the flow. Understanding these differences is important when looking at analysis and test results.
Q8) Explain stream function?
A8)
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow it is defined as Ψ = f(x, y) such that
 Ψ /
Ψ / 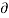 x= v
x= v
And 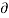 Ψ /
Ψ / 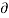 y = -u
y = -u
Q9) Explain velocity potential function?
A9)
The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y, z, t)
AndØ = f(x, y, z)
u = - 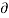 Ø /
Ø /  x
x
v = - 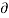 Ø /
Ø /  y
y
w = - 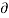 Ø /
Ø / 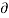 z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q10) Explain one, two and three dimensional continuity equation in Cartesian coordinate?
A10)
One dimensional
The continuity equation is based on the principle of conservation of mass. It states as follows
If no fluid is added or removed from the pipe in any length then the mass passing across different sections shall be same.
Consider two cross-sections of a pipe as shown in fig.
Let, A1&A2 = Areas of the pipe at section 1-1 & section 2-2 respectively.
V1 & V2 = Velocities of the fluid at section 1-1 & section 2-2 respectively.
1 &2 = Densities of the fluid at section 1-1 & section 2-2 respectively.
Total quantity of fluid passing through the section 1-1 = 1A1V1
Total quantity of fluid passing through the section 2-2 = 2A2V2
From the law of conservation of matter
1 A1 V1 = 2 A2 V2
In case of incompressible fluids 1 =2
A1 V1 = A2 V2
Three dimensional
Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.

Fig no 17 3d continuity equation
Let,  = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 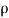 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
= u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
=  u dy dz +
u dy dz + 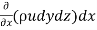
:. Mass accelerated per unit time, due to flow in x-direction
= 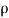 u dydz–[
u dydz–[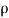 u+
u+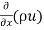 dx]dydz
dx]dydz
= - -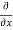 (
(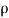 u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
=  (
(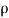 v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
=  (
(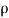 w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[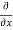 (
(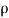 u) +
u) +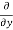 (
(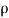 v) +
v) +  (
( w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
=  (
( d x, d y d z) ………………….. 2
d x, d y d z) ………………….. 2
From Equation 1& 2
-[ (
(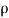 u) +
u) + (
(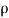 v) +
v) + (
( w)] dx dy dz =
w)] dx dy dz =  (
(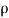 dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
 (
(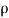 u) +
u) + 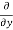 (
(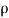 v) +
v) +  (
(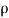 w) +
w) + 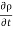 =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow ( =0) incompressible fluids (
=0) incompressible fluids (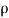 = constant) the equation reduces to
= constant) the equation reduces to
 +
+ +
+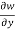 =0
=0
For two dimensional flow eq. Reduce to
Q11) The velocity of a flow is given by v = -x i + 2y j + (5 - 2) k. Derive the equation of streamline passing through a point (2, 1, 1).
A11)
u = -x, v = 2y and w = 5-z
The streamline, put the value of u, v and w
put the value of u, v and w

Consider,
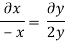
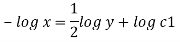
 Stream line passing through x=2, y=1, z= 1
Stream line passing through x=2, y=1, z= 1
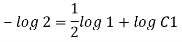
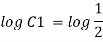
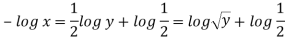

Now consider,
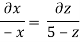
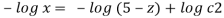
Stream line passing through x=2, z=1

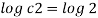

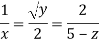
Q12) For the three-dimensional flow, the velocity distribution is given by u-x, v-4-2y, w z-2. What is the stream line equation passing through (1.-2, 3).
A12)
The streamline,
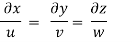
Put the value of u, v and w

Consider 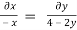
By integrating
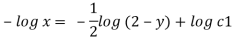

Stream line passing through x=1 y= =-2


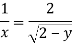
Now considering
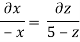

From equation

Q13) The velocity component in a steady flow are u=2kx, v= 2ky, w = - 4kz. What is the through the point (1, 0, 1).
A13)
The streamline,
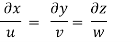
Put the value of u, v and w

Now considering
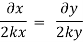
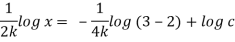
Stream line passing through x = 1, z=3,
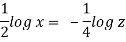
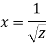
This is the equation of streamline passing through the point (1,0,1)
Q14) The velocity field for a two-dimensional flow is given by V= 4x³i-12x²yj. What is the equation of streamlines?
A14)
Velocity vector v = 4x'i-12x²yj
Velocity component u = 4x², v = -12x²y
Stream line
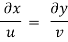
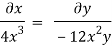
By integrating,
3 log x = -log y + log C
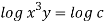
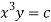
The curve defined by x³y = C₁, C₂, C, ...etc.
Q15) A stream function is given by  check if the flow is rotational and satisfies continuity equation
check if the flow is rotational and satisfies continuity equation
A15)
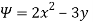
By definition
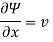
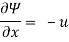
Velocity


1) Irritational flow
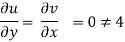
2) Continuity equation
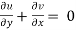
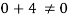
The flow is not continuous
Q16) Determine whether the following specified flows are rotational or otherwise. Determine the expression for the velocity potential in case of irrotational flow

A16)
For rotational flow


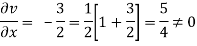
Flow is rotational.
Q17) the velocity potential function is given by an expression 
1) Find the velocity components in x and y direction
2) Show that  represents a possible case of flow
represents a possible case of flow
A17)
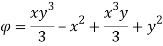
Case I) the partial derivation of 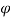 w.r.t x and y are
w.r.t x and y are

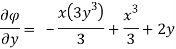
The velocity components u and v
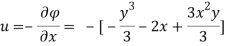
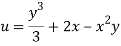
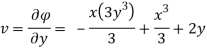
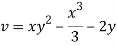
Case II) The value of  represents a possible case of flow if it satisfy the laplace equation
represents a possible case of flow if it satisfy the laplace equation



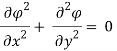
 Represents possible case of flow
Represents possible case of flow
Q18) The velocity potential function for a two dimensional flow is 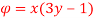 at a point P (4, 5) determine
at a point P (4, 5) determine
1) The velocity
A18)
Velocity potential
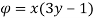
Differential w.r.t x
Hence
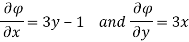
By definition of 
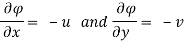
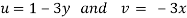
Velocity at P (4, 5)
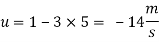
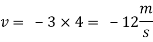
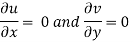

Flow is continuous
Q19) The velocity components for a two-dimensional incompressible flow is given by
1) u = 4 xy + y². v = 6 xy + 3x
2) 
Check whether the flow satisfies continuity?
A19)
(i) u = 4 xy + y², v = 6 xy + 3x
Differentiating

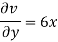
For two dimensional flow continuous equation is
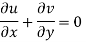

Velocity components does not satisfy the continuity equation
Ii) 
Differentiating


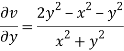

For two dimensional flow continuous equation is


Satisfy the continuous equation.
Q20) The velocity components for a three dimensional incompressible flow is given by: 
Check whether the flow satisfies continuity?
A20)
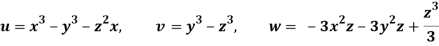
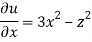
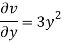
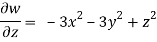
Foe three dimensional equation continuous equation is
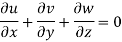
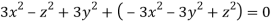
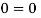
The flow is satisfy
Q21) The velocity field is given by  check whether the flow satisfies continuity?
check whether the flow satisfies continuity?
A21)
Velocity field

Velocity component

For three dimensional flow



Steady incompressible fluid flow is possible.
Unit - 3
Fluid Kinematics
Q1) Explain the terms a) Steady and unsteady flow b) Uniform and non uniform flow?
A1)
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically,  u/
u/  t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
Unsteady flow: It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically,  u/
u/  t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,
t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,  / t not = to 0
/ t not = to 0
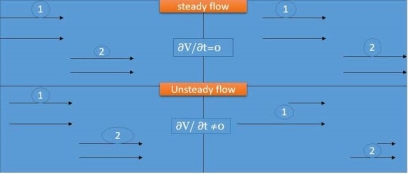
Fig no 1 Steady and unsteady flow
Uniform And Non-Uniform Flow
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically,  V /
V / 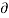 s = 0 when t = constant
s = 0 when t = constant
Non-uniform flow – It is that type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow through non-prismatic conduit.
Mathematically, 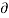 V/
V/ 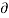 s not = to 0 when t = constant
s not = to 0 when t = constant

Fig no 2 Uniform and non-uniform flow
Q2) Explain the terms 1) laminar and turbulent flow 2) rotational and irrotational?
A2)
Laminar and Turbulent Flow
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig zag way.
E.g.: High velocity flows in conduit of large size.
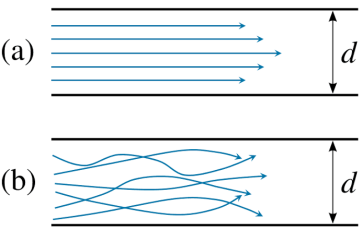
Fig no 3 Laminar and turbulent flow
Rotational and Irrotational Flow
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.

Fig no 4Rotational and irrotational flow
Q3) Explain the terms
a) compressible and incompressible flow
b) ideal and real fluid
A3)
Compressible and Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density () of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically, 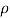 not = to constant
not = to constant
Incompressible Flow – It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically, 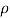 = constant
= constant
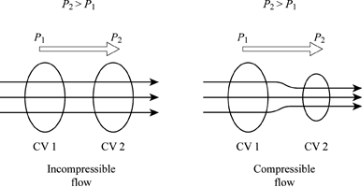
Fig no 5 Compressible and incompressible flow
Ideal and Real Fluid Flow
Ideal Fluid
- Ideal fluid is a fluid that is unmistakable and has no internal resistance to flow (zero viscosity). In addition the proper particles of fluid do not change according to their (thoughtless) center of gravity.
- Fine fluid can travel in a circular pattern, but the particles of each body do not think. Real fluids reflect all of these properties to some degree, but we will usually imitate the fluid as suitable for measuring the performance of a real liquid.
- In doing so, one must be very careful in applying the effects associated with a good liquid to a bad liquid.
- Liquids without viscosity, soil incompatibility, shear strength, and pressure are called fine liquids or complete liquids.
- The right liquid is all fluid that is considered and does not naturally contain those structures. However, a few liquids such as air and water have a very low viscosity, soil thickness, and high resistance to pressure are considered suitable water for all purposes as their thinking helps to simplify statistical analysis.
Real liquid
Liquids with viscosity, earth thickness, shear strength, and pressure are called real liquids. All liquids, in fact, are real liquids and provide a certain amount of resistance during liquid movements. The actual liquid is slightly separated as given below.
1. Newtonian fluid
The actual fluid, where the shear pressure is directly proportional to the shear strain level or velocity gradient is known as the Newtonian fluid as shown in Fig. 1.1. Examples of Newtonian liquids are water, air, paraffin, body oils, and glycerine, etc. These liquids follow Newton's law of viscosity.
2. Non-Newtonian fluid
The actual fluid where the shear pressure does not match the degree of shear hardness or velocity gradient is known as Non-Newtonian fluid. These liquids do not obey Newton's law of viscosity.
3. Ideal plastic or Bingham liquid
Liquids where shear pressure exceeds yield pressure and shear pressure is equal to the level of the shear machine or velocity gradient are more commonly known as the appropriate plastic fluid. Examples of plastic fluids suitable for the suspension of water with clay and fly ash.
4. Thiotropotropic fluid
These are non-Newtonian liquids with a non-linear relationship between stock pressure and angular fluctuations above a certain yield pressure. Examples of thixotropic liquids with crude oil, Bentonitic liquid drilling fluid and printer ink,etc.
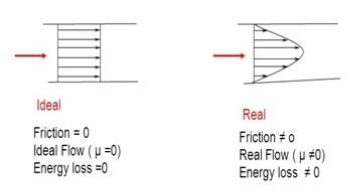
Fig no 6 Ideal and real fluid flow
Q4) Explain one, two and three dimensional flows?
A4)
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x, y) v = f2(x, y) & w = 0
Three-dimensional flow – It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x, y, z) v = f2(x, y, z) & w = f3(x, y, z)

Fig no 7 One two and three dimensional flow
Q5) Explain stream line?
A5)
- A streamline may be defined on as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
- An important concept in aerodynamics research touches on the concept of simple methods. The arrangement is the process followed by the weightless particles as they flow and flow.
- It is easier to visualize a streamline when moving through the body (unlike walking and flow).
- The integrated broadcast around the airfoil and near the cylinder. In both cases, we move with the object and the flow continues from left to right.
- As the streamline is followed by moving particles, at all points along the path the velocity is moving along the path. Since there is no standard object on the road, the magnitude cannot cross a straight line.
- The complexity contained between any two streams remains the same throughout the flow. We can use Bernoulli's figure to associate the pressure and speed associated with easy movement.
- Since there is no weight passing over the airfoil (or cylinder), the surface of the object is the easiest way.
- The planes fly through almost dry air, but we build and test them using air channels, where the model of the plane is stopped and the wind is blown through that model.
- The idea of keeping the airfoil fixed and that the airflow passing through the airfoil can be a little confusing. However you experience the same kind of thing every day
- If you stand on a corner and watch the car pass by, the atmosphere is a little quieter. As the car moves in the air there is aerodynamic force present.
- Now if you were in a car and you put your hand out the window, you could feel the aerodynamic force pushing your hand. It sounds like air is passing through your hand as fast as the car is moving.
- The power of the car is the same, whether you are standing in a corner or riding in a car. The same is true of airplanes. Whether the plane is moving in the air, or the wind is being pushed past the plane, the force is exactly the same.
- It is usually simple, inexpensive, and (in some cases) harmless to check planes in the air tunnel before attempting to fly.
- Streamlines are a family of curves that quickly turn into velocity vector flow. This indicates the direction in which the waterless object will move at any time.
- Stream line, In Liquid Mechanics, a method of synthetic particles suspended in a liquid and traveled with it.
- With a constant flow, the fluid flows but straight lines are arranged. Where the dispersion meets, the velocity of the liquid is very high; when they open, the liquid is quiet. See also laminar flow, turbulent flow.

Fig no 8 Stream line
Q6) Explain path lines?
A6)
- Path lines are the trajectories that are followed by the particles of each body. This can be thought of as "recording" the method of a liquid object in a certain flow of time. The direction of the path to be determined will be determined by the fluid flow of the liquid at each time point.
- Path Lines - A line path is a line followed by water particles as they travel over a period of time. Thus the line of motion indicates the direction of the velocity of the same particle of liquid at successive times. While the formulation shows the speed direction of many liquid particles at the same time.
- The liquid particle always moves the tangent to the grid, so, with a constant flow, the lines of direction and direction are the same.
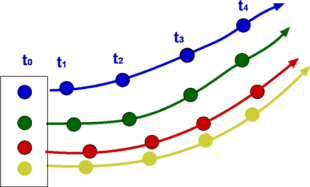
Fig no 9 Path lines
Q7) Explain streak line and stream tube?
A7)

Fig no 10 Streak line
Redirect is a line that positively connects velocity vectors over a period of time. In other words, the flow diagram presented in simple lines is similar to the abstract flow at the same time.
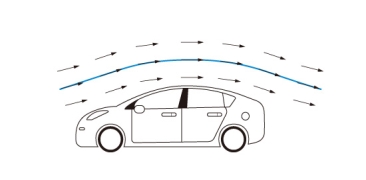
Fig no 11 Stream line example
A streak line is a curved line formed by a string of liquid particles that pass through a certain area. An example of a streak line is the smoke path from the chimney.

Fig no 12 Streak line example
Path line is the process followed by liquid particles. One example of a path line is a method described as a balloon floating in the air.
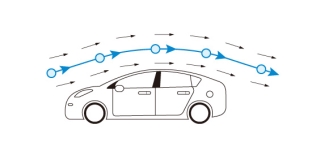
Fig no 13 Path line example
With constant flow, streamline, streak line, and path line are the same line. The constant flow of time is called the steady flow. On the other hand, the flow that varies with time is called transient flow. In the short-term flow, streamline, streak line, and path line are all different lines.
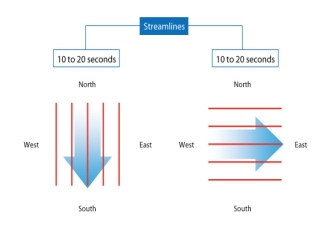
Fig no 14 Stream line direction
The streak line represents all the points that have passed in one place. In this case, where the streak line can be compared to represent the chimney from the chimney, the streak line goes south within the first ten seconds because all the smoke goes south. Then, when the wind blows eastward, all the smoke particles that start to move south (released by Time <10 seconds) begin to be placed eastward. New smoke particles (released Time> 10 seconds) are oriented eastward. After 20 seconds the streak line is in the right corner as shown on the right
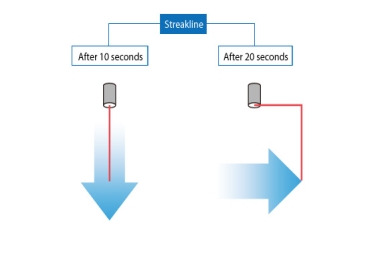
Fig no 15 Streak line direction
The path line goes south within the first 10 seconds as a streak line. The path line can be thought of as a process followed by a balloon floating in the air. When the wind shifts eastward, the balloon begins to move eastward. The path line goes east from where the direction of the wind is changed. As a result, after 20 seconds the path line bends at the right angle as shown on the right

Fig no 16 Path line direction
As can be seen in this chapter, the results of the temporal flow analysis will vary depending on the method used to detect the flow. Understanding these differences is important when looking at analysis and test results.
Q8) Explain stream function?
A8)
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow it is defined as Ψ = f(x, y) such that
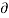 Ψ /
Ψ /  x= v
x= v
And 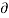 Ψ /
Ψ / 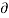 y = -u
y = -u
Q9) Explain velocity potential function?
A9)
The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y, z, t)
AndØ = f(x, y, z)
u = - 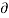 Ø /
Ø / 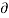 x
x
v = -  Ø /
Ø /  y
y
w = -  Ø /
Ø /  z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q10) Explain one, two and three dimensional continuity equation in Cartesian coordinate?
A10)
One dimensional
The continuity equation is based on the principle of conservation of mass. It states as follows
If no fluid is added or removed from the pipe in any length then the mass passing across different sections shall be same.
Consider two cross-sections of a pipe as shown in fig.
Let, A1&A2 = Areas of the pipe at section 1-1 & section 2-2 respectively.
V1 & V2 = Velocities of the fluid at section 1-1 & section 2-2 respectively.
1 &2 = Densities of the fluid at section 1-1 & section 2-2 respectively.
Total quantity of fluid passing through the section 1-1 = 1A1V1
Total quantity of fluid passing through the section 2-2 = 2A2V2
From the law of conservation of matter
1 A1 V1 = 2 A2 V2
In case of incompressible fluids 1 =2
A1 V1 = A2 V2
Three dimensional
Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.

Fig no 17 3d continuity equation
Let,  = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 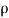 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=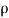 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
= 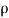 u dy dz +
u dy dz + 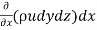
:. Mass accelerated per unit time, due to flow in x-direction
= 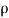 u dydz–[
u dydz–[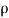 u+
u+ dx]dydz
dx]dydz
= - -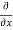 (
(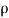 u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
=  (
( v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
= 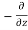 (
(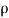 w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[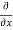 (
(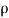 u) +
u) +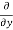 (
(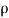 v) +
v) + 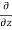 (
( w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
= 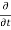 (
(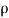 d x, d y d z) ………………….. 2
d x, d y d z) ………………….. 2
From Equation 1& 2
-[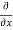 (
(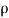 u) +
u) +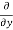 (
(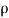 v) +
v) +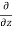 (
( w)] dx dy dz =
w)] dx dy dz =  (
(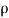 dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
 (
(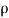 u) +
u) + 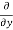 (
(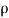 v) +
v) +  (
(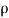 w) +
w) +  =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow (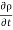 =0) incompressible fluids (
=0) incompressible fluids (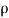 = constant) the equation reduces to
= constant) the equation reduces to
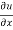 +
+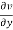 +
+ =0
=0
For two dimensional flow eq. Reduce to
Q11) The velocity of a flow is given by v = -x i + 2y j + (5 - 2) k. Derive the equation of streamline passing through a point (2, 1, 1).
A11)
u = -x, v = 2y and w = 5-z
The streamline,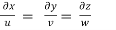 put the value of u, v and w
put the value of u, v and w

Consider,

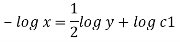
 Stream line passing through x=2, y=1, z= 1
Stream line passing through x=2, y=1, z= 1

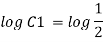
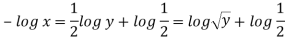

Now consider,
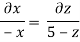
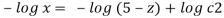
Stream line passing through x=2, z=1
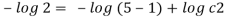
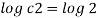

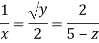
Q12) For the three-dimensional flow, the velocity distribution is given by u-x, v-4-2y, w z-2. What is the stream line equation passing through (1.-2, 3).
A12)
The streamline,

Put the value of u, v and w
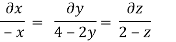
Consider 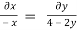
By integrating
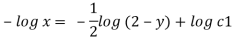
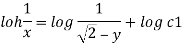
Stream line passing through x=1 y= =-2
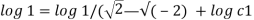


Now considering

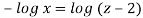
From equation

Q13) The velocity component in a steady flow are u=2kx, v= 2ky, w = - 4kz. What is the through the point (1, 0, 1).
A13)
The streamline,

Put the value of u, v and w
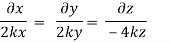
Now considering


Stream line passing through x = 1, z=3,
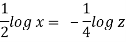
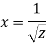
This is the equation of streamline passing through the point (1,0,1)
Q14) The velocity field for a two-dimensional flow is given by V= 4x³i-12x²yj. What is the equation of streamlines?
A14)
Velocity vector v = 4x'i-12x²yj
Velocity component u = 4x², v = -12x²y
Stream line
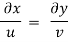

By integrating,
3 log x = -log y + log C
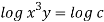
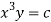
The curve defined by x³y = C₁, C₂, C, ...etc.
Q15) A stream function is given by 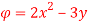 check if the flow is rotational and satisfies continuity equation
check if the flow is rotational and satisfies continuity equation
A15)

By definition

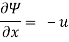
Velocity
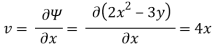

1) Irritational flow
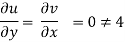
2) Continuity equation

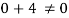
The flow is not continuous
Q16) Determine whether the following specified flows are rotational or otherwise. Determine the expression for the velocity potential in case of irrotational flow

A16)
For rotational flow
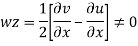
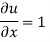
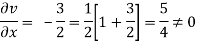
Flow is rotational.
Q17) the velocity potential function is given by an expression 
1) Find the velocity components in x and y direction
2) Show that  represents a possible case of flow
represents a possible case of flow
A17)

Case I) the partial derivation of 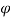 w.r.t x and y are
w.r.t x and y are
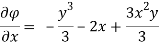
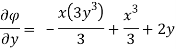
The velocity components u and v
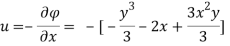
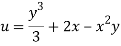

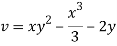
Case II) The value of  represents a possible case of flow if it satisfy the laplace equation
represents a possible case of flow if it satisfy the laplace equation

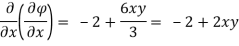


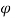 Represents possible case of flow
Represents possible case of flow
Q18) The velocity potential function for a two dimensional flow is 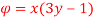 at a point P (4, 5) determine
at a point P (4, 5) determine
1) The velocity
A18)
Velocity potential
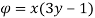
Differential w.r.t x
Hence

By definition of 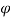
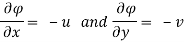
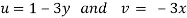
Velocity at P (4, 5)
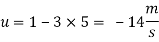
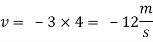


Flow is continuous
Q19) The velocity components for a two-dimensional incompressible flow is given by
1) u = 4 xy + y². v = 6 xy + 3x
2) 
Check whether the flow satisfies continuity?
A19)
(i) u = 4 xy + y², v = 6 xy + 3x
Differentiating
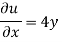

For two dimensional flow continuous equation is

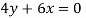
Velocity components does not satisfy the continuity equation
Ii) 
Differentiating

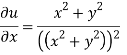
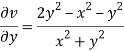
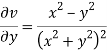
For two dimensional flow continuous equation is
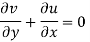
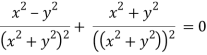
Satisfy the continuous equation.
Q20) The velocity components for a three dimensional incompressible flow is given by: 
Check whether the flow satisfies continuity?
A20)
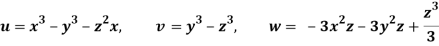


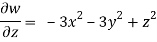
Foe three dimensional equation continuous equation is

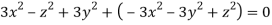
The flow is satisfy
Q21) The velocity field is given by 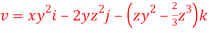 check whether the flow satisfies continuity?
check whether the flow satisfies continuity?
A21)
Velocity field
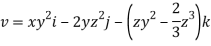
Velocity component

For three dimensional flow

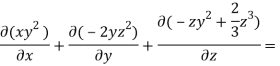
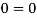
Steady incompressible fluid flow is possible.
Unit - 3
Fluid Kinematics
Q1) Explain the terms a) Steady and unsteady flow b) Uniform and non uniform flow?
A1)
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically,  u/
u/  t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
Unsteady flow: It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically, 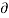 u/
u/  t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,
t not = to 0 , w/ t not = to 0 , p/ t not = to 0 , 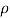 / t not = to 0
/ t not = to 0
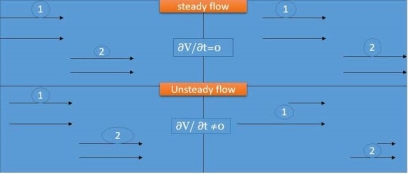
Fig no 1 Steady and unsteady flow
Uniform And Non-Uniform Flow
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically, 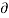 V /
V /  s = 0 when t = constant
s = 0 when t = constant
Non-uniform flow – It is that type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow through non-prismatic conduit.
Mathematically,  V/
V/  s not = to 0 when t = constant
s not = to 0 when t = constant
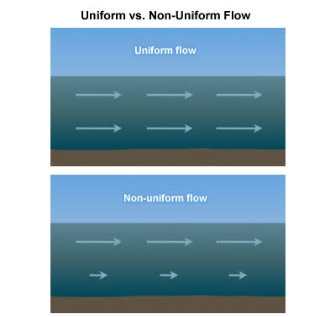
Fig no 2 Uniform and non-uniform flow
Q2) Explain the terms 1) laminar and turbulent flow 2) rotational and irrotational?
A2)
Laminar and Turbulent Flow
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig zag way.
E.g.: High velocity flows in conduit of large size.
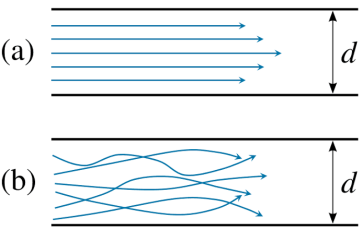
Fig no 3 Laminar and turbulent flow
Rotational and Irrotational Flow
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.
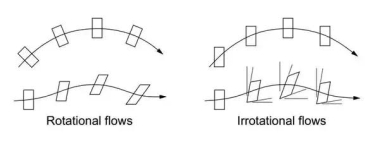
Fig no 4Rotational and irrotational flow
Q3) Explain the terms
a) compressible and incompressible flow
b) ideal and real fluid
A3)
Compressible and Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density () of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically, 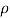 not = to constant
not = to constant
Incompressible Flow – It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically,  = constant
= constant
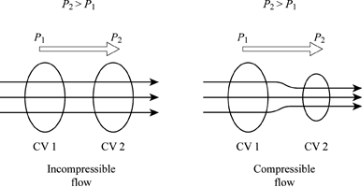
Fig no 5 Compressible and incompressible flow
Ideal and Real Fluid Flow
Ideal Fluid
- Ideal fluid is a fluid that is unmistakable and has no internal resistance to flow (zero viscosity). In addition the proper particles of fluid do not change according to their (thoughtless) center of gravity.
- Fine fluid can travel in a circular pattern, but the particles of each body do not think. Real fluids reflect all of these properties to some degree, but we will usually imitate the fluid as suitable for measuring the performance of a real liquid.
- In doing so, one must be very careful in applying the effects associated with a good liquid to a bad liquid.
- Liquids without viscosity, soil incompatibility, shear strength, and pressure are called fine liquids or complete liquids.
- The right liquid is all fluid that is considered and does not naturally contain those structures. However, a few liquids such as air and water have a very low viscosity, soil thickness, and high resistance to pressure are considered suitable water for all purposes as their thinking helps to simplify statistical analysis.
Real liquid
Liquids with viscosity, earth thickness, shear strength, and pressure are called real liquids. All liquids, in fact, are real liquids and provide a certain amount of resistance during liquid movements. The actual liquid is slightly separated as given below.
1. Newtonian fluid
The actual fluid, where the shear pressure is directly proportional to the shear strain level or velocity gradient is known as the Newtonian fluid as shown in Fig. 1.1. Examples of Newtonian liquids are water, air, paraffin, body oils, and glycerine, etc. These liquids follow Newton's law of viscosity.
2. Non-Newtonian fluid
The actual fluid where the shear pressure does not match the degree of shear hardness or velocity gradient is known as Non-Newtonian fluid. These liquids do not obey Newton's law of viscosity.
3. Ideal plastic or Bingham liquid
Liquids where shear pressure exceeds yield pressure and shear pressure is equal to the level of the shear machine or velocity gradient are more commonly known as the appropriate plastic fluid. Examples of plastic fluids suitable for the suspension of water with clay and fly ash.
4. Thiotropotropic fluid
These are non-Newtonian liquids with a non-linear relationship between stock pressure and angular fluctuations above a certain yield pressure. Examples of thixotropic liquids with crude oil, Bentonitic liquid drilling fluid and printer ink,etc.
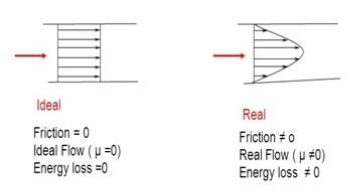
Fig no 6 Ideal and real fluid flow
Q4) Explain one, two and three dimensional flows?
A4)
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x, y) v = f2(x, y) & w = 0
Three-dimensional flow – It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x, y, z) v = f2(x, y, z) & w = f3(x, y, z)
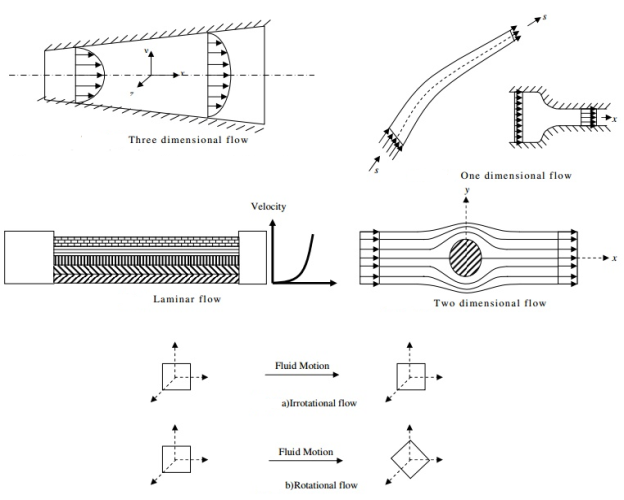
Fig no 7 One two and three dimensional flow
Q5) Explain stream line?
A5)
- A streamline may be defined on as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
- An important concept in aerodynamics research touches on the concept of simple methods. The arrangement is the process followed by the weightless particles as they flow and flow.
- It is easier to visualize a streamline when moving through the body (unlike walking and flow).
- The integrated broadcast around the airfoil and near the cylinder. In both cases, we move with the object and the flow continues from left to right.
- As the streamline is followed by moving particles, at all points along the path the velocity is moving along the path. Since there is no standard object on the road, the magnitude cannot cross a straight line.
- The complexity contained between any two streams remains the same throughout the flow. We can use Bernoulli's figure to associate the pressure and speed associated with easy movement.
- Since there is no weight passing over the airfoil (or cylinder), the surface of the object is the easiest way.
- The planes fly through almost dry air, but we build and test them using air channels, where the model of the plane is stopped and the wind is blown through that model.
- The idea of keeping the airfoil fixed and that the airflow passing through the airfoil can be a little confusing. However you experience the same kind of thing every day
- If you stand on a corner and watch the car pass by, the atmosphere is a little quieter. As the car moves in the air there is aerodynamic force present.
- Now if you were in a car and you put your hand out the window, you could feel the aerodynamic force pushing your hand. It sounds like air is passing through your hand as fast as the car is moving.
- The power of the car is the same, whether you are standing in a corner or riding in a car. The same is true of airplanes. Whether the plane is moving in the air, or the wind is being pushed past the plane, the force is exactly the same.
- It is usually simple, inexpensive, and (in some cases) harmless to check planes in the air tunnel before attempting to fly.
- Streamlines are a family of curves that quickly turn into velocity vector flow. This indicates the direction in which the waterless object will move at any time.
- Stream line, In Liquid Mechanics, a method of synthetic particles suspended in a liquid and traveled with it.
- With a constant flow, the fluid flows but straight lines are arranged. Where the dispersion meets, the velocity of the liquid is very high; when they open, the liquid is quiet. See also laminar flow, turbulent flow.

Fig no 8 Stream line
Q6) Explain path lines?
A6)
- Path lines are the trajectories that are followed by the particles of each body. This can be thought of as "recording" the method of a liquid object in a certain flow of time. The direction of the path to be determined will be determined by the fluid flow of the liquid at each time point.
- Path Lines - A line path is a line followed by water particles as they travel over a period of time. Thus the line of motion indicates the direction of the velocity of the same particle of liquid at successive times. While the formulation shows the speed direction of many liquid particles at the same time.
- The liquid particle always moves the tangent to the grid, so, with a constant flow, the lines of direction and direction are the same.

Fig no 9 Path lines
Q7) Explain streak line and stream tube?
A7)

Fig no 10 Streak line
Redirect is a line that positively connects velocity vectors over a period of time. In other words, the flow diagram presented in simple lines is similar to the abstract flow at the same time.

Fig no 11 Stream line example
A streak line is a curved line formed by a string of liquid particles that pass through a certain area. An example of a streak line is the smoke path from the chimney.
Fig no 12 Streak line example
Path line is the process followed by liquid particles. One example of a path line is a method described as a balloon floating in the air.
Fig no 13 Path line example
With constant flow, streamline, streak line, and path line are the same line. The constant flow of time is called the steady flow. On the other hand, the flow that varies with time is called transient flow. In the short-term flow, streamline, streak line, and path line are all different lines.

Fig no 14 Stream line direction
The streak line represents all the points that have passed in one place. In this case, where the streak line can be compared to represent the chimney from the chimney, the streak line goes south within the first ten seconds because all the smoke goes south. Then, when the wind blows eastward, all the smoke particles that start to move south (released by Time <10 seconds) begin to be placed eastward. New smoke particles (released Time> 10 seconds) are oriented eastward. After 20 seconds the streak line is in the right corner as shown on the right
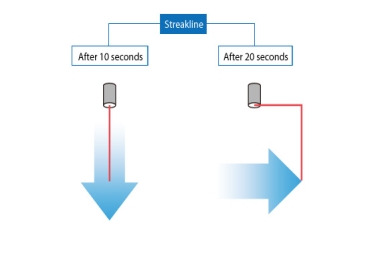
Fig no 15 Streak line direction
The path line goes south within the first 10 seconds as a streak line. The path line can be thought of as a process followed by a balloon floating in the air. When the wind shifts eastward, the balloon begins to move eastward. The path line goes east from where the direction of the wind is changed. As a result, after 20 seconds the path line bends at the right angle as shown on the right
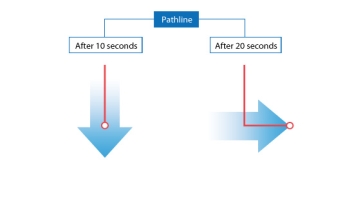
Fig no 16 Path line direction
As can be seen in this chapter, the results of the temporal flow analysis will vary depending on the method used to detect the flow. Understanding these differences is important when looking at analysis and test results.
Q8) Explain stream function?
A8)
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow it is defined as Ψ = f(x, y) such that
 Ψ /
Ψ / 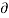 x= v
x= v
And  Ψ /
Ψ /  y = -u
y = -u
Q9) Explain velocity potential function?
A9)
The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y, z, t)
AndØ = f(x, y, z)
u = -  Ø /
Ø / 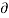 x
x
v = -  Ø /
Ø /  y
y
w = - 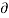 Ø /
Ø / 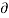 z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q10) Explain one, two and three dimensional continuity equation in Cartesian coordinate?
A10)
One dimensional
The continuity equation is based on the principle of conservation of mass. It states as follows
If no fluid is added or removed from the pipe in any length then the mass passing across different sections shall be same.
Consider two cross-sections of a pipe as shown in fig.
Let, A1&A2 = Areas of the pipe at section 1-1 & section 2-2 respectively.
V1 & V2 = Velocities of the fluid at section 1-1 & section 2-2 respectively.
1 &2 = Densities of the fluid at section 1-1 & section 2-2 respectively.
Total quantity of fluid passing through the section 1-1 = 1A1V1
Total quantity of fluid passing through the section 2-2 = 2A2V2
From the law of conservation of matter
1 A1 V1 = 2 A2 V2
In case of incompressible fluids 1 =2
A1 V1 = A2 V2
Three dimensional
Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.
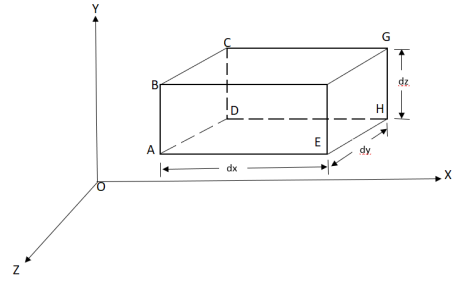
Fig no 17 3d continuity equation
Let, 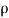 = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 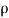 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=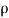 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
= 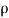 u dy dz +
u dy dz + 
:. Mass accelerated per unit time, due to flow in x-direction
= 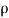 u dydz–[
u dydz–[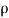 u+
u+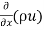 dx]dydz
dx]dydz
= - -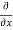 (
( u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
= 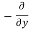 (
(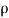 v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
= 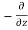 (
(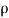 w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[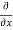 (
(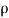 u) +
u) +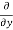 (
(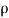 v) +
v) + 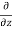 (
( w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
=  (
(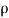 d x, d y d z) ………………….. 2
d x, d y d z) ………………….. 2
From Equation 1& 2
-[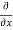 (
(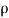 u) +
u) +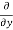 (
(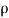 v) +
v) + (
(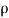 w)] dx dy dz =
w)] dx dy dz = 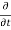 (
( dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
 (
( u) +
u) +  (
( v) +
v) + 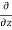 (
( w) +
w) + 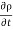 =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow (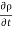 =0) incompressible fluids (
=0) incompressible fluids (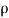 = constant) the equation reduces to
= constant) the equation reduces to
 +
+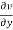 +
+ =0
=0
For two dimensional flow eq. Reduce to
Q11) The velocity of a flow is given by v = -x i + 2y j + (5 - 2) k. Derive the equation of streamline passing through a point (2, 1, 1).
A11)
u = -x, v = 2y and w = 5-z
The streamline,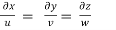 put the value of u, v and w
put the value of u, v and w
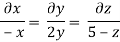
Consider,
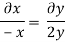

 Stream line passing through x=2, y=1, z= 1
Stream line passing through x=2, y=1, z= 1
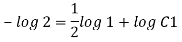

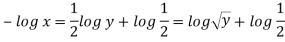

Now consider,

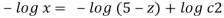
Stream line passing through x=2, z=1
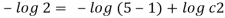
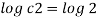
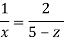
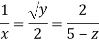
Q12) For the three-dimensional flow, the velocity distribution is given by u-x, v-4-2y, w z-2. What is the stream line equation passing through (1.-2, 3).
A12)
The streamline,

Put the value of u, v and w
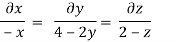
Consider 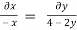
By integrating
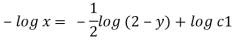
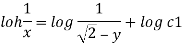
Stream line passing through x=1 y= =-2

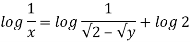

Now considering

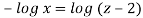
From equation

Q13) The velocity component in a steady flow are u=2kx, v= 2ky, w = - 4kz. What is the through the point (1, 0, 1).
A13)
The streamline,
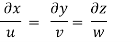
Put the value of u, v and w
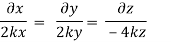
Now considering

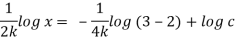
Stream line passing through x = 1, z=3,
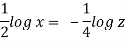
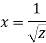
This is the equation of streamline passing through the point (1,0,1)
Q14) The velocity field for a two-dimensional flow is given by V= 4x³i-12x²yj. What is the equation of streamlines?
A14)
Velocity vector v = 4x'i-12x²yj
Velocity component u = 4x², v = -12x²y
Stream line


By integrating,
3 log x = -log y + log C
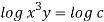

The curve defined by x³y = C₁, C₂, C, ...etc.
Q15) A stream function is given by 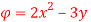 check if the flow is rotational and satisfies continuity equation
check if the flow is rotational and satisfies continuity equation
A15)

By definition

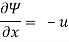
Velocity
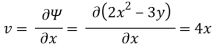

1) Irritational flow
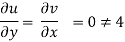
2) Continuity equation

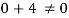
The flow is not continuous
Q16) Determine whether the following specified flows are rotational or otherwise. Determine the expression for the velocity potential in case of irrotational flow

A16)
For rotational flow

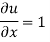
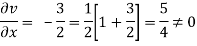
Flow is rotational.
Q17) the velocity potential function is given by an expression 
1) Find the velocity components in x and y direction
2) Show that  represents a possible case of flow
represents a possible case of flow
A17)

Case I) the partial derivation of 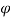 w.r.t x and y are
w.r.t x and y are
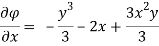

The velocity components u and v

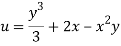
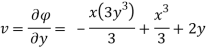

Case II) The value of 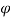 represents a possible case of flow if it satisfy the laplace equation
represents a possible case of flow if it satisfy the laplace equation
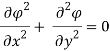
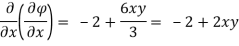
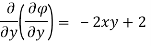
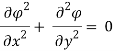
 Represents possible case of flow
Represents possible case of flow
Q18) The velocity potential function for a two dimensional flow is  at a point P (4, 5) determine
at a point P (4, 5) determine
1) The velocity
A18)
Velocity potential
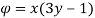
Differential w.r.t x
Hence
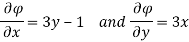
By definition of 
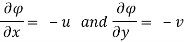
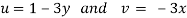
Velocity at P (4, 5)

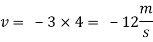
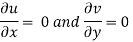
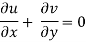
Flow is continuous
Q19) The velocity components for a two-dimensional incompressible flow is given by
1) u = 4 xy + y². v = 6 xy + 3x
2) 
Check whether the flow satisfies continuity?
A19)
(i) u = 4 xy + y², v = 6 xy + 3x
Differentiating

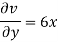
For two dimensional flow continuous equation is
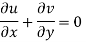

Velocity components does not satisfy the continuity equation
Ii) 
Differentiating
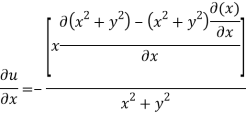
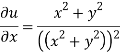

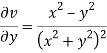
For two dimensional flow continuous equation is

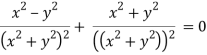
Satisfy the continuous equation.
Q20) The velocity components for a three dimensional incompressible flow is given by: 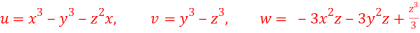
Check whether the flow satisfies continuity?
A20)
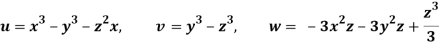
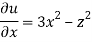


Foe three dimensional equation continuous equation is
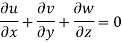

The flow is satisfy
Q21) The velocity field is given by 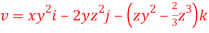 check whether the flow satisfies continuity?
check whether the flow satisfies continuity?
A21)
Velocity field
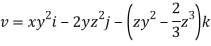
Velocity component

For three dimensional flow
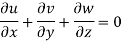

Steady incompressible fluid flow is possible.
Unit - 3
Unit - 3
Fluid Kinematics
Q1) Explain the terms a) Steady and unsteady flow b) Uniform and non uniform flow?
A1)
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow through a prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically,  u/
u/  t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
t = 0, w/ t = 0 , p/ t = 0 , rho / t = 0
Unsteady flow: It is that type of flow in which the velocity, pressure or density at a point change w.r.t. Time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically,  u/
u/ 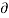 t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,
t not = to 0 , w/ t not = to 0 , p/ t not = to 0 ,  / t not = to 0
/ t not = to 0

Fig no 1 Steady and unsteady flow
Uniform And Non-Uniform Flow
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically, 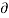 V /
V / 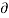 s = 0 when t = constant
s = 0 when t = constant
Non-uniform flow – It is that type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow through non-prismatic conduit.
Mathematically,  V/
V/  s not = to 0 when t = constant
s not = to 0 when t = constant

Fig no 2 Uniform and non-uniform flow
Q2) Explain the terms 1) laminar and turbulent flow 2) rotational and irrotational?
A2)
Laminar and Turbulent Flow
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig zag way.
E.g.: High velocity flows in conduit of large size.

Fig no 3 Laminar and turbulent flow
Rotational and Irrotational Flow
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.

Fig no 4Rotational and irrotational flow
Q3) Explain the terms
a) compressible and incompressible flow
b) ideal and real fluid
A3)
Compressible and Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density () of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically,  not = to constant
not = to constant
Incompressible Flow – It is that type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically, 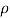 = constant
= constant

Fig no 5 Compressible and incompressible flow
Ideal and Real Fluid Flow
Ideal Fluid
- Ideal fluid is a fluid that is unmistakable and has no internal resistance to flow (zero viscosity). In addition the proper particles of fluid do not change according to their (thoughtless) center of gravity.
- Fine fluid can travel in a circular pattern, but the particles of each body do not think. Real fluids reflect all of these properties to some degree, but we will usually imitate the fluid as suitable for measuring the performance of a real liquid.
- In doing so, one must be very careful in applying the effects associated with a good liquid to a bad liquid.
- Liquids without viscosity, soil incompatibility, shear strength, and pressure are called fine liquids or complete liquids.
- The right liquid is all fluid that is considered and does not naturally contain those structures. However, a few liquids such as air and water have a very low viscosity, soil thickness, and high resistance to pressure are considered suitable water for all purposes as their thinking helps to simplify statistical analysis.
Real liquid
Liquids with viscosity, earth thickness, shear strength, and pressure are called real liquids. All liquids, in fact, are real liquids and provide a certain amount of resistance during liquid movements. The actual liquid is slightly separated as given below.
1. Newtonian fluid
The actual fluid, where the shear pressure is directly proportional to the shear strain level or velocity gradient is known as the Newtonian fluid as shown in Fig. 1.1. Examples of Newtonian liquids are water, air, paraffin, body oils, and glycerine, etc. These liquids follow Newton's law of viscosity.
2. Non-Newtonian fluid
The actual fluid where the shear pressure does not match the degree of shear hardness or velocity gradient is known as Non-Newtonian fluid. These liquids do not obey Newton's law of viscosity.
3. Ideal plastic or Bingham liquid
Liquids where shear pressure exceeds yield pressure and shear pressure is equal to the level of the shear machine or velocity gradient are more commonly known as the appropriate plastic fluid. Examples of plastic fluids suitable for the suspension of water with clay and fly ash.
4. Thiotropotropic fluid
These are non-Newtonian liquids with a non-linear relationship between stock pressure and angular fluctuations above a certain yield pressure. Examples of thixotropic liquids with crude oil, Bentonitic liquid drilling fluid and printer ink,etc.

Fig no 6 Ideal and real fluid flow
Q4) Explain one, two and three dimensional flows?
A4)
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x, y) v = f2(x, y) & w = 0
Three-dimensional flow – It is that type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x, y, z) v = f2(x, y, z) & w = f3(x, y, z)
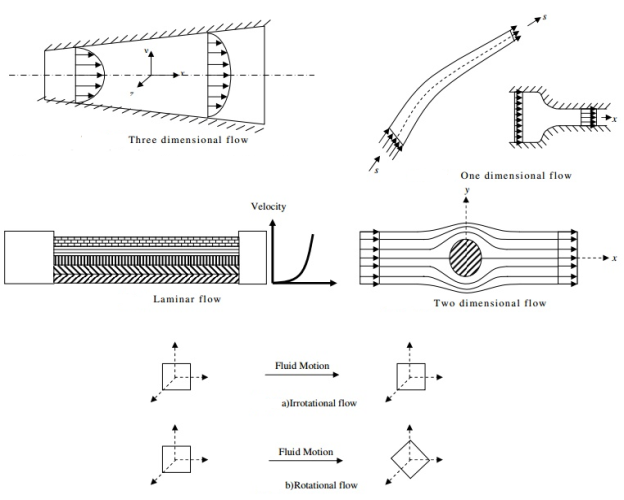
Fig no 7 One two and three dimensional flow
Q5) Explain stream line?
A5)
- A streamline may be defined on as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
- An important concept in aerodynamics research touches on the concept of simple methods. The arrangement is the process followed by the weightless particles as they flow and flow.
- It is easier to visualize a streamline when moving through the body (unlike walking and flow).
- The integrated broadcast around the airfoil and near the cylinder. In both cases, we move with the object and the flow continues from left to right.
- As the streamline is followed by moving particles, at all points along the path the velocity is moving along the path. Since there is no standard object on the road, the magnitude cannot cross a straight line.
- The complexity contained between any two streams remains the same throughout the flow. We can use Bernoulli's figure to associate the pressure and speed associated with easy movement.
- Since there is no weight passing over the airfoil (or cylinder), the surface of the object is the easiest way.
- The planes fly through almost dry air, but we build and test them using air channels, where the model of the plane is stopped and the wind is blown through that model.
- The idea of keeping the airfoil fixed and that the airflow passing through the airfoil can be a little confusing. However you experience the same kind of thing every day
- If you stand on a corner and watch the car pass by, the atmosphere is a little quieter. As the car moves in the air there is aerodynamic force present.
- Now if you were in a car and you put your hand out the window, you could feel the aerodynamic force pushing your hand. It sounds like air is passing through your hand as fast as the car is moving.
- The power of the car is the same, whether you are standing in a corner or riding in a car. The same is true of airplanes. Whether the plane is moving in the air, or the wind is being pushed past the plane, the force is exactly the same.
- It is usually simple, inexpensive, and (in some cases) harmless to check planes in the air tunnel before attempting to fly.
- Streamlines are a family of curves that quickly turn into velocity vector flow. This indicates the direction in which the waterless object will move at any time.
- Stream line, In Liquid Mechanics, a method of synthetic particles suspended in a liquid and traveled with it.
- With a constant flow, the fluid flows but straight lines are arranged. Where the dispersion meets, the velocity of the liquid is very high; when they open, the liquid is quiet. See also laminar flow, turbulent flow.
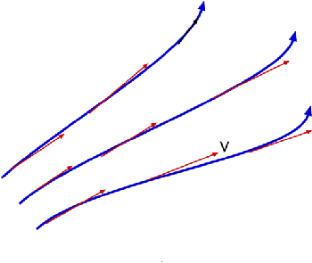
Fig no 8 Stream line
Q6) Explain path lines?
A6)
- Path lines are the trajectories that are followed by the particles of each body. This can be thought of as "recording" the method of a liquid object in a certain flow of time. The direction of the path to be determined will be determined by the fluid flow of the liquid at each time point.
- Path Lines - A line path is a line followed by water particles as they travel over a period of time. Thus the line of motion indicates the direction of the velocity of the same particle of liquid at successive times. While the formulation shows the speed direction of many liquid particles at the same time.
- The liquid particle always moves the tangent to the grid, so, with a constant flow, the lines of direction and direction are the same.

Fig no 9 Path lines
Q7) Explain streak line and stream tube?
A7)

Fig no 10 Streak line
Redirect is a line that positively connects velocity vectors over a period of time. In other words, the flow diagram presented in simple lines is similar to the abstract flow at the same time.

Fig no 11 Stream line example
A streak line is a curved line formed by a string of liquid particles that pass through a certain area. An example of a streak line is the smoke path from the chimney.
Fig no 12 Streak line example
Path line is the process followed by liquid particles. One example of a path line is a method described as a balloon floating in the air.
Fig no 13 Path line example
With constant flow, streamline, streak line, and path line are the same line. The constant flow of time is called the steady flow. On the other hand, the flow that varies with time is called transient flow. In the short-term flow, streamline, streak line, and path line are all different lines.

Fig no 14 Stream line direction
The streak line represents all the points that have passed in one place. In this case, where the streak line can be compared to represent the chimney from the chimney, the streak line goes south within the first ten seconds because all the smoke goes south. Then, when the wind blows eastward, all the smoke particles that start to move south (released by Time <10 seconds) begin to be placed eastward. New smoke particles (released Time> 10 seconds) are oriented eastward. After 20 seconds the streak line is in the right corner as shown on the right

Fig no 15 Streak line direction
The path line goes south within the first 10 seconds as a streak line. The path line can be thought of as a process followed by a balloon floating in the air. When the wind shifts eastward, the balloon begins to move eastward. The path line goes east from where the direction of the wind is changed. As a result, after 20 seconds the path line bends at the right angle as shown on the right

Fig no 16 Path line direction
As can be seen in this chapter, the results of the temporal flow analysis will vary depending on the method used to detect the flow. Understanding these differences is important when looking at analysis and test results.
Q8) Explain stream function?
A8)
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow it is defined as Ψ = f(x, y) such that
 Ψ /
Ψ /  x= v
x= v
And  Ψ /
Ψ /  y = -u
y = -u
Q9) Explain velocity potential function?
A9)
The velocity potential is defined as scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y, z, t)
AndØ = f(x, y, z)
u = -  Ø /
Ø /  x
x
v = -  Ø /
Ø /  y
y
w = -  Ø /
Ø /  z
z
Where u, v and w are the components of velocity in the x, y and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y and z. In other words, it indicates that the flow is always in the direction of decreasing Ø.
Q10) Explain one, two and three dimensional continuity equation in Cartesian coordinate?
A10)
One dimensional
The continuity equation is based on the principle of conservation of mass. It states as follows
If no fluid is added or removed from the pipe in any length then the mass passing across different sections shall be same.
Consider two cross-sections of a pipe as shown in fig.
Let, A1&A2 = Areas of the pipe at section 1-1 & section 2-2 respectively.
V1 & V2 = Velocities of the fluid at section 1-1 & section 2-2 respectively.
1 &2 = Densities of the fluid at section 1-1 & section 2-2 respectively.
Total quantity of fluid passing through the section 1-1 = 1A1V1
Total quantity of fluid passing through the section 2-2 = 2A2V2
From the law of conservation of matter
1 A1 V1 = 2 A2 V2
In case of incompressible fluids 1 =2
A1 V1 = A2 V2
Three dimensional
Considers a fluid element (control volume)- parallelepiped with sides dx, dy and dz as shown in fig.

Fig no 17 3d continuity equation
Let, 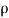 = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 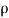 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=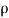 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
=  u dy dz +
u dy dz + 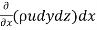
:. Mass accelerated per unit time, due to flow in x-direction
=  u dydz–[
u dydz–[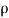 u+
u+ dx]dydz
dx]dydz
= - -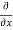 (
( u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
= 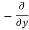 (
(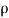 v) d x d y d z ……… (in Y- direction)
v) d x d y d z ……… (in Y- direction)
=  (
(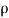 w) d x d y d z ……… (in Z- direction)
w) d x d y d z ……… (in Z- direction)
The, total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[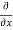 (
(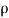 u) +
u) +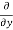 (
(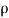 v) +
v) + 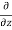 (
(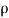 w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
= 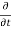 (
( d x, d y d z) ………………….. 2
d x, d y d z) ………………….. 2
From Equation 1& 2
-[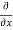 (
(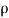 u) +
u) +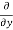 (
( v) +
v) + (
(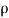 w)] dx dy dz =
w)] dx dy dz = 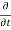 (
( dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
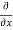 (
(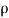 u) +
u) + 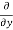 (
(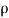 v) +
v) + 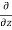 (
(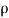 w) +
w) + 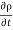 =0
=0
This eq. Is the general equation of continuity in three dimension and is applicable to any type of flow and for any fluid whether compressible as incompressible
For steady flow ( =0) incompressible fluids (
=0) incompressible fluids (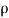 = constant) the equation reduces to
= constant) the equation reduces to
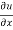 +
+ +
+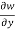 =0
=0
For two dimensional flow eq. Reduce to
Q11) The velocity of a flow is given by v = -x i + 2y j + (5 - 2) k. Derive the equation of streamline passing through a point (2, 1, 1).
A11)
u = -x, v = 2y and w = 5-z
The streamline,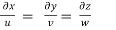 put the value of u, v and w
put the value of u, v and w

Consider,
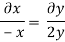

 Stream line passing through x=2, y=1, z= 1
Stream line passing through x=2, y=1, z= 1



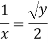
Now consider,
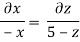

Stream line passing through x=2, z=1
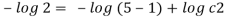

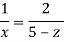

Q12) For the three-dimensional flow, the velocity distribution is given by u-x, v-4-2y, w z-2. What is the stream line equation passing through (1.-2, 3).
A12)
The streamline,
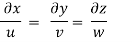
Put the value of u, v and w
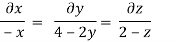
Consider 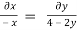
By integrating
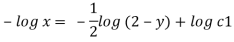

Stream line passing through x=1 y= =-2



Now considering


From equation

Q13) The velocity component in a steady flow are u=2kx, v= 2ky, w = - 4kz. What is the through the point (1, 0, 1).
A13)
The streamline,

Put the value of u, v and w

Now considering
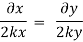

Stream line passing through x = 1, z=3,
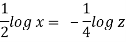

This is the equation of streamline passing through the point (1,0,1)
Q14) The velocity field for a two-dimensional flow is given by V= 4x³i-12x²yj. What is the equation of streamlines?
A14)
Velocity vector v = 4x'i-12x²yj
Velocity component u = 4x², v = -12x²y
Stream line

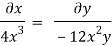
By integrating,
3 log x = -log y + log C

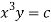
The curve defined by x³y = C₁, C₂, C, ...etc.
Q15) A stream function is given by 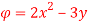 check if the flow is rotational and satisfies continuity equation
check if the flow is rotational and satisfies continuity equation
A15)

By definition
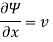
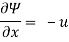
Velocity
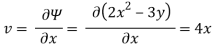
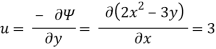
1) Irritational flow
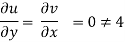
2) Continuity equation
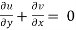
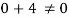
The flow is not continuous
Q16) Determine whether the following specified flows are rotational or otherwise. Determine the expression for the velocity potential in case of irrotational flow
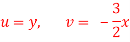
A16)
For rotational flow


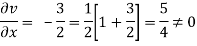
Flow is rotational.
Q17) the velocity potential function is given by an expression 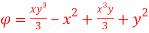
1) Find the velocity components in x and y direction
2) Show that  represents a possible case of flow
represents a possible case of flow
A17)
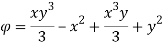
Case I) the partial derivation of 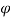 w.r.t x and y are
w.r.t x and y are

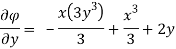
The velocity components u and v


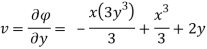
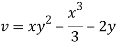
Case II) The value of 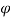 represents a possible case of flow if it satisfy the laplace equation
represents a possible case of flow if it satisfy the laplace equation
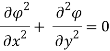

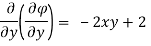
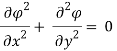
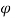 Represents possible case of flow
Represents possible case of flow
Q18) The velocity potential function for a two dimensional flow is  at a point P (4, 5) determine
at a point P (4, 5) determine
1) The velocity
A18)
Velocity potential
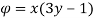
Differential w.r.t x
Hence

By definition of 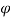


Velocity at P (4, 5)


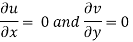
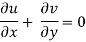
Flow is continuous
Q19) The velocity components for a two-dimensional incompressible flow is given by
1) u = 4 xy + y². v = 6 xy + 3x
2) 
Check whether the flow satisfies continuity?
A19)
(i) u = 4 xy + y², v = 6 xy + 3x
Differentiating


For two dimensional flow continuous equation is
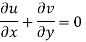

Velocity components does not satisfy the continuity equation
Ii) 
Differentiating

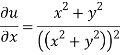
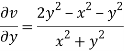
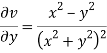
For two dimensional flow continuous equation is
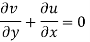

Satisfy the continuous equation.
Q20) The velocity components for a three dimensional incompressible flow is given by: 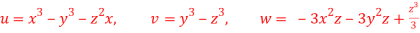
Check whether the flow satisfies continuity?
A20)



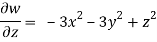
Foe three dimensional equation continuous equation is

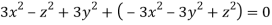
The flow is satisfy
Q21) The velocity field is given by 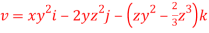 check whether the flow satisfies continuity?
check whether the flow satisfies continuity?
A21)
Velocity field
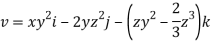
Velocity component

For three dimensional flow
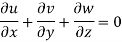

Steady incompressible fluid flow is possible.