Unit - 4
Fluid Dynamics
Q1) Explain surface and body forces.
A1)
The motion of the fluid element is influenced by the following forces
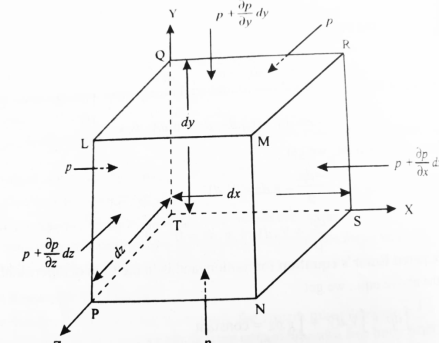
Fig no 1 Surface and body forces
1. Normal pressure force
The net pressure force in X direction = p dydz – ( p + 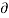 p/
p/ 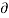 X dx) dy dz
X dx) dy dz
= - 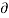 p/
p/  X dx dy dz
X dx dy dz
2. Gravity or body force
Let B be the body force per unit mass of fluid having components Bx, By&Bz
In x, y & z directions respectively.
Then, body force acting on the parallelepiped in the direction of X coordinate is = Bxdx dydz
3. Inertia force
The inertia force acting on the fluid mass, along the X coordinates is given by,
Mass x acceleration = 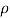 dx dydz du/ dy
dx dydz du/ dy
Q2) Explain Bernoulli’s equation?
A2)
Assumptions made in Bernoulli’s equation.
- The liquid is ideal & incompressible.
- The flow is steady & continuous.
- The flow is along stream line.
- The velocity is uniform over the section & equal to mean velocity.
- Only forces acting on the fluid are the gravity forces & pressure forces.
Bernoulli equation
- Bernoulli’s equation is derived based on the assumption that fluid is non viscous & therefore frictionless.
- Practically, all fluids are real & therefore viscous & there are always some losses in fluid flows.
- These losses have, therefore, to be taken into account in the application of Bernoulli’s equation which gets modified as follows
- P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2 + h L
Where h L= Loss of energy between section 1 & 2.
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
- Venturi meter
- Pitot- tube
- Sub-merged orifice
- Orifice meters
- Rotameter.
Limitation of Bernoulli’s equation
- Velocity of every liquid particle, across any section of pipe is not uniform.
- Bernoulli’s equation is not applicable for fluid with unsteady flow.
- If fluid flowing in a curved path, the energy due to centrifugal force should be taken into account.
Q3) Explain energy principle?
A3)
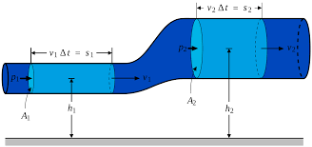
Fig no 2 Energy principal determination apparatus
- In fluid dynamics, Bernoulli's policy states that fluctuations in fluid flow occur simultaneously with a decrease in stationary pressure or a decrease in the available fluid strength.
- The program is named after Daniel Bernoulli who published it in his book Hydrodynamics in 1738. Although Bernoulli found that pressure decreased as the flow rate increased, it was Leonhard Euler who discovered Bernoulli's measurement in a normal way in 1752.
- The principle applies only to isentropic flow: where the effects of irreversible processes (such as disorder) and non-adiabatic processes (e.g. Heat radiation) are small and ignored.
- Bernoulli's system can be applied to a variety of fluid flows, leading to a variety of Bernoulli's calculations; there are different types of Bernoulli's figure for different types of flow.
- The simplest form of Bernoulli's equation is valid for unmistakable flow (e.g. High flow of air and gases running through a low March number). Advanced forms can be used for pressurized flow in high March numbers (see Bernoulli statistical availability).
- Bernoulli's policy can be based on the principle of energy conservation. This means that, with a constant flow, the sum of all the energies in the liquid along the simplest path is the same at all points in that flow path. This requires that the amount of kinetic energy, potential energy and internal energy remain constant.
- Thus an increase in the velocity of a liquid - i.e. an increase in its kinetic energy (strong pressure) - occurs simultaneously a decrease (total energy) of its energy (including constant pressure) and internal energy. When the liquid comes out of a pond, the amount of all kinds of energy is the same in all streamlines because in the pond the energy of each unit (total pressure and gravity ρ g h) is the same everywhere.
- Bernoulli's goal can also be found directly in Isaac Newton's Second Movement of Movement. If a small amount of fluid flows horizontally from a high pressure area to a low pressure region, then there is more pressure behind than before. This gives the net power by volume, speeding it up with ease.
- Fluid particles are subject to pressure and weight only If the fluid flows horizontally and partially along a certain flow path, where the speed increases it may be due to the fluid in that phase moving from the high-pressure region to the low-pressure region; and if its speed decreases, it may be because it has moved from a low-pressure region to a high-pressure region.
- As a result, inside the liquid flowing upwards, the greatest velocity occurs when the pressure is too low, and the lowest velocity occurs when the pressure is too high.
Q4) What is mean by venturimeter?
A4) A venturi meter is a device used for measuring the rate of a flow of a fluid flowing through a pipe it consists of three parts:
- A short converging part,
- Throat, and
- Diverging Part
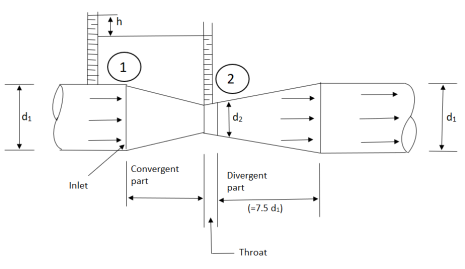
Fig no 3 Venturimeter
It is based on the principle of Bernoulli’s equation.
Expression for Rate of flow through venturimeter
Consider a venturimeter fitted in a horizontal pipe through which a fluid is following as shown in fig.
Let d 1 = diameter at inlet or at section (1)
P 1= pressure at section (1)
V 1= velocity of fluid at section (1)
A 1= area of section (1) =π/4. D2
And d 2, P 2, V 2, a 2 are corresponding values at section (2)
Applying Bernoulli’s equation at section (1) and (2) we get
P1/ 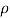 g+V12 /2g+Z1=P2 /
g+V12 /2g+Z1=P2 / 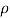 g+V22 /2g+Z2
g+V22 /2g+Z2
As pipe is horizontal hence z 1 = z2
P1/  g+V12 /2g =P2 /
g+V12 /2g =P2 /  g+V22 /2g
g+V22 /2g
(P1 – p 2 )/ 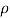 g = V22 /2g - V12 /2g
g = V22 /2g - V12 /2g
But (P1 – p 2 )/ 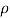 g is the difference of pressure heads at section land 2 and it is equal to h
g is the difference of pressure heads at section land 2 and it is equal to h
∴(P1 – p 2 )/ 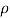 g =h
g =h
Substituting this value of (P1 – p 2 )/  g in the above eqn we get.
g in the above eqn we get.
∴h = V22 /2g - V12 /2g………….. (1)
Now applying continuity equation at section 1 and
a 1 v 1 = a 2 v 2
∴ v 1 = a 2 v 2 / a 1
Substituting this value of v 1 in equation 1
h= V22 /2g – (a 2 v 2 / a 1) 2 / 2 g
h= V22 /2g [ (a 12 –a 22 )/ a 12 ]
V22 = (2 g h a 12 )/ (a 12 –a 22)
Q = a2 v 2
= [a 1 a 2 / 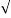 ( a 12 –a 22 ) ] *
( a 12 –a 22 ) ] * 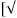 ( 2 g h ) ]
( 2 g h ) ]
Above equation gives the discharge under ideal conditions and is called, Theoretical discharge Actual discharge will be less than theoretical discharge.
Q act = C d * [a 1 a 2 / 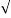 ( a 12 –a 22 )] *
( a 12 –a 22 )] *  ( 2 g h ) ]
( 2 g h ) ]
Where C d = co-efficient of venturimeter and its value is less than 1.
Q5) Explain orifice meter and pitot tube?
A5)
ORIFICE METER
- It is a device used for measuring the rate of flow of a fluid through a pipe.
- It is a cheaper device compared to venturimeter
- It also works on the same principle as that of venturimeters.
- It consists of a flat circle plate which has a circular shapes edged holed called orifice, which is concentric with the pipe.
- The orifice diameter is kept generally 0.5 times the diameters of the pipe, though it may vary from 0.4 to 0.8 times the pipe diameters.
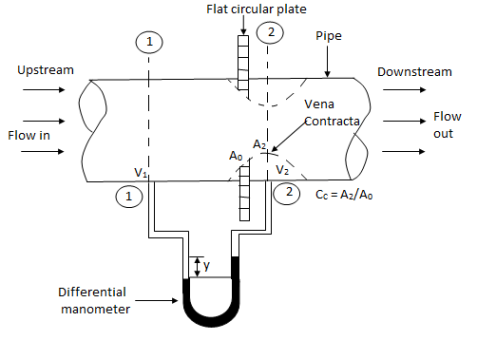
Fig no 4 Orifice meter
A differential manometers is connect at section
(1) Which is at a distance of about 1.5 to 2.0 times the pipe diameters upstream from the orifice plate, and at section
(2) Which is at a distance of about half the diameters of the orifice on the downstream side from the orifice plate.
PITOT TUBES
- It is a device used for measuring the velocity of flow at any point in a pipe or a channel.
- It is based on the principle, that if the velocity of flow at a point becomes zero, the pressure there is increased due to the conservation of the kinetic energy into pressure energy.
- In its simplest form, the pitot- tube consists of a glass tube, bent at right angles as shown in fig.
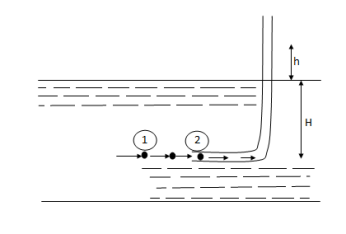
Fig no 5 Pitot tubes
- The Lower end, which is bent though 90° is directed in the upstream direction as shown in fig.
- The liquid rises up in the tube due to the conservation of kinetic energy into pressure energy.
- The velocity is determined by measuring the rise of liquid in the tube.
P1= intensity of pressure at point (1)
V1 = velocity of flow at (1)
P2 = pressure at point (2)
V2 = velocity at point (2), which is zero
H = depth of tube in the liquid.
h = rise of liquid in the tube above the fire Surface
Applying Bernoulli’s equation at points (1) and (2)
We get
P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
But Z 1 = Z2as points (1) and (2) are an the same line and V2 =0
P1 /g= pressure Lead at (1) = H
P2 /g = pressure head at (2) = (h +H)
Substituting these values, we get
H+ V1 2 / 2 g = (h+H)
:. h= V1 2 / 2 g or v 1 =  (2 g h )
(2 g h )
This is theoretical velocity.
Actual velocity is gives by (V 1) = Cv (2 g h)
(2 g h)
Where C v= Co-efficient of pitot-tube
:. Velocity at any point V= Cv (2 g h)
(2 g h)
Q6) Explain momentum principle?
A6)
- It is based on law of conservation of momentum or on the momentum principle, which states that the net force acting on a fluid mass is equal to the change in momentum of flow per unit time in that direction.
- The force acting on a fluid mass m is given by the Newtons second law of motion.
F = m x a
Where a is the acceleration acting in the same direction as force F
But a = d v /d t
F = m x d v /d t
= d (m v) /d t, m is constant can be taken inside the differential
Above equation is known as the momentum principle.
It can be written as F d t = d (m v)
Which is known as the momentum equation & states that the impulse of force F acting on a fluid of mass m in a short interval of time dt is equal to the change of momentum d(m v) in the direction of force.
Momentum Correction Factor ( )
)
Momentum correction factor is defined as the ratio of momentum of the flow per second based on actual velocity to the momentum of the flow per second based on average velocity across a section. It is denoted by.
 = Momentum per second based on actual velocity/ Momentum per second based on average velocity
= Momentum per second based on actual velocity/ Momentum per second based on average velocity
 = 1 for uniform flow
= 1 for uniform flow
 = 1.01 to 1.07 for turbulent flow in pipes
= 1.01 to 1.07 for turbulent flow in pipes
 = 4/3 = 1.33 for laminar flow in pipes.
= 4/3 = 1.33 for laminar flow in pipes.
Q7) Explain forces exerted by fluid flow on pipe bend
A7) The energy produced by the liquid flowing in the pipe - bending
- In order to prevent the reflection of the resulting force applied by the liquid flowing into the bend pipe, we will use the basic concept of impulse pressure equation. Before proceeding, it is very important to find and learn the concept of equation intensity.
- Let us assume that the liquid flows through a curved pipe as shown here in the following figure. We are looking at two phases here namely phase 1-1 and section 2-2.

Fig no 6 The energy produced by liquid flowing in bending pipe
V1 = Speed of liquid flowing in phase 1
P1 = Pressure fluid pressure in phase 1
A1 = Section 1 location
V2 = Speed of liquid flowing in phase 2
P2 = Pressure fluid pressure in phase 2
A2 = Section 2 location
FX = The energy released by the liquid flowing in the rotation of the pipe in the X-direction.
FY = A police force that causes fluid to flow in a pipe turn in a Y-direction.
- As we thought above that Fx and FY are forces, they are produced fluid flowing in the pipe turns X and Y respectively.
- In view of Newton's third law of motion, the forces charged by the bending of the pipe in the flowing liquid would be - FX and FY in the direction of X and Y respectively.
- There will be more energy and it will work in the flowing liquid. P1A1 and P2A2 are the compressive forces acting on the liquid in phase 1 and phase 2 respectively.
- We will now remember the pressure number and will have the next X direction figure.
Net power applied to the liquid in the X-direction = Level of pressure change in the X-direction
P1A1 - P2A2 Cos θ - FX = Mass at each unit time x velocity change
P1A1 - P2A2 Cos θ - FX = ρ Q (Final speed in X direction - First velocity in X-direction)
P1A1 - P2A2 Cos θ - FX = ρ Q (V2 Cos θ - V1)
FX = ρ Q (V1 - V2 Cos +) + P1A1 - P2A2 Cos θ
- Similarly, we will remember the pressure figure and will have the following Y direction number.
Net power applies to liquids in Y-direction = Level of pressure change in Y-direction
- P2A2 θ sin - FY = Mass for each unit time x speed change
- P2A2 Sin θ - FY = ρ Q (Final velocity in Y direction - First velocity in Y direction)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
FY = ρ Q (-V2 Sin θ) - P2A2 Sin θ
Let us determine the following (FR) forces acting on the bending of the pipe and the bending of the angle with the resulting force (FR) with the horizontal direction.
F = √ ( Fx2 + Fy2 )
Tan θ = Fy/ Fx
Q8) Explain vortex flow in terms of free and forced?
A8)
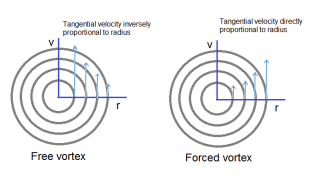
Fig no 7 Vortex flow
Vortex flow: the circulating weight of a liquid or a liquid flow in a curved path.
Free vortex flow
- No external torque or power required. Liquid circulation under certain energies previously given to them. In free vortex machines, the overall energy flow remains constant. There is no power connection between the external source and the flow and any power dissipation of the equipment in motion.
- Liquid circulation is due to the preservation of angular pressure.
- Velocity equals radius.
For a Free vortex flow
v r = constant or v = c/ r
Free vortex flow - Velocity equation
At the center (r = 0) of the velocity rotation approaches infinitely, that point is called a single point.
- Free flow of vortex is irrational; therefore, it is also known as irrotational vortex.
- In free vortex flow, Bernoulli's equation can be used.
Examples include a whirlpool in a river, water flowing from a bathtub or sink, flowing into the space of a centrifugal pump and flowing around a circular bend of a pipe.
Forced vortex flow
- To maintain the forced flow of the vortex, it required a continuous supply of power or external torque.
- All liquid particles circulate with an infinite angular velocity ω as a solid body. Therefore, the flow of a forced vortex is called a solid body rotation.
- Tangential velocity is directly proportional to the radius.
v = r ω
ω = Angular speed.
r = Radius of particle fluid from the axis of rotation.
- The profile of the vortex flow area is illustrated.

Fig no 8 Forced vortex Verified vortex - Height rate
- At the forced vortex force each unit weight increases with increasing radius.
- The forced vortex is not unthinkable; instead the rotational flow with a constant vorticity 2ω.
Examples of forced vortex flow rotate a vessel containing a liquid with constant angular velocity, flowing within a centrifugal pump.
Q9) Explain Euler equation.
A9)
EQUATION OF MOTION - EULERS EQUATION
Consider steady flow of an ideal fluid along the shown tube.
Separate out a small element of fluid of cross- sectional area d A and length d s from stream tube as a free body from the during fluid.
Fig. Shows such a small elements LM of fluid of cross Section area d A and length d s.
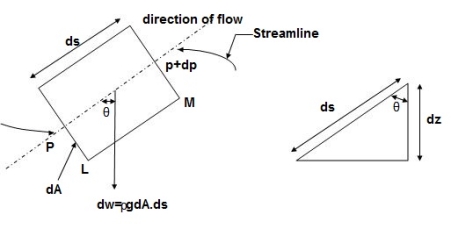
Fig no 9 Equation of motion Diagram
Let, p= Pressure of an the elements at L
p + dp = Pressure on the element at M,and
V=Velocity of the fluid element.
The external forces tending to accelerate the fluid elements in the direction of streamline are as follows:
Net pressure force in the direction of flows is, p.dA – (p+dp)dA = -dp. DA
Component of the weight of the fluid elements in the direction of flows is
= - rho.g.dA.ds.cos
=- rho.g.dA.ds (dz/ds) (:. Cos = dz/ds)
= - rho .g.dA.dz
Mass flow of the fluid element =  .dA.ds
.dA.ds
The Acceleration of the fluid element
a = dv/dt = dv/ds * ds/dt = v. Dv/ds
Now , according to Newton’s second law of motion, force= mass x acceleration.
:. – dp. DA.-  .g.dA.dz=
.g.dA.dz=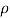 .dA.ds x v.dv/ds
.dA.ds x v.dv/ds
Dividing both sides by rho .dA, we get
-dp/-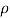 . g.dz= v.dv
. g.dz= v.dv
Or dp/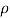 + v.dv + g.dz=0
+ v.dv + g.dz=0
This is the required Euler’s equation for motion.
Integrating the Euler’s equation of motion we get
1/ ∫ d p +∫v. d v+ ∫g.dz=constant
∫ d p +∫v. d v+ ∫g.dz=constant
p/ ρ + v2 /2 + g z = constant
Dividing by g, we get
p/ 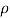 g + v 2/2g + z = constant
g + v 2/2g + z = constant
p /w + v 2/2g + z = constant
Or in other words, P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
Which proves Bernoulli’s equation
Q10) What are the applications of Bernoulli’s equation?
A10)
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
6. Venturi meter
7. Pitot- tube
8. Sub-merged orifice
9. Orifice meters
10. Rotameter.
Q11) Water is flowing through a tapered pipe having diameters 300 mm and 150 mm at section 1 and 2 respectively. The discharge through the pipe is 40 litre/sec. Section 1 is 10 m above datum and section 2 is 6 m above datum. Find the intensity of pressure at section 2 if that at section 1 is
A11)
Given
Q = 40 litre/sec=0.04 m²/sec
Z₁ = 10m
Z₂ =6m
d₁ =300mm=0.3 m
d2 = 150 mm-0.15m
P1 = 400 kN/m²
To find: P:
Area
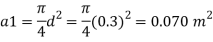
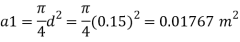
By continuity equation,
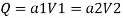
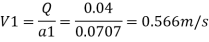

By Bernoulli's equation
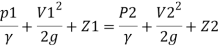
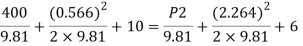
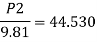
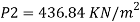
The intensity of pressure of section 2 is 436.84 kN/m²
Q12) A pipe has a length of 200 m with a slope of 1 in 100. The diameter of pipes changes from 1.0 m at higher end to 0.5 m at lower end. Find the pressure at the lower end if the discharge flowing through pipe is 5.00 m/min and pressure at higher end is 45 kN/m².
A12)
Given:
L = 200 m,
P₁ =45 kN/m²
Slope = 1 in 100. z₁ = 0,
d₂ -0.5 m
Q = 5 m/min =5/60 = 0.0833 m/sec
To find: P₂
Slope of pipe is 1 in 100
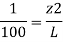
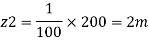
(Since it is below z₁
i.e., datum, it is considered negative) = -2m
By continuity equation

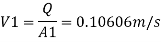
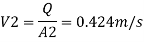
By Bernoulli's equation
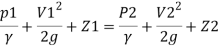
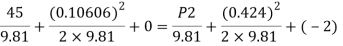
Multiplying by 9.81
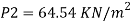
Q13) In a vertical pipe conveying oil of specific gravity 0.8, two pressure gauges has been installed at A and B, where diameters are 16 cm and 8 cm respectively. A is 2 m above B. The pressure gauge readings have shown that pressure at B is greater than at A by 0.981 N/cm². Neglecting all losses calculate flow rate
A13)
Given:
Specific gravity S=0.8
Diameter at A, dA = 16 cm = 0.16 m
Diameter at B, dB = 8 cm=0.08 m ZA = 2 m, ZB=0
PB=PA+0/981 N/cm^2
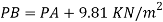
Pressure difference PB-PA= 9.81KN/m^2
Area

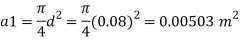
By continuity equation,
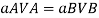

By Bernoulli's equation

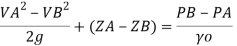
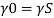
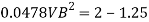
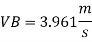
Discharge

Q14) The top and bottom diameters of a 2m long vertical tapering pipe are 100 mm and 50 mm respectively. Water flows down the pipe at 30 liters por second. Find the pressure difference between the two ends of the pipe.
A14)
Given:
Q = 30 Lps = 0.03 m³/sec,
d1 = 0.05 m. d₂ = 0.1 m, z₁ = 0, z₂ = 2 m
Area
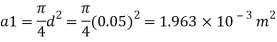
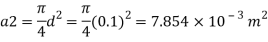
By continuity equation,
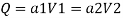

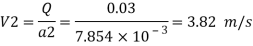
By Bernoulli's equation
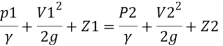
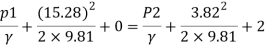

:. The pressure difference between two ends of pipe is 10.415x 10³ Pa
Q15) Obtain an expression for pressure drop from inlet to throat in a horizontally mounted venturimeter in terms of rate of volume flow (0) and inlet diameter (D) for inlet throat diameter ratio is equal to 2.
A15)
d1= Inlet diameter = D
Inlet diameter /Throat diameter= 2
d2 = Throat diameter = D/2
By continuity equation

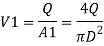
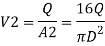
By Bernoulli's equation


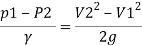
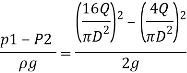

Q16) A venturimeter has an area ratio 9.1, the large diameter being 30 cm. During the flow, the recorded pressure head in the large section is 7.75 m and that at the throat is 5.5 m. Find the discharge through the meter if C,0.98.
A16)
Given:
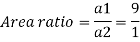
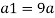
Discharge of inlet
d1 = 0.3 m
Pressure at inlet 
Pressure head at throat 
Coefficient of discharge Cd, = 0.98
Area
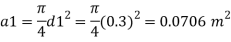

Pressure head
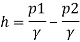
Discharge
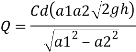

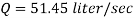
Q17) A venturimeter is to be fitted to a pipe of area 0.01787 m² A maximum discharge of 5 m/min under a pressure of 4.5 m flows through the pipe. Find the diameter at the throat for no negative pressure at the throat.
A17)
Given:
a1 = 0.01767 m². Q = 5 m²/min=0.08333 m/sec
Pressure head = 4.5 m
To find: Diameter of throat i.e. d
Considering the venturimeter to be horizontal
Applying Bernoulli’s equation at section (1) and (2)
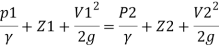
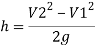
But Z1=Z2
And as there is no negative pressure at throat considering
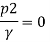
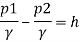
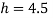
Discharge Q
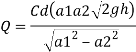
(Assuming C=0.98)

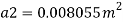
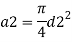

Q18) A venturimeter is fitted to 25 cm diameter pipe. A discharge 10 m³/s flowing through venturimeter, develops a venturi head of 3.5 m of water. Find the minimum diameter of throat so that there is no negative head developed.
A18)
Given:
D = 25 cm = 0.25 m, Q = 10 m³/s,
To find:
d for no negative head developed.
a₁ = area of inlet = 
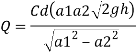
Cd=1

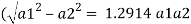
Squaring both side
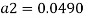
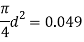
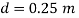
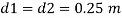
Q19) A jet of water 50 mm diameter is discharging under a constant head of 70 metres. Find the force exerted by the jet on a fixed plate. Take coefficient velocity as 0.9.
A19)
Given:
d = 50 mm = 0.05 m; H = 70 m
And Cd= 0.9
We know that cross-section area of the jet,
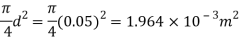
Velocity of the jet,

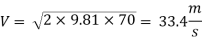
Force exerted by the jet on the fixed plate,
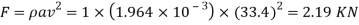
Q20) A horizontal jet of water is issuing under an effective head of 25 m calculate the diameter of the jet if the force exerted by the jet on a vertical plate is 2.22 KN. Take Cv =1
A20)
Given:
H=25 m; F= 2.22 kN and Cv=1
Let d = Diameter of the jet in meters
We know that cross-section area of the jet,

Velocity of the jet,
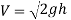
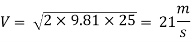
Force exerted by the jet on the fixed plate,
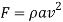
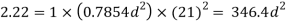
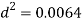
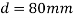
Q21) A jet of water of 100 mm diameter, moving with a velocity of 20 m/s striker a stationary plate Find the force on the plate in the direction of the jet, when
(a) The plate is normal to the jet, and
(b) The angle between the jet and plate is 45°.
A21)
Given:
d = 100 mm= 0.1 ni; V=20 m/s and 8 = 45°.
(a) Force on the plate when it is normal to the jet
We known that cross-section area of the jet,

And force on the plate when it is normal to the jet,
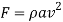
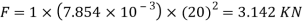
(b) Force on the plate when the angle between the jet and plate is 45%
We also known that force on the plate when the angel between the jet the and plate is 45°,
F =  av² = 1x sin²45
av² = 1x sin²45
= 3.142 sin² 45° = 3.142 x (0.707)² = 1.57 kN
Q22) A submarine is cruising at a depth of 20 m in ocean water (density 1020 kg/m). If the forward speed of the submarine is 10 m/s, what readings would be given by pitot and static pressure probes? Assume static probe is located to register free stream static pressure.
A22)
Given:
Speed V = 10 m/s

:. Velocity of submarine
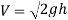
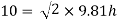
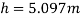
Dynamic pressure

At 20 m depth, static pressure
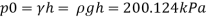
Pitot Probe pressure = Static pressure + Dynamic pressure
= 200.124 +51
= 251.124 kPa
Q23) A pitot tube records of 7.85 kN/m² as the stagnation pressure when it is head at the centre of pipe of 250 mm diameter conveying water. The static pressure in the pipe is 40 mm of mercury (gauge-vacuum). Calculate the discharge through the pipe assuming that the mean velocity of flow is 0.8 times the maximum velocity. Take C, = 0.98.
A23)
Given:
Stagnation pressure p₁= 7.85 kN/m²
Pressure at center of pipe = 40 mm of mercury
P2 = -0.04×13.6 x 9.81-5.336 kN/m
Mean velocity = 0.8 x maximum velocity
Pressure head
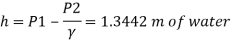
Maximum velocity
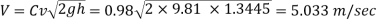
Mean velocity (V)=0.8 x Maximum velocity = 0.8 x 5.033=4.0264 m/sec
Discharge Q

Discharge through the pipe 197.65 Lps.
Q24) A pitot static tube is used to measure velocity of an aeroplane. U-tube differential manometer gives deflection of 100 mm of water. If specific weight of air is 12 N/m and coefficient of pitot tube is 0.98. Determine speed of aeroplane. Neglect compressibility effects.
A24)
Given:
C = 0.98. Ya = 12 N/m².
x = 100 mm of water = 0.1m
To find: Speed of aero plane V = ?
Specific gravity of air
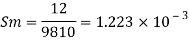
Specific gravity of water
Sw = 1
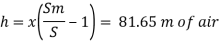
Speed of aircraft
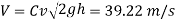
The speed of aeroplane is 39.28 m/s.
Q25) An Orifice of diameter 12 cm is inserted in a pipe of 24 cm diameter. The end pressure gauge fitted upstream and 2 downstream of the orifice metre gives readings 29.43 N/cm and 14.72 N/cm respectively. Co-efficient of discharge of orifice metre is given by 0.6. Find the discharge of water through pipe.
A25)
Given:
d= 24 cm = 0.24 m, p, 29.43 N/cm² = 294.3 kN/m² 4-12 cm-0.12 m. p₂= 14.72 N/cm² Cv= 0.6
Area A₁
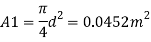
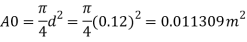
Pressure difference
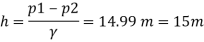
Discharge, Q

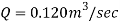
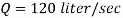
The discharge through the pipe is 120 Lps.
Unit - 4
Fluid Dynamics
Q1) Explain surface and body forces.
A1)
The motion of the fluid element is influenced by the following forces
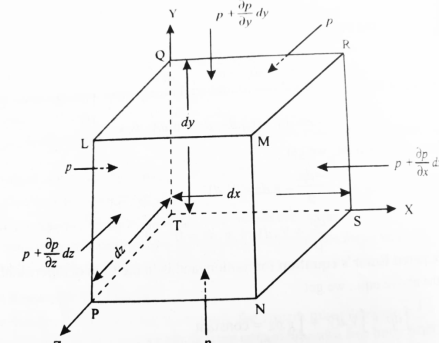
Fig no 1 Surface and body forces
1. Normal pressure force
The net pressure force in X direction = p dydz – ( p + 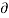 p/
p/  X dx) dy dz
X dx) dy dz
= - 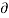 p/
p/ 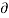 X dx dy dz
X dx dy dz
2. Gravity or body force
Let B be the body force per unit mass of fluid having components Bx, By&Bz
In x, y & z directions respectively.
Then, body force acting on the parallelepiped in the direction of X coordinate is = Bxdx dydz
3. Inertia force
The inertia force acting on the fluid mass, along the X coordinates is given by,
Mass x acceleration = 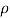 dx dydz du/ dy
dx dydz du/ dy
Q2) Explain Bernoulli’s equation?
A2)
Assumptions made in Bernoulli’s equation.
- The liquid is ideal & incompressible.
- The flow is steady & continuous.
- The flow is along stream line.
- The velocity is uniform over the section & equal to mean velocity.
- Only forces acting on the fluid are the gravity forces & pressure forces.
Bernoulli equation
- Bernoulli’s equation is derived based on the assumption that fluid is non viscous & therefore frictionless.
- Practically, all fluids are real & therefore viscous & there are always some losses in fluid flows.
- These losses have, therefore, to be taken into account in the application of Bernoulli’s equation which gets modified as follows
- P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2 + h L
Where h L= Loss of energy between section 1 & 2.
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
- Venturi meter
- Pitot- tube
- Sub-merged orifice
- Orifice meters
- Rotameter.
Limitation of Bernoulli’s equation
- Velocity of every liquid particle, across any section of pipe is not uniform.
- Bernoulli’s equation is not applicable for fluid with unsteady flow.
- If fluid flowing in a curved path, the energy due to centrifugal force should be taken into account.
Q3) Explain energy principle?
A3)
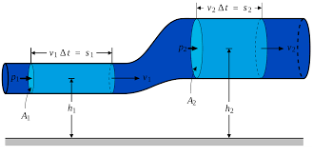
Fig no 2 Energy principal determination apparatus
- In fluid dynamics, Bernoulli's policy states that fluctuations in fluid flow occur simultaneously with a decrease in stationary pressure or a decrease in the available fluid strength.
- The program is named after Daniel Bernoulli who published it in his book Hydrodynamics in 1738. Although Bernoulli found that pressure decreased as the flow rate increased, it was Leonhard Euler who discovered Bernoulli's measurement in a normal way in 1752.
- The principle applies only to isentropic flow: where the effects of irreversible processes (such as disorder) and non-adiabatic processes (e.g. Heat radiation) are small and ignored.
- Bernoulli's system can be applied to a variety of fluid flows, leading to a variety of Bernoulli's calculations; there are different types of Bernoulli's figure for different types of flow.
- The simplest form of Bernoulli's equation is valid for unmistakable flow (e.g. High flow of air and gases running through a low March number). Advanced forms can be used for pressurized flow in high March numbers (see Bernoulli statistical availability).
- Bernoulli's policy can be based on the principle of energy conservation. This means that, with a constant flow, the sum of all the energies in the liquid along the simplest path is the same at all points in that flow path. This requires that the amount of kinetic energy, potential energy and internal energy remain constant.
- Thus an increase in the velocity of a liquid - i.e. an increase in its kinetic energy (strong pressure) - occurs simultaneously a decrease (total energy) of its energy (including constant pressure) and internal energy. When the liquid comes out of a pond, the amount of all kinds of energy is the same in all streamlines because in the pond the energy of each unit (total pressure and gravity ρ g h) is the same everywhere.
- Bernoulli's goal can also be found directly in Isaac Newton's Second Movement of Movement. If a small amount of fluid flows horizontally from a high pressure area to a low pressure region, then there is more pressure behind than before. This gives the net power by volume, speeding it up with ease.
- Fluid particles are subject to pressure and weight only If the fluid flows horizontally and partially along a certain flow path, where the speed increases it may be due to the fluid in that phase moving from the high-pressure region to the low-pressure region; and if its speed decreases, it may be because it has moved from a low-pressure region to a high-pressure region.
- As a result, inside the liquid flowing upwards, the greatest velocity occurs when the pressure is too low, and the lowest velocity occurs when the pressure is too high.
Q4) What is mean by venturimeter?
A4) A venturi meter is a device used for measuring the rate of a flow of a fluid flowing through a pipe it consists of three parts:
- A short converging part,
- Throat, and
- Diverging Part
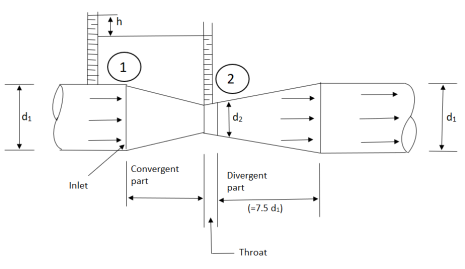
Fig no 3 Venturimeter
It is based on the principle of Bernoulli’s equation.
Expression for Rate of flow through venturimeter
Consider a venturimeter fitted in a horizontal pipe through which a fluid is following as shown in fig.
Let d 1 = diameter at inlet or at section (1)
P 1= pressure at section (1)
V 1= velocity of fluid at section (1)
A 1= area of section (1) =π/4. D2
And d 2, P 2, V 2, a 2 are corresponding values at section (2)
Applying Bernoulli’s equation at section (1) and (2) we get
P1/ 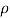 g+V12 /2g+Z1=P2 /
g+V12 /2g+Z1=P2 /  g+V22 /2g+Z2
g+V22 /2g+Z2
As pipe is horizontal hence z 1 = z2
P1/ 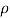 g+V12 /2g =P2 /
g+V12 /2g =P2 /  g+V22 /2g
g+V22 /2g
(P1 – p 2 )/ 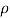 g = V22 /2g - V12 /2g
g = V22 /2g - V12 /2g
But (P1 – p 2 )/ 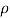 g is the difference of pressure heads at section land 2 and it is equal to h
g is the difference of pressure heads at section land 2 and it is equal to h
∴(P1 – p 2 )/ 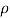 g =h
g =h
Substituting this value of (P1 – p 2 )/ 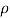 g in the above eqn we get.
g in the above eqn we get.
∴h = V22 /2g - V12 /2g………….. (1)
Now applying continuity equation at section 1 and
a 1 v 1 = a 2 v 2
∴ v 1 = a 2 v 2 / a 1
Substituting this value of v 1 in equation 1
h= V22 /2g – (a 2 v 2 / a 1) 2 / 2 g
h= V22 /2g [ (a 12 –a 22 )/ a 12 ]
V22 = (2 g h a 12 )/ (a 12 –a 22)
Q = a2 v 2
= [a 1 a 2 / 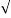 ( a 12 –a 22 ) ] *
( a 12 –a 22 ) ] *  ( 2 g h ) ]
( 2 g h ) ]
Above equation gives the discharge under ideal conditions and is called, Theoretical discharge Actual discharge will be less than theoretical discharge.
Q act = C d * [a 1 a 2 / 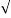 ( a 12 –a 22 )] *
( a 12 –a 22 )] * 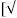 ( 2 g h ) ]
( 2 g h ) ]
Where C d = co-efficient of venturimeter and its value is less than 1.
Q5) Explain orifice meter and pitot tube?
A5)
ORIFICE METER
- It is a device used for measuring the rate of flow of a fluid through a pipe.
- It is a cheaper device compared to venturimeter
- It also works on the same principle as that of venturimeters.
- It consists of a flat circle plate which has a circular shapes edged holed called orifice, which is concentric with the pipe.
- The orifice diameter is kept generally 0.5 times the diameters of the pipe, though it may vary from 0.4 to 0.8 times the pipe diameters.
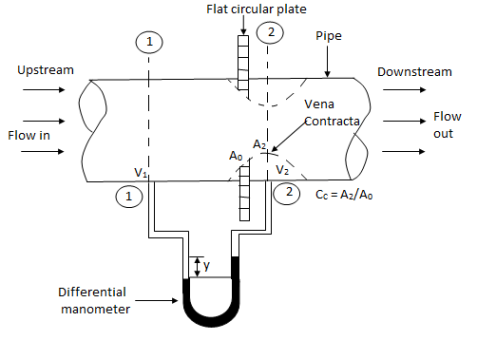
Fig no 4 Orifice meter
A differential manometers is connect at section
(1) Which is at a distance of about 1.5 to 2.0 times the pipe diameters upstream from the orifice plate, and at section
(2) Which is at a distance of about half the diameters of the orifice on the downstream side from the orifice plate.
PITOT TUBES
- It is a device used for measuring the velocity of flow at any point in a pipe or a channel.
- It is based on the principle, that if the velocity of flow at a point becomes zero, the pressure there is increased due to the conservation of the kinetic energy into pressure energy.
- In its simplest form, the pitot- tube consists of a glass tube, bent at right angles as shown in fig.
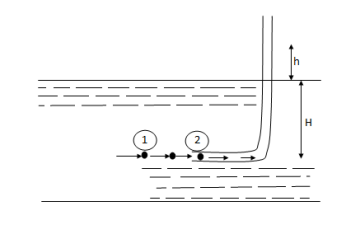
Fig no 5 Pitot tubes
- The Lower end, which is bent though 90° is directed in the upstream direction as shown in fig.
- The liquid rises up in the tube due to the conservation of kinetic energy into pressure energy.
- The velocity is determined by measuring the rise of liquid in the tube.
P1= intensity of pressure at point (1)
V1 = velocity of flow at (1)
P2 = pressure at point (2)
V2 = velocity at point (2), which is zero
H = depth of tube in the liquid.
h = rise of liquid in the tube above the fire Surface
Applying Bernoulli’s equation at points (1) and (2)
We get
P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
But Z 1 = Z2as points (1) and (2) are an the same line and V2 =0
P1 /g= pressure Lead at (1) = H
P2 /g = pressure head at (2) = (h +H)
Substituting these values, we get
H+ V1 2 / 2 g = (h+H)
:. h= V1 2 / 2 g or v 1 = 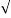 (2 g h )
(2 g h )
This is theoretical velocity.
Actual velocity is gives by (V 1) = Cv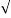 (2 g h)
(2 g h)
Where C v= Co-efficient of pitot-tube
:. Velocity at any point V= Cv (2 g h)
(2 g h)
Q6) Explain momentum principle?
A6)
- It is based on law of conservation of momentum or on the momentum principle, which states that the net force acting on a fluid mass is equal to the change in momentum of flow per unit time in that direction.
- The force acting on a fluid mass m is given by the Newtons second law of motion.
F = m x a
Where a is the acceleration acting in the same direction as force F
But a = d v /d t
F = m x d v /d t
= d (m v) /d t, m is constant can be taken inside the differential
Above equation is known as the momentum principle.
It can be written as F d t = d (m v)
Which is known as the momentum equation & states that the impulse of force F acting on a fluid of mass m in a short interval of time dt is equal to the change of momentum d(m v) in the direction of force.
Momentum Correction Factor ( )
)
Momentum correction factor is defined as the ratio of momentum of the flow per second based on actual velocity to the momentum of the flow per second based on average velocity across a section. It is denoted by.
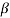 = Momentum per second based on actual velocity/ Momentum per second based on average velocity
= Momentum per second based on actual velocity/ Momentum per second based on average velocity
 = 1 for uniform flow
= 1 for uniform flow
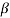 = 1.01 to 1.07 for turbulent flow in pipes
= 1.01 to 1.07 for turbulent flow in pipes
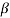 = 4/3 = 1.33 for laminar flow in pipes.
= 4/3 = 1.33 for laminar flow in pipes.
Q7) Explain forces exerted by fluid flow on pipe bend
A7) The energy produced by the liquid flowing in the pipe - bending
- In order to prevent the reflection of the resulting force applied by the liquid flowing into the bend pipe, we will use the basic concept of impulse pressure equation. Before proceeding, it is very important to find and learn the concept of equation intensity.
- Let us assume that the liquid flows through a curved pipe as shown here in the following figure. We are looking at two phases here namely phase 1-1 and section 2-2.

Fig no 6 The energy produced by liquid flowing in bending pipe
V1 = Speed of liquid flowing in phase 1
P1 = Pressure fluid pressure in phase 1
A1 = Section 1 location
V2 = Speed of liquid flowing in phase 2
P2 = Pressure fluid pressure in phase 2
A2 = Section 2 location
FX = The energy released by the liquid flowing in the rotation of the pipe in the X-direction.
FY = A police force that causes fluid to flow in a pipe turn in a Y-direction.
- As we thought above that Fx and FY are forces, they are produced fluid flowing in the pipe turns X and Y respectively.
- In view of Newton's third law of motion, the forces charged by the bending of the pipe in the flowing liquid would be - FX and FY in the direction of X and Y respectively.
- There will be more energy and it will work in the flowing liquid. P1A1 and P2A2 are the compressive forces acting on the liquid in phase 1 and phase 2 respectively.
- We will now remember the pressure number and will have the next X direction figure.
Net power applied to the liquid in the X-direction = Level of pressure change in the X-direction
P1A1 - P2A2 Cos θ - FX = Mass at each unit time x velocity change
P1A1 - P2A2 Cos θ - FX = ρ Q (Final speed in X direction - First velocity in X-direction)
P1A1 - P2A2 Cos θ - FX = ρ Q (V2 Cos θ - V1)
FX = ρ Q (V1 - V2 Cos +) + P1A1 - P2A2 Cos θ
- Similarly, we will remember the pressure figure and will have the following Y direction number.
Net power applies to liquids in Y-direction = Level of pressure change in Y-direction
- P2A2 θ sin - FY = Mass for each unit time x speed change
- P2A2 Sin θ - FY = ρ Q (Final velocity in Y direction - First velocity in Y direction)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
FY = ρ Q (-V2 Sin θ) - P2A2 Sin θ
Let us determine the following (FR) forces acting on the bending of the pipe and the bending of the angle with the resulting force (FR) with the horizontal direction.
F = √ ( Fx2 + Fy2 )
Tan θ = Fy/ Fx
Q8) Explain vortex flow in terms of free and forced?
A8)
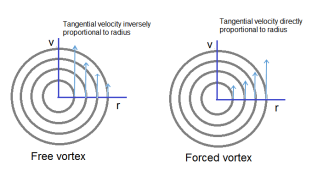
Fig no 7 Vortex flow
Vortex flow: the circulating weight of a liquid or a liquid flow in a curved path.
Free vortex flow
- No external torque or power required. Liquid circulation under certain energies previously given to them. In free vortex machines, the overall energy flow remains constant. There is no power connection between the external source and the flow and any power dissipation of the equipment in motion.
- Liquid circulation is due to the preservation of angular pressure.
- Velocity equals radius.
For a Free vortex flow
v r = constant or v = c/ r
Free vortex flow - Velocity equation
At the center (r = 0) of the velocity rotation approaches infinitely, that point is called a single point.
- Free flow of vortex is irrational; therefore, it is also known as irrotational vortex.
- In free vortex flow, Bernoulli's equation can be used.
Examples include a whirlpool in a river, water flowing from a bathtub or sink, flowing into the space of a centrifugal pump and flowing around a circular bend of a pipe.
Forced vortex flow
- To maintain the forced flow of the vortex, it required a continuous supply of power or external torque.
- All liquid particles circulate with an infinite angular velocity ω as a solid body. Therefore, the flow of a forced vortex is called a solid body rotation.
- Tangential velocity is directly proportional to the radius.
v = r ω
ω = Angular speed.
r = Radius of particle fluid from the axis of rotation.
- The profile of the vortex flow area is illustrated.

Fig no 8 Forced vortex Verified vortex - Height rate
- At the forced vortex force each unit weight increases with increasing radius.
- The forced vortex is not unthinkable; instead the rotational flow with a constant vorticity 2ω.
Examples of forced vortex flow rotate a vessel containing a liquid with constant angular velocity, flowing within a centrifugal pump.
Q9) Explain Euler equation.
A9)
EQUATION OF MOTION - EULERS EQUATION
Consider steady flow of an ideal fluid along the shown tube.
Separate out a small element of fluid of cross- sectional area d A and length d s from stream tube as a free body from the during fluid.
Fig. Shows such a small elements LM of fluid of cross Section area d A and length d s.
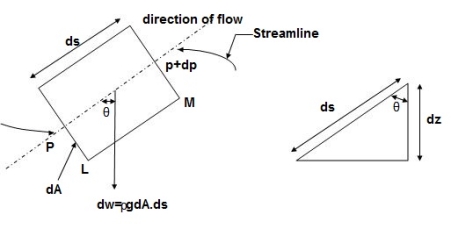
Fig no 9 Equation of motion Diagram
Let, p= Pressure of an the elements at L
p + dp = Pressure on the element at M,and
V=Velocity of the fluid element.
The external forces tending to accelerate the fluid elements in the direction of streamline are as follows:
Net pressure force in the direction of flows is, p.dA – (p+dp)dA = -dp. DA
Component of the weight of the fluid elements in the direction of flows is
= - rho.g.dA.ds.cos
=- rho.g.dA.ds (dz/ds) (:. Cos = dz/ds)
= - rho .g.dA.dz
Mass flow of the fluid element = 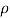 .dA.ds
.dA.ds
The Acceleration of the fluid element
a = dv/dt = dv/ds * ds/dt = v. Dv/ds
Now , according to Newton’s second law of motion, force= mass x acceleration.
:. – dp. DA.-  .g.dA.dz=
.g.dA.dz= .dA.ds x v.dv/ds
.dA.ds x v.dv/ds
Dividing both sides by rho .dA, we get
-dp/-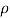 . g.dz= v.dv
. g.dz= v.dv
Or dp/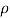 + v.dv + g.dz=0
+ v.dv + g.dz=0
This is the required Euler’s equation for motion.
Integrating the Euler’s equation of motion we get
1/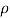 ∫ d p +∫v. d v+ ∫g.dz=constant
∫ d p +∫v. d v+ ∫g.dz=constant
p/ ρ + v2 /2 + g z = constant
Dividing by g, we get
p/  g + v 2/2g + z = constant
g + v 2/2g + z = constant
p /w + v 2/2g + z = constant
Or in other words, P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
Which proves Bernoulli’s equation
Q10) What are the applications of Bernoulli’s equation?
A10)
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
6. Venturi meter
7. Pitot- tube
8. Sub-merged orifice
9. Orifice meters
10. Rotameter.
Q11) Water is flowing through a tapered pipe having diameters 300 mm and 150 mm at section 1 and 2 respectively. The discharge through the pipe is 40 litre/sec. Section 1 is 10 m above datum and section 2 is 6 m above datum. Find the intensity of pressure at section 2 if that at section 1 is
A11)
Given
Q = 40 litre/sec=0.04 m²/sec
Z₁ = 10m
Z₂ =6m
d₁ =300mm=0.3 m
d2 = 150 mm-0.15m
P1 = 400 kN/m²
To find: P:
Area
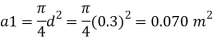
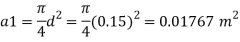
By continuity equation,

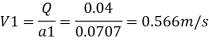
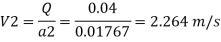
By Bernoulli's equation
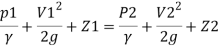
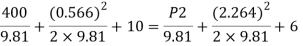

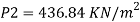
The intensity of pressure of section 2 is 436.84 kN/m²
Q12) A pipe has a length of 200 m with a slope of 1 in 100. The diameter of pipes changes from 1.0 m at higher end to 0.5 m at lower end. Find the pressure at the lower end if the discharge flowing through pipe is 5.00 m/min and pressure at higher end is 45 kN/m².
A12)
Given:
L = 200 m,
P₁ =45 kN/m²
Slope = 1 in 100. z₁ = 0,
d₂ -0.5 m
Q = 5 m/min =5/60 = 0.0833 m/sec
To find: P₂
Slope of pipe is 1 in 100
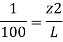

(Since it is below z₁
i.e., datum, it is considered negative) = -2m
By continuity equation
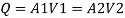
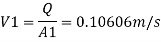
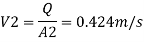
By Bernoulli's equation


Multiplying by 9.81

Q13) In a vertical pipe conveying oil of specific gravity 0.8, two pressure gauges has been installed at A and B, where diameters are 16 cm and 8 cm respectively. A is 2 m above B. The pressure gauge readings have shown that pressure at B is greater than at A by 0.981 N/cm². Neglecting all losses calculate flow rate
A13)
Given:
Specific gravity S=0.8
Diameter at A, dA = 16 cm = 0.16 m
Diameter at B, dB = 8 cm=0.08 m ZA = 2 m, ZB=0
PB=PA+0/981 N/cm^2
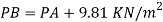
Pressure difference PB-PA= 9.81KN/m^2
Area
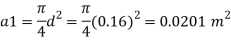
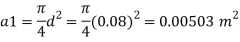
By continuity equation,
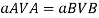
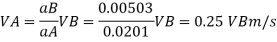
By Bernoulli's equation
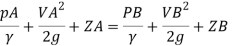
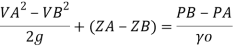
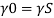
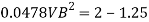
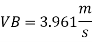
Discharge
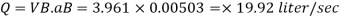
Q14) The top and bottom diameters of a 2m long vertical tapering pipe are 100 mm and 50 mm respectively. Water flows down the pipe at 30 liters por second. Find the pressure difference between the two ends of the pipe.
A14)
Given:
Q = 30 Lps = 0.03 m³/sec,
d1 = 0.05 m. d₂ = 0.1 m, z₁ = 0, z₂ = 2 m
Area


By continuity equation,

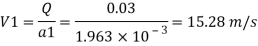
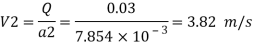
By Bernoulli's equation
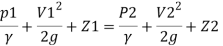
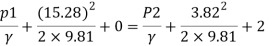

:. The pressure difference between two ends of pipe is 10.415x 10³ Pa
Q15) Obtain an expression for pressure drop from inlet to throat in a horizontally mounted venturimeter in terms of rate of volume flow (0) and inlet diameter (D) for inlet throat diameter ratio is equal to 2.
A15)
d1= Inlet diameter = D
Inlet diameter /Throat diameter= 2
d2 = Throat diameter = D/2
By continuity equation
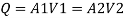
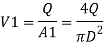
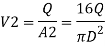
By Bernoulli's equation
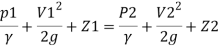
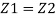
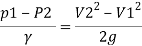
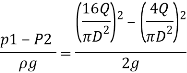

Q16) A venturimeter has an area ratio 9.1, the large diameter being 30 cm. During the flow, the recorded pressure head in the large section is 7.75 m and that at the throat is 5.5 m. Find the discharge through the meter if C,0.98.
A16)
Given:
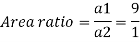

Discharge of inlet
d1 = 0.3 m
Pressure at inlet 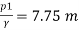
Pressure head at throat 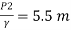
Coefficient of discharge Cd, = 0.98
Area
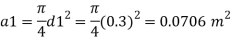
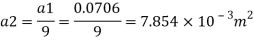
Pressure head
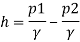
Discharge

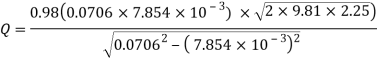

Q17) A venturimeter is to be fitted to a pipe of area 0.01787 m² A maximum discharge of 5 m/min under a pressure of 4.5 m flows through the pipe. Find the diameter at the throat for no negative pressure at the throat.
A17)
Given:
a1 = 0.01767 m². Q = 5 m²/min=0.08333 m/sec
Pressure head = 4.5 m
To find: Diameter of throat i.e. d
Considering the venturimeter to be horizontal
Applying Bernoulli’s equation at section (1) and (2)
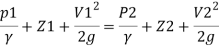
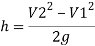
But Z1=Z2
And as there is no negative pressure at throat considering

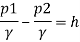
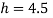
Discharge Q
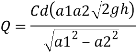
(Assuming C=0.98)
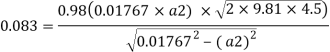

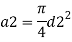

Q18) A venturimeter is fitted to 25 cm diameter pipe. A discharge 10 m³/s flowing through venturimeter, develops a venturi head of 3.5 m of water. Find the minimum diameter of throat so that there is no negative head developed.
A18)
Given:
D = 25 cm = 0.25 m, Q = 10 m³/s,
To find:
d for no negative head developed.
a₁ = area of inlet = 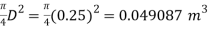
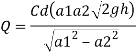
Cd=1
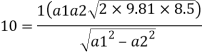
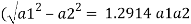
Squaring both side
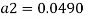
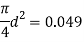
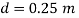
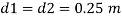
Q19) A jet of water 50 mm diameter is discharging under a constant head of 70 metres. Find the force exerted by the jet on a fixed plate. Take coefficient velocity as 0.9.
A19)
Given:
d = 50 mm = 0.05 m; H = 70 m
And Cd= 0.9
We know that cross-section area of the jet,
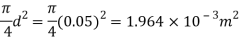
Velocity of the jet,

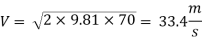
Force exerted by the jet on the fixed plate,
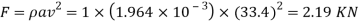
Q20) A horizontal jet of water is issuing under an effective head of 25 m calculate the diameter of the jet if the force exerted by the jet on a vertical plate is 2.22 KN. Take Cv =1
A20)
Given:
H=25 m; F= 2.22 kN and Cv=1
Let d = Diameter of the jet in meters
We know that cross-section area of the jet,
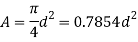
Velocity of the jet,
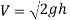
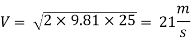
Force exerted by the jet on the fixed plate,
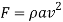
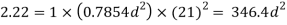
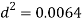
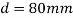
Q21) A jet of water of 100 mm diameter, moving with a velocity of 20 m/s striker a stationary plate Find the force on the plate in the direction of the jet, when
(a) The plate is normal to the jet, and
(b) The angle between the jet and plate is 45°.
A21)
Given:
d = 100 mm= 0.1 ni; V=20 m/s and 8 = 45°.
(a) Force on the plate when it is normal to the jet
We known that cross-section area of the jet,
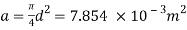
And force on the plate when it is normal to the jet,
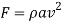
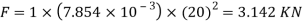
(b) Force on the plate when the angle between the jet and plate is 45%
We also known that force on the plate when the angel between the jet the and plate is 45°,
F = 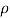 av² = 1x sin²45
av² = 1x sin²45
= 3.142 sin² 45° = 3.142 x (0.707)² = 1.57 kN
Q22) A submarine is cruising at a depth of 20 m in ocean water (density 1020 kg/m). If the forward speed of the submarine is 10 m/s, what readings would be given by pitot and static pressure probes? Assume static probe is located to register free stream static pressure.
A22)
Given:
Speed V = 10 m/s
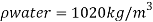
:. Velocity of submarine
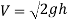

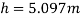
Dynamic pressure
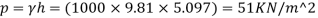
At 20 m depth, static pressure
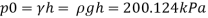
Pitot Probe pressure = Static pressure + Dynamic pressure
= 200.124 +51
= 251.124 kPa
Q23) A pitot tube records of 7.85 kN/m² as the stagnation pressure when it is head at the centre of pipe of 250 mm diameter conveying water. The static pressure in the pipe is 40 mm of mercury (gauge-vacuum). Calculate the discharge through the pipe assuming that the mean velocity of flow is 0.8 times the maximum velocity. Take C, = 0.98.
A23)
Given:
Stagnation pressure p₁= 7.85 kN/m²
Pressure at center of pipe = 40 mm of mercury
P2 = -0.04×13.6 x 9.81-5.336 kN/m
Mean velocity = 0.8 x maximum velocity
Pressure head

Maximum velocity
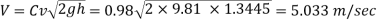
Mean velocity (V)=0.8 x Maximum velocity = 0.8 x 5.033=4.0264 m/sec
Discharge Q
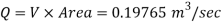
Discharge through the pipe 197.65 Lps.
Q24) A pitot static tube is used to measure velocity of an aeroplane. U-tube differential manometer gives deflection of 100 mm of water. If specific weight of air is 12 N/m and coefficient of pitot tube is 0.98. Determine speed of aeroplane. Neglect compressibility effects.
A24)
Given:
C = 0.98. Ya = 12 N/m².
x = 100 mm of water = 0.1m
To find: Speed of aero plane V = ?
Specific gravity of air
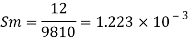
Specific gravity of water
Sw = 1
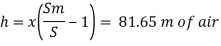
Speed of aircraft
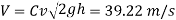
The speed of aeroplane is 39.28 m/s.
Q25) An Orifice of diameter 12 cm is inserted in a pipe of 24 cm diameter. The end pressure gauge fitted upstream and 2 downstream of the orifice metre gives readings 29.43 N/cm and 14.72 N/cm respectively. Co-efficient of discharge of orifice metre is given by 0.6. Find the discharge of water through pipe.
A25)
Given:
d= 24 cm = 0.24 m, p, 29.43 N/cm² = 294.3 kN/m² 4-12 cm-0.12 m. p₂= 14.72 N/cm² Cv= 0.6
Area A₁

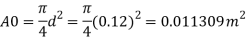
Pressure difference
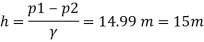
Discharge, Q
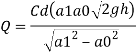
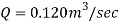
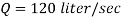
The discharge through the pipe is 120 Lps.
Unit - 4
Unit - 4
Fluid Dynamics
Q1) Explain surface and body forces.
A1)
The motion of the fluid element is influenced by the following forces
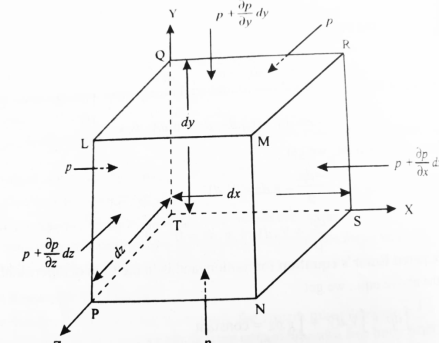
Fig no 1 Surface and body forces
1. Normal pressure force
The net pressure force in X direction = p dydz – ( p + 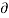 p/
p/  X dx) dy dz
X dx) dy dz
= -  p/
p/ 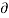 X dx dy dz
X dx dy dz
2. Gravity or body force
Let B be the body force per unit mass of fluid having components Bx, By&Bz
In x, y & z directions respectively.
Then, body force acting on the parallelepiped in the direction of X coordinate is = Bxdx dydz
3. Inertia force
The inertia force acting on the fluid mass, along the X coordinates is given by,
Mass x acceleration = 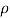 dx dydz du/ dy
dx dydz du/ dy
Q2) Explain Bernoulli’s equation?
A2)
Assumptions made in Bernoulli’s equation.
- The liquid is ideal & incompressible.
- The flow is steady & continuous.
- The flow is along stream line.
- The velocity is uniform over the section & equal to mean velocity.
- Only forces acting on the fluid are the gravity forces & pressure forces.
Bernoulli equation
- Bernoulli’s equation is derived based on the assumption that fluid is non viscous & therefore frictionless.
- Practically, all fluids are real & therefore viscous & there are always some losses in fluid flows.
- These losses have, therefore, to be taken into account in the application of Bernoulli’s equation which gets modified as follows
- P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2 + h L
Where h L= Loss of energy between section 1 & 2.
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
- Venturi meter
- Pitot- tube
- Sub-merged orifice
- Orifice meters
- Rotameter.
Limitation of Bernoulli’s equation
- Velocity of every liquid particle, across any section of pipe is not uniform.
- Bernoulli’s equation is not applicable for fluid with unsteady flow.
- If fluid flowing in a curved path, the energy due to centrifugal force should be taken into account.
Q3) Explain energy principle?
A3)
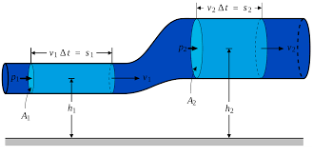
Fig no 2 Energy principal determination apparatus
- In fluid dynamics, Bernoulli's policy states that fluctuations in fluid flow occur simultaneously with a decrease in stationary pressure or a decrease in the available fluid strength.
- The program is named after Daniel Bernoulli who published it in his book Hydrodynamics in 1738. Although Bernoulli found that pressure decreased as the flow rate increased, it was Leonhard Euler who discovered Bernoulli's measurement in a normal way in 1752.
- The principle applies only to isentropic flow: where the effects of irreversible processes (such as disorder) and non-adiabatic processes (e.g. Heat radiation) are small and ignored.
- Bernoulli's system can be applied to a variety of fluid flows, leading to a variety of Bernoulli's calculations; there are different types of Bernoulli's figure for different types of flow.
- The simplest form of Bernoulli's equation is valid for unmistakable flow (e.g. High flow of air and gases running through a low March number). Advanced forms can be used for pressurized flow in high March numbers (see Bernoulli statistical availability).
- Bernoulli's policy can be based on the principle of energy conservation. This means that, with a constant flow, the sum of all the energies in the liquid along the simplest path is the same at all points in that flow path. This requires that the amount of kinetic energy, potential energy and internal energy remain constant.
- Thus an increase in the velocity of a liquid - i.e. an increase in its kinetic energy (strong pressure) - occurs simultaneously a decrease (total energy) of its energy (including constant pressure) and internal energy. When the liquid comes out of a pond, the amount of all kinds of energy is the same in all streamlines because in the pond the energy of each unit (total pressure and gravity ρ g h) is the same everywhere.
- Bernoulli's goal can also be found directly in Isaac Newton's Second Movement of Movement. If a small amount of fluid flows horizontally from a high pressure area to a low pressure region, then there is more pressure behind than before. This gives the net power by volume, speeding it up with ease.
- Fluid particles are subject to pressure and weight only If the fluid flows horizontally and partially along a certain flow path, where the speed increases it may be due to the fluid in that phase moving from the high-pressure region to the low-pressure region; and if its speed decreases, it may be because it has moved from a low-pressure region to a high-pressure region.
- As a result, inside the liquid flowing upwards, the greatest velocity occurs when the pressure is too low, and the lowest velocity occurs when the pressure is too high.
Q4) What is mean by venturimeter?
A4) A venturi meter is a device used for measuring the rate of a flow of a fluid flowing through a pipe it consists of three parts:
- A short converging part,
- Throat, and
- Diverging Part
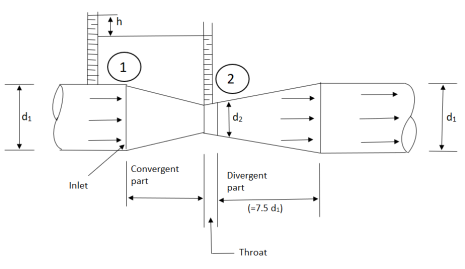
Fig no 3 Venturimeter
It is based on the principle of Bernoulli’s equation.
Expression for Rate of flow through venturimeter
Consider a venturimeter fitted in a horizontal pipe through which a fluid is following as shown in fig.
Let d 1 = diameter at inlet or at section (1)
P 1= pressure at section (1)
V 1= velocity of fluid at section (1)
A 1= area of section (1) =π/4. D2
And d 2, P 2, V 2, a 2 are corresponding values at section (2)
Applying Bernoulli’s equation at section (1) and (2) we get
P1/  g+V12 /2g+Z1=P2 /
g+V12 /2g+Z1=P2 / 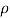 g+V22 /2g+Z2
g+V22 /2g+Z2
As pipe is horizontal hence z 1 = z2
P1/  g+V12 /2g =P2 /
g+V12 /2g =P2 /  g+V22 /2g
g+V22 /2g
(P1 – p 2 )/ 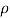 g = V22 /2g - V12 /2g
g = V22 /2g - V12 /2g
But (P1 – p 2 )/ 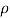 g is the difference of pressure heads at section land 2 and it is equal to h
g is the difference of pressure heads at section land 2 and it is equal to h
∴(P1 – p 2 )/ 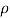 g =h
g =h
Substituting this value of (P1 – p 2 )/ 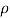 g in the above eqn we get.
g in the above eqn we get.
∴h = V22 /2g - V12 /2g………….. (1)
Now applying continuity equation at section 1 and
a 1 v 1 = a 2 v 2
∴ v 1 = a 2 v 2 / a 1
Substituting this value of v 1 in equation 1
h= V22 /2g – (a 2 v 2 / a 1) 2 / 2 g
h= V22 /2g [ (a 12 –a 22 )/ a 12 ]
V22 = (2 g h a 12 )/ (a 12 –a 22)
Q = a2 v 2
= [a 1 a 2 / 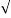 ( a 12 –a 22 ) ] *
( a 12 –a 22 ) ] * 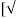 ( 2 g h ) ]
( 2 g h ) ]
Above equation gives the discharge under ideal conditions and is called, Theoretical discharge Actual discharge will be less than theoretical discharge.
Q act = C d * [a 1 a 2 / 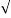 ( a 12 –a 22 )] *
( a 12 –a 22 )] * 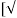 ( 2 g h ) ]
( 2 g h ) ]
Where C d = co-efficient of venturimeter and its value is less than 1.
Q5) Explain orifice meter and pitot tube?
A5)
ORIFICE METER
- It is a device used for measuring the rate of flow of a fluid through a pipe.
- It is a cheaper device compared to venturimeter
- It also works on the same principle as that of venturimeters.
- It consists of a flat circle plate which has a circular shapes edged holed called orifice, which is concentric with the pipe.
- The orifice diameter is kept generally 0.5 times the diameters of the pipe, though it may vary from 0.4 to 0.8 times the pipe diameters.
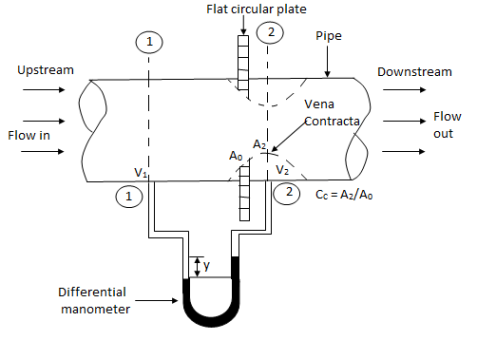
Fig no 4 Orifice meter
A differential manometers is connect at section
(1) Which is at a distance of about 1.5 to 2.0 times the pipe diameters upstream from the orifice plate, and at section
(2) Which is at a distance of about half the diameters of the orifice on the downstream side from the orifice plate.
PITOT TUBES
- It is a device used for measuring the velocity of flow at any point in a pipe or a channel.
- It is based on the principle, that if the velocity of flow at a point becomes zero, the pressure there is increased due to the conservation of the kinetic energy into pressure energy.
- In its simplest form, the pitot- tube consists of a glass tube, bent at right angles as shown in fig.
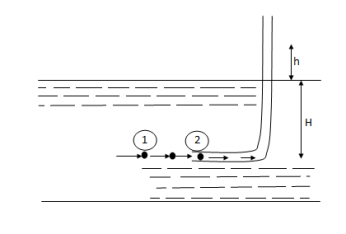
Fig no 5 Pitot tubes
- The Lower end, which is bent though 90° is directed in the upstream direction as shown in fig.
- The liquid rises up in the tube due to the conservation of kinetic energy into pressure energy.
- The velocity is determined by measuring the rise of liquid in the tube.
P1= intensity of pressure at point (1)
V1 = velocity of flow at (1)
P2 = pressure at point (2)
V2 = velocity at point (2), which is zero
H = depth of tube in the liquid.
h = rise of liquid in the tube above the fire Surface
Applying Bernoulli’s equation at points (1) and (2)
We get
P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
But Z 1 = Z2as points (1) and (2) are an the same line and V2 =0
P1 /g= pressure Lead at (1) = H
P2 /g = pressure head at (2) = (h +H)
Substituting these values, we get
H+ V1 2 / 2 g = (h+H)
:. h= V1 2 / 2 g or v 1 = 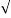 (2 g h )
(2 g h )
This is theoretical velocity.
Actual velocity is gives by (V 1) = Cv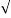 (2 g h)
(2 g h)
Where C v= Co-efficient of pitot-tube
:. Velocity at any point V= Cv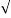 (2 g h)
(2 g h)
Q6) Explain momentum principle?
A6)
- It is based on law of conservation of momentum or on the momentum principle, which states that the net force acting on a fluid mass is equal to the change in momentum of flow per unit time in that direction.
- The force acting on a fluid mass m is given by the Newtons second law of motion.
F = m x a
Where a is the acceleration acting in the same direction as force F
But a = d v /d t
F = m x d v /d t
= d (m v) /d t, m is constant can be taken inside the differential
Above equation is known as the momentum principle.
It can be written as F d t = d (m v)
Which is known as the momentum equation & states that the impulse of force F acting on a fluid of mass m in a short interval of time dt is equal to the change of momentum d(m v) in the direction of force.
Momentum Correction Factor (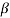 )
)
Momentum correction factor is defined as the ratio of momentum of the flow per second based on actual velocity to the momentum of the flow per second based on average velocity across a section. It is denoted by.
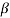 = Momentum per second based on actual velocity/ Momentum per second based on average velocity
= Momentum per second based on actual velocity/ Momentum per second based on average velocity
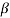 = 1 for uniform flow
= 1 for uniform flow
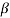 = 1.01 to 1.07 for turbulent flow in pipes
= 1.01 to 1.07 for turbulent flow in pipes
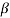 = 4/3 = 1.33 for laminar flow in pipes.
= 4/3 = 1.33 for laminar flow in pipes.
Q7) Explain forces exerted by fluid flow on pipe bend
A7) The energy produced by the liquid flowing in the pipe - bending
- In order to prevent the reflection of the resulting force applied by the liquid flowing into the bend pipe, we will use the basic concept of impulse pressure equation. Before proceeding, it is very important to find and learn the concept of equation intensity.
- Let us assume that the liquid flows through a curved pipe as shown here in the following figure. We are looking at two phases here namely phase 1-1 and section 2-2.
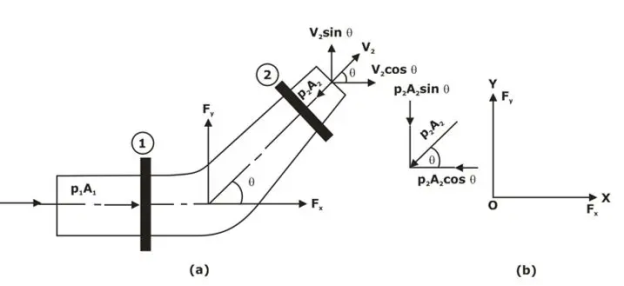
Fig no 6 The energy produced by liquid flowing in bending pipe
V1 = Speed of liquid flowing in phase 1
P1 = Pressure fluid pressure in phase 1
A1 = Section 1 location
V2 = Speed of liquid flowing in phase 2
P2 = Pressure fluid pressure in phase 2
A2 = Section 2 location
FX = The energy released by the liquid flowing in the rotation of the pipe in the X-direction.
FY = A police force that causes fluid to flow in a pipe turn in a Y-direction.
- As we thought above that Fx and FY are forces, they are produced fluid flowing in the pipe turns X and Y respectively.
- In view of Newton's third law of motion, the forces charged by the bending of the pipe in the flowing liquid would be - FX and FY in the direction of X and Y respectively.
- There will be more energy and it will work in the flowing liquid. P1A1 and P2A2 are the compressive forces acting on the liquid in phase 1 and phase 2 respectively.
- We will now remember the pressure number and will have the next X direction figure.
Net power applied to the liquid in the X-direction = Level of pressure change in the X-direction
P1A1 - P2A2 Cos θ - FX = Mass at each unit time x velocity change
P1A1 - P2A2 Cos θ - FX = ρ Q (Final speed in X direction - First velocity in X-direction)
P1A1 - P2A2 Cos θ - FX = ρ Q (V2 Cos θ - V1)
FX = ρ Q (V1 - V2 Cos +) + P1A1 - P2A2 Cos θ
- Similarly, we will remember the pressure figure and will have the following Y direction number.
Net power applies to liquids in Y-direction = Level of pressure change in Y-direction
- P2A2 θ sin - FY = Mass for each unit time x speed change
- P2A2 Sin θ - FY = ρ Q (Final velocity in Y direction - First velocity in Y direction)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
FY = ρ Q (-V2 Sin θ) - P2A2 Sin θ
Let us determine the following (FR) forces acting on the bending of the pipe and the bending of the angle with the resulting force (FR) with the horizontal direction.
F = √ ( Fx2 + Fy2 )
Tan θ = Fy/ Fx
Q8) Explain vortex flow in terms of free and forced?
A8)
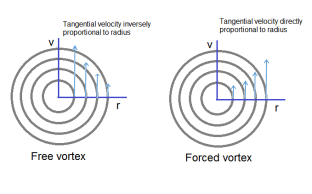
Fig no 7 Vortex flow
Vortex flow: the circulating weight of a liquid or a liquid flow in a curved path.
Free vortex flow
- No external torque or power required. Liquid circulation under certain energies previously given to them. In free vortex machines, the overall energy flow remains constant. There is no power connection between the external source and the flow and any power dissipation of the equipment in motion.
- Liquid circulation is due to the preservation of angular pressure.
- Velocity equals radius.
For a Free vortex flow
v r = constant or v = c/ r
Free vortex flow - Velocity equation
At the center (r = 0) of the velocity rotation approaches infinitely, that point is called a single point.
- Free flow of vortex is irrational; therefore, it is also known as irrotational vortex.
- In free vortex flow, Bernoulli's equation can be used.
Examples include a whirlpool in a river, water flowing from a bathtub or sink, flowing into the space of a centrifugal pump and flowing around a circular bend of a pipe.
Forced vortex flow
- To maintain the forced flow of the vortex, it required a continuous supply of power or external torque.
- All liquid particles circulate with an infinite angular velocity ω as a solid body. Therefore, the flow of a forced vortex is called a solid body rotation.
- Tangential velocity is directly proportional to the radius.
v = r ω
ω = Angular speed.
r = Radius of particle fluid from the axis of rotation.
- The profile of the vortex flow area is illustrated.
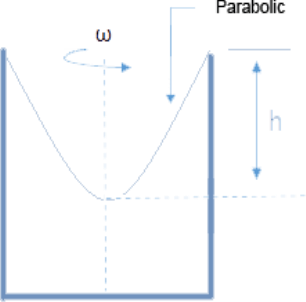
Fig no 8 Forced vortex Verified vortex - Height rate
- At the forced vortex force each unit weight increases with increasing radius.
- The forced vortex is not unthinkable; instead the rotational flow with a constant vorticity 2ω.
Examples of forced vortex flow rotate a vessel containing a liquid with constant angular velocity, flowing within a centrifugal pump.
Q9) Explain Euler equation.
A9)
EQUATION OF MOTION - EULERS EQUATION
Consider steady flow of an ideal fluid along the shown tube.
Separate out a small element of fluid of cross- sectional area d A and length d s from stream tube as a free body from the during fluid.
Fig. Shows such a small elements LM of fluid of cross Section area d A and length d s.
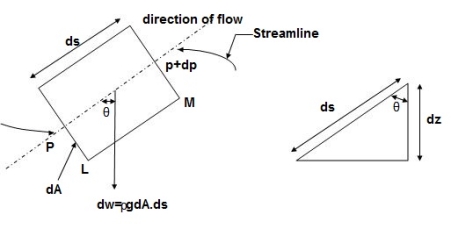
Fig no 9 Equation of motion Diagram
Let, p= Pressure of an the elements at L
p + dp = Pressure on the element at M,and
V=Velocity of the fluid element.
The external forces tending to accelerate the fluid elements in the direction of streamline are as follows:
Net pressure force in the direction of flows is, p.dA – (p+dp)dA = -dp. DA
Component of the weight of the fluid elements in the direction of flows is
= - rho.g.dA.ds.cos
=- rho.g.dA.ds (dz/ds) (:. Cos = dz/ds)
= - rho .g.dA.dz
Mass flow of the fluid element =  .dA.ds
.dA.ds
The Acceleration of the fluid element
a = dv/dt = dv/ds * ds/dt = v. Dv/ds
Now , according to Newton’s second law of motion, force= mass x acceleration.
:. – dp. DA.- 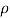 .g.dA.dz=
.g.dA.dz=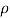 .dA.ds x v.dv/ds
.dA.ds x v.dv/ds
Dividing both sides by rho .dA, we get
-dp/-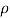 . g.dz= v.dv
. g.dz= v.dv
Or dp/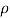 + v.dv + g.dz=0
+ v.dv + g.dz=0
This is the required Euler’s equation for motion.
Integrating the Euler’s equation of motion we get
1/ ∫ d p +∫v. d v+ ∫g.dz=constant
∫ d p +∫v. d v+ ∫g.dz=constant
p/ ρ + v2 /2 + g z = constant
Dividing by g, we get
p/ 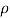 g + v 2/2g + z = constant
g + v 2/2g + z = constant
p /w + v 2/2g + z = constant
Or in other words, P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
Which proves Bernoulli’s equation
Q10) What are the applications of Bernoulli’s equation?
A10)
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
6. Venturi meter
7. Pitot- tube
8. Sub-merged orifice
9. Orifice meters
10. Rotameter.
Q11) Water is flowing through a tapered pipe having diameters 300 mm and 150 mm at section 1 and 2 respectively. The discharge through the pipe is 40 litre/sec. Section 1 is 10 m above datum and section 2 is 6 m above datum. Find the intensity of pressure at section 2 if that at section 1 is
A11)
Given
Q = 40 litre/sec=0.04 m²/sec
Z₁ = 10m
Z₂ =6m
d₁ =300mm=0.3 m
d2 = 150 mm-0.15m
P1 = 400 kN/m²
To find: P:
Area
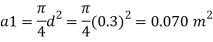
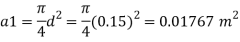
By continuity equation,
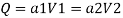


By Bernoulli's equation
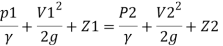
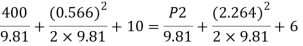
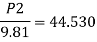
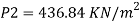
The intensity of pressure of section 2 is 436.84 kN/m²
Q12) A pipe has a length of 200 m with a slope of 1 in 100. The diameter of pipes changes from 1.0 m at higher end to 0.5 m at lower end. Find the pressure at the lower end if the discharge flowing through pipe is 5.00 m/min and pressure at higher end is 45 kN/m².
A12)
Given:
L = 200 m,
P₁ =45 kN/m²
Slope = 1 in 100. z₁ = 0,
d₂ -0.5 m
Q = 5 m/min =5/60 = 0.0833 m/sec
To find: P₂
Slope of pipe is 1 in 100

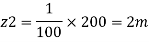
(Since it is below z₁
i.e., datum, it is considered negative) = -2m
By continuity equation

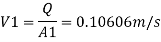
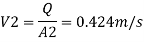
By Bernoulli's equation
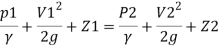

Multiplying by 9.81

Q13) In a vertical pipe conveying oil of specific gravity 0.8, two pressure gauges has been installed at A and B, where diameters are 16 cm and 8 cm respectively. A is 2 m above B. The pressure gauge readings have shown that pressure at B is greater than at A by 0.981 N/cm². Neglecting all losses calculate flow rate
A13)
Given:
Specific gravity S=0.8
Diameter at A, dA = 16 cm = 0.16 m
Diameter at B, dB = 8 cm=0.08 m ZA = 2 m, ZB=0
PB=PA+0/981 N/cm^2
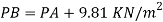
Pressure difference PB-PA= 9.81KN/m^2
Area
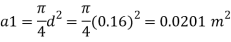
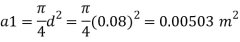
By continuity equation,
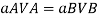
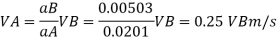
By Bernoulli's equation
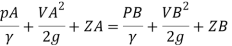
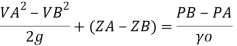
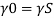

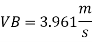
Discharge
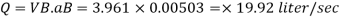
Q14) The top and bottom diameters of a 2m long vertical tapering pipe are 100 mm and 50 mm respectively. Water flows down the pipe at 30 liters por second. Find the pressure difference between the two ends of the pipe.
A14)
Given:
Q = 30 Lps = 0.03 m³/sec,
d1 = 0.05 m. d₂ = 0.1 m, z₁ = 0, z₂ = 2 m
Area

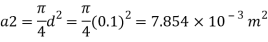
By continuity equation,
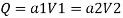

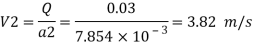
By Bernoulli's equation
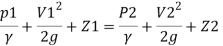
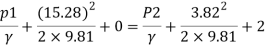

:. The pressure difference between two ends of pipe is 10.415x 10³ Pa
Q15) Obtain an expression for pressure drop from inlet to throat in a horizontally mounted venturimeter in terms of rate of volume flow (0) and inlet diameter (D) for inlet throat diameter ratio is equal to 2.
A15)
d1= Inlet diameter = D
Inlet diameter /Throat diameter= 2
d2 = Throat diameter = D/2
By continuity equation
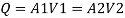
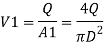
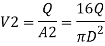
By Bernoulli's equation
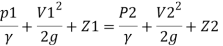
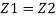
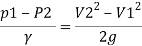
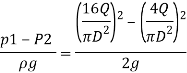
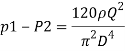
Q16) A venturimeter has an area ratio 9.1, the large diameter being 30 cm. During the flow, the recorded pressure head in the large section is 7.75 m and that at the throat is 5.5 m. Find the discharge through the meter if C,0.98.
A16)
Given:
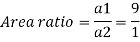

Discharge of inlet
d1 = 0.3 m
Pressure at inlet 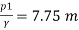
Pressure head at throat 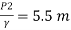
Coefficient of discharge Cd, = 0.98
Area
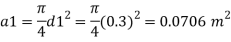
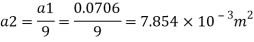
Pressure head
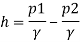
Discharge
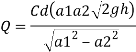
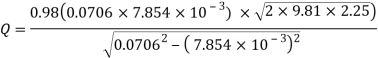
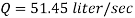
Q17) A venturimeter is to be fitted to a pipe of area 0.01787 m² A maximum discharge of 5 m/min under a pressure of 4.5 m flows through the pipe. Find the diameter at the throat for no negative pressure at the throat.
A17)
Given:
a1 = 0.01767 m². Q = 5 m²/min=0.08333 m/sec
Pressure head = 4.5 m
To find: Diameter of throat i.e. d
Considering the venturimeter to be horizontal
Applying Bernoulli’s equation at section (1) and (2)
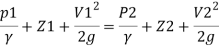
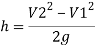
But Z1=Z2
And as there is no negative pressure at throat considering
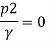

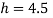
Discharge Q
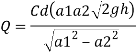
(Assuming C=0.98)
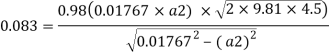
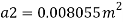
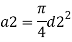
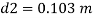
Q18) A venturimeter is fitted to 25 cm diameter pipe. A discharge 10 m³/s flowing through venturimeter, develops a venturi head of 3.5 m of water. Find the minimum diameter of throat so that there is no negative head developed.
A18)
Given:
D = 25 cm = 0.25 m, Q = 10 m³/s,
To find:
d for no negative head developed.
a₁ = area of inlet = 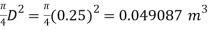

Cd=1
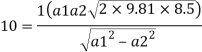

Squaring both side
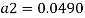
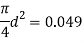
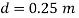
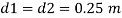
Q19) A jet of water 50 mm diameter is discharging under a constant head of 70 metres. Find the force exerted by the jet on a fixed plate. Take coefficient velocity as 0.9.
A19)
Given:
d = 50 mm = 0.05 m; H = 70 m
And Cd= 0.9
We know that cross-section area of the jet,

Velocity of the jet,
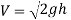
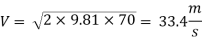
Force exerted by the jet on the fixed plate,
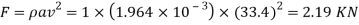
Q20) A horizontal jet of water is issuing under an effective head of 25 m calculate the diameter of the jet if the force exerted by the jet on a vertical plate is 2.22 KN. Take Cv =1
A20)
Given:
H=25 m; F= 2.22 kN and Cv=1
Let d = Diameter of the jet in meters
We know that cross-section area of the jet,
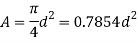
Velocity of the jet,
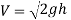
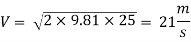
Force exerted by the jet on the fixed plate,
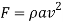
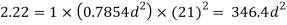
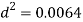
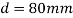
Q21) A jet of water of 100 mm diameter, moving with a velocity of 20 m/s striker a stationary plate Find the force on the plate in the direction of the jet, when
(a) The plate is normal to the jet, and
(b) The angle between the jet and plate is 45°.
A21)
Given:
d = 100 mm= 0.1 ni; V=20 m/s and 8 = 45°.
(a) Force on the plate when it is normal to the jet
We known that cross-section area of the jet,

And force on the plate when it is normal to the jet,
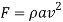
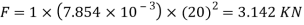
(b) Force on the plate when the angle between the jet and plate is 45%
We also known that force on the plate when the angel between the jet the and plate is 45°,
F = 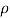 av² = 1x sin²45
av² = 1x sin²45
= 3.142 sin² 45° = 3.142 x (0.707)² = 1.57 kN
Q22) A submarine is cruising at a depth of 20 m in ocean water (density 1020 kg/m). If the forward speed of the submarine is 10 m/s, what readings would be given by pitot and static pressure probes? Assume static probe is located to register free stream static pressure.
A22)
Given:
Speed V = 10 m/s
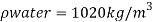
:. Velocity of submarine

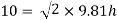
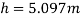
Dynamic pressure
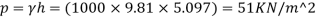
At 20 m depth, static pressure
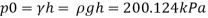
Pitot Probe pressure = Static pressure + Dynamic pressure
= 200.124 +51
= 251.124 kPa
Q23) A pitot tube records of 7.85 kN/m² as the stagnation pressure when it is head at the centre of pipe of 250 mm diameter conveying water. The static pressure in the pipe is 40 mm of mercury (gauge-vacuum). Calculate the discharge through the pipe assuming that the mean velocity of flow is 0.8 times the maximum velocity. Take C, = 0.98.
A23)
Given:
Stagnation pressure p₁= 7.85 kN/m²
Pressure at center of pipe = 40 mm of mercury
P2 = -0.04×13.6 x 9.81-5.336 kN/m
Mean velocity = 0.8 x maximum velocity
Pressure head
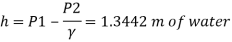
Maximum velocity
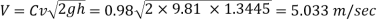
Mean velocity (V)=0.8 x Maximum velocity = 0.8 x 5.033=4.0264 m/sec
Discharge Q
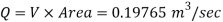
Discharge through the pipe 197.65 Lps.
Q24) A pitot static tube is used to measure velocity of an aeroplane. U-tube differential manometer gives deflection of 100 mm of water. If specific weight of air is 12 N/m and coefficient of pitot tube is 0.98. Determine speed of aeroplane. Neglect compressibility effects.
A24)
Given:
C = 0.98. Ya = 12 N/m².
x = 100 mm of water = 0.1m
To find: Speed of aero plane V = ?
Specific gravity of air
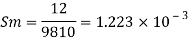
Specific gravity of water
Sw = 1
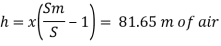
Speed of aircraft
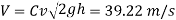
The speed of aeroplane is 39.28 m/s.
Q25) An Orifice of diameter 12 cm is inserted in a pipe of 24 cm diameter. The end pressure gauge fitted upstream and 2 downstream of the orifice metre gives readings 29.43 N/cm and 14.72 N/cm respectively. Co-efficient of discharge of orifice metre is given by 0.6. Find the discharge of water through pipe.
A25)
Given:
d= 24 cm = 0.24 m, p, 29.43 N/cm² = 294.3 kN/m² 4-12 cm-0.12 m. p₂= 14.72 N/cm² Cv= 0.6
Area A₁

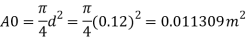
Pressure difference
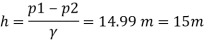
Discharge, Q
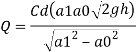
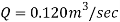
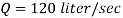
The discharge through the pipe is 120 Lps.
Unit - 4
Unit - 4
Fluid Dynamics
Q1) Explain surface and body forces.
A1)
The motion of the fluid element is influenced by the following forces
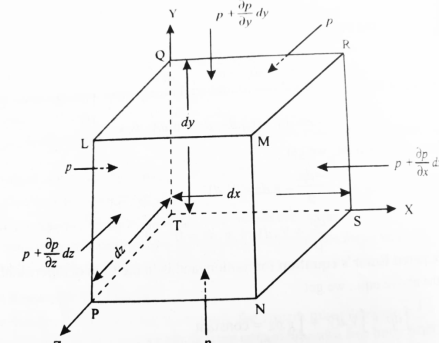
Fig no 1 Surface and body forces
1. Normal pressure force
The net pressure force in X direction = p dydz – ( p + 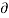 p/
p/ 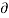 X dx) dy dz
X dx) dy dz
= -  p/
p/ 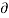 X dx dy dz
X dx dy dz
2. Gravity or body force
Let B be the body force per unit mass of fluid having components Bx, By&Bz
In x, y & z directions respectively.
Then, body force acting on the parallelepiped in the direction of X coordinate is = Bxdx dydz
3. Inertia force
The inertia force acting on the fluid mass, along the X coordinates is given by,
Mass x acceleration =  dx dydz du/ dy
dx dydz du/ dy
Q2) Explain Bernoulli’s equation?
A2)
Assumptions made in Bernoulli’s equation.
- The liquid is ideal & incompressible.
- The flow is steady & continuous.
- The flow is along stream line.
- The velocity is uniform over the section & equal to mean velocity.
- Only forces acting on the fluid are the gravity forces & pressure forces.
Bernoulli equation
- Bernoulli’s equation is derived based on the assumption that fluid is non viscous & therefore frictionless.
- Practically, all fluids are real & therefore viscous & there are always some losses in fluid flows.
- These losses have, therefore, to be taken into account in the application of Bernoulli’s equation which gets modified as follows
- P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2 + h L
Where h L= Loss of energy between section 1 & 2.
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
- Venturi meter
- Pitot- tube
- Sub-merged orifice
- Orifice meters
- Rotameter.
Limitation of Bernoulli’s equation
- Velocity of every liquid particle, across any section of pipe is not uniform.
- Bernoulli’s equation is not applicable for fluid with unsteady flow.
- If fluid flowing in a curved path, the energy due to centrifugal force should be taken into account.
Q3) Explain energy principle?
A3)
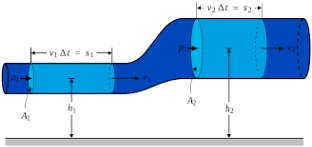
Fig no 2 Energy principal determination apparatus
- In fluid dynamics, Bernoulli's policy states that fluctuations in fluid flow occur simultaneously with a decrease in stationary pressure or a decrease in the available fluid strength.
- The program is named after Daniel Bernoulli who published it in his book Hydrodynamics in 1738. Although Bernoulli found that pressure decreased as the flow rate increased, it was Leonhard Euler who discovered Bernoulli's measurement in a normal way in 1752.
- The principle applies only to isentropic flow: where the effects of irreversible processes (such as disorder) and non-adiabatic processes (e.g. Heat radiation) are small and ignored.
- Bernoulli's system can be applied to a variety of fluid flows, leading to a variety of Bernoulli's calculations; there are different types of Bernoulli's figure for different types of flow.
- The simplest form of Bernoulli's equation is valid for unmistakable flow (e.g. High flow of air and gases running through a low March number). Advanced forms can be used for pressurized flow in high March numbers (see Bernoulli statistical availability).
- Bernoulli's policy can be based on the principle of energy conservation. This means that, with a constant flow, the sum of all the energies in the liquid along the simplest path is the same at all points in that flow path. This requires that the amount of kinetic energy, potential energy and internal energy remain constant.
- Thus an increase in the velocity of a liquid - i.e. an increase in its kinetic energy (strong pressure) - occurs simultaneously a decrease (total energy) of its energy (including constant pressure) and internal energy. When the liquid comes out of a pond, the amount of all kinds of energy is the same in all streamlines because in the pond the energy of each unit (total pressure and gravity ρ g h) is the same everywhere.
- Bernoulli's goal can also be found directly in Isaac Newton's Second Movement of Movement. If a small amount of fluid flows horizontally from a high pressure area to a low pressure region, then there is more pressure behind than before. This gives the net power by volume, speeding it up with ease.
- Fluid particles are subject to pressure and weight only If the fluid flows horizontally and partially along a certain flow path, where the speed increases it may be due to the fluid in that phase moving from the high-pressure region to the low-pressure region; and if its speed decreases, it may be because it has moved from a low-pressure region to a high-pressure region.
- As a result, inside the liquid flowing upwards, the greatest velocity occurs when the pressure is too low, and the lowest velocity occurs when the pressure is too high.
Q4) What is mean by venturimeter?
A4) A venturi meter is a device used for measuring the rate of a flow of a fluid flowing through a pipe it consists of three parts:
- A short converging part,
- Throat, and
- Diverging Part
Fig no 3 Venturimeter
It is based on the principle of Bernoulli’s equation.
Expression for Rate of flow through venturimeter
Consider a venturimeter fitted in a horizontal pipe through which a fluid is following as shown in fig.
Let d 1 = diameter at inlet or at section (1)
P 1= pressure at section (1)
V 1= velocity of fluid at section (1)
A 1= area of section (1) =π/4. D2
And d 2, P 2, V 2, a 2 are corresponding values at section (2)
Applying Bernoulli’s equation at section (1) and (2) we get
P1/ 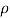 g+V12 /2g+Z1=P2 /
g+V12 /2g+Z1=P2 /  g+V22 /2g+Z2
g+V22 /2g+Z2
As pipe is horizontal hence z 1 = z2
P1/ 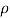 g+V12 /2g =P2 /
g+V12 /2g =P2 /  g+V22 /2g
g+V22 /2g
(P1 – p 2 )/ 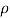 g = V22 /2g - V12 /2g
g = V22 /2g - V12 /2g
But (P1 – p 2 )/ 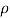 g is the difference of pressure heads at section land 2 and it is equal to h
g is the difference of pressure heads at section land 2 and it is equal to h
∴(P1 – p 2 )/  g =h
g =h
Substituting this value of (P1 – p 2 )/ 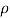 g in the above eqn we get.
g in the above eqn we get.
∴h = V22 /2g - V12 /2g………….. (1)
Now applying continuity equation at section 1 and
a 1 v 1 = a 2 v 2
∴ v 1 = a 2 v 2 / a 1
Substituting this value of v 1 in equation 1
h= V22 /2g – (a 2 v 2 / a 1) 2 / 2 g
h= V22 /2g [ (a 12 –a 22 )/ a 12 ]
V22 = (2 g h a 12 )/ (a 12 –a 22)
Q = a2 v 2
= [a 1 a 2 /  ( a 12 –a 22 ) ] *
( a 12 –a 22 ) ] *  ( 2 g h ) ]
( 2 g h ) ]
Above equation gives the discharge under ideal conditions and is called, Theoretical discharge Actual discharge will be less than theoretical discharge.
Q act = C d * [a 1 a 2 /  ( a 12 –a 22 )] *
( a 12 –a 22 )] * 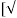 ( 2 g h ) ]
( 2 g h ) ]
Where C d = co-efficient of venturimeter and its value is less than 1.
Q5) Explain orifice meter and pitot tube?
A5)
ORIFICE METER
- It is a device used for measuring the rate of flow of a fluid through a pipe.
- It is a cheaper device compared to venturimeter
- It also works on the same principle as that of venturimeters.
- It consists of a flat circle plate which has a circular shapes edged holed called orifice, which is concentric with the pipe.
- The orifice diameter is kept generally 0.5 times the diameters of the pipe, though it may vary from 0.4 to 0.8 times the pipe diameters.
Fig no 4 Orifice meter
A differential manometers is connect at section
(1) Which is at a distance of about 1.5 to 2.0 times the pipe diameters upstream from the orifice plate, and at section
(2) Which is at a distance of about half the diameters of the orifice on the downstream side from the orifice plate.
PITOT TUBES
- It is a device used for measuring the velocity of flow at any point in a pipe or a channel.
- It is based on the principle, that if the velocity of flow at a point becomes zero, the pressure there is increased due to the conservation of the kinetic energy into pressure energy.
- In its simplest form, the pitot- tube consists of a glass tube, bent at right angles as shown in fig.
Fig no 5 Pitot tubes
- The Lower end, which is bent though 90° is directed in the upstream direction as shown in fig.
- The liquid rises up in the tube due to the conservation of kinetic energy into pressure energy.
- The velocity is determined by measuring the rise of liquid in the tube.
P1= intensity of pressure at point (1)
V1 = velocity of flow at (1)
P2 = pressure at point (2)
V2 = velocity at point (2), which is zero
H = depth of tube in the liquid.
h = rise of liquid in the tube above the fire Surface
Applying Bernoulli’s equation at points (1) and (2)
We get
P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
But Z 1 = Z2as points (1) and (2) are an the same line and V2 =0
P1 /g= pressure Lead at (1) = H
P2 /g = pressure head at (2) = (h +H)
Substituting these values, we get
H+ V1 2 / 2 g = (h+H)
:. h= V1 2 / 2 g or v 1 = 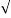 (2 g h )
(2 g h )
This is theoretical velocity.
Actual velocity is gives by (V 1) = Cv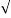 (2 g h)
(2 g h)
Where C v= Co-efficient of pitot-tube
:. Velocity at any point V= Cv (2 g h)
(2 g h)
Q6) Explain momentum principle?
A6)
- It is based on law of conservation of momentum or on the momentum principle, which states that the net force acting on a fluid mass is equal to the change in momentum of flow per unit time in that direction.
- The force acting on a fluid mass m is given by the Newtons second law of motion.
F = m x a
Where a is the acceleration acting in the same direction as force F
But a = d v /d t
F = m x d v /d t
= d (m v) /d t, m is constant can be taken inside the differential
Above equation is known as the momentum principle.
It can be written as F d t = d (m v)
Which is known as the momentum equation & states that the impulse of force F acting on a fluid of mass m in a short interval of time dt is equal to the change of momentum d(m v) in the direction of force.
Momentum Correction Factor (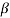 )
)
Momentum correction factor is defined as the ratio of momentum of the flow per second based on actual velocity to the momentum of the flow per second based on average velocity across a section. It is denoted by.
 = Momentum per second based on actual velocity/ Momentum per second based on average velocity
= Momentum per second based on actual velocity/ Momentum per second based on average velocity
 = 1 for uniform flow
= 1 for uniform flow
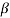 = 1.01 to 1.07 for turbulent flow in pipes
= 1.01 to 1.07 for turbulent flow in pipes
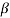 = 4/3 = 1.33 for laminar flow in pipes.
= 4/3 = 1.33 for laminar flow in pipes.
Q7) Explain forces exerted by fluid flow on pipe bend
A7) The energy produced by the liquid flowing in the pipe - bending
- In order to prevent the reflection of the resulting force applied by the liquid flowing into the bend pipe, we will use the basic concept of impulse pressure equation. Before proceeding, it is very important to find and learn the concept of equation intensity.
- Let us assume that the liquid flows through a curved pipe as shown here in the following figure. We are looking at two phases here namely phase 1-1 and section 2-2.
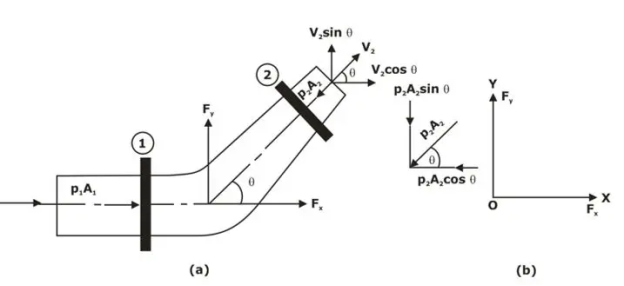
Fig no 6 The energy produced by liquid flowing in bending pipe
V1 = Speed of liquid flowing in phase 1
P1 = Pressure fluid pressure in phase 1
A1 = Section 1 location
V2 = Speed of liquid flowing in phase 2
P2 = Pressure fluid pressure in phase 2
A2 = Section 2 location
FX = The energy released by the liquid flowing in the rotation of the pipe in the X-direction.
FY = A police force that causes fluid to flow in a pipe turn in a Y-direction.
- As we thought above that Fx and FY are forces, they are produced fluid flowing in the pipe turns X and Y respectively.
- In view of Newton's third law of motion, the forces charged by the bending of the pipe in the flowing liquid would be - FX and FY in the direction of X and Y respectively.
- There will be more energy and it will work in the flowing liquid. P1A1 and P2A2 are the compressive forces acting on the liquid in phase 1 and phase 2 respectively.
- We will now remember the pressure number and will have the next X direction figure.
Net power applied to the liquid in the X-direction = Level of pressure change in the X-direction
P1A1 - P2A2 Cos θ - FX = Mass at each unit time x velocity change
P1A1 - P2A2 Cos θ - FX = ρ Q (Final speed in X direction - First velocity in X-direction)
P1A1 - P2A2 Cos θ - FX = ρ Q (V2 Cos θ - V1)
FX = ρ Q (V1 - V2 Cos +) + P1A1 - P2A2 Cos θ
- Similarly, we will remember the pressure figure and will have the following Y direction number.
Net power applies to liquids in Y-direction = Level of pressure change in Y-direction
- P2A2 θ sin - FY = Mass for each unit time x speed change
- P2A2 Sin θ - FY = ρ Q (Final velocity in Y direction - First velocity in Y direction)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
- P2A2 θ sin - FY = ρ Q (V2 Sin θ - 0)
FY = ρ Q (-V2 Sin θ) - P2A2 Sin θ
Let us determine the following (FR) forces acting on the bending of the pipe and the bending of the angle with the resulting force (FR) with the horizontal direction.
F = √ ( Fx2 + Fy2 )
Tan θ = Fy/ Fx
Q8) Explain vortex flow in terms of free and forced?
A8)
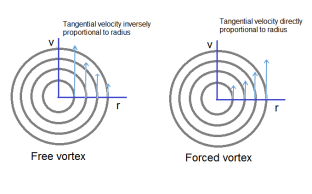
Fig no 7 Vortex flow
Vortex flow: the circulating weight of a liquid or a liquid flow in a curved path.
Free vortex flow
- No external torque or power required. Liquid circulation under certain energies previously given to them. In free vortex machines, the overall energy flow remains constant. There is no power connection between the external source and the flow and any power dissipation of the equipment in motion.
- Liquid circulation is due to the preservation of angular pressure.
- Velocity equals radius.
For a Free vortex flow
v r = constant or v = c/ r
Free vortex flow - Velocity equation
At the center (r = 0) of the velocity rotation approaches infinitely, that point is called a single point.
- Free flow of vortex is irrational; therefore, it is also known as irrotational vortex.
- In free vortex flow, Bernoulli's equation can be used.
Examples include a whirlpool in a river, water flowing from a bathtub or sink, flowing into the space of a centrifugal pump and flowing around a circular bend of a pipe.
Forced vortex flow
- To maintain the forced flow of the vortex, it required a continuous supply of power or external torque.
- All liquid particles circulate with an infinite angular velocity ω as a solid body. Therefore, the flow of a forced vortex is called a solid body rotation.
- Tangential velocity is directly proportional to the radius.
v = r ω
ω = Angular speed.
r = Radius of particle fluid from the axis of rotation.
- The profile of the vortex flow area is illustrated.

Fig no 8 Forced vortex Verified vortex - Height rate
- At the forced vortex force each unit weight increases with increasing radius.
- The forced vortex is not unthinkable; instead the rotational flow with a constant vorticity 2ω.
Examples of forced vortex flow rotate a vessel containing a liquid with constant angular velocity, flowing within a centrifugal pump.
Q9) Explain Euler equation.
A9)
EQUATION OF MOTION - EULERS EQUATION
Consider steady flow of an ideal fluid along the shown tube.
Separate out a small element of fluid of cross- sectional area d A and length d s from stream tube as a free body from the during fluid.
Fig. Shows such a small elements LM of fluid of cross Section area d A and length d s.
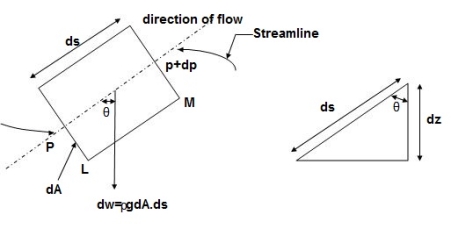
Fig no 9 Equation of motion Diagram
Let, p= Pressure of an the elements at L
p + dp = Pressure on the element at M,and
V=Velocity of the fluid element.
The external forces tending to accelerate the fluid elements in the direction of streamline are as follows:
Net pressure force in the direction of flows is, p.dA – (p+dp)dA = -dp. DA
Component of the weight of the fluid elements in the direction of flows is
= - rho.g.dA.ds.cos
=- rho.g.dA.ds (dz/ds) (:. Cos = dz/ds)
= - rho .g.dA.dz
Mass flow of the fluid element = 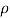 .dA.ds
.dA.ds
The Acceleration of the fluid element
a = dv/dt = dv/ds * ds/dt = v. Dv/ds
Now , according to Newton’s second law of motion, force= mass x acceleration.
:. – dp. DA.- 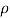 .g.dA.dz=
.g.dA.dz=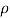 .dA.ds x v.dv/ds
.dA.ds x v.dv/ds
Dividing both sides by rho .dA, we get
-dp/-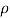 . g.dz= v.dv
. g.dz= v.dv
Or dp/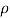 + v.dv + g.dz=0
+ v.dv + g.dz=0
This is the required Euler’s equation for motion.
Integrating the Euler’s equation of motion we get
1/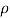 ∫ d p +∫v. d v+ ∫g.dz=constant
∫ d p +∫v. d v+ ∫g.dz=constant
p/ ρ + v2 /2 + g z = constant
Dividing by g, we get
p/ 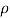 g + v 2/2g + z = constant
g + v 2/2g + z = constant
p /w + v 2/2g + z = constant
Or in other words, P1/ W+V1 2 / 2 g +Z1=P2/ W+V22 / 2g+Z2
Which proves Bernoulli’s equation
Q10) What are the applications of Bernoulli’s equation?
A10)
Applications of Bernoulli’s equation
Bernoulli’s equation is applied is all problems of incompressible fluid flow where energy considerations are involved, But we shall consider its applications to the following measuring devices:
6. Venturi meter
7. Pitot- tube
8. Sub-merged orifice
9. Orifice meters
10. Rotameter.
Q11) Water is flowing through a tapered pipe having diameters 300 mm and 150 mm at section 1 and 2 respectively. The discharge through the pipe is 40 litre/sec. Section 1 is 10 m above datum and section 2 is 6 m above datum. Find the intensity of pressure at section 2 if that at section 1 is
A11)
Given
Q = 40 litre/sec=0.04 m²/sec
Z₁ = 10m
Z₂ =6m
d₁ =300mm=0.3 m
d2 = 150 mm-0.15m
P1 = 400 kN/m²
To find: P:
Area
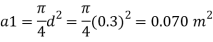
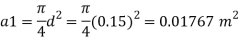
By continuity equation,
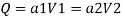

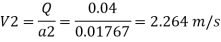
By Bernoulli's equation
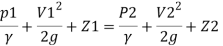
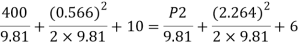
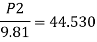
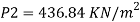
The intensity of pressure of section 2 is 436.84 kN/m²
Q12) A pipe has a length of 200 m with a slope of 1 in 100. The diameter of pipes changes from 1.0 m at higher end to 0.5 m at lower end. Find the pressure at the lower end if the discharge flowing through pipe is 5.00 m/min and pressure at higher end is 45 kN/m².
A12)
Given:
L = 200 m,
P₁ =45 kN/m²
Slope = 1 in 100. z₁ = 0,
d₂ -0.5 m
Q = 5 m/min =5/60 = 0.0833 m/sec
To find: P₂
Slope of pipe is 1 in 100
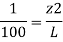
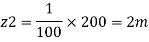
(Since it is below z₁
i.e., datum, it is considered negative) = -2m
By continuity equation
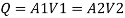
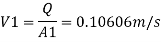

By Bernoulli's equation
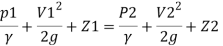

Multiplying by 9.81
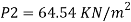
Q13) In a vertical pipe conveying oil of specific gravity 0.8, two pressure gauges has been installed at A and B, where diameters are 16 cm and 8 cm respectively. A is 2 m above B. The pressure gauge readings have shown that pressure at B is greater than at A by 0.981 N/cm². Neglecting all losses calculate flow rate
A13)
Given:
Specific gravity S=0.8
Diameter at A, dA = 16 cm = 0.16 m
Diameter at B, dB = 8 cm=0.08 m ZA = 2 m, ZB=0
PB=PA+0/981 N/cm^2

Pressure difference PB-PA= 9.81KN/m^2
Area


By continuity equation,
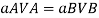
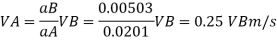
By Bernoulli's equation
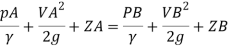
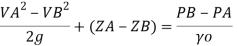
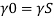

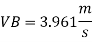
Discharge
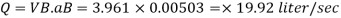
Q14) The top and bottom diameters of a 2m long vertical tapering pipe are 100 mm and 50 mm respectively. Water flows down the pipe at 30 liters por second. Find the pressure difference between the two ends of the pipe.
A14)
Given:
Q = 30 Lps = 0.03 m³/sec,
d1 = 0.05 m. d₂ = 0.1 m, z₁ = 0, z₂ = 2 m
Area
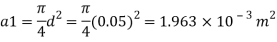

By continuity equation,

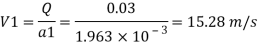
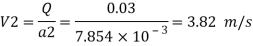
By Bernoulli's equation
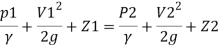
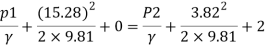
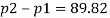
:. The pressure difference between two ends of pipe is 10.415x 10³ Pa
Q15) Obtain an expression for pressure drop from inlet to throat in a horizontally mounted venturimeter in terms of rate of volume flow (0) and inlet diameter (D) for inlet throat diameter ratio is equal to 2.
A15)
d1= Inlet diameter = D
Inlet diameter /Throat diameter= 2
d2 = Throat diameter = D/2
By continuity equation
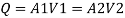

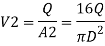
By Bernoulli's equation
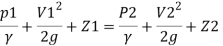

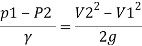
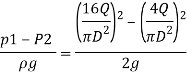
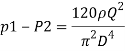
Q16) A venturimeter has an area ratio 9.1, the large diameter being 30 cm. During the flow, the recorded pressure head in the large section is 7.75 m and that at the throat is 5.5 m. Find the discharge through the meter if C,0.98.
A16)
Given:

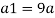
Discharge of inlet
d1 = 0.3 m
Pressure at inlet 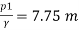
Pressure head at throat 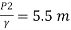
Coefficient of discharge Cd, = 0.98
Area
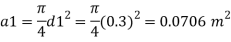
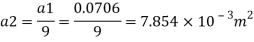
Pressure head
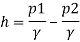
Discharge
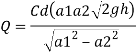
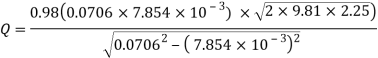
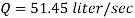
Q17) A venturimeter is to be fitted to a pipe of area 0.01787 m² A maximum discharge of 5 m/min under a pressure of 4.5 m flows through the pipe. Find the diameter at the throat for no negative pressure at the throat.
A17)
Given:
a1 = 0.01767 m². Q = 5 m²/min=0.08333 m/sec
Pressure head = 4.5 m
To find: Diameter of throat i.e. d
Considering the venturimeter to be horizontal
Applying Bernoulli’s equation at section (1) and (2)
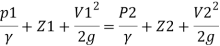
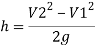
But Z1=Z2
And as there is no negative pressure at throat considering
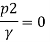
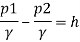
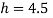
Discharge Q
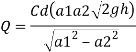
(Assuming C=0.98)
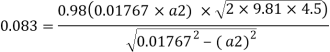
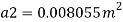


Q18) A venturimeter is fitted to 25 cm diameter pipe. A discharge 10 m³/s flowing through venturimeter, develops a venturi head of 3.5 m of water. Find the minimum diameter of throat so that there is no negative head developed.
A18)
Given:
D = 25 cm = 0.25 m, Q = 10 m³/s,
To find:
d for no negative head developed.
a₁ = area of inlet = 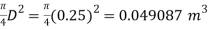

Cd=1
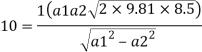
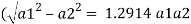
Squaring both side

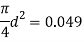
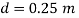
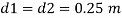
Q19) A jet of water 50 mm diameter is discharging under a constant head of 70 metres. Find the force exerted by the jet on a fixed plate. Take coefficient velocity as 0.9.
A19)
Given:
d = 50 mm = 0.05 m; H = 70 m
And Cd= 0.9
We know that cross-section area of the jet,
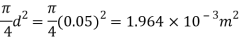
Velocity of the jet,

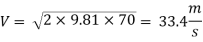
Force exerted by the jet on the fixed plate,
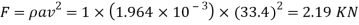
Q20) A horizontal jet of water is issuing under an effective head of 25 m calculate the diameter of the jet if the force exerted by the jet on a vertical plate is 2.22 KN. Take Cv =1
A20)
Given:
H=25 m; F= 2.22 kN and Cv=1
Let d = Diameter of the jet in meters
We know that cross-section area of the jet,
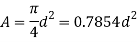
Velocity of the jet,
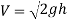
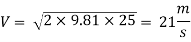
Force exerted by the jet on the fixed plate,
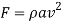
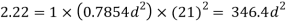
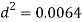

Q21) A jet of water of 100 mm diameter, moving with a velocity of 20 m/s striker a stationary plate Find the force on the plate in the direction of the jet, when
(a) The plate is normal to the jet, and
(b) The angle between the jet and plate is 45°.
A21)
Given:
d = 100 mm= 0.1 ni; V=20 m/s and 8 = 45°.
(a) Force on the plate when it is normal to the jet
We known that cross-section area of the jet,

And force on the plate when it is normal to the jet,
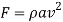

(b) Force on the plate when the angle between the jet and plate is 45%
We also known that force on the plate when the angel between the jet the and plate is 45°,
F = 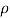 av² = 1x sin²45
av² = 1x sin²45
= 3.142 sin² 45° = 3.142 x (0.707)² = 1.57 kN
Q22) A submarine is cruising at a depth of 20 m in ocean water (density 1020 kg/m). If the forward speed of the submarine is 10 m/s, what readings would be given by pitot and static pressure probes? Assume static probe is located to register free stream static pressure.
A22)
Given:
Speed V = 10 m/s
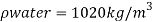
:. Velocity of submarine
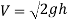
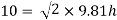

Dynamic pressure
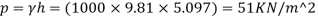
At 20 m depth, static pressure
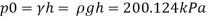
Pitot Probe pressure = Static pressure + Dynamic pressure
= 200.124 +51
= 251.124 kPa
Q23) A pitot tube records of 7.85 kN/m² as the stagnation pressure when it is head at the centre of pipe of 250 mm diameter conveying water. The static pressure in the pipe is 40 mm of mercury (gauge-vacuum). Calculate the discharge through the pipe assuming that the mean velocity of flow is 0.8 times the maximum velocity. Take C, = 0.98.
A23)
Given:
Stagnation pressure p₁= 7.85 kN/m²
Pressure at center of pipe = 40 mm of mercury
P2 = -0.04×13.6 x 9.81-5.336 kN/m
Mean velocity = 0.8 x maximum velocity
Pressure head
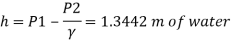
Maximum velocity

Mean velocity (V)=0.8 x Maximum velocity = 0.8 x 5.033=4.0264 m/sec
Discharge Q
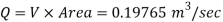
Discharge through the pipe 197.65 Lps.
Q24) A pitot static tube is used to measure velocity of an aeroplane. U-tube differential manometer gives deflection of 100 mm of water. If specific weight of air is 12 N/m and coefficient of pitot tube is 0.98. Determine speed of aeroplane. Neglect compressibility effects.
A24)
Given:
C = 0.98. Ya = 12 N/m².
x = 100 mm of water = 0.1m
To find: Speed of aero plane V = ?
Specific gravity of air
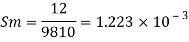
Specific gravity of water
Sw = 1

Speed of aircraft
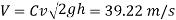
The speed of aeroplane is 39.28 m/s.
Q25) An Orifice of diameter 12 cm is inserted in a pipe of 24 cm diameter. The end pressure gauge fitted upstream and 2 downstream of the orifice metre gives readings 29.43 N/cm and 14.72 N/cm respectively. Co-efficient of discharge of orifice metre is given by 0.6. Find the discharge of water through pipe.
A25)
Given:
d= 24 cm = 0.24 m, p, 29.43 N/cm² = 294.3 kN/m² 4-12 cm-0.12 m. p₂= 14.72 N/cm² Cv= 0.6
Area A₁
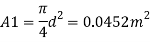
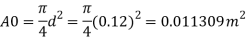
Pressure difference
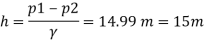
Discharge, Q
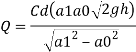
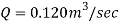
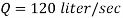
The discharge through the pipe is 120 Lps.