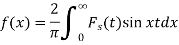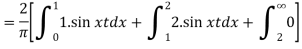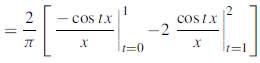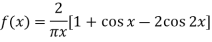Unit – 4
Fourier Series & Fourier Transform
Q1) What is periodic function?
A1)
If the value of the function (y-axis) repeats itself on x-axis, then the function is called periodic function.
Suppose f(x) is a function, then
If f(x) = f(x + t) = f (x + 2t) = …………….
Then t is called the periodic of the function f(x).
Suppose if we take sin x, then it repeats its value after the period of 2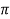
such that, we write this as
Sin x = sin (x + 2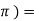
sin ( x + 4
) ………….
We can say that sin x is a periodic function with the period of 2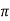
Q2) Define Fourier series.
A2)
A series of the form,
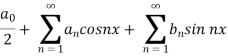
Is called fourier series, where
are constants.
Any periodic function can be expanded in the form of fourier series.
Q3) How do we determine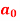 
, 
, 
of Fourier series
A3)
Determination of 
, 
, 
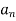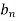of Fourier series-
We know that, the fourier series,
f(x) = 
------------ eq.(1)
To find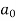 
–
Intergrate equation (1) on both sides, from 0 to 2π
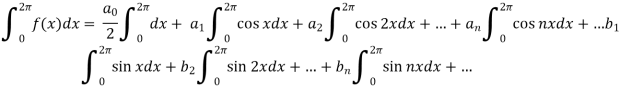
Which gives,
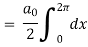
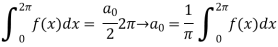
To find 
-
Multiply each side of eq. (1) by cos nx and integrate from 0 to 2π
We get,
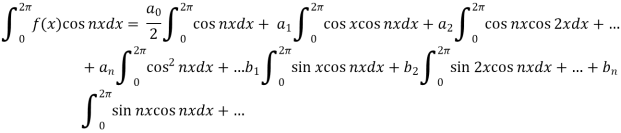
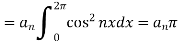
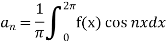
Similarly we can find 
by, multiplying eq. (1) by sin nx and integrating from 0 to 2π
Q4) Find the fourier series of the function f(x) = x where 0 < x < 2 π.
A4)
We know that, from fourier series,
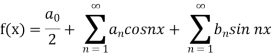
First we will find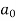 
,
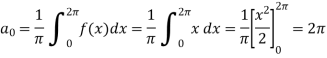
Now,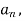 
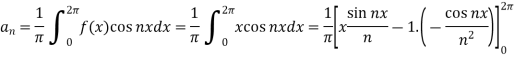
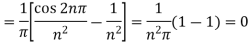
And 
,
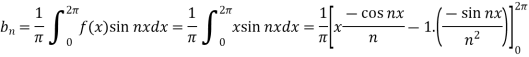
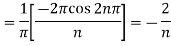
Put these value in Fourier series, we get
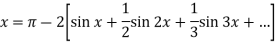
Q5) Find the Fourier series for f(x) = 
in the interval 
.
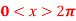A5)
Suppose
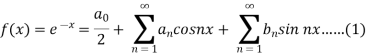
Then-
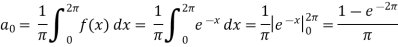
And
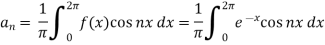
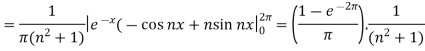
So that-
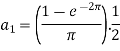
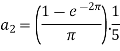
And then-
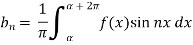
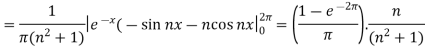
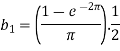
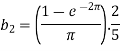
Now put these value in equations (1), we get-
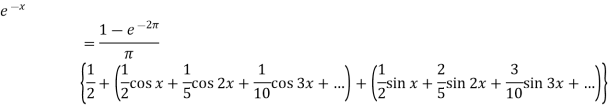
Q6) Find the fourier series of the function f(x) = x where 0 < x < 2 π.
A6)
We know that, from fourier series,
f(x) =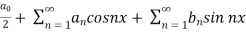 
First we will find 
,
Now,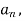 
And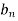 
,
Put these value in fourier series, we get
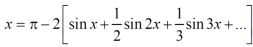
Q7) Find the fourier series for f(x) = x / 2 over the interval 0 < x < 2π
And has period 2π
A7)
First we will find
= 
= 
=
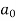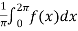
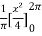
= π
= π
Similarly,
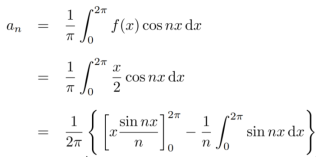
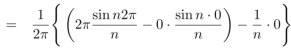
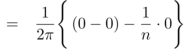
Which gives,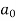 
= 0
Now,
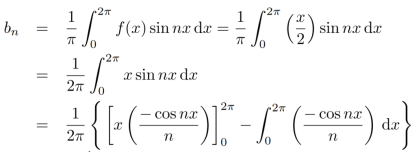
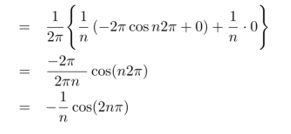
We get,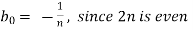 
We know that, the fourier series
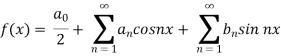
Put these values in fourier series, we get
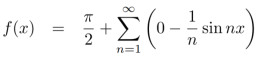
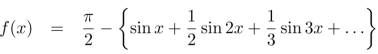
Q8) What do you understand by change of interval?
A8)
In many problems, the period of the function is not always 2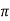 
but T or 2c.
This period must be converted to the length 2
. The independent variable x is also to be changed proportionally.
Let the function f (x) be defined in the interval ( – c, c). Now we want to change the function to the period of 2
so that we can use the formulae of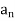 
, 
.
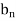So that 2 c is the interval for the variable x
1 is the interval for the variable = x/2c
2 
is the interval for the variable = x 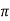2 
/ 2 =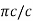 
So put,
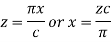
Thus the function f (x) of period 2c is transformed to the function
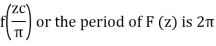
F (z) can be expanded in the Fourier series.
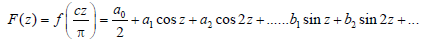
Where
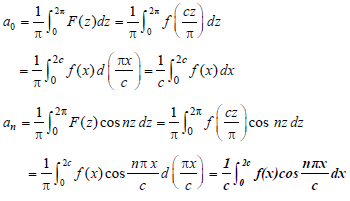
And
Q9) What is odd function?
A9)
Odd function-
A function f(x), which is written as,
f( - x ) = - f(x)
Is known as odd function.
The graph of the odd function looks like-
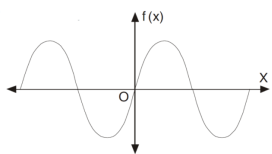
The graph of the function is symmetric about x-axis.
And the area under the curve from -π to π is always zero.(see fig)
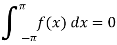
Q10) Find the Fourier expression of f(x) = x³ for –π< x <π.
A10)
Here, we can see that f(x) Is an odd function
So that,
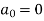and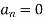
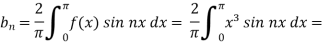
We will use here,
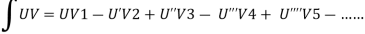
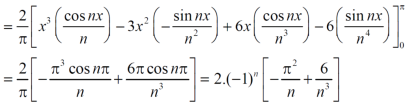
We get the value of f(x),
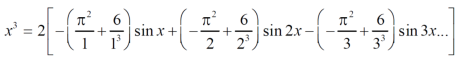
A12) Find the Fourier series expansion of the periodic function of period 2π.
f(x) = x², -π ≤x ≤π
A12)
The given function is even, so that,
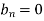
We will find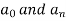 
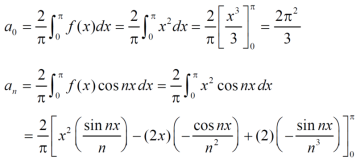
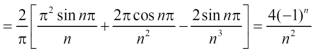
The Fourier series will be,
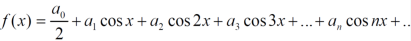
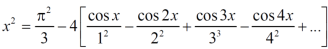
Q11) Find a Fourier series for
; 
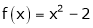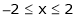
A13)
Here
; 
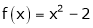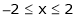
Since f(x) is even function hence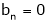 
It’s Fourier series is
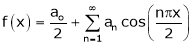… (1)
Where
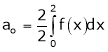
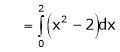
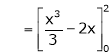
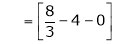
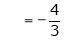
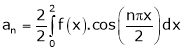
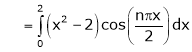
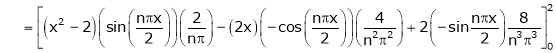
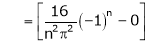
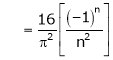
Hence equation (1) becomes,
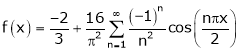
Q12) Find the Fourier sine series for the function-
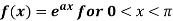
Where ‘a’ is a constant.
A14)
Here we know-
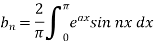
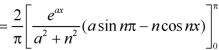
We know that-
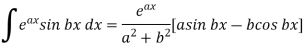
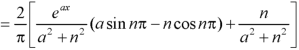
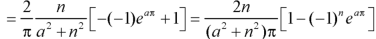
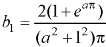
And
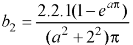
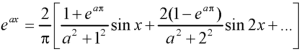
Q13) What do you understand by complex form of fourier series?
A15)
The Fourier integral of f(x) is-
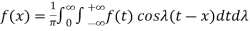………. (1)
Which can be written as-
…………… (2)
Because 
is an even function of 
. Also since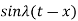 
is an odd function of 
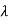then we have-
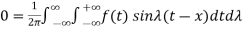……… (3)
Multiply (3) by ‘i’ and add to (2)-
We get-
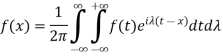
Which is the complex form of the Fourier integral.
Q14) Using the complex form, find the Fourier series of the function
f(x) = sinx = 
A16)
We calculate the coefficients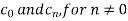 
= 
=
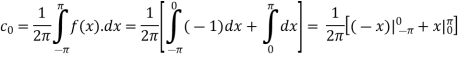
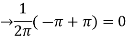
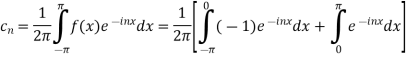
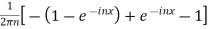
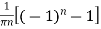
Hence the Fourier series of the function in complex form is
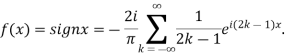
We can transform the series and write it in the real form by renaming it as
n=2k-1,n=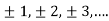
= 
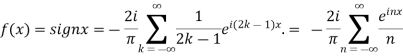
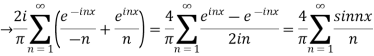
Q15) Find the Fourier transform of-
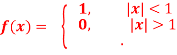
Hence evaluate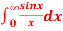 
A17)
As we know that the Fourier transform of f(x) will be-
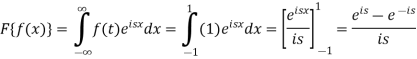
So that-
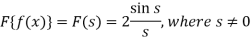
For s = 0, we get- F(s) = 2
Hence by the inverse formula, we get-
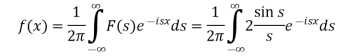
Putting x = 0, we get
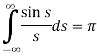
So-
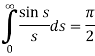
Q16) Find the Fourier transform of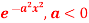 
A18)
As we know that the Fourier transform of f(x) will be-
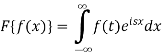
So that-
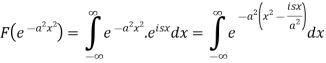
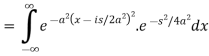
Now put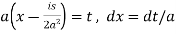 
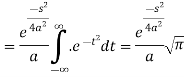
So that-
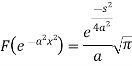
Q17) Find the Fourier sine transform of 
A19)
Here x being positive in the interval (0, ∞)
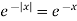
Fourier sine transform of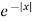 
will be-
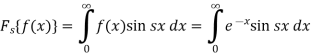
Q18) Find the Fourier cosine transform of-
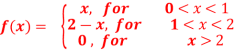
A20)
We know that the Fourier cosine transform of f(x)-
= 
= 
= 
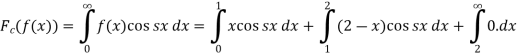
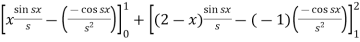
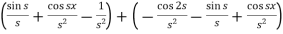
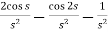
Q19) State and prove convolution theorem.
A21)
The Fourier transform of the convolution of f (x) and g (x) is the product of their Fourier Transforms.
Which means-
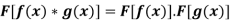
Proof:
We have-
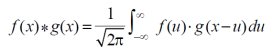
Take Fourier to transform on both sides of the above equation, we get-
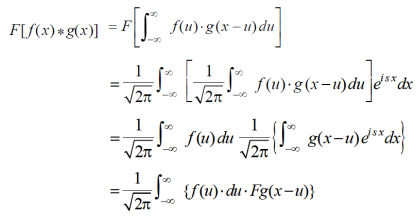
By shifting property-
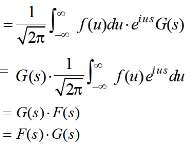
By inversion, we get-
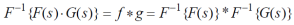
Q20) Example) Solve for f (x) the integral equation
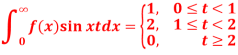
A22)
By the definition,
