Unit - 3
Theory of relativity
Q1) Calculate the time interval of the signal using Lorentz transformation when a spacecraft S’ is crossed by another spacecraft S at a speed of c/2 on their way to Alpha Centauri. When spacecraft S Crosses S’, the captain of S’ sends a signal that lasts for 1.2s. What is the fine time interval of the single?
A1)
Given:
Δt’ = t‘2−t‘1 = 1.2s
Δx’ = x‘2−x‘1 = 0
The time signal starts and ends at (x’, t‘1)
Using the first Lorentz transformation equation we get,
Δt = t2 – t1
Δx = x2 – x1
Δt= 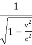 (
(  t’ +
t’ + 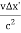 )
)
As the time interval of S’ is fixed, we can consider Δx’ = 0 and the time interval δt becomes:
Δt=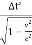
Substituting Δt’ = 1.2s we get
Δt=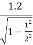
Δt = 1.6s
Q2) A certain process requires 10-6 sec to occur in an atom at rest in laboratory. How much time will this process require to an observer in the laboratory? When the atom is moving with speed of 5 x107 m/sec.
A2)
Given Δt = 10-6 sec
v = 5 x109 cm/sec
∆t= 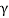 (∆t')
(∆t')
∆t' = ∆t /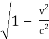
∆t' = = 1.028 x 10-6 sec
= 1.028 x 10-6 sec
Q3) Compute the contracted length of an object whose initial length 10m and travel with a velocity 0.75c?
A3)
Given parameters are,
L0 = 10m
v = 0.75c
c = Speed of light (3.0 x 108 m/s)
The formula for length contraction is
l0 = 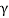 l
l
Or L0 = 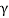 L
L
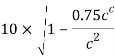
L = 10 × √1- (0.75)2
L = 10 × √(o.438)
L = 6.618 m.
Q4) A observer on earth sees a rocket zoom by at .95c. If the rocket is measured to be 5.5 m in length, how long is the rocket ship as measure by the astronaut inside the rocket?
A4)
The formula for length contraction is
l0 =  l
l
Or
L0 =  L
L
L = 5.5 m
v = .95c
L0 = ?
5.5 = L0×(1- 0.95c2/c2)
5.5 = L0×(0.312)
L0 = 5.5/(0.312) = 17.6 m
Q5) Difference between inertial & non-inertial frames?
A5)
Inertial frame of reference
An inertial frame is defined as one in which Newton’s law of inertia holds that is anybody which isn’t being acted on by an outside force stays at rest if it is initially at rest, or continues to move at a constant velocity if that’s what it was doing to begin with.
Non-inertial frame of reference
A frame that is accelerated with respect to the assumed inertial frame of reference. Newton’s law will not hold true in these frames.
Q6) The Hypersonic Technology Vehicle 2 (HTV-2) is an experimental rocket vehicle capable of traveling at 21,000 km/h (5830 m/s). If an electronic clock in the HTV-2 measures a time interval of exactly 1-s duration, what would observers on Earth measure the time interval to be?
A6)
Given
Δτ=1s
v=5830m/s.
Δt =?
We know ∆t= t1 – t2 =  (∆t')
(∆t')
Δt=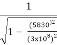 = 1.000000000189s
= 1.000000000189s
Q7) What is Galilean Transformations? How velocity and acceleration transform?
A7) Galilean Coordinate Transformations: For simplicity: - Let coordinates in both references equal at (t = 0). Use Cartesian coordinate systems.
Newton’s laws are only applicable at inertial reference frames. According to Galilean transformations, Newton’s laws are invariant at any inertial reference frame. In other words, the mechanical movement of a particle is exactly the same at two different reference frames, If both of them are inertial (i.e. one moves with constant velocity w.r.t the other & vice versa).
There is no mechanical experiment by which one can distinguish whether a system is at rest or is moving with a constant speed in a straight line (Galilean relativity).
Suppose we know from experiment that these laws of mechanics are true in one frame of reference. How do they look in another frame, moving with respect to the first frame?
To find out, we have to figure out how to get from position, velocity and acceleration in one frame to the corresponding quantities in the second frame.
Obviously, the two frames must have a constant relative velocity, otherwise the law of inertia won’t hold in both of them. Let’s choose the coordinates so that this velocity is along the x-axis of both of them.
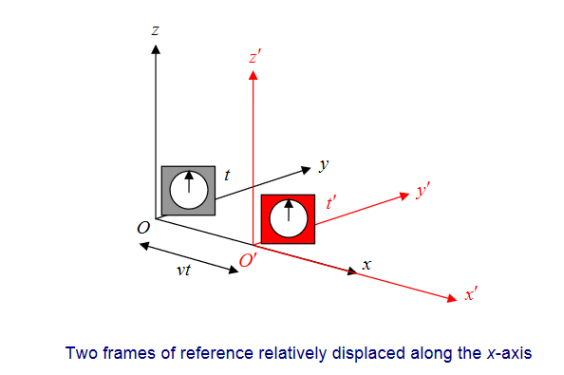
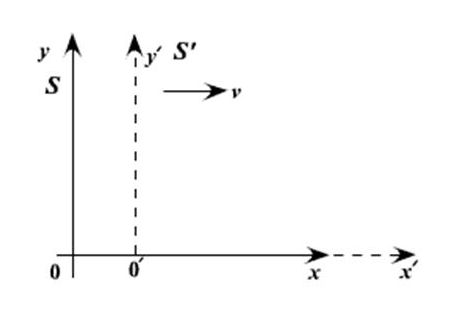
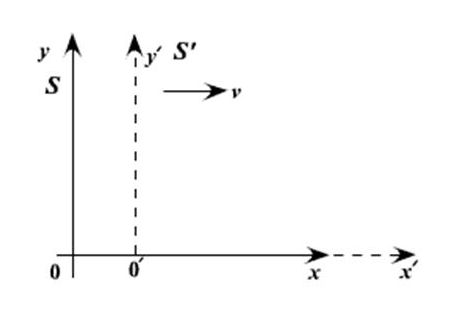
Notice we also throw in a clock with each frame. Suppose S′ is moving relative to S at speed v along the x-axis. For convenience, let us label the moment when O′ passes O as the zero point of timekeeping. That mean at t=0 O and O’ coincide with each other.
Now let us try to find the coordinates of the event (x, y, z, t) in S′
It’s easy to see t′ = t
We synchronized the clocks when O′ passed O. Also, evidently, y′ = y and z′ = z, from the figure.
We can also see that x = x′ +vt.
Thus (x, y, z, t) in S corresponds to (x′, y′, z′, t′) in S′, where
x = x′ +vt.
y = y’
z = z’
t =t’
That’s how positions transform; these are known as the Galilean Transformations.
Velocity
The velocity in S′ in the x′ direction is given by u’x
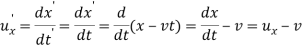
This is obvious anyway it’s just the addition of velocities formula.
ux = u’x +v
Acceleration
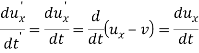
Since v is constant. That is to say,
a’x =ax
The acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.
Q8) Explain construction, working and conclusion of Michelson Morley experiment?
A8)
The experiment was devised to determine the absolute velocity of earth with respect to ether frame. The simplified plan of the experiment is shown in figure.
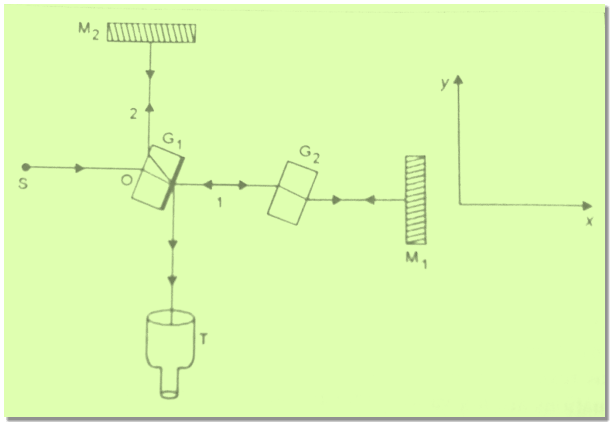
Figure: Michelson Morley Experiment
Construction
Light from a source S falls on a glass plate G1 inclined at an angle of 45° to the incident beam. The back surface of the plate G1 is half silvered. This plate divides the incident beam into two beams 1 and 2 of equal intensity. The beam 1 (transmitted by the plate G1) goes straight to another glass plate G2 of the same thickness as G1 and inclined at an angle of 45° to the beam. This beam after crossing G2 falls normally on the plate mirror M1 and is reflected back towards glass plate G1. On reaching the back surface of G1 it again suffers 50% reflection. The reflected part enters the telescope T.
The beam 2 (reflected by the glass plate G1) moves towards the mirror M2, to fall normally on it. It retraces its path after reflection from M2. When it reaches the back surface of G1, it suffers 50% transmission. The transmitted part goes into the telescope and interferes with the reflected part of the beam 1. Interference fringes are therefore observed in the telescope.
Since the two glass plates G1 and G2 are identical then the path of the two beams are equal in glass. Each beam passes three times through a glass plate in its journey from the source to the telescope. If the mirrors M1 and M2 are at the same distance from 0, then the optical path lengths of two beams shall be equal say l.
Working
We know that the earth moves around the sun with an orbital velocity of Since the earth is moving through ether with velocity v it experiences an ether wind of velocity  . Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
. Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
Time for round trip of light beam 1 travelling in x direction.
When the beam 1 transmitted by G1 travels along the positive x direction from 0 to M1, its effective velocity v1 in earth’s frame is
v1 = c - v
Because the ether wind is opposed to the direction of motion of the beam. The time taken by the light to travel from 0 to M1 is
t1 =  =
= 
In the return journey from M1 to 0 the ether wind is supporting the beam of light. Therefore its effective velocity v2 in earth's frame is
v2 = c + v
The time taken by the light for its return journey M1O is
t2 =  =
= 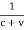
Thus the time of the round trip OM1O is
T1 = t1 + t2
T1 = 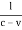 +
+ 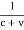 +
+ 
T1 = 2lc (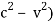 – 1 =
– 1 = 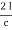 (1 -
(1 -  ) – 1
) – 1
Since  =
= 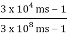 = 10-4 << 1
= 10-4 << 1
We can expand the above expression by Binomial theorem. This gives
T1 = 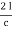 (1 -
(1 - 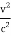 )
)
Time for round trip of light beam 2 travelling in y direction.
The beam 2 travels from O to the mirror M2 parallel to y direction. Since it to moving parallel to y direction its effective velocity must be in the y direction.
Suppose  is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector
is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector 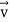 . Therefore the actual velocity of beam 2 should be in OA direction. From figure.
. Therefore the actual velocity of beam 2 should be in OA direction. From figure.
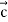 +
+ 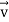 =
= 
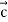 =
= 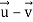
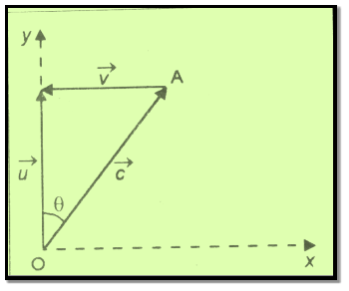
The square of magnitude of 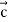 is
is
c2 = u2 + v2 – 2 u v cos 90°
c2 = u2 + v2
Or u2 = c2 - v2
u = 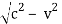
The effective velocity u of the beam is the same during its return journey from M2 to O. Thus time taken to travel from M2 to O is
t2' = t1' = 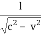
Thus the time of round trip for the beam 2 is
T2 = 2t1' = 
T2 = 2l (c2 – v2)-1/2 =  (1 -
(1 -  )-1/2
)-1/2
Since  << 1 we can expand by Binomial theorem. This gives
<< 1 we can expand by Binomial theorem. This gives
T2 =  ( 1 +
( 1 + 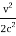 )
)
Expected Fringe shift
The time difference between the two beams for their round trips is
T = T1 – T2  ( 1 +
( 1 + 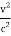 ) -
) -  ( 1 +
( 1 + 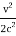 )
)
T= 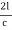 [( 1 +
[( 1 +  - 1 -
- 1 - 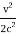 )]
)]
T= 
Thus the optical path difference  between two beams corresponding to the time difference T is
between two beams corresponding to the time difference T is
 cT =
cT =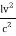
Now suppose this whole apparatus is turned through 90° so that the two beams interchange their paths. Thus path OM2, becomes parallel to the incident beam and OM1 becomes perpendicular to the incident beam. Then the two times T1 and T2 will interchange and the path difference between two beams changes from 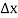 to -
to - 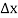 . Thus the path difference between two beams in the second position is
. Thus the path difference between two beams in the second position is
 = -
= - 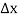 = -
= -
The change in the optical path difference between the two beams due to 90° rotation of the apparatus
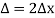 =
= 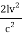
This path difference should lead to the shift of fringes.
Order of Fringe Shift
Suppose λ is the wavelength of light used. We know that a path difference of one wavelength λ corresponds to shift of one fringe across a cross wire in the field of view of telescope. If  n is the number of fringes which shift on turning the apparatus through 90° then
n is the number of fringes which shift on turning the apparatus through 90° then
 n =
n =
In Michelson Morely experiment the distance l= 11 meters,
λ = 5900 Å = 5.9 x 10-7 m
v/c = 10-4. Thus
 n = 0 37
n = 0 37
Thus a fringe shift was expected in this experiment if the concept of the ether frame was valid. In actual experiment no fringe shift was observed.
Conclusion
The negative result of Michelson Morley experiment led to the following conclusion.
The motion of earth through the ether could not to be detected experimentally. Thus the concept of a preferred ether frame must be discarded.
The speed of light in vacuum is same in all frames of reference which are in uniform relative motion.
This experiment paved the way for the development of the special theory of relativity by Einstein in the year 1905.
Q9) Write postulates of special theory of relativity?
A9)
Einstein gave two postulates to explain the negative result of Michelson-Morley experiment. They form the basis of the special theory of relativity. These postulates are:
- The laws of physics take the same form in all inertial frames.
According to first postulate all inertial frames are equivalent. It is meaningless to talk of absolute motion. Only the motion relative to frame of reference has any physical meaning.
- The velocity of light in vacuum has the same value c and is independent of the relative velocity of the source and the observer.
The second postulate simply expresses the result of Michelson Morley experiment.
Q10) Derive Lorentz Transformation equation?
A10)
Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.
The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame.
Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame.
Expressing these relations in Cartesian coordinates gives
x2+y2+z2−c2t2 =0 ………..(1)
x′2+y′2+z′2−c2t′2=0………..(2)
The left-hand sides Equations and can be set equal because both are zero.
Because y=y′ and z=z′ so, we obtain
x2−c2t2 =x′2−c2t′2………..(3)
However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.
To find the correct set of transformation equations, assume the two coordinate systems S and S′
The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified.
Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.
Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis.
The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as,
x’ = Ax +Bt………..(4)
y’ = y………..(5)
z’ = z………..(6)
t’ = Cx +Dt………..(7)
The transformations are linear.
If they are not linear one system would predict acceleration while in other system velocity was even constant.
Because of symmetry, y' and z' axes are left unchanged by the transformation.
Constant can be calculated by using the following boundary conditions.
Observers in S and S' see (O') the origin of S' as
x = vt
x’ = 0
Substituting values of x and x' in equation (4) We get
i = Avt +Bt
i.e. B =- Av………..(8)
Observer in S' and S see the origin (O) of S as
x' = vt
x = 0
Substituting values of x and x' in equation (4) we get
-vt’ =Bt………..(9)
Substituting the value of B from equation (8), we get
-vt’ =- Avt………..(10)
In equation (7) put x=0 we get
t’ = Dt
So equation (10) become
-v Dt =- Avt
A =D ………..(11)
A light pulse is sent out from the origin of S towards x at t=0, its location is given by
x= ct
x’ = ct’
Substituting values of x and x' in equation (4), we get
Ct’ = Act +Bt
Substituting the value of B from equation (8), we get
Ct’ = Act +(- Avt) ………..(12)
Substituting values of x and D from eq no (11) in eq no.(7) , we get
t’ = Cct +At
Substituting above value of t' in eq no.(12) , we get
c(Cct +At) = Act - Avt
Or
Cc2t +Act =Act - Avt
Cc2t =Avt
Or
C =-A  ………..(13)
………..(13)
A light pulse is emitted along y axis in S system at t=0, its coordinate in both systems are
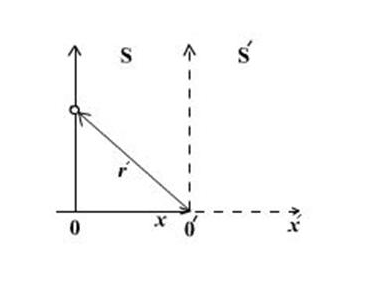
y= ct, x=0
r’=ct’ where
Using equation (5)
r’2 = x’2+y’2
Using equation (5)
y’= y= ct
And from equation (4) and (7)
x’ = Bt = -Avt
t’= Dt =-At
Therefore r’2 = x’2+y’2 become
c2t’2 = (-Avt)2 +(ct)2
Substituting value of t' above equation became
c2A2t2 = A2v2t2 +c2t2
(c2 - v2) A2t2 = c2t2
A2 = 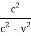
A = 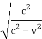
A = 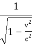 ………..(14)
………..(14)
Substituting the Values of A, B, C, D in equation (1) and (4)
So the Lorentz transformation equations would be,
x’=  -
- 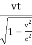
x’= 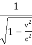 (x-vt)
(x-vt)
x’=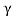 (x-vt)
(x-vt)
Where 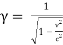
And
t’ = -  +
+ 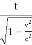
t’=  ( t -
( t -  )
)
Lorentz transformation equations therefore are
x’=  (x - vt)
(x - vt)
y’ =y
z’ = z
t’= 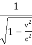 ( t -
( t -  )
)
Or these can be written as
x’=  (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 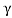 ( t -
( t -  )
)
Where 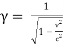
This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.
The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:
x=  (x’+ vt)
(x’+ vt)
y =y’
z = z’
t=  (t’ +
(t’ + 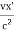 )
)
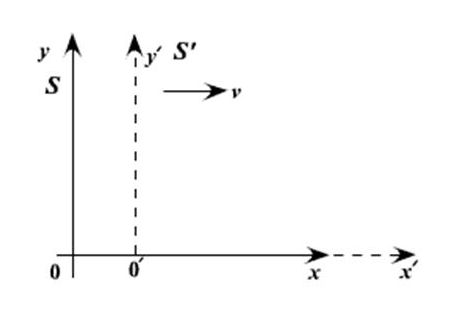
Q11) What do you meant by length contraction?
A11)
Consider a frame S' moving with respect to S with a uniform speed v along x direction figure. Let the rod AB lie along the O'x' axis. The rod is at rest in the frame S' and its length as measured by an observer in S' shall be called the rest length of the rod. To calculate this length the observer in S' will measure the x' coordinates of the two ends A and B. These coordinates shall be independent of the time of observation. If x1' and x2' are the x' coordinates of two ends A and B then the rest length l0 of the rod is
l0 = x'2 – x'1
In the coordinate system S the x coordinates of ends A and B will change with time. In order to measure the length of the rod, the observer in S will have to measure simultaneously the coordinates of the ends A and B. If x1 and x2 are the coordinates of ends A and B at the time of observation t then the length of rod as observed in frame S is
l= x2 – x1

Figure: Length Contraction
From Lorentz equations we have
x'2 = 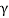 (x2 – v t)
(x2 – v t)
x1' = 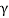 (x1 – v t)
(x1 – v t)
Subtracting these equations, we obtain
x2' - x1' =  (x2 — x1)
(x2 — x1)
Using eq. (6.57) and (6.58) it becomes
l0 = 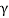 l
l
I = 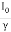
Since 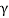 is always greater than one, therefore
is always greater than one, therefore
l < l0
Thus the length of the rod measured from the frame in which it is moving is smaller than its length in a frame in which it is at rest by a factor 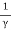 or
or 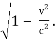 Hence the length of a moving rod appears shortened by a factor
Hence the length of a moving rod appears shortened by a factor 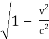
Note the following points
- The length contraction is significant only if the speed of rod approaches the velocity of light.
- The shortening of rod occurs only along the line of motion. The length of meter stick oriented perpendicular to the direction of motion is not changed.
Q12) What do you meant by time dilation?
A12)
Consider a frame S' moving with velocity v with respect to frame S along the x direction Fig (6.6). Suppose the clock is located in the system S' at the point x'. The clock is stationary with respect to S'. Let t1 ' and t2' are two instants of time recorded on this clock by an observer in S'. Then the interval of time as observed in S' is
∆t' = t2' – t1'

Figure: Time dilation
Now we wish to find how this time interval appears to an observer in S. This can be done by applying a Lorentz transformation from S' to S with the same value of x'. The transformation equation required is (6.51 d). From this we can write
t1 =
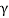 (t1' + v x' / c2)
(t1' + v x' / c2)
t2 = 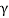 (t'2 + v x' / c2)
(t'2 + v x' / c2)
Subtracting eq. (6.64) from eq. (6.63) we obtain
∆t= t1 – t2 =  (t1' – t2')
(t1' – t2')
Using eq. (6.62) it becomes
∆t= t1 – t2 =  (∆t')
(∆t')
Since  > 1 so
> 1 so
∆t > ∆t'
This means that the time interval as observed in the moving frame of clock is greater than the One observed in the rest frame of the clock. We can express the same thing by saying that a moving clock appears to run slow. We often say that time has been dilated or lengthened in the moving observer frame. The time as observed by an observer moving with the clock is less than the one observed by a moving observer.
We highlight the following points
- Time intervals are affected by relative motion.
- A stationary clock measures a longer time interval between events occurring in a moving frame of reference than does a clock in the moving frame.
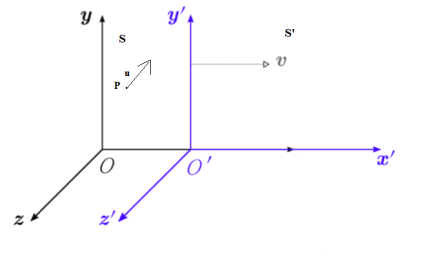
Q13) Discuss velocity addition theorem?
A13)
Let S and S’ be two frames of reference such that S’ is moving with respect to S with a uniform speed v in the positive x direction Figure. Consider a particle P which is moving in the frame S. Suppose its position coordinates at time t are (x,y,z).
Then its velocity components in the frame S are
ux =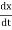 uy =
uy =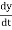 uz =
uz =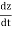 ………….(1)
………….(1)
We wish to find how do these velocity components appear to the observer in the frame S’. If (x’,y’,z’) are the instantaneous position coordinates of the particle P at time t’, then its velocity components in frame S’ are
u’x =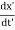 u’y =
u’y =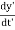 u’z =
u’z =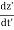 ………….(2)
………….(2)
Consider the Lorentz transformation equations (2) i.e.
x’= 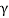 (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 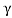 ( t -
( t -  )
)
Where 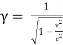
Take the differentials of both sides of above equations. This gives
dx’=  (dx- vdt)………….(3)
(dx- vdt)………….(3)
Dy’ =dy………….(4)
Dz’ = dz………….(5)
Dt’= 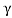 (dt -
(dt - 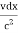 ) ………….(6)
) ………….(6)
Divide equation (3) by (6)
u’x =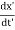 =
= 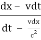
u’x = 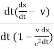
Using equation (1) it becomes
u’x = 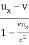
Now divide equation (4) by (6)
u’y =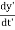 =
= 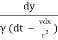
=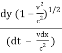
=
Using equation (1) it becomes
u’y =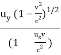
Now divide equation (4) by (6)
u’z = =
=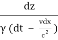
=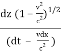
=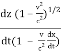
Using equation (1) it becomes
u’z =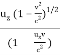
Collecting together equations for u’x ,u’y ,u’z we have
u’x = 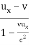 ………….(7)
………….(7)
u’y =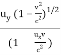 ………….(8)
………….(8)
u’z =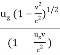 ………….(9)
………….(9)
These equations allow us to observe the observed velocity in frame S and S’ which is in uniform translation motion.
To obtain inverse transformation from S and S’ change dashed quantities to undashed one and vice versa and change v to –v in equation (7), (8) and (9) we get
ux = 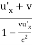 ………….(10)
………….(10)
uy =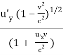 ………….(11)
………….(11)
uz =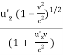 ………….(12)
………….(12)
Q14) Discuss the variation of mass with velocity?
A14)
To explain the variation of mass with velocity, consider two frames of references S and S'. S' is moving with constant velocity v along X-direction.
Let us consider two exactly similar balls A and B each of mass m. Both these balls A and B are moving in opposite direction along X-axis with equal speed u' in frame S'.
After collision they merge into one body.
Applying the law of conservation of momentum on the collision of the balls in frame S', we have
Momentum of balls before collision = Momentum of balls after collision
After collision, the merged mass must be at rest in frame S'. Hence, it moves with velocity v in frame S.
Let u1, u2 be the velocities and m1, m2 be the masses of balls A and B, respectively, in frame S.
Using the law of addition of velocities, the above velocities can be written as
 ………(1)
………(1)
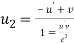 ………(2)
………(2)
Applying the law of conservation of momentum on the collision of the balls in frame s, we have
m1u1 + m2u2 = (m1 + m2)v ………(3)
Substituting u1 and u2 values from Equations (1) and (2), we have
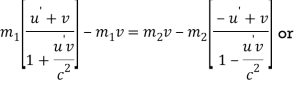

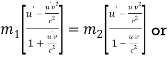
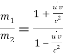 ………(4)
………(4)
The above equation makes a relationship between the masses of balls in frame S and their velocities in frame S'.
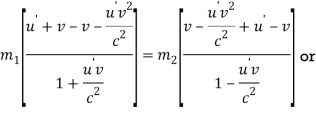
Now, to obtain relation between masses of balls and their velocities in frame S, we proceed as follows. Squaring Equation (1)
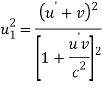
And using the above equation, the value 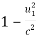 is
is
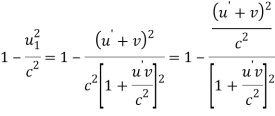
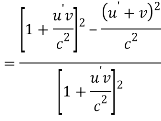
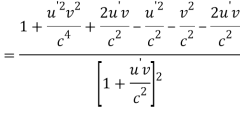
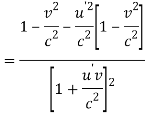
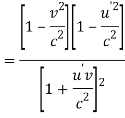
Therefore,
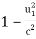 =
= 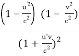 ………(5)
………(5)
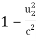 =
= 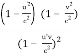 ………(6)
………(6)
Dividing Equation (6) by Equation (5) and taking square root throughout, we have
 =
=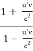 ………(7)
………(7)
Now from equation (4) and (7) we get
 =
= 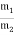 ……… (8)
……… (8)
Suppose, m2 is at rest in frame S, then u2 = 0 and m2 = m0 (say) where m0 is the rest mass of the ball B, then Equation (8) becomes
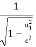 =
= 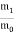 ………(9)
………(9)
As both the balls are similar, hence the rest masses of both balls are the same, so we can write the rest mass of m2 is equal to rest mass of m1, that is equal to mo. Then, Equation (9) becomes
 =
=  ………(10)
………(10)
Here, m1 is the mass of ball A when it is moving with velocity u1 in frame S. After collision, the merged mass containing mass of ball A moves with velocity v in frame S.
In general, if we take the mass of ball A as m, when it is moving with velocity v in frame S, then
 = m ………(11)
= m ………(11)
Where m0 is the rest mass of the body and m is the effective mass.
Equation (11) is the relativistic formula for the variation of mass with velocity. Here, we see some special cases:
Case (i)
When the velocity of the body, v is very small compared to velocity of height, c and then  is negligible compared to one. Therefore,
is negligible compared to one. Therefore,
m = m*0
Case (ii)
If the velocity of the body v is comparable to the velocity of light c, then  is less than one, so m > mo.
is less than one, so m > mo.
The mass of a moving body appears greater than its rest mass.
Case (iii)
Suppose the velocity of a body is equal to velocity of light c, then it possess infinite mass.
The effective mass of particles has been experimentally verified by using particle accelerators in case of electrons and protons by increasing their velocities very close to velocity of light.
Q15) What is Einstein’s mass energy relation?
A15)
Mass-energy equivalence implies that even though the total mass of a system changes, the total energy and momentum remain constant. Consider the collision of an electron and a proton. It destroys the mass of both particles but generates a large amount of energy in the form of photons.
Mass-energy equivalence states that every object possesses certain energy even in a stationary position.
A stationary body does not have kinetic energy. It only possesses potential energy and probable chemical and thermal energy.
According to the field of applied mechanics, the sum of all these energies is smaller than the product of the mass of the object and square of the speed of light.
Mass-energy equivalence means mass and energy are the same and can be converted into each other.
Einstein put this idea forth but he was not the first to bring this into the light. He described the relationship between mass and energy accurately using his theory of relativity. The equation is known as Einstein's mass-energy equation and is expressed as,
E = mc2
Where E= equivalent kinetic energy of the object.
The mass–energy equivalence gives the relation between the mass and energy.
Q16) Derive mass energy equation?
A16)
Derivation of mass energy equation
We know that according to classical mechanics, the rate of change of momentum is proportional to force acting upon the body in the direction of motion.
F = 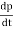 =
=  …...... (1)
…...... (1)
According to the theory of relativity, mass and velocity are variable so equation (1) after become.
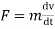 =
= 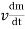 …...... (2)
…...... (2)
The force F acting on the body makes a displacement dx.
We know that work done dw is given by product of force and displacement
And also the work is stored as kinetic energy (dε) in the body. So work done can be written as
Dw = dε = Fdx ...........(3)
Substituting force F from Equation (2) in Equation (3). We get
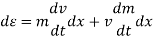

dε = mvdv + v2dm ...........(4)
And also velocity v = dx/dt
The relativistic mass m is given by
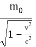 = m
= m
Squaring on both sides, we get
m2 = =
= 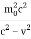
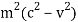 =
= ........... (5)
........... (5)
Differentiating Equation (5), we get
2mc2dm − 2mv2dm − 2m2vdv = 0
c2dm = v2dm + mvdv …........ (6)
Comparing Equations (4) and (6), we get
dε = c2dm ............(7)
By applying force, the mass of a body increases from m0 to m and hence the kinetic energy. The total energy acquired by the body is obtained by integrating Equation (7)
ε = 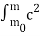 dm =
dm =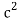
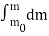 =
=  (m - m0)............ (8)
(m - m0)............ (8)
Therefore, increase in kinetic energy is due to the increase in mass of the body. The total energy (E) of the body is the sum of increase in kinetic energy of the body due to motion and the energy at rest (moc2):
∴ E = ε + moc2
Using Equation (8)
E = mc2 − moc2 + moc2 = mc2
E = mc2 …………. (9)
This shows that increase in energy equivalent to increase in mass. In other words anything that increase energy will increase its relativistic mass.
The above equation shows the equivalence between mass and energy also known as Einstein’s mass–energy relation.
Unit - 3
Theory of relativity
Q1) Calculate the time interval of the signal using Lorentz transformation when a spacecraft S’ is crossed by another spacecraft S at a speed of c/2 on their way to Alpha Centauri. When spacecraft S Crosses S’, the captain of S’ sends a signal that lasts for 1.2s. What is the fine time interval of the single?
A1)
Given:
Δt’ = t‘2−t‘1 = 1.2s
Δx’ = x‘2−x‘1 = 0
The time signal starts and ends at (x’, t‘1)
Using the first Lorentz transformation equation we get,
Δt = t2 – t1
Δx = x2 – x1
Δt=  (
(  t’ +
t’ + 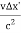 )
)
As the time interval of S’ is fixed, we can consider Δx’ = 0 and the time interval δt becomes:
Δt=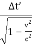
Substituting Δt’ = 1.2s we get
Δt=
Δt = 1.6s
Q2) A certain process requires 10-6 sec to occur in an atom at rest in laboratory. How much time will this process require to an observer in the laboratory? When the atom is moving with speed of 5 x107 m/sec.
A2)
Given Δt = 10-6 sec
v = 5 x109 cm/sec
∆t=  (∆t')
(∆t')
∆t' = ∆t /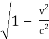
∆t' =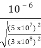 = 1.028 x 10-6 sec
= 1.028 x 10-6 sec
Q3) Compute the contracted length of an object whose initial length 10m and travel with a velocity 0.75c?
A3)
Given parameters are,
L0 = 10m
v = 0.75c
c = Speed of light (3.0 x 108 m/s)
The formula for length contraction is
l0 = 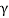 l
l
Or L0 = 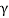 L
L

L = 10 × √1- (0.75)2
L = 10 × √(o.438)
L = 6.618 m.
Q4) A observer on earth sees a rocket zoom by at .95c. If the rocket is measured to be 5.5 m in length, how long is the rocket ship as measure by the astronaut inside the rocket?
A4)
The formula for length contraction is
l0 = 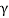 l
l
Or
L0 = 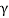 L
L
L = 5.5 m
v = .95c
L0 = ?
5.5 = L0×(1- 0.95c2/c2)
5.5 = L0×(0.312)
L0 = 5.5/(0.312) = 17.6 m
Q5) Difference between inertial & non-inertial frames?
A5)
Inertial frame of reference
An inertial frame is defined as one in which Newton’s law of inertia holds that is anybody which isn’t being acted on by an outside force stays at rest if it is initially at rest, or continues to move at a constant velocity if that’s what it was doing to begin with.
Non-inertial frame of reference
A frame that is accelerated with respect to the assumed inertial frame of reference. Newton’s law will not hold true in these frames.
Q6) The Hypersonic Technology Vehicle 2 (HTV-2) is an experimental rocket vehicle capable of traveling at 21,000 km/h (5830 m/s). If an electronic clock in the HTV-2 measures a time interval of exactly 1-s duration, what would observers on Earth measure the time interval to be?
A6)
Given
Δτ=1s
v=5830m/s.
Δt =?
We know ∆t= t1 – t2 =  (∆t')
(∆t')
Δt=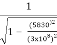 = 1.000000000189s
= 1.000000000189s
Q7) What is Galilean Transformations? How velocity and acceleration transform?
A7) Galilean Coordinate Transformations: For simplicity: - Let coordinates in both references equal at (t = 0). Use Cartesian coordinate systems.
Newton’s laws are only applicable at inertial reference frames. According to Galilean transformations, Newton’s laws are invariant at any inertial reference frame. In other words, the mechanical movement of a particle is exactly the same at two different reference frames, If both of them are inertial (i.e. one moves with constant velocity w.r.t the other & vice versa).
There is no mechanical experiment by which one can distinguish whether a system is at rest or is moving with a constant speed in a straight line (Galilean relativity).
Suppose we know from experiment that these laws of mechanics are true in one frame of reference. How do they look in another frame, moving with respect to the first frame?
To find out, we have to figure out how to get from position, velocity and acceleration in one frame to the corresponding quantities in the second frame.
Obviously, the two frames must have a constant relative velocity, otherwise the law of inertia won’t hold in both of them. Let’s choose the coordinates so that this velocity is along the x-axis of both of them.
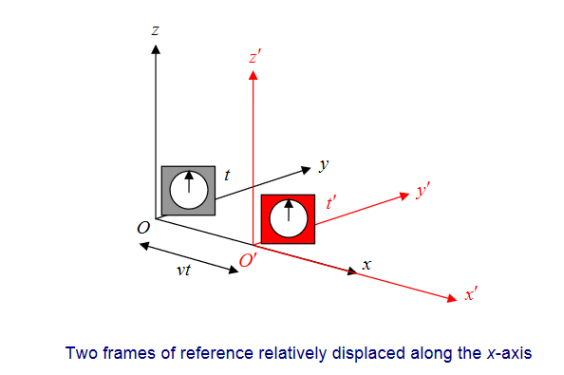
Notice we also throw in a clock with each frame. Suppose S′ is moving relative to S at speed v along the x-axis. For convenience, let us label the moment when O′ passes O as the zero point of timekeeping. That mean at t=0 O and O’ coincide with each other.
Now let us try to find the coordinates of the event (x, y, z, t) in S′
It’s easy to see t′ = t
We synchronized the clocks when O′ passed O. Also, evidently, y′ = y and z′ = z, from the figure.
We can also see that x = x′ +vt.
Thus (x, y, z, t) in S corresponds to (x′, y′, z′, t′) in S′, where
x = x′ +vt.
y = y’
z = z’
t =t’
That’s how positions transform; these are known as the Galilean Transformations.
Velocity
The velocity in S′ in the x′ direction is given by u’x
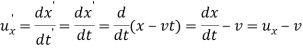
This is obvious anyway it’s just the addition of velocities formula.
ux = u’x +v
Acceleration
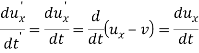
Since v is constant. That is to say,
a’x =ax
The acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.
Q8) Explain construction, working and conclusion of Michelson Morley experiment?
A8)
The experiment was devised to determine the absolute velocity of earth with respect to ether frame. The simplified plan of the experiment is shown in figure.
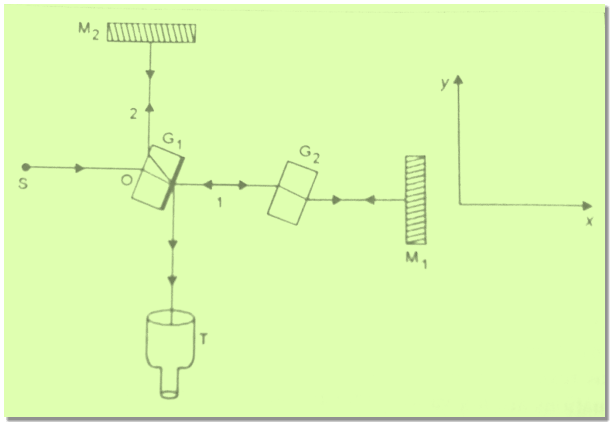
Figure: Michelson Morley Experiment
Construction
Light from a source S falls on a glass plate G1 inclined at an angle of 45° to the incident beam. The back surface of the plate G1 is half silvered. This plate divides the incident beam into two beams 1 and 2 of equal intensity. The beam 1 (transmitted by the plate G1) goes straight to another glass plate G2 of the same thickness as G1 and inclined at an angle of 45° to the beam. This beam after crossing G2 falls normally on the plate mirror M1 and is reflected back towards glass plate G1. On reaching the back surface of G1 it again suffers 50% reflection. The reflected part enters the telescope T.
The beam 2 (reflected by the glass plate G1) moves towards the mirror M2, to fall normally on it. It retraces its path after reflection from M2. When it reaches the back surface of G1, it suffers 50% transmission. The transmitted part goes into the telescope and interferes with the reflected part of the beam 1. Interference fringes are therefore observed in the telescope.
Since the two glass plates G1 and G2 are identical then the path of the two beams are equal in glass. Each beam passes three times through a glass plate in its journey from the source to the telescope. If the mirrors M1 and M2 are at the same distance from 0, then the optical path lengths of two beams shall be equal say l.
Working
We know that the earth moves around the sun with an orbital velocity of Since the earth is moving through ether with velocity v it experiences an ether wind of velocity 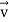 . Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
. Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
Time for round trip of light beam 1 travelling in x direction.
When the beam 1 transmitted by G1 travels along the positive x direction from 0 to M1, its effective velocity v1 in earth’s frame is
v1 = c - v
Because the ether wind is opposed to the direction of motion of the beam. The time taken by the light to travel from 0 to M1 is
t1 =  =
= 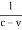
In the return journey from M1 to 0 the ether wind is supporting the beam of light. Therefore its effective velocity v2 in earth's frame is
v2 = c + v
The time taken by the light for its return journey M1O is
t2 =  =
= 
Thus the time of the round trip OM1O is
T1 = t1 + t2
T1 =  +
+  +
+ 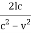
T1 = 2lc (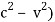 – 1 =
– 1 = 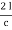 (1 -
(1 -  ) – 1
) – 1
Since  =
= 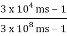 = 10-4 << 1
= 10-4 << 1
We can expand the above expression by Binomial theorem. This gives
T1 = 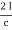 (1 -
(1 - 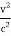 )
)
Time for round trip of light beam 2 travelling in y direction.
The beam 2 travels from O to the mirror M2 parallel to y direction. Since it to moving parallel to y direction its effective velocity must be in the y direction.
Suppose 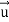 is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector
is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector 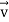 . Therefore the actual velocity of beam 2 should be in OA direction. From figure.
. Therefore the actual velocity of beam 2 should be in OA direction. From figure.
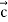 +
+ 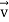 =
= 
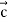 =
= 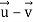

The square of magnitude of 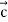 is
is
c2 = u2 + v2 – 2 u v cos 90°
c2 = u2 + v2
Or u2 = c2 - v2
u = 
The effective velocity u of the beam is the same during its return journey from M2 to O. Thus time taken to travel from M2 to O is
t2' = t1' = 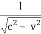
Thus the time of round trip for the beam 2 is
T2 = 2t1' = 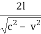
T2 = 2l (c2 – v2)-1/2 = 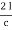 (1 -
(1 -  )-1/2
)-1/2
Since  << 1 we can expand by Binomial theorem. This gives
<< 1 we can expand by Binomial theorem. This gives
T2 =  ( 1 +
( 1 + 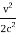 )
)
Expected Fringe shift
The time difference between the two beams for their round trips is
T = T1 – T2  ( 1 +
( 1 + 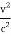 ) -
) - 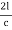 ( 1 +
( 1 + 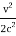 )
)
T=  [( 1 +
[( 1 + 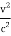 - 1 -
- 1 - 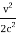 )]
)]
T= 
Thus the optical path difference 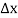 between two beams corresponding to the time difference T is
between two beams corresponding to the time difference T is
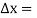 cT =
cT =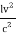
Now suppose this whole apparatus is turned through 90° so that the two beams interchange their paths. Thus path OM2, becomes parallel to the incident beam and OM1 becomes perpendicular to the incident beam. Then the two times T1 and T2 will interchange and the path difference between two beams changes from 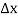 to -
to - 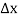 . Thus the path difference between two beams in the second position is
. Thus the path difference between two beams in the second position is
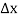 = -
= - 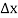 = -
= -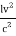
The change in the optical path difference between the two beams due to 90° rotation of the apparatus
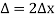 =
= 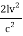
This path difference should lead to the shift of fringes.
Order of Fringe Shift
Suppose λ is the wavelength of light used. We know that a path difference of one wavelength λ corresponds to shift of one fringe across a cross wire in the field of view of telescope. If  n is the number of fringes which shift on turning the apparatus through 90° then
n is the number of fringes which shift on turning the apparatus through 90° then
 n =
n =
In Michelson Morely experiment the distance l= 11 meters,
λ = 5900 Å = 5.9 x 10-7 m
v/c = 10-4. Thus
 n = 0 37
n = 0 37
Thus a fringe shift was expected in this experiment if the concept of the ether frame was valid. In actual experiment no fringe shift was observed.
Conclusion
The negative result of Michelson Morley experiment led to the following conclusion.
The motion of earth through the ether could not to be detected experimentally. Thus the concept of a preferred ether frame must be discarded.
The speed of light in vacuum is same in all frames of reference which are in uniform relative motion.
This experiment paved the way for the development of the special theory of relativity by Einstein in the year 1905.
Q9) Write postulates of special theory of relativity?
A9)
Einstein gave two postulates to explain the negative result of Michelson-Morley experiment. They form the basis of the special theory of relativity. These postulates are:
- The laws of physics take the same form in all inertial frames.
According to first postulate all inertial frames are equivalent. It is meaningless to talk of absolute motion. Only the motion relative to frame of reference has any physical meaning.
- The velocity of light in vacuum has the same value c and is independent of the relative velocity of the source and the observer.
The second postulate simply expresses the result of Michelson Morley experiment.
Q10) Derive Lorentz Transformation equation?
A10)
Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.
The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame.
Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame.
Expressing these relations in Cartesian coordinates gives
x2+y2+z2−c2t2 =0 ………..(1)
x′2+y′2+z′2−c2t′2=0………..(2)
The left-hand sides Equations and can be set equal because both are zero.
Because y=y′ and z=z′ so, we obtain
x2−c2t2 =x′2−c2t′2………..(3)
However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.
To find the correct set of transformation equations, assume the two coordinate systems S and S′
The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified.
Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.
Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis.
The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as,
x’ = Ax +Bt………..(4)
y’ = y………..(5)
z’ = z………..(6)
t’ = Cx +Dt………..(7)
The transformations are linear.
If they are not linear one system would predict acceleration while in other system velocity was even constant.
Because of symmetry, y' and z' axes are left unchanged by the transformation.
Constant can be calculated by using the following boundary conditions.
Observers in S and S' see (O') the origin of S' as
x = vt
x’ = 0
Substituting values of x and x' in equation (4) We get
i = Avt +Bt
i.e. B =- Av………..(8)
Observer in S' and S see the origin (O) of S as
x' = vt
x = 0
Substituting values of x and x' in equation (4) we get
-vt’ =Bt………..(9)
Substituting the value of B from equation (8), we get
-vt’ =- Avt………..(10)
In equation (7) put x=0 we get
t’ = Dt
So equation (10) become
-v Dt =- Avt
A =D ………..(11)
A light pulse is sent out from the origin of S towards x at t=0, its location is given by
x= ct
x’ = ct’
Substituting values of x and x' in equation (4), we get
Ct’ = Act +Bt
Substituting the value of B from equation (8), we get
Ct’ = Act +(- Avt) ………..(12)
Substituting values of x and D from eq no (11) in eq no.(7) , we get
t’ = Cct +At
Substituting above value of t' in eq no.(12) , we get
c(Cct +At) = Act - Avt
Or
Cc2t +Act =Act - Avt
Cc2t =Avt
Or
C =-A  ………..(13)
………..(13)
A light pulse is emitted along y axis in S system at t=0, its coordinate in both systems are
y= ct, x=0
r’=ct’ where
Using equation (5)
r’2 = x’2+y’2
Using equation (5)
y’= y= ct
And from equation (4) and (7)
x’ = Bt = -Avt
t’= Dt =-At
Therefore r’2 = x’2+y’2 become
c2t’2 = (-Avt)2 +(ct)2
Substituting value of t' above equation became
c2A2t2 = A2v2t2 +c2t2
(c2 - v2) A2t2 = c2t2
A2 = 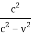
A = 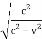
A = 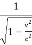 ………..(14)
………..(14)
Substituting the Values of A, B, C, D in equation (1) and (4)
So the Lorentz transformation equations would be,
x’= 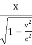 -
- 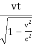
x’= 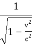 (x-vt)
(x-vt)
x’= (x-vt)
(x-vt)
Where 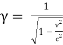
And
t’ = -  +
+ 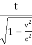
t’= 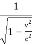 ( t -
( t -  )
)
Lorentz transformation equations therefore are
x’= 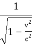 (x - vt)
(x - vt)
y’ =y
z’ = z
t’=  ( t -
( t -  )
)
Or these can be written as
x’= 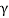 (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 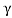 ( t -
( t -  )
)
Where 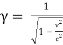
This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.
The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:
x= 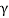 (x’+ vt)
(x’+ vt)
y =y’
z = z’
t=  (t’ +
(t’ + 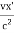 )
)
Q11) What do you meant by length contraction?
A11)
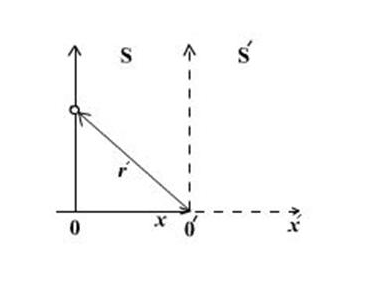
Consider a frame S' moving with respect to S with a uniform speed v along x direction figure. Let the rod AB lie along the O'x' axis. The rod is at rest in the frame S' and its length as measured by an observer in S' shall be called the rest length of the rod. To calculate this length the observer in S' will measure the x' coordinates of the two ends A and B. These coordinates shall be independent of the time of observation. If x1' and x2' are the x' coordinates of two ends A and B then the rest length l0 of the rod is
l0 = x'2 – x'1
In the coordinate system S the x coordinates of ends A and B will change with time. In order to measure the length of the rod, the observer in S will have to measure simultaneously the coordinates of the ends A and B. If x1 and x2 are the coordinates of ends A and B at the time of observation t then the length of rod as observed in frame S is
l= x2 – x1

Figure: Length Contraction
From Lorentz equations we have
x'2 = 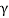 (x2 – v t)
(x2 – v t)
x1' = 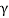 (x1 – v t)
(x1 – v t)
Subtracting these equations, we obtain
x2' - x1' = 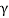 (x2 — x1)
(x2 — x1)
Using eq. (6.57) and (6.58) it becomes
l0 =  l
l
I = 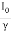
Since  is always greater than one, therefore
is always greater than one, therefore
l < l0
Thus the length of the rod measured from the frame in which it is moving is smaller than its length in a frame in which it is at rest by a factor  or
or 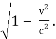 Hence the length of a moving rod appears shortened by a factor
Hence the length of a moving rod appears shortened by a factor 
Note the following points
- The length contraction is significant only if the speed of rod approaches the velocity of light.
- The shortening of rod occurs only along the line of motion. The length of meter stick oriented perpendicular to the direction of motion is not changed.
Q12) What do you meant by time dilation?
A12)
Consider a frame S' moving with velocity v with respect to frame S along the x direction Fig (6.6). Suppose the clock is located in the system S' at the point x'. The clock is stationary with respect to S'. Let t1 ' and t2' are two instants of time recorded on this clock by an observer in S'. Then the interval of time as observed in S' is
∆t' = t2' – t1'
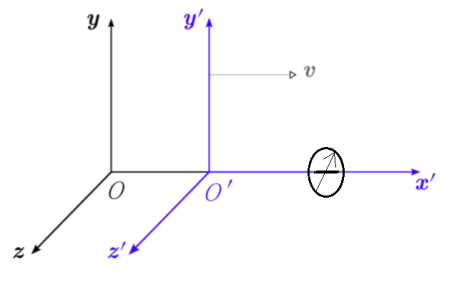
Figure: Time dilation
Now we wish to find how this time interval appears to an observer in S. This can be done by applying a Lorentz transformation from S' to S with the same value of x'. The transformation equation required is (6.51 d). From this we can write
t1 =
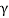 (t1' + v x' / c2)
(t1' + v x' / c2)
t2 = 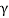 (t'2 + v x' / c2)
(t'2 + v x' / c2)
Subtracting eq. (6.64) from eq. (6.63) we obtain
∆t= t1 – t2 = 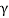 (t1' – t2')
(t1' – t2')
Using eq. (6.62) it becomes
∆t= t1 – t2 = 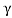 (∆t')
(∆t')
Since 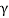 > 1 so
> 1 so
∆t > ∆t'
This means that the time interval as observed in the moving frame of clock is greater than the One observed in the rest frame of the clock. We can express the same thing by saying that a moving clock appears to run slow. We often say that time has been dilated or lengthened in the moving observer frame. The time as observed by an observer moving with the clock is less than the one observed by a moving observer.
We highlight the following points
- Time intervals are affected by relative motion.
- A stationary clock measures a longer time interval between events occurring in a moving frame of reference than does a clock in the moving frame.
Q13) Discuss velocity addition theorem?
A13)
Let S and S’ be two frames of reference such that S’ is moving with respect to S with a uniform speed v in the positive x direction Figure. Consider a particle P which is moving in the frame S. Suppose its position coordinates at time t are (x,y,z).
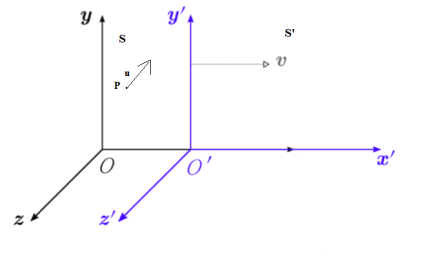
Then its velocity components in the frame S are
ux =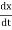 uy =
uy =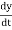 uz =
uz = ………….(1)
………….(1)
We wish to find how do these velocity components appear to the observer in the frame S’. If (x’,y’,z’) are the instantaneous position coordinates of the particle P at time t’, then its velocity components in frame S’ are
u’x =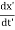 u’y =
u’y =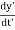 u’z =
u’z =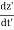 ………….(2)
………….(2)
Consider the Lorentz transformation equations (2) i.e.
x’= 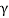 (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 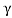 ( t -
( t -  )
)
Where 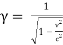
Take the differentials of both sides of above equations. This gives
dx’= 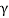 (dx- vdt)………….(3)
(dx- vdt)………….(3)
Dy’ =dy………….(4)
Dz’ = dz………….(5)
Dt’= 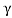 (dt -
(dt -  ) ………….(6)
) ………….(6)
Divide equation (3) by (6)
u’x =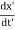 =
= 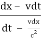
u’x = 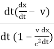
Using equation (1) it becomes
u’x = 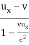
Now divide equation (4) by (6)
u’y = =
= 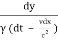
=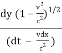
=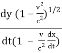
Using equation (1) it becomes
u’y =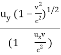
Now divide equation (4) by (6)
u’z =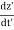 =
=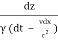
=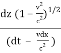
=
Using equation (1) it becomes
u’z =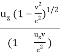
Collecting together equations for u’x ,u’y ,u’z we have
u’x =  ………….(7)
………….(7)
u’y =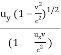 ………….(8)
………….(8)
u’z =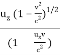 ………….(9)
………….(9)
These equations allow us to observe the observed velocity in frame S and S’ which is in uniform translation motion.
To obtain inverse transformation from S and S’ change dashed quantities to undashed one and vice versa and change v to –v in equation (7), (8) and (9) we get
ux = 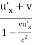 ………….(10)
………….(10)
uy = ………….(11)
………….(11)
uz =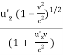 ………….(12)
………….(12)
Q14) Discuss the variation of mass with velocity?
A14)
To explain the variation of mass with velocity, consider two frames of references S and S'. S' is moving with constant velocity v along X-direction.
Let us consider two exactly similar balls A and B each of mass m. Both these balls A and B are moving in opposite direction along X-axis with equal speed u' in frame S'.
After collision they merge into one body.
Applying the law of conservation of momentum on the collision of the balls in frame S', we have
Momentum of balls before collision = Momentum of balls after collision
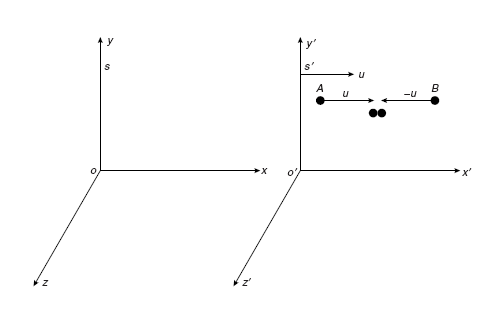
After collision, the merged mass must be at rest in frame S'. Hence, it moves with velocity v in frame S.
Let u1, u2 be the velocities and m1, m2 be the masses of balls A and B, respectively, in frame S.
Using the law of addition of velocities, the above velocities can be written as
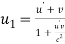 ………(1)
………(1)
 ………(2)
………(2)
Applying the law of conservation of momentum on the collision of the balls in frame s, we have
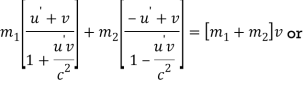
m1u1 + m2u2 = (m1 + m2)v ………(3)
Substituting u1 and u2 values from Equations (1) and (2), we have
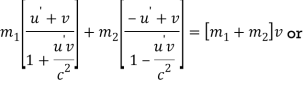
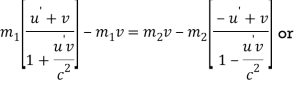
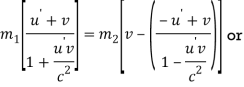

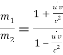 ………(4)
………(4)
The above equation makes a relationship between the masses of balls in frame S and their velocities in frame S'.
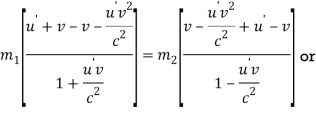
Now, to obtain relation between masses of balls and their velocities in frame S, we proceed as follows. Squaring Equation (1)

And using the above equation, the value 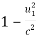 is
is
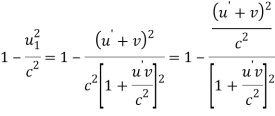
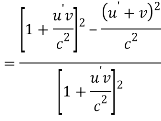
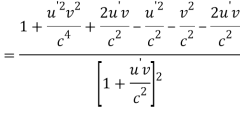

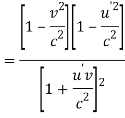
Therefore,
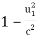 =
= 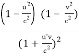 ………(5)
………(5)
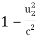 =
=  ………(6)
………(6)
Dividing Equation (6) by Equation (5) and taking square root throughout, we have
 =
=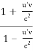 ………(7)
………(7)
Now from equation (4) and (7) we get
 =
= 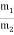 ……… (8)
……… (8)
Suppose, m2 is at rest in frame S, then u2 = 0 and m2 = m0 (say) where m0 is the rest mass of the ball B, then Equation (8) becomes
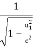 =
= 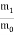 ………(9)
………(9)
As both the balls are similar, hence the rest masses of both balls are the same, so we can write the rest mass of m2 is equal to rest mass of m1, that is equal to mo. Then, Equation (9) becomes
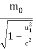 =
=  ………(10)
………(10)
Here, m1 is the mass of ball A when it is moving with velocity u1 in frame S. After collision, the merged mass containing mass of ball A moves with velocity v in frame S.
In general, if we take the mass of ball A as m, when it is moving with velocity v in frame S, then
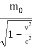 = m ………(11)
= m ………(11)
Where m0 is the rest mass of the body and m is the effective mass.
Equation (11) is the relativistic formula for the variation of mass with velocity. Here, we see some special cases:
Case (i)
When the velocity of the body, v is very small compared to velocity of height, c and then 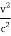 is negligible compared to one. Therefore,
is negligible compared to one. Therefore,
m = m*0
Case (ii)
If the velocity of the body v is comparable to the velocity of light c, then  is less than one, so m > mo.
is less than one, so m > mo.
The mass of a moving body appears greater than its rest mass.
Case (iii)
Suppose the velocity of a body is equal to velocity of light c, then it possess infinite mass.
The effective mass of particles has been experimentally verified by using particle accelerators in case of electrons and protons by increasing their velocities very close to velocity of light.
Q15) What is Einstein’s mass energy relation?
A15)
Mass-energy equivalence implies that even though the total mass of a system changes, the total energy and momentum remain constant. Consider the collision of an electron and a proton. It destroys the mass of both particles but generates a large amount of energy in the form of photons.
Mass-energy equivalence states that every object possesses certain energy even in a stationary position.
A stationary body does not have kinetic energy. It only possesses potential energy and probable chemical and thermal energy.
According to the field of applied mechanics, the sum of all these energies is smaller than the product of the mass of the object and square of the speed of light.
Mass-energy equivalence means mass and energy are the same and can be converted into each other.
Einstein put this idea forth but he was not the first to bring this into the light. He described the relationship between mass and energy accurately using his theory of relativity. The equation is known as Einstein's mass-energy equation and is expressed as,
E = mc2
Where E= equivalent kinetic energy of the object.
The mass–energy equivalence gives the relation between the mass and energy.
Q16) Derive mass energy equation?
A16)
Derivation of mass energy equation
We know that according to classical mechanics, the rate of change of momentum is proportional to force acting upon the body in the direction of motion.
F =  =
= 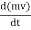 …...... (1)
…...... (1)
According to the theory of relativity, mass and velocity are variable so equation (1) after become.
 =
=  …...... (2)
…...... (2)
The force F acting on the body makes a displacement dx.
We know that work done dw is given by product of force and displacement
And also the work is stored as kinetic energy (dε) in the body. So work done can be written as
Dw = dε = Fdx ...........(3)
Substituting force F from Equation (2) in Equation (3). We get
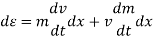
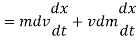
dε = mvdv + v2dm ...........(4)
And also velocity v = dx/dt
The relativistic mass m is given by
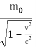 = m
= m
Squaring on both sides, we get
m2 =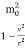 =
= 
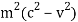 =
= ........... (5)
........... (5)
Differentiating Equation (5), we get
2mc2dm − 2mv2dm − 2m2vdv = 0
c2dm = v2dm + mvdv …........ (6)
Comparing Equations (4) and (6), we get
dε = c2dm ............(7)
By applying force, the mass of a body increases from m0 to m and hence the kinetic energy. The total energy acquired by the body is obtained by integrating Equation (7)
ε = 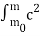 dm =
dm =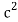
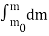 =
= 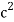 (m - m0)............ (8)
(m - m0)............ (8)
Therefore, increase in kinetic energy is due to the increase in mass of the body. The total energy (E) of the body is the sum of increase in kinetic energy of the body due to motion and the energy at rest (moc2):
∴ E = ε + moc2
Using Equation (8)
E = mc2 − moc2 + moc2 = mc2
E = mc2 …………. (9)
This shows that increase in energy equivalent to increase in mass. In other words anything that increase energy will increase its relativistic mass.
The above equation shows the equivalence between mass and energy also known as Einstein’s mass–energy relation.
Unit - 3
Unit - 3
Theory of relativity
Q1) Calculate the time interval of the signal using Lorentz transformation when a spacecraft S’ is crossed by another spacecraft S at a speed of c/2 on their way to Alpha Centauri. When spacecraft S Crosses S’, the captain of S’ sends a signal that lasts for 1.2s. What is the fine time interval of the single?
A1)
Given:
Δt’ = t‘2−t‘1 = 1.2s
Δx’ = x‘2−x‘1 = 0
The time signal starts and ends at (x’, t‘1)
Using the first Lorentz transformation equation we get,
Δt = t2 – t1
Δx = x2 – x1
Δt= 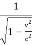 (
( 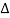 t’ +
t’ +  )
)
As the time interval of S’ is fixed, we can consider Δx’ = 0 and the time interval δt becomes:
Δt=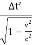
Substituting Δt’ = 1.2s we get
Δt=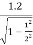
Δt = 1.6s
Q2) A certain process requires 10-6 sec to occur in an atom at rest in laboratory. How much time will this process require to an observer in the laboratory? When the atom is moving with speed of 5 x107 m/sec.
A2)
Given Δt = 10-6 sec
v = 5 x109 cm/sec
∆t=  (∆t')
(∆t')
∆t' = ∆t /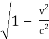
∆t' =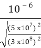 = 1.028 x 10-6 sec
= 1.028 x 10-6 sec
Q3) Compute the contracted length of an object whose initial length 10m and travel with a velocity 0.75c?
A3)
Given parameters are,
L0 = 10m
v = 0.75c
c = Speed of light (3.0 x 108 m/s)
The formula for length contraction is
l0 = 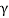 l
l
Or L0 =  L
L

L = 10 × √1- (0.75)2
L = 10 × √(o.438)
L = 6.618 m.
Q4) A observer on earth sees a rocket zoom by at .95c. If the rocket is measured to be 5.5 m in length, how long is the rocket ship as measure by the astronaut inside the rocket?
A4)
The formula for length contraction is
l0 =  l
l
Or
L0 =  L
L
L = 5.5 m
v = .95c
L0 = ?
5.5 = L0×(1- 0.95c2/c2)
5.5 = L0×(0.312)
L0 = 5.5/(0.312) = 17.6 m
Q5) Difference between inertial & non-inertial frames?
A5)
Inertial frame of reference
An inertial frame is defined as one in which Newton’s law of inertia holds that is anybody which isn’t being acted on by an outside force stays at rest if it is initially at rest, or continues to move at a constant velocity if that’s what it was doing to begin with.
Non-inertial frame of reference
A frame that is accelerated with respect to the assumed inertial frame of reference. Newton’s law will not hold true in these frames.
Q6) The Hypersonic Technology Vehicle 2 (HTV-2) is an experimental rocket vehicle capable of traveling at 21,000 km/h (5830 m/s). If an electronic clock in the HTV-2 measures a time interval of exactly 1-s duration, what would observers on Earth measure the time interval to be?
A6)
Given
Δτ=1s
v=5830m/s.
Δt =?
We know ∆t= t1 – t2 = 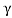 (∆t')
(∆t')
Δt=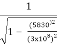 = 1.000000000189s
= 1.000000000189s
Q7) What is Galilean Transformations? How velocity and acceleration transform?
A7) Galilean Coordinate Transformations: For simplicity: - Let coordinates in both references equal at (t = 0). Use Cartesian coordinate systems.
Newton’s laws are only applicable at inertial reference frames. According to Galilean transformations, Newton’s laws are invariant at any inertial reference frame. In other words, the mechanical movement of a particle is exactly the same at two different reference frames, If both of them are inertial (i.e. one moves with constant velocity w.r.t the other & vice versa).
There is no mechanical experiment by which one can distinguish whether a system is at rest or is moving with a constant speed in a straight line (Galilean relativity).
Suppose we know from experiment that these laws of mechanics are true in one frame of reference. How do they look in another frame, moving with respect to the first frame?
To find out, we have to figure out how to get from position, velocity and acceleration in one frame to the corresponding quantities in the second frame.
Obviously, the two frames must have a constant relative velocity, otherwise the law of inertia won’t hold in both of them. Let’s choose the coordinates so that this velocity is along the x-axis of both of them.
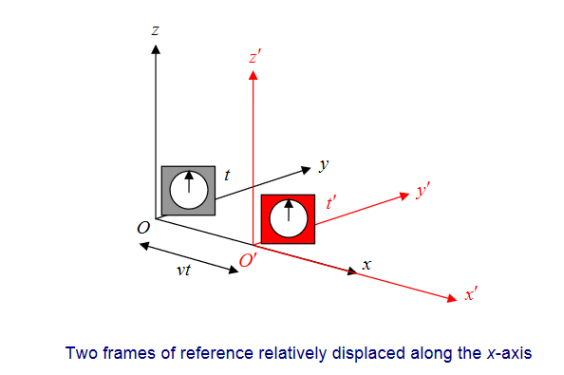
Notice we also throw in a clock with each frame. Suppose S′ is moving relative to S at speed v along the x-axis. For convenience, let us label the moment when O′ passes O as the zero point of timekeeping. That mean at t=0 O and O’ coincide with each other.
Now let us try to find the coordinates of the event (x, y, z, t) in S′
It’s easy to see t′ = t
We synchronized the clocks when O′ passed O. Also, evidently, y′ = y and z′ = z, from the figure.
We can also see that x = x′ +vt.
Thus (x, y, z, t) in S corresponds to (x′, y′, z′, t′) in S′, where
x = x′ +vt.
y = y’
z = z’
t =t’
That’s how positions transform; these are known as the Galilean Transformations.
Velocity
The velocity in S′ in the x′ direction is given by u’x
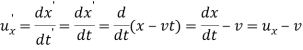
This is obvious anyway it’s just the addition of velocities formula.
ux = u’x +v
Acceleration
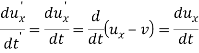
Since v is constant. That is to say,
a’x =ax
The acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.
Q8) Explain construction, working and conclusion of Michelson Morley experiment?
A8)
The experiment was devised to determine the absolute velocity of earth with respect to ether frame. The simplified plan of the experiment is shown in figure.
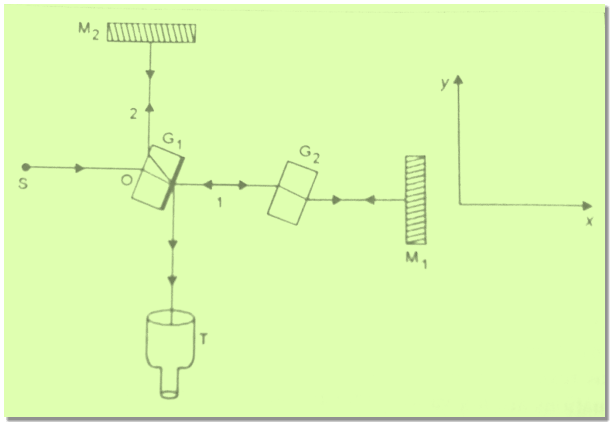
Figure: Michelson Morley Experiment
Construction
Light from a source S falls on a glass plate G1 inclined at an angle of 45° to the incident beam. The back surface of the plate G1 is half silvered. This plate divides the incident beam into two beams 1 and 2 of equal intensity. The beam 1 (transmitted by the plate G1) goes straight to another glass plate G2 of the same thickness as G1 and inclined at an angle of 45° to the beam. This beam after crossing G2 falls normally on the plate mirror M1 and is reflected back towards glass plate G1. On reaching the back surface of G1 it again suffers 50% reflection. The reflected part enters the telescope T.
The beam 2 (reflected by the glass plate G1) moves towards the mirror M2, to fall normally on it. It retraces its path after reflection from M2. When it reaches the back surface of G1, it suffers 50% transmission. The transmitted part goes into the telescope and interferes with the reflected part of the beam 1. Interference fringes are therefore observed in the telescope.
Since the two glass plates G1 and G2 are identical then the path of the two beams are equal in glass. Each beam passes three times through a glass plate in its journey from the source to the telescope. If the mirrors M1 and M2 are at the same distance from 0, then the optical path lengths of two beams shall be equal say l.
Working
We know that the earth moves around the sun with an orbital velocity of Since the earth is moving through ether with velocity v it experiences an ether wind of velocity 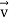 . Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
. Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
Time for round trip of light beam 1 travelling in x direction.
When the beam 1 transmitted by G1 travels along the positive x direction from 0 to M1, its effective velocity v1 in earth’s frame is
v1 = c - v
Because the ether wind is opposed to the direction of motion of the beam. The time taken by the light to travel from 0 to M1 is
t1 =  =
= 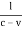
In the return journey from M1 to 0 the ether wind is supporting the beam of light. Therefore its effective velocity v2 in earth's frame is
v2 = c + v
The time taken by the light for its return journey M1O is
t2 =  =
= 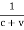
Thus the time of the round trip OM1O is
T1 = t1 + t2
T1 =  +
+ 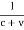 +
+ 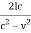
T1 = 2lc (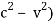 – 1 =
– 1 =  (1 -
(1 - 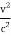 ) – 1
) – 1
Since  =
= 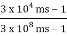 = 10-4 << 1
= 10-4 << 1
We can expand the above expression by Binomial theorem. This gives
T1 = 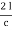 (1 -
(1 - 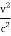 )
)
Time for round trip of light beam 2 travelling in y direction.
The beam 2 travels from O to the mirror M2 parallel to y direction. Since it to moving parallel to y direction its effective velocity must be in the y direction.
Suppose 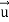 is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector
is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector  . Therefore the actual velocity of beam 2 should be in OA direction. From figure.
. Therefore the actual velocity of beam 2 should be in OA direction. From figure.
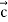 +
+ 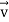 =
= 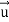
 =
= 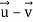
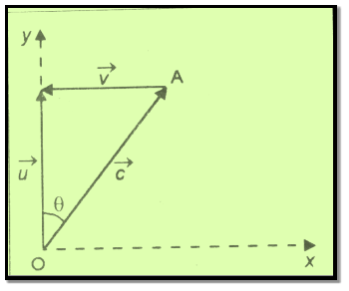
The square of magnitude of 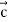 is
is
c2 = u2 + v2 – 2 u v cos 90°
c2 = u2 + v2
Or u2 = c2 - v2
u = 
The effective velocity u of the beam is the same during its return journey from M2 to O. Thus time taken to travel from M2 to O is
t2' = t1' = 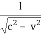
Thus the time of round trip for the beam 2 is
T2 = 2t1' = 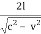
T2 = 2l (c2 – v2)-1/2 = 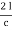 (1 -
(1 - 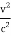 )-1/2
)-1/2
Since  << 1 we can expand by Binomial theorem. This gives
<< 1 we can expand by Binomial theorem. This gives
T2 =  ( 1 +
( 1 +  )
)
Expected Fringe shift
The time difference between the two beams for their round trips is
T = T1 – T2  ( 1 +
( 1 + 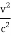 ) -
) - 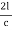 ( 1 +
( 1 + 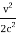 )
)
T= 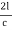 [( 1 +
[( 1 + 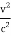 - 1 -
- 1 - 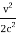 )]
)]
T= 
Thus the optical path difference 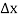 between two beams corresponding to the time difference T is
between two beams corresponding to the time difference T is
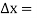 cT =
cT =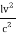
Now suppose this whole apparatus is turned through 90° so that the two beams interchange their paths. Thus path OM2, becomes parallel to the incident beam and OM1 becomes perpendicular to the incident beam. Then the two times T1 and T2 will interchange and the path difference between two beams changes from 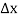 to -
to - 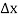 . Thus the path difference between two beams in the second position is
. Thus the path difference between two beams in the second position is
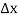 = -
= - 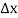 = -
= -
The change in the optical path difference between the two beams due to 90° rotation of the apparatus
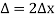 =
= 
This path difference should lead to the shift of fringes.
Order of Fringe Shift
Suppose λ is the wavelength of light used. We know that a path difference of one wavelength λ corresponds to shift of one fringe across a cross wire in the field of view of telescope. If  n is the number of fringes which shift on turning the apparatus through 90° then
n is the number of fringes which shift on turning the apparatus through 90° then
 n =
n =
In Michelson Morely experiment the distance l= 11 meters,
λ = 5900 Å = 5.9 x 10-7 m
v/c = 10-4. Thus
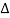 n = 0 37
n = 0 37
Thus a fringe shift was expected in this experiment if the concept of the ether frame was valid. In actual experiment no fringe shift was observed.
Conclusion
The negative result of Michelson Morley experiment led to the following conclusion.
The motion of earth through the ether could not to be detected experimentally. Thus the concept of a preferred ether frame must be discarded.
The speed of light in vacuum is same in all frames of reference which are in uniform relative motion.
This experiment paved the way for the development of the special theory of relativity by Einstein in the year 1905.
Q9) Write postulates of special theory of relativity?
A9)
Einstein gave two postulates to explain the negative result of Michelson-Morley experiment. They form the basis of the special theory of relativity. These postulates are:
- The laws of physics take the same form in all inertial frames.
According to first postulate all inertial frames are equivalent. It is meaningless to talk of absolute motion. Only the motion relative to frame of reference has any physical meaning.
- The velocity of light in vacuum has the same value c and is independent of the relative velocity of the source and the observer.
The second postulate simply expresses the result of Michelson Morley experiment.
Q10) Derive Lorentz Transformation equation?
A10)
Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.
The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame.
Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame.
Expressing these relations in Cartesian coordinates gives
x2+y2+z2−c2t2 =0 ………..(1)
x′2+y′2+z′2−c2t′2=0………..(2)
The left-hand sides Equations and can be set equal because both are zero.
Because y=y′ and z=z′ so, we obtain
x2−c2t2 =x′2−c2t′2………..(3)
However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.
To find the correct set of transformation equations, assume the two coordinate systems S and S′
The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified.
Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.
Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis.
The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as,
x’ = Ax +Bt………..(4)
y’ = y………..(5)
z’ = z………..(6)
t’ = Cx +Dt………..(7)
The transformations are linear.
If they are not linear one system would predict acceleration while in other system velocity was even constant.
Because of symmetry, y' and z' axes are left unchanged by the transformation.
Constant can be calculated by using the following boundary conditions.
Observers in S and S' see (O') the origin of S' as
x = vt
x’ = 0
Substituting values of x and x' in equation (4) We get
i = Avt +Bt
i.e. B =- Av………..(8)
Observer in S' and S see the origin (O) of S as
x' = vt
x = 0
Substituting values of x and x' in equation (4) we get
-vt’ =Bt………..(9)
Substituting the value of B from equation (8), we get
-vt’ =- Avt………..(10)
In equation (7) put x=0 we get
t’ = Dt
So equation (10) become
-v Dt =- Avt
A =D ………..(11)
A light pulse is sent out from the origin of S towards x at t=0, its location is given by
x= ct
x’ = ct’
Substituting values of x and x' in equation (4), we get
Ct’ = Act +Bt
Substituting the value of B from equation (8), we get
Ct’ = Act +(- Avt) ………..(12)
Substituting values of x and D from eq no (11) in eq no.(7) , we get
t’ = Cct +At
Substituting above value of t' in eq no.(12) , we get
c(Cct +At) = Act - Avt
Or
Cc2t +Act =Act - Avt
Cc2t =Avt
Or
C =-A  ………..(13)
………..(13)
A light pulse is emitted along y axis in S system at t=0, its coordinate in both systems are
y= ct, x=0
r’=ct’ where
Using equation (5)
r’2 = x’2+y’2
Using equation (5)
y’= y= ct
And from equation (4) and (7)
x’ = Bt = -Avt
t’= Dt =-At
Therefore r’2 = x’2+y’2 become
c2t’2 = (-Avt)2 +(ct)2
Substituting value of t' above equation became
c2A2t2 = A2v2t2 +c2t2
(c2 - v2) A2t2 = c2t2
A2 = 
A = 
A = 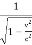 ………..(14)
………..(14)
Substituting the Values of A, B, C, D in equation (1) and (4)
So the Lorentz transformation equations would be,
x’= 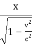 -
- 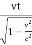
x’=  (x-vt)
(x-vt)
x’= (x-vt)
(x-vt)
Where 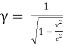
And
t’ = - 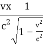 +
+ 
t’= 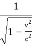 ( t -
( t -  )
)
Lorentz transformation equations therefore are
x’= 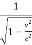 (x - vt)
(x - vt)
y’ =y
z’ = z
t’= 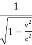 ( t -
( t -  )
)
Or these can be written as
x’= 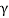 (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 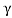 ( t -
( t -  )
)
Where 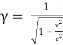
This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.
The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:
x= 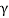 (x’+ vt)
(x’+ vt)
y =y’
z = z’
t=  (t’ +
(t’ + 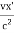 )
)
Q11) What do you meant by length contraction?
A11)
Consider a frame S' moving with respect to S with a uniform speed v along x direction figure. Let the rod AB lie along the O'x' axis. The rod is at rest in the frame S' and its length as measured by an observer in S' shall be called the rest length of the rod. To calculate this length the observer in S' will measure the x' coordinates of the two ends A and B. These coordinates shall be independent of the time of observation. If x1' and x2' are the x' coordinates of two ends A and B then the rest length l0 of the rod is
l0 = x'2 – x'1
In the coordinate system S the x coordinates of ends A and B will change with time. In order to measure the length of the rod, the observer in S will have to measure simultaneously the coordinates of the ends A and B. If x1 and x2 are the coordinates of ends A and B at the time of observation t then the length of rod as observed in frame S is
l= x2 – x1
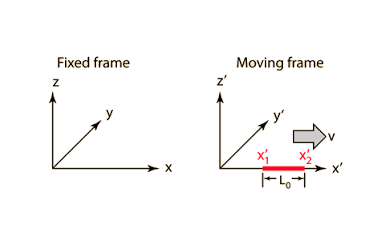
Figure: Length Contraction
From Lorentz equations we have
x'2 = 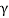 (x2 – v t)
(x2 – v t)
x1' = 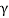 (x1 – v t)
(x1 – v t)
Subtracting these equations, we obtain
x2' - x1' = 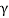 (x2 — x1)
(x2 — x1)
Using eq. (6.57) and (6.58) it becomes
l0 = 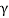 l
l
I = 
Since 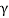 is always greater than one, therefore
is always greater than one, therefore
l < l0
Thus the length of the rod measured from the frame in which it is moving is smaller than its length in a frame in which it is at rest by a factor 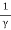 or
or  Hence the length of a moving rod appears shortened by a factor
Hence the length of a moving rod appears shortened by a factor 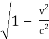
Note the following points
- The length contraction is significant only if the speed of rod approaches the velocity of light.
- The shortening of rod occurs only along the line of motion. The length of meter stick oriented perpendicular to the direction of motion is not changed.
Q12) What do you meant by time dilation?
A12)
Consider a frame S' moving with velocity v with respect to frame S along the x direction Fig (6.6). Suppose the clock is located in the system S' at the point x'. The clock is stationary with respect to S'. Let t1 ' and t2' are two instants of time recorded on this clock by an observer in S'. Then the interval of time as observed in S' is
∆t' = t2' – t1'
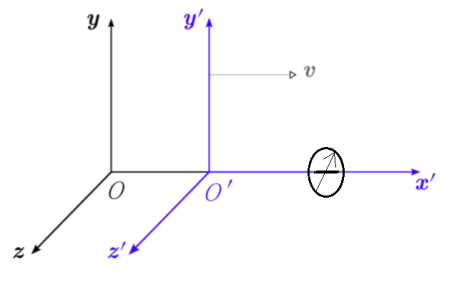
Figure: Time dilation
Now we wish to find how this time interval appears to an observer in S. This can be done by applying a Lorentz transformation from S' to S with the same value of x'. The transformation equation required is (6.51 d). From this we can write
t1 =
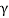 (t1' + v x' / c2)
(t1' + v x' / c2)
t2 =  (t'2 + v x' / c2)
(t'2 + v x' / c2)
Subtracting eq. (6.64) from eq. (6.63) we obtain
∆t= t1 – t2 = 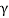 (t1' – t2')
(t1' – t2')
Using eq. (6.62) it becomes
∆t= t1 – t2 =  (∆t')
(∆t')
Since  > 1 so
> 1 so
∆t > ∆t'
This means that the time interval as observed in the moving frame of clock is greater than the One observed in the rest frame of the clock. We can express the same thing by saying that a moving clock appears to run slow. We often say that time has been dilated or lengthened in the moving observer frame. The time as observed by an observer moving with the clock is less than the one observed by a moving observer.
We highlight the following points
- Time intervals are affected by relative motion.
- A stationary clock measures a longer time interval between events occurring in a moving frame of reference than does a clock in the moving frame.
Q13) Discuss velocity addition theorem?
A13)
Let S and S’ be two frames of reference such that S’ is moving with respect to S with a uniform speed v in the positive x direction Figure. Consider a particle P which is moving in the frame S. Suppose its position coordinates at time t are (x,y,z).
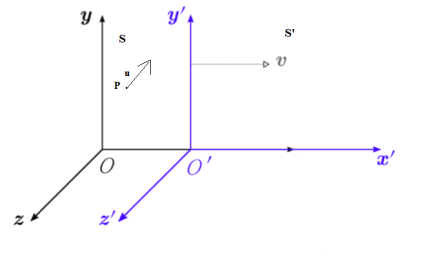
Then its velocity components in the frame S are
ux = uy =
uy =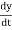 uz =
uz =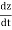 ………….(1)
………….(1)
We wish to find how do these velocity components appear to the observer in the frame S’. If (x’,y’,z’) are the instantaneous position coordinates of the particle P at time t’, then its velocity components in frame S’ are
u’x =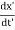 u’y =
u’y =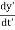 u’z =
u’z = ………….(2)
………….(2)
Consider the Lorentz transformation equations (2) i.e.
x’= 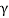 (x-vt)
(x-vt)
y’ =y
z’ = z
t’=  ( t -
( t -  )
)
Where 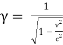
Take the differentials of both sides of above equations. This gives
dx’= 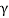 (dx- vdt)………….(3)
(dx- vdt)………….(3)
Dy’ =dy………….(4)
Dz’ = dz………….(5)
Dt’= 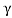 (dt -
(dt - 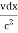 ) ………….(6)
) ………….(6)
Divide equation (3) by (6)
u’x =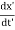 =
= 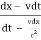
u’x = 
Using equation (1) it becomes
u’x = 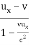
Now divide equation (4) by (6)
u’y =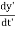 =
= 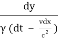
=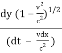
=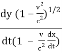
Using equation (1) it becomes
u’y =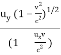
Now divide equation (4) by (6)
u’z =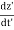 =
=
=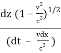
=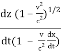
Using equation (1) it becomes
u’z =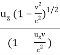
Collecting together equations for u’x ,u’y ,u’z we have
u’x = 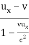 ………….(7)
………….(7)
u’y =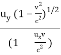 ………….(8)
………….(8)
u’z =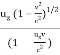 ………….(9)
………….(9)
These equations allow us to observe the observed velocity in frame S and S’ which is in uniform translation motion.
To obtain inverse transformation from S and S’ change dashed quantities to undashed one and vice versa and change v to –v in equation (7), (8) and (9) we get
ux = 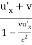 ………….(10)
………….(10)
uy = ………….(11)
………….(11)
uz =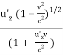 ………….(12)
………….(12)
Q14) Discuss the variation of mass with velocity?
A14)
To explain the variation of mass with velocity, consider two frames of references S and S'. S' is moving with constant velocity v along X-direction.
Let us consider two exactly similar balls A and B each of mass m. Both these balls A and B are moving in opposite direction along X-axis with equal speed u' in frame S'.
After collision they merge into one body.
Applying the law of conservation of momentum on the collision of the balls in frame S', we have
Momentum of balls before collision = Momentum of balls after collision
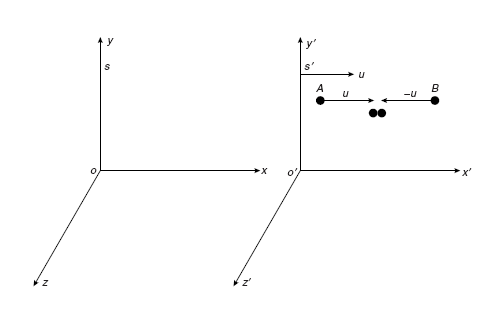
After collision, the merged mass must be at rest in frame S'. Hence, it moves with velocity v in frame S.
Let u1, u2 be the velocities and m1, m2 be the masses of balls A and B, respectively, in frame S.
Using the law of addition of velocities, the above velocities can be written as
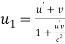 ………(1)
………(1)
 ………(2)
………(2)
Applying the law of conservation of momentum on the collision of the balls in frame s, we have
m1u1 + m2u2 = (m1 + m2)v ………(3)
Substituting u1 and u2 values from Equations (1) and (2), we have
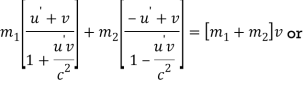
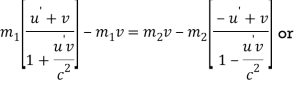
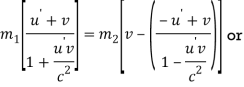

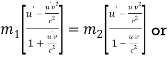
 ………(4)
………(4)
The above equation makes a relationship between the masses of balls in frame S and their velocities in frame S'.
Now, to obtain relation between masses of balls and their velocities in frame S, we proceed as follows. Squaring Equation (1)
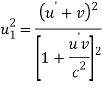
And using the above equation, the value  is
is
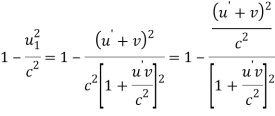
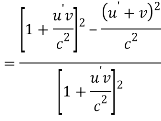
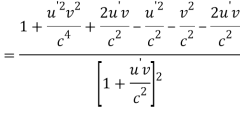
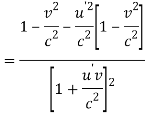
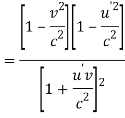
Therefore,
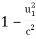 =
= 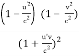 ………(5)
………(5)
 =
= 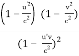 ………(6)
………(6)
Dividing Equation (6) by Equation (5) and taking square root throughout, we have
 =
= ………(7)
………(7)
Now from equation (4) and (7) we get
 =
= 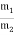 ……… (8)
……… (8)
Suppose, m2 is at rest in frame S, then u2 = 0 and m2 = m0 (say) where m0 is the rest mass of the ball B, then Equation (8) becomes
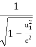 =
=  ………(9)
………(9)
As both the balls are similar, hence the rest masses of both balls are the same, so we can write the rest mass of m2 is equal to rest mass of m1, that is equal to mo. Then, Equation (9) becomes
 =
=  ………(10)
………(10)
Here, m1 is the mass of ball A when it is moving with velocity u1 in frame S. After collision, the merged mass containing mass of ball A moves with velocity v in frame S.
In general, if we take the mass of ball A as m, when it is moving with velocity v in frame S, then
 = m ………(11)
= m ………(11)
Where m0 is the rest mass of the body and m is the effective mass.
Equation (11) is the relativistic formula for the variation of mass with velocity. Here, we see some special cases:
Case (i)
When the velocity of the body, v is very small compared to velocity of height, c and then 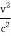 is negligible compared to one. Therefore,
is negligible compared to one. Therefore,
m = m*0
Case (ii)
If the velocity of the body v is comparable to the velocity of light c, then 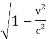 is less than one, so m > mo.
is less than one, so m > mo.
The mass of a moving body appears greater than its rest mass.
Case (iii)
Suppose the velocity of a body is equal to velocity of light c, then it possess infinite mass.
The effective mass of particles has been experimentally verified by using particle accelerators in case of electrons and protons by increasing their velocities very close to velocity of light.
Q15) What is Einstein’s mass energy relation?
A15)
Mass-energy equivalence implies that even though the total mass of a system changes, the total energy and momentum remain constant. Consider the collision of an electron and a proton. It destroys the mass of both particles but generates a large amount of energy in the form of photons.
Mass-energy equivalence states that every object possesses certain energy even in a stationary position.
A stationary body does not have kinetic energy. It only possesses potential energy and probable chemical and thermal energy.
According to the field of applied mechanics, the sum of all these energies is smaller than the product of the mass of the object and square of the speed of light.
Mass-energy equivalence means mass and energy are the same and can be converted into each other.
Einstein put this idea forth but he was not the first to bring this into the light. He described the relationship between mass and energy accurately using his theory of relativity. The equation is known as Einstein's mass-energy equation and is expressed as,
E = mc2
Where E= equivalent kinetic energy of the object.
The mass–energy equivalence gives the relation between the mass and energy.
Q16) Derive mass energy equation?
A16)
Derivation of mass energy equation
We know that according to classical mechanics, the rate of change of momentum is proportional to force acting upon the body in the direction of motion.
F = 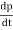 =
=  …...... (1)
…...... (1)
According to the theory of relativity, mass and velocity are variable so equation (1) after become.
 =
= 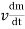 …...... (2)
…...... (2)
The force F acting on the body makes a displacement dx.
We know that work done dw is given by product of force and displacement
And also the work is stored as kinetic energy (dε) in the body. So work done can be written as
Dw = dε = Fdx ...........(3)
Substituting force F from Equation (2) in Equation (3). We get
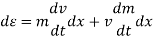
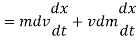
dε = mvdv + v2dm ...........(4)
And also velocity v = dx/dt
The relativistic mass m is given by
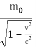 = m
= m
Squaring on both sides, we get
m2 = =
= 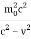
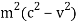 =
=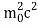 ........... (5)
........... (5)
Differentiating Equation (5), we get
2mc2dm − 2mv2dm − 2m2vdv = 0
c2dm = v2dm + mvdv …........ (6)
Comparing Equations (4) and (6), we get
dε = c2dm ............(7)
By applying force, the mass of a body increases from m0 to m and hence the kinetic energy. The total energy acquired by the body is obtained by integrating Equation (7)
ε = 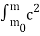 dm =
dm =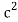
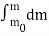 =
= 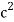 (m - m0)............ (8)
(m - m0)............ (8)
Therefore, increase in kinetic energy is due to the increase in mass of the body. The total energy (E) of the body is the sum of increase in kinetic energy of the body due to motion and the energy at rest (moc2):
∴ E = ε + moc2
Using Equation (8)
E = mc2 − moc2 + moc2 = mc2
E = mc2 …………. (9)
This shows that increase in energy equivalent to increase in mass. In other words anything that increase energy will increase its relativistic mass.
The above equation shows the equivalence between mass and energy also known as Einstein’s mass–energy relation.
Unit - 3
Unit - 3
Unit - 3
Theory of relativity
Q1) Calculate the time interval of the signal using Lorentz transformation when a spacecraft S’ is crossed by another spacecraft S at a speed of c/2 on their way to Alpha Centauri. When spacecraft S Crosses S’, the captain of S’ sends a signal that lasts for 1.2s. What is the fine time interval of the single?
A1)
Given:
Δt’ = t‘2−t‘1 = 1.2s
Δx’ = x‘2−x‘1 = 0
The time signal starts and ends at (x’, t‘1)
Using the first Lorentz transformation equation we get,
Δt = t2 – t1
Δx = x2 – x1
Δt= 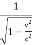 (
(  t’ +
t’ + 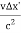 )
)
As the time interval of S’ is fixed, we can consider Δx’ = 0 and the time interval δt becomes:
Δt=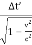
Substituting Δt’ = 1.2s we get
Δt=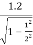
Δt = 1.6s
Q2) A certain process requires 10-6 sec to occur in an atom at rest in laboratory. How much time will this process require to an observer in the laboratory? When the atom is moving with speed of 5 x107 m/sec.
A2)
Given Δt = 10-6 sec
v = 5 x109 cm/sec
∆t=  (∆t')
(∆t')
∆t' = ∆t /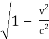
∆t' = = 1.028 x 10-6 sec
= 1.028 x 10-6 sec
Q3) Compute the contracted length of an object whose initial length 10m and travel with a velocity 0.75c?
A3)
Given parameters are,
L0 = 10m
v = 0.75c
c = Speed of light (3.0 x 108 m/s)
The formula for length contraction is
l0 =  l
l
Or L0 =  L
L
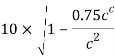
L = 10 × √1- (0.75)2
L = 10 × √(o.438)
L = 6.618 m.
Q4) A observer on earth sees a rocket zoom by at .95c. If the rocket is measured to be 5.5 m in length, how long is the rocket ship as measure by the astronaut inside the rocket?
A4)
The formula for length contraction is
l0 =  l
l
Or
L0 =  L
L
L = 5.5 m
v = .95c
L0 = ?
5.5 = L0×(1- 0.95c2/c2)
5.5 = L0×(0.312)
L0 = 5.5/(0.312) = 17.6 m
Q5) Difference between inertial & non-inertial frames?
A5)
Inertial frame of reference
An inertial frame is defined as one in which Newton’s law of inertia holds that is anybody which isn’t being acted on by an outside force stays at rest if it is initially at rest, or continues to move at a constant velocity if that’s what it was doing to begin with.
Non-inertial frame of reference
A frame that is accelerated with respect to the assumed inertial frame of reference. Newton’s law will not hold true in these frames.
Q6) The Hypersonic Technology Vehicle 2 (HTV-2) is an experimental rocket vehicle capable of traveling at 21,000 km/h (5830 m/s). If an electronic clock in the HTV-2 measures a time interval of exactly 1-s duration, what would observers on Earth measure the time interval to be?
A6)
Given
Δτ=1s
v=5830m/s.
Δt =?
We know ∆t= t1 – t2 = 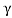 (∆t')
(∆t')
Δt=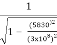 = 1.000000000189s
= 1.000000000189s
Q7) What is Galilean Transformations? How velocity and acceleration transform?
A7) Galilean Coordinate Transformations: For simplicity: - Let coordinates in both references equal at (t = 0). Use Cartesian coordinate systems.
Newton’s laws are only applicable at inertial reference frames. According to Galilean transformations, Newton’s laws are invariant at any inertial reference frame. In other words, the mechanical movement of a particle is exactly the same at two different reference frames, If both of them are inertial (i.e. one moves with constant velocity w.r.t the other & vice versa).
There is no mechanical experiment by which one can distinguish whether a system is at rest or is moving with a constant speed in a straight line (Galilean relativity).
Suppose we know from experiment that these laws of mechanics are true in one frame of reference. How do they look in another frame, moving with respect to the first frame?
To find out, we have to figure out how to get from position, velocity and acceleration in one frame to the corresponding quantities in the second frame.
Obviously, the two frames must have a constant relative velocity, otherwise the law of inertia won’t hold in both of them. Let’s choose the coordinates so that this velocity is along the x-axis of both of them.
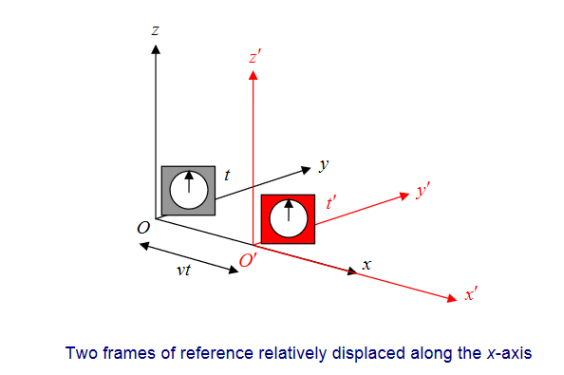
Notice we also throw in a clock with each frame. Suppose S′ is moving relative to S at speed v along the x-axis. For convenience, let us label the moment when O′ passes O as the zero point of timekeeping. That mean at t=0 O and O’ coincide with each other.
Now let us try to find the coordinates of the event (x, y, z, t) in S′
It’s easy to see t′ = t
We synchronized the clocks when O′ passed O. Also, evidently, y′ = y and z′ = z, from the figure.
We can also see that x = x′ +vt.
Thus (x, y, z, t) in S corresponds to (x′, y′, z′, t′) in S′, where
x = x′ +vt.
y = y’
z = z’
t =t’
That’s how positions transform; these are known as the Galilean Transformations.
Velocity
The velocity in S′ in the x′ direction is given by u’x
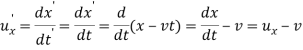
This is obvious anyway it’s just the addition of velocities formula.
ux = u’x +v
Acceleration
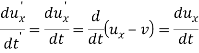
Since v is constant. That is to say,
a’x =ax
The acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.
Q8) Explain construction, working and conclusion of Michelson Morley experiment?
A8)
The experiment was devised to determine the absolute velocity of earth with respect to ether frame. The simplified plan of the experiment is shown in figure.
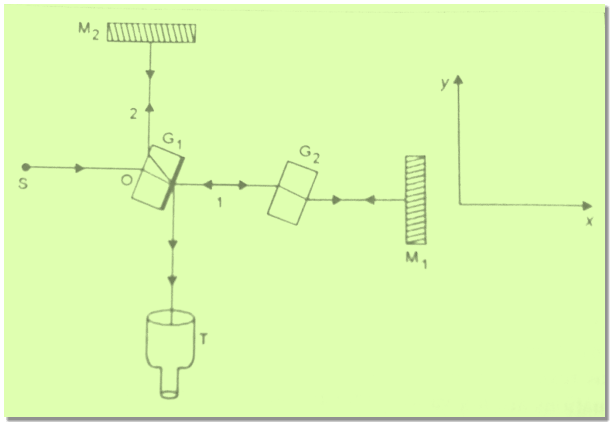
Figure: Michelson Morley Experiment
Construction
Light from a source S falls on a glass plate G1 inclined at an angle of 45° to the incident beam. The back surface of the plate G1 is half silvered. This plate divides the incident beam into two beams 1 and 2 of equal intensity. The beam 1 (transmitted by the plate G1) goes straight to another glass plate G2 of the same thickness as G1 and inclined at an angle of 45° to the beam. This beam after crossing G2 falls normally on the plate mirror M1 and is reflected back towards glass plate G1. On reaching the back surface of G1 it again suffers 50% reflection. The reflected part enters the telescope T.
The beam 2 (reflected by the glass plate G1) moves towards the mirror M2, to fall normally on it. It retraces its path after reflection from M2. When it reaches the back surface of G1, it suffers 50% transmission. The transmitted part goes into the telescope and interferes with the reflected part of the beam 1. Interference fringes are therefore observed in the telescope.
Since the two glass plates G1 and G2 are identical then the path of the two beams are equal in glass. Each beam passes three times through a glass plate in its journey from the source to the telescope. If the mirrors M1 and M2 are at the same distance from 0, then the optical path lengths of two beams shall be equal say l.
Working
We know that the earth moves around the sun with an orbital velocity of Since the earth is moving through ether with velocity v it experiences an ether wind of velocity  . Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
. Suppose the velocity of the earth is along positive x-direction. Let the apparatus be oriented in such a way that incident beam travels parallel to the velocity of earth. Then the velocity of beam is along positive x-axis. When this incident beam travels in the positive x direction, it faces an ether wind blowing in negative x direction.
Time for round trip of light beam 1 travelling in x direction.
When the beam 1 transmitted by G1 travels along the positive x direction from 0 to M1, its effective velocity v1 in earth’s frame is
v1 = c - v
Because the ether wind is opposed to the direction of motion of the beam. The time taken by the light to travel from 0 to M1 is
t1 =  =
= 
In the return journey from M1 to 0 the ether wind is supporting the beam of light. Therefore its effective velocity v2 in earth's frame is
v2 = c + v
The time taken by the light for its return journey M1O is
t2 = 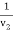 =
= 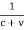
Thus the time of the round trip OM1O is
T1 = t1 + t2
T1 = 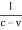 +
+ 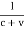 +
+ 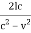
T1 = 2lc ( – 1 =
– 1 = 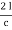 (1 -
(1 - 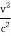 ) – 1
) – 1
Since  =
= 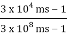 = 10-4 << 1
= 10-4 << 1
We can expand the above expression by Binomial theorem. This gives
T1 = 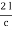 (1 -
(1 - 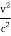 )
)
Time for round trip of light beam 2 travelling in y direction.
The beam 2 travels from O to the mirror M2 parallel to y direction. Since it to moving parallel to y direction its effective velocity must be in the y direction.
Suppose 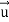 is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector
is the effective velocity of beam 2 in y direction as shown in figure. The ether wind velocity is in the negative x direction and is shown by vector 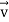 . Therefore the actual velocity of beam 2 should be in OA direction. From figure.
. Therefore the actual velocity of beam 2 should be in OA direction. From figure.
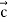 +
+  =
= 
 =
= 
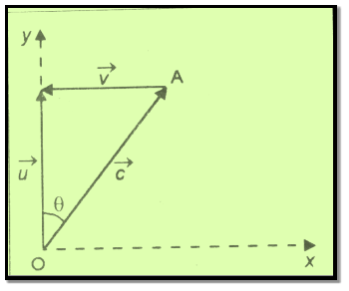
The square of magnitude of  is
is
c2 = u2 + v2 – 2 u v cos 90°
c2 = u2 + v2
Or u2 = c2 - v2
u = 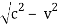
The effective velocity u of the beam is the same during its return journey from M2 to O. Thus time taken to travel from M2 to O is
t2' = t1' = 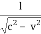
Thus the time of round trip for the beam 2 is
T2 = 2t1' = 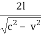
T2 = 2l (c2 – v2)-1/2 = 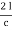 (1 -
(1 - 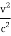 )-1/2
)-1/2
Since  << 1 we can expand by Binomial theorem. This gives
<< 1 we can expand by Binomial theorem. This gives
T2 =  ( 1 +
( 1 + 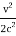 )
)
Expected Fringe shift
The time difference between the two beams for their round trips is
T = T1 – T2  ( 1 +
( 1 + 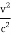 ) -
) - 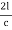 ( 1 +
( 1 + 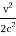 )
)
T= 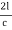 [( 1 +
[( 1 + 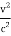 - 1 -
- 1 - 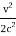 )]
)]
T= 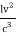
Thus the optical path difference 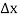 between two beams corresponding to the time difference T is
between two beams corresponding to the time difference T is
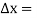 cT =
cT =
Now suppose this whole apparatus is turned through 90° so that the two beams interchange their paths. Thus path OM2, becomes parallel to the incident beam and OM1 becomes perpendicular to the incident beam. Then the two times T1 and T2 will interchange and the path difference between two beams changes from 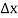 to -
to - 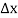 . Thus the path difference between two beams in the second position is
. Thus the path difference between two beams in the second position is
 = -
= - 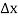 = -
= -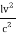
The change in the optical path difference between the two beams due to 90° rotation of the apparatus
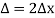 =
= 
This path difference should lead to the shift of fringes.
Order of Fringe Shift
Suppose λ is the wavelength of light used. We know that a path difference of one wavelength λ corresponds to shift of one fringe across a cross wire in the field of view of telescope. If  n is the number of fringes which shift on turning the apparatus through 90° then
n is the number of fringes which shift on turning the apparatus through 90° then
 n =
n =
In Michelson Morely experiment the distance l= 11 meters,
λ = 5900 Å = 5.9 x 10-7 m
v/c = 10-4. Thus
 n = 0 37
n = 0 37
Thus a fringe shift was expected in this experiment if the concept of the ether frame was valid. In actual experiment no fringe shift was observed.
Conclusion
The negative result of Michelson Morley experiment led to the following conclusion.
The motion of earth through the ether could not to be detected experimentally. Thus the concept of a preferred ether frame must be discarded.
The speed of light in vacuum is same in all frames of reference which are in uniform relative motion.
This experiment paved the way for the development of the special theory of relativity by Einstein in the year 1905.
Q9) Write postulates of special theory of relativity?
A9)
Einstein gave two postulates to explain the negative result of Michelson-Morley experiment. They form the basis of the special theory of relativity. These postulates are:
- The laws of physics take the same form in all inertial frames.
According to first postulate all inertial frames are equivalent. It is meaningless to talk of absolute motion. Only the motion relative to frame of reference has any physical meaning.
- The velocity of light in vacuum has the same value c and is independent of the relative velocity of the source and the observer.
The second postulate simply expresses the result of Michelson Morley experiment.
Q10) Derive Lorentz Transformation equation?
A10)
Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.
The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame.
Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame.
Expressing these relations in Cartesian coordinates gives
x2+y2+z2−c2t2 =0 ………..(1)
x′2+y′2+z′2−c2t′2=0………..(2)
The left-hand sides Equations and can be set equal because both are zero.
Because y=y′ and z=z′ so, we obtain
x2−c2t2 =x′2−c2t′2………..(3)
However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.
To find the correct set of transformation equations, assume the two coordinate systems S and S′
The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified.
Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.
Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis.
The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as,
x’ = Ax +Bt………..(4)
y’ = y………..(5)
z’ = z………..(6)
t’ = Cx +Dt………..(7)
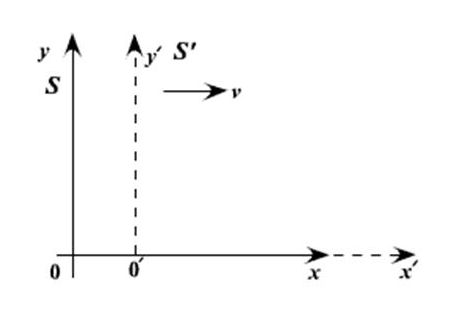
The transformations are linear.
If they are not linear one system would predict acceleration while in other system velocity was even constant.
Because of symmetry, y' and z' axes are left unchanged by the transformation.
Constant can be calculated by using the following boundary conditions.
Observers in S and S' see (O') the origin of S' as
x = vt
x’ = 0
Substituting values of x and x' in equation (4) We get
i = Avt +Bt
i.e. B =- Av………..(8)
Observer in S' and S see the origin (O) of S as
x' = vt
x = 0
Substituting values of x and x' in equation (4) we get
-vt’ =Bt………..(9)
Substituting the value of B from equation (8), we get
-vt’ =- Avt………..(10)
In equation (7) put x=0 we get
t’ = Dt
So equation (10) become
-v Dt =- Avt
A =D ………..(11)
A light pulse is sent out from the origin of S towards x at t=0, its location is given by
x= ct
x’ = ct’
Substituting values of x and x' in equation (4), we get
Ct’ = Act +Bt
Substituting the value of B from equation (8), we get
Ct’ = Act +(- Avt) ………..(12)
Substituting values of x and D from eq no (11) in eq no.(7) , we get
t’ = Cct +At
Substituting above value of t' in eq no.(12) , we get
c(Cct +At) = Act - Avt
Or
Cc2t +Act =Act - Avt
Cc2t =Avt
Or
C =-A  ………..(13)
………..(13)
A light pulse is emitted along y axis in S system at t=0, its coordinate in both systems are
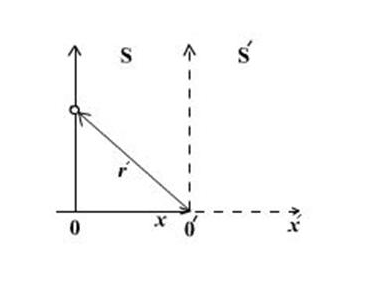
y= ct, x=0
r’=ct’ where
Using equation (5)
r’2 = x’2+y’2
Using equation (5)
y’= y= ct
And from equation (4) and (7)
x’ = Bt = -Avt
t’= Dt =-At
Therefore r’2 = x’2+y’2 become
c2t’2 = (-Avt)2 +(ct)2
Substituting value of t' above equation became
c2A2t2 = A2v2t2 +c2t2
(c2 - v2) A2t2 = c2t2
A2 = 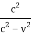
A = 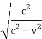
A =  ………..(14)
………..(14)
Substituting the Values of A, B, C, D in equation (1) and (4)
So the Lorentz transformation equations would be,
x’= 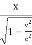 -
- 
x’= 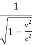 (x-vt)
(x-vt)
x’=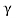 (x-vt)
(x-vt)
Where 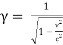
And
t’ = - 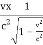 +
+ 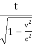
t’= 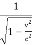 ( t -
( t -  )
)
Lorentz transformation equations therefore are
x’=  (x - vt)
(x - vt)
y’ =y
z’ = z
t’= 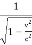 ( t -
( t -  )
)
Or these can be written as
x’= 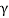 (x-vt)
(x-vt)
y’ =y
z’ = z
t’= 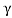 ( t -
( t -  )
)
Where 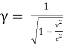
This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.
The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:
x= 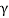 (x’+ vt)
(x’+ vt)
y =y’
z = z’
t= 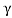 (t’ +
(t’ +  )
)
Q11) What do you meant by length contraction?
A11)
Consider a frame S' moving with respect to S with a uniform speed v along x direction figure. Let the rod AB lie along the O'x' axis. The rod is at rest in the frame S' and its length as measured by an observer in S' shall be called the rest length of the rod. To calculate this length the observer in S' will measure the x' coordinates of the two ends A and B. These coordinates shall be independent of the time of observation. If x1' and x2' are the x' coordinates of two ends A and B then the rest length l0 of the rod is
l0 = x'2 – x'1
In the coordinate system S the x coordinates of ends A and B will change with time. In order to measure the length of the rod, the observer in S will have to measure simultaneously the coordinates of the ends A and B. If x1 and x2 are the coordinates of ends A and B at the time of observation t then the length of rod as observed in frame S is
l= x2 – x1
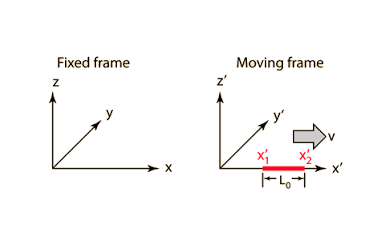
Figure: Length Contraction
From Lorentz equations we have
x'2 = 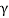 (x2 – v t)
(x2 – v t)
x1' =  (x1 – v t)
(x1 – v t)
Subtracting these equations, we obtain
x2' - x1' =  (x2 — x1)
(x2 — x1)
Using eq. (6.57) and (6.58) it becomes
l0 =  l
l
I = 
Since 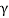 is always greater than one, therefore
is always greater than one, therefore
l < l0
Thus the length of the rod measured from the frame in which it is moving is smaller than its length in a frame in which it is at rest by a factor  or
or  Hence the length of a moving rod appears shortened by a factor
Hence the length of a moving rod appears shortened by a factor 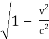
Note the following points
- The length contraction is significant only if the speed of rod approaches the velocity of light.
- The shortening of rod occurs only along the line of motion. The length of meter stick oriented perpendicular to the direction of motion is not changed.
Q12) What do you meant by time dilation?
A12)
Consider a frame S' moving with velocity v with respect to frame S along the x direction Fig (6.6). Suppose the clock is located in the system S' at the point x'. The clock is stationary with respect to S'. Let t1 ' and t2' are two instants of time recorded on this clock by an observer in S'. Then the interval of time as observed in S' is
∆t' = t2' – t1'
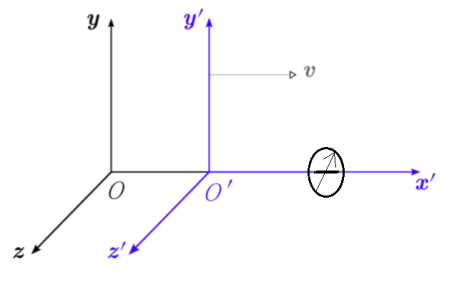
Figure: Time dilation
Now we wish to find how this time interval appears to an observer in S. This can be done by applying a Lorentz transformation from S' to S with the same value of x'. The transformation equation required is (6.51 d). From this we can write
t1 =
 (t1' + v x' / c2)
(t1' + v x' / c2)
t2 = 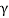 (t'2 + v x' / c2)
(t'2 + v x' / c2)
Subtracting eq. (6.64) from eq. (6.63) we obtain
∆t= t1 – t2 =  (t1' – t2')
(t1' – t2')
Using eq. (6.62) it becomes
∆t= t1 – t2 =  (∆t')
(∆t')
Since  > 1 so
> 1 so
∆t > ∆t'
This means that the time interval as observed in the moving frame of clock is greater than the One observed in the rest frame of the clock. We can express the same thing by saying that a moving clock appears to run slow. We often say that time has been dilated or lengthened in the moving observer frame. The time as observed by an observer moving with the clock is less than the one observed by a moving observer.
We highlight the following points
- Time intervals are affected by relative motion.
- A stationary clock measures a longer time interval between events occurring in a moving frame of reference than does a clock in the moving frame.
Q13) Discuss velocity addition theorem?
A13)
Let S and S’ be two frames of reference such that S’ is moving with respect to S with a uniform speed v in the positive x direction Figure. Consider a particle P which is moving in the frame S. Suppose its position coordinates at time t are (x,y,z).
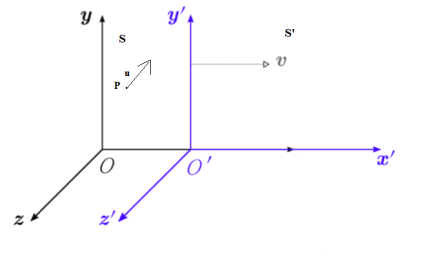
Then its velocity components in the frame S are
ux =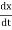 uy =
uy =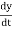 uz =
uz = ………….(1)
………….(1)
We wish to find how do these velocity components appear to the observer in the frame S’. If (x’,y’,z’) are the instantaneous position coordinates of the particle P at time t’, then its velocity components in frame S’ are
u’x =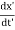 u’y =
u’y = u’z =
u’z = ………….(2)
………….(2)
Consider the Lorentz transformation equations (2) i.e.
x’=  (x-vt)
(x-vt)
y’ =y
z’ = z
t’=  ( t -
( t -  )
)
Where 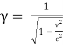
Take the differentials of both sides of above equations. This gives
dx’=  (dx- vdt)………….(3)
(dx- vdt)………….(3)
Dy’ =dy………….(4)
Dz’ = dz………….(5)
Dt’= 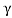 (dt -
(dt - 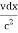 ) ………….(6)
) ………….(6)
Divide equation (3) by (6)
u’x =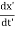 =
= 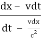
u’x = 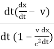
Using equation (1) it becomes
u’x = 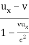
Now divide equation (4) by (6)
u’y = =
= 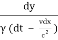
=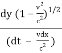
=
Using equation (1) it becomes
u’y =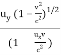
Now divide equation (4) by (6)
u’z = =
=
=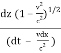
=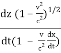
Using equation (1) it becomes
u’z =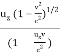
Collecting together equations for u’x ,u’y ,u’z we have
u’x = 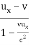 ………….(7)
………….(7)
u’y =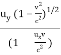 ………….(8)
………….(8)
u’z = ………….(9)
………….(9)
These equations allow us to observe the observed velocity in frame S and S’ which is in uniform translation motion.
To obtain inverse transformation from S and S’ change dashed quantities to undashed one and vice versa and change v to –v in equation (7), (8) and (9) we get
ux = 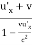 ………….(10)
………….(10)
uy =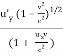 ………….(11)
………….(11)
uz = ………….(12)
………….(12)
Q14) Discuss the variation of mass with velocity?
A14)
To explain the variation of mass with velocity, consider two frames of references S and S'. S' is moving with constant velocity v along X-direction.
Let us consider two exactly similar balls A and B each of mass m. Both these balls A and B are moving in opposite direction along X-axis with equal speed u' in frame S'.
After collision they merge into one body.
Applying the law of conservation of momentum on the collision of the balls in frame S', we have
Momentum of balls before collision = Momentum of balls after collision
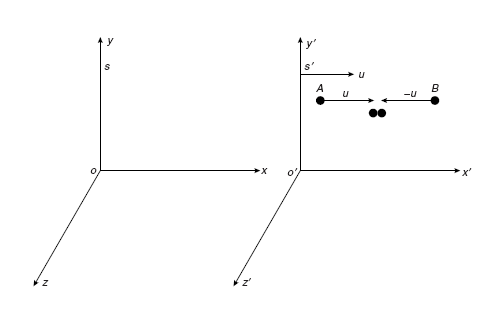
After collision, the merged mass must be at rest in frame S'. Hence, it moves with velocity v in frame S.
Let u1, u2 be the velocities and m1, m2 be the masses of balls A and B, respectively, in frame S.
Using the law of addition of velocities, the above velocities can be written as
 ………(1)
………(1)
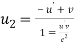 ………(2)
………(2)
Applying the law of conservation of momentum on the collision of the balls in frame s, we have
m1u1 + m2u2 = (m1 + m2)v ………(3)
Substituting u1 and u2 values from Equations (1) and (2), we have
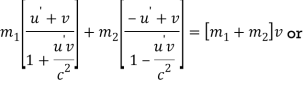
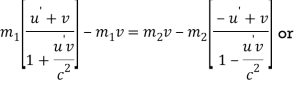

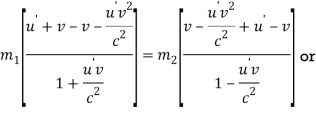
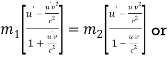
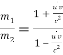 ………(4)
………(4)
The above equation makes a relationship between the masses of balls in frame S and their velocities in frame S'.
Now, to obtain relation between masses of balls and their velocities in frame S, we proceed as follows. Squaring Equation (1)
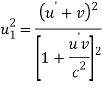
And using the above equation, the value  is
is
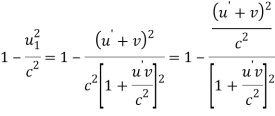
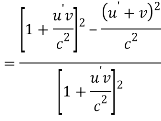
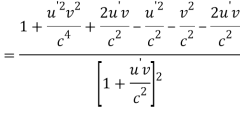
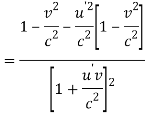
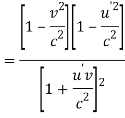
Therefore,
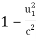 =
= 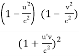 ………(5)
………(5)
 =
= 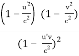 ………(6)
………(6)
Dividing Equation (6) by Equation (5) and taking square root throughout, we have
 =
=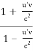 ………(7)
………(7)
Now from equation (4) and (7) we get
 =
= 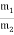 ……… (8)
……… (8)
Suppose, m2 is at rest in frame S, then u2 = 0 and m2 = m0 (say) where m0 is the rest mass of the ball B, then Equation (8) becomes
 =
= 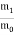 ………(9)
………(9)
As both the balls are similar, hence the rest masses of both balls are the same, so we can write the rest mass of m2 is equal to rest mass of m1, that is equal to mo. Then, Equation (9) becomes
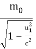 =
=  ………(10)
………(10)
Here, m1 is the mass of ball A when it is moving with velocity u1 in frame S. After collision, the merged mass containing mass of ball A moves with velocity v in frame S.
In general, if we take the mass of ball A as m, when it is moving with velocity v in frame S, then
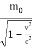 = m ………(11)
= m ………(11)
Where m0 is the rest mass of the body and m is the effective mass.
Equation (11) is the relativistic formula for the variation of mass with velocity. Here, we see some special cases:
Case (i)
When the velocity of the body, v is very small compared to velocity of height, c and then 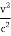 is negligible compared to one. Therefore,
is negligible compared to one. Therefore,
m = m*0
Case (ii)
If the velocity of the body v is comparable to the velocity of light c, then 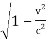 is less than one, so m > mo.
is less than one, so m > mo.
The mass of a moving body appears greater than its rest mass.
Case (iii)
Suppose the velocity of a body is equal to velocity of light c, then it possess infinite mass.
The effective mass of particles has been experimentally verified by using particle accelerators in case of electrons and protons by increasing their velocities very close to velocity of light.
Q15) What is Einstein’s mass energy relation?
A15)
Mass-energy equivalence implies that even though the total mass of a system changes, the total energy and momentum remain constant. Consider the collision of an electron and a proton. It destroys the mass of both particles but generates a large amount of energy in the form of photons.
Mass-energy equivalence states that every object possesses certain energy even in a stationary position.
A stationary body does not have kinetic energy. It only possesses potential energy and probable chemical and thermal energy.
According to the field of applied mechanics, the sum of all these energies is smaller than the product of the mass of the object and square of the speed of light.
Mass-energy equivalence means mass and energy are the same and can be converted into each other.
Einstein put this idea forth but he was not the first to bring this into the light. He described the relationship between mass and energy accurately using his theory of relativity. The equation is known as Einstein's mass-energy equation and is expressed as,
E = mc2
Where E= equivalent kinetic energy of the object.
The mass–energy equivalence gives the relation between the mass and energy.
Q16) Derive mass energy equation?
A16)
Derivation of mass energy equation
We know that according to classical mechanics, the rate of change of momentum is proportional to force acting upon the body in the direction of motion.
F = 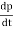 =
= 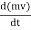 …...... (1)
…...... (1)
According to the theory of relativity, mass and velocity are variable so equation (1) after become.
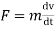 =
= 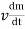 …...... (2)
…...... (2)
The force F acting on the body makes a displacement dx.
We know that work done dw is given by product of force and displacement
And also the work is stored as kinetic energy (dε) in the body. So work done can be written as
Dw = dε = Fdx ...........(3)
Substituting force F from Equation (2) in Equation (3). We get
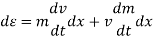
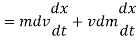
dε = mvdv + v2dm ...........(4)
And also velocity v = dx/dt
The relativistic mass m is given by
 = m
= m
Squaring on both sides, we get
m2 = =
= 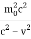
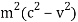 =
=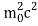 ........... (5)
........... (5)
Differentiating Equation (5), we get
2mc2dm − 2mv2dm − 2m2vdv = 0
c2dm = v2dm + mvdv …........ (6)
Comparing Equations (4) and (6), we get
dε = c2dm ............(7)
By applying force, the mass of a body increases from m0 to m and hence the kinetic energy. The total energy acquired by the body is obtained by integrating Equation (7)
ε = 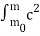 dm =
dm =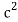
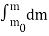 =
= 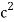 (m - m0)............ (8)
(m - m0)............ (8)
Therefore, increase in kinetic energy is due to the increase in mass of the body. The total energy (E) of the body is the sum of increase in kinetic energy of the body due to motion and the energy at rest (moc2):
∴ E = ε + moc2
Using Equation (8)
E = mc2 − moc2 + moc2 = mc2
E = mc2 …………. (9)
This shows that increase in energy equivalent to increase in mass. In other words anything that increase energy will increase its relativistic mass.
The above equation shows the equivalence between mass and energy also known as Einstein’s mass–energy relation.