Unit - 1
Principles of Electro mechanical Energy Conversion
Q1) An iron ring has a X-section of 4cm2 and a mean diameter of 20cm. An air gap of 0.5 mm is cut across the section of the ring. The ring is wound with a coil of 200 turns allowing 2A current passing through it. The total magnetic flux is 0.3mWb. Calculate relative permeability of iron.
A1) 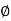 = 0.3mWb
= 0.3mWb
A=4cm2=4x10-4 m2
B=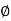 /A = 0.3x10-3/4x10-4 = 0.75Wb/m2
/A = 0.3x10-3/4x10-4 = 0.75Wb/m2
AT of iron ring = H x l= (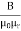 ) x l = (
) x l = (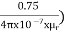 x 0.20 = 119.37x103/
x 0.20 = 119.37x103/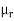
AT for air gap = H x l= ( ) x l = (
) x l = (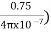 x 0.5 x 10-3 =298.42
x 0.5 x 10-3 =298.42
Total AT required = (119.37x103/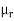 )+ 298.42
)+ 298.42
Also, Total AT required = 200x2= 400
(119.37x103/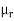 )+ 298.42 = 400
)+ 298.42 = 400
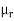 =1175
=1175
Q2) A laminated soft iron ring has a relative permeability 1000 has a mean circumference of 600mm. Cross-sectional area of 250mm2. A radial air gap of 1mm width I cut in the ring which wounds 1000 turns. Calculate the current required to produce an air gap flux of 0.4mWb. (leakage factor = 1.3 and stacking factor = 0.9)
A2) Total AT required = 
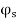 = 0.4mWb
= 0.4mWb
lg = 1mm=1x10-3m
Ag=250mm2=250x10-6m2
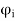 = 1.3 x 0.4x10-3 = 0.52mWb
= 1.3 x 0.4x10-3 = 0.52mWb
Net cross-sectional area = Ai x stacking factor = 250 x 10-6 x 0.9 = 225x10-6m2
Total AT required =  =
=  = 1803.75
= 1803.75
I= 1803.75/1000 = 1.803A
Q3) A ring sample of iron fitted with primary and secondary winding is to be tested by the method of reversal to obtain B-H curve. In the test the primary has 350 turns carrying 2A current. ON reversal a change of 8mWb-turns is recorded in secondary winding of 10 turns. The ring is made of 50 laminations, each 0.5mm thick with outer and inner diameter of 25 and 23 cm respectively. Determine B, H and permeability?
A3) Flux linkage = 2φN2 = 8x 10-3 Wb-turns
2φ x 10 = 8x 10-3
Φ = 4x10-4 Wb
A= 2.5x10-4 m2
B= Φ/A = 4x10-4/2.5x10-4 = 1.6Wb/m2
H= 350 x 2/0.24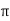 = 928.4 AT/m
= 928.4 AT/m
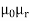 = B/H
= B/H
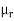 = B/H
= B/H = 1.6/(4
= 1.6/(4 x10-7x928.4) = 1371
x10-7x928.4) = 1371
Q4) The pole face area of an electromagnet is 0.5m2/pole. It has to lift an iron ingot weighing 860kg. If pole faces are parallel to the surface of the ingot at a distance of 0.8mm determine the coil mmf required.
A4) Force at two poles = 2x B2A/2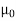 = B2A/
= B2A/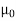
B2 0.5/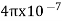 = 860 x 9.8
= 860 x 9.8
B= 0.145Wb/m2
H= B/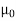 = 0.145/
= 0.145/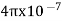 = 115.4x103 AT/m
= 115.4x103 AT/m
L=2x0.8mm=1.6x10-3m
AT required = 115.4 x103x1.6x10-3 = 184.64
Q5) Calculate the magnetising force and flux density at a distance of 4cm from a long straight circular conductor carrying a current of 250A and placed in air?
A5) H=I/2 r
r
=250/2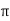 x 0.04=994.71 AT/m
x 0.04=994.71 AT/m
B= μoH
=4 x10-7x994.71=1.25x10-3 Wb/m2
x10-7x994.71=1.25x10-3 Wb/m2
Q6) A ring has a diameter of 20cm and a cross-sectional area of 10 cm2. The ring is made up of semi-circular section of cast iron and cast steel, with each joint having reluctance equal to an air gap of 0.2mm. Find the ampere turns required to produce a flux of 8x10-4Wb. The relative permeability of cast steel and cast iron are 800 and 166 respectively?
A6) φ = 8x10-4Wb
A= 10 cm2 = 10-3m2
B= Φ/A = 8x10-4/10-3= = 0.8Wb/m2
Air gap
H= B/μo = 0.8/4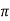 x10-7=6.37x105AT/m
x10-7=6.37x105AT/m
Total air gap length = 2x0.2 =0.4mm=4x10-4m
AT required = H x l = 6.37x105 x 4x10-4 = 255
Cast steel path
H= B/μo μr = 0.8/4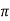 x10-7x800 = 796 AT/m
x10-7x800 = 796 AT/m
Path = D/2 = 20 x
D/2 = 20 x 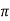 /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = H x l = 796x 0.314 = 250
Cast iron path
H= B/μo μr = 0.8/4 x10-7x166 = 3835 AT/m
x10-7x166 = 3835 AT/m
Path =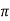 D/2 = 20 x
D/2 = 20 x 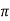 /2 = 31.42mm=0.314m
/2 = 31.42mm=0.314m
AT required = 3835x0.314= 1204
Total AT required = 255 + 250 + 1204 = 1709
Q7) Derive torque as a partial derivative of stored energy with respect to angular position of a rotating element?
A7) For torque equation deriving with reference to the above section we can write
DWe=id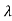 -Td
-Td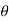
The energy stored in magnetic field is given as
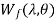 =
=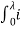 (
( d
d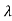
i=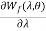
T=-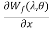
The energy stored in magnetic field is given as
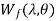 =
= (
(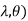 d
d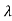
For magnetically linear system 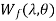 =
= 
The Torque acting will be T=-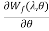
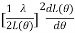 =
=  i2
i2 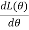
The above equation is the required value of torque as a partial derivative of stored energy with respect to angular position of a rotating element.
Q8) Explain the working of galvanometer?
A8) A moving coil galvanometer is an instrument which is used to measure electric current. A current-carrying coil when placed in an external magnetic field experiences magnetic torque. The angle through which the coil is deflected due to the effect of the magnetic torque is proportional to the magnitude of current in the coil. The moving coil galvanometer is made up of a rectangular coil that has many turns and it is usually made of thinly insulated or fine copper wire that is wounded on a metallic frame.
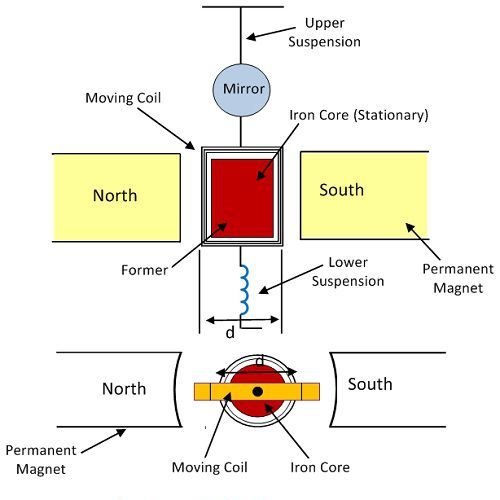
Fig 1 Galvanometer
The coil is free to rotate about a fixed axis. A phosphor-bronze strip that is connected to a movable torsion head is used to suspend the coil in a uniform radial magnetic field. A cylindrical soft iron core is symmetrically positioned inside the coil to improve the strength of the magnetic field and to make the field radial. The lower part of the coil is attached to a phosphor-bronze spring having a small number of turns. The other end of the spring is connected to binding screws.
We know that torque τ = force x perpendicular distance between the forces
τ = F × b
Substituting the value of F we already know,
Torque τ acting on single-loop of the coil = BIl × b
Where lx b is the area A of the coil,
Hence the torque acting on n turns of the coil is given by
τ = nIAB
The magnetic torque thus produced causes the coil to rotate, and the phosphor bronze strip twists. In turn, the spring S attached to the coil produces a counter torque or restoring torque kθ which results in a steady angular deflection.
Under equilibrium condition:
kθ = nIAB
Here k is called the torsional constant of the spring (restoring couple per unit twist). The deflection or twist θ is measured as the value indicated on a scale by a pointer which is connected to the suspension wire.
θ= ( nAB / k)I
Therefore θ ∝ I
The quantity nAB / k is a constant for a given galvanometer. Hence it is understood that the deflection that occurs the galvanometer is directly proportional to the current that flows through it.
Q9) What are relays. Explain its types and working?
A9) The relay contacts are opened or closed by a magnetic force. Basic parts and functions of electromechanical relays include:
- Frame: Heavy-duty frame that contains and supports the parts of the relay.
- Coil: Wire is wound around a metal core. The coil of wire causes an electromagnetic field.
- Armature: A relays moving part. The armature opens and closes the contacts. An attached spring returns the armature to its original position.
- Contacts: The conducting part of the switch that makes (closes) or breaks (opens) a circuit.
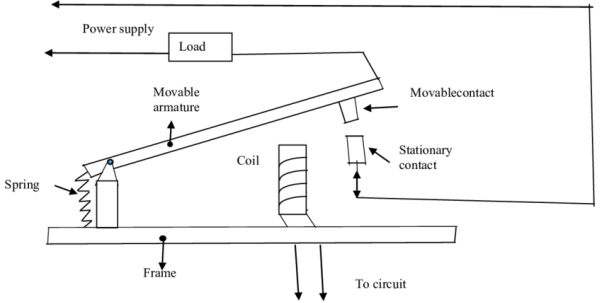
Fig 2 Relays
Relays have the energizing circuit and the contact circuit. The coil is on the energizing side and the relays contacts are on the contact side. When a relays coil is energized, current flow through the coil creates a magnetic field. The magnetic coil attracts a ferrous plate, which is part of the armature. One end of the armature is attached to the metal frame, which is formed so that the armature can pivot, while the other end opens and closes the contacts. Contacts come in a number of different configurations, depending on the number of Breaks, poles and Throws that make up the relay
- Break -This is the number of separate places or contacts that a switch uses to open or close a single electrical circuit. All contacts are either single break or double break. A single break (SB) contact breaks an electrical circuit in one place, while a double break (DB) contact breaks it in two places. Single break contacts are normally used when switching lower power devices such as indicating lights. Double break contacts are used when switching high-power devices such as solenoids.
- Pole -This is the number of completely isolated circuits that relays can pass through a switch. A single-pole contact (SP) can carry current through only one circuit at a time. A double-pole contact (DP) can carry current through two isolated circuits simultaneously.
- Throw -This is the number of closed contact positions per pole that are available on a switch. A switch with a single throw contact can control only one circuit, while a double-throw contact can control two.
Q10) An iron wire ring of 15 cm mean diameter having a cross-section of 90cm2 is wound with 400 turns of wire. Calculate the exciting current required to establish a flux density of 1Wb/m2 if relative permeability of iron is 1000. What is the value of energy stored?
A10) B=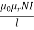
1=4 x
x 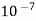 x 1000 x 400 x I/0.15
x 1000 x 400 x I/0.15 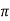
I=0.94A
L= AN2/l=4
AN2/l=4 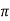 x
x 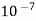 x 1000 x (90x 10-4) x (400)2/0.15
x 1000 x (90x 10-4) x (400)2/0.15  =3.84H
=3.84H
E= LI2
LI2
= x 3.84 x (0.94)2=1.696J
x 3.84 x (0.94)2=1.696J
Q11) Reluctance of a magnetic circuit is 105 AT/Wb and excitation coil has 220 turns. Current in the coil is changing at200A/s. Find the inductance of the coil and voltage induced in the coil.
A11) L= N2/S = 2202/105 = 0.48H
eL=L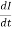 =0.48 x 200 =96.8V
=0.48 x 200 =96.8V
Q12) An iron ring of mean diameter 15cm has a cross sectional area of 100cm2 is wounded with 400turns of wire. Calculate the exciting current required to establish a flux density of 1Wb/m2. If relative permeability is 1000.
A12) B= 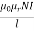
1=  x 1000x400xI/0.15
x 1000x400xI/0.15
I =0.94A
Q13) An iron ring of mean diameter 15cm has a cross sectional area of 100cm2 is wounded with 400turns of wire. Calculate the energy stored. The flux density is 1Wb/m2. If relative permeability is 1000.
A13) E=  L I2
L I2
B= 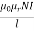
1=  x 1000x400xI/0.15
x 1000x400xI/0.15
I =0.94A
L= 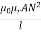 =
=  x 1000x 100x10-4x4002/0.15
x 1000x 100x10-4x4002/0.15 =4.27H
=4.27H
E=  L I2
L I2
=  x 4.27 x 0.94 = 2.01J
x 4.27 x 0.94 = 2.01J
Q14) Determine torque established by the armature of six pole DC motor having 680 conductors, two paths in parallel, 24mWb flux/pole and armature current 50A?
A14) 
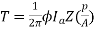
T=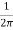 x24x10-3x50x680x(4/2)=259.74 N-m
x24x10-3x50x680x(4/2)=259.74 N-m
Q15) A 220V DC Shunt motor runs at 450rpm when armature current is 39A. Calculate speed if the torque is doubled. The armature has a resistance of 0.2 ohm?
A15) Ta α φIa
Ta1 α φIa1 and Ta2 α φIa2
2= Ia2/39
Ia2=78A
N2/N1=Eb2/Eb1
Eb1=220-(39x0.2)=212.2Volts
Eb2=220-(78x0.2)=204.4Volts
N2/450=204.4/212.2
N2=433.46 rpm
Q16) A 4 pole, 210 V shunt motor has 450 lap wound conductor. It takes 32 A from supply mains and develops output power of 4.5 kW. The field takes 1 A. The armature resistance is 0.09 ohm and flux per pole is 30 mWb. Calculate speed and torque.
A16) Ia = 32-1 = 31 n
Eb = V – Ia Ra = 210 - (0.09×31) = 207.21 V
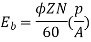
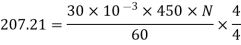
N = 920.9 rpm
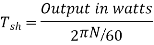
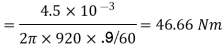
Q17) A 220 V, dc shunt motor has an armature resistance of 0.3 ohm and field resistance of 105 ohm. At no load speed is 1200 rpm and armature current is 2.3 A on application of rated load speed drops to 1120 rpm. Find the current and power input when motor delivers rated load.
A17) N1 = 1200 rpm Eb1 = 220 – (0.3×2.3) = 219.31 V
N2 = 1120 rpm Eb2 = 220 – 0.3 Ia2
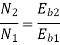
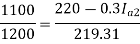
Ia2 = 37.93 A
Line current = Ia2 + Ish = 37.93 + (220/105) = 40 A
Power input = 220 × 40 = 8800 W
Q18) A 230 V shunt motor runs at 1000 rpm at no load and takes 6A. The total armature resistance is 0.2 ohm and field resistance is 210 ohm. Calculate the speed when loaded and taking 40 A. Assume flux constant.
A18) 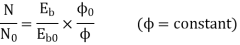
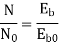
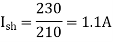
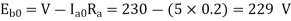

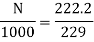
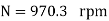
Q19) The armature resistance of a 15 kW, 230 V series motor is 0? 1 ohm, the brush voltage drop is 3 V and series field resistance is 0.05 ohm. The motor takes 60 A speed is 600 rpm. Calculate speed when current is 100 A.
A19)
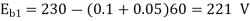
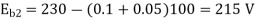
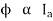
Φ1  60
60
Φ2  100
100
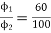
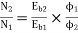
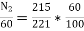
N2=350.22 rpm