Unit – 5
Partial Differential Equations
Q1) What do you understand by PDE?
A1)
A differential equation involving partial derivatives with respect to more than one independent variable is called a partial differential equation.
The independent variables will be denoted by x and y and the dependent variable by z. The partial differential coefficients are denoted as-
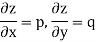
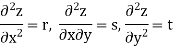
ORDER of a partial differential equation is the same as that of the order of the highest differential coefficient in it.
Classification of the partial differential equation-
Suppose the equation is-
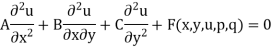
Here A, B, C are the constants of x and y, then the equation-
1. Elliptical- if 
2. Parabolic- if 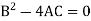
3. Hyperbolic- if if 
Q2) Form a partial differential equation from-
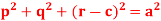
A2)
Here we have-
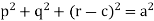
It contains two arbitrary constants a and c
Differentiate the equation with respect to p, we get-
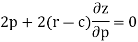
Or
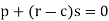
Now differentiate the equation with respect to q, we get-
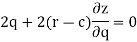
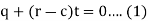
Now eliminate ‘c’,
We get
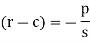
Now put z-c in (1), we get-
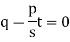
Or
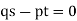
Q3) Solve-
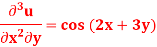
A3)
Here we have-
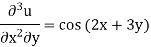
Integrate w.r.t. x, we get-
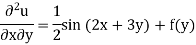
Integrate w.r.t. x, we get-
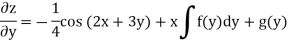
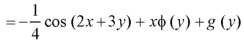
Integrate w.r.t. y, we get-
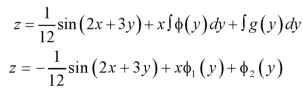
Q4) Solve the differential equation-
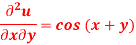
Given that 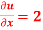 when y = 0, and u =
when y = 0, and u = 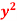 when x = 0.
when x = 0.
A4)
We have-
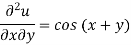
Integrating partially w.r.t. y, we get-
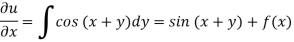
Now from the boundary conditions, 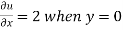
Then-
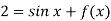
From which,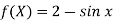
It means,
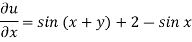
On integrating partially w.r.t. x givens-
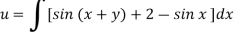
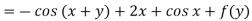
From the boundary conditions, u =  when x = 0
when x = 0
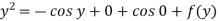
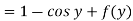
From which-
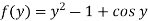
Therefore, the solution of the given equation is-
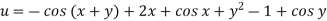
Q5) What is Lagrange’s Linear equation?
A5)
A linear partial differential equation of the first order, commonly known as Lagrange’s Linear equation is of the form
Pp + Qq = R (1)
Where, P, Q, and R are functions of x, y, z. This equation is called a quast linear equation. When P, Q, and R are independent of z it is known as a linear equation.
Q6) Solve 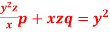
A6)
Rewriting the given equation as
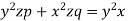
The subsidiary equations are 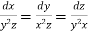
The first two fractions give 
Integrating we get 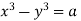 n(i)
n(i)
Again the first and third fraction give xdx = zdz
Integrating, we get 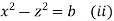
Hence from (i) and (ii), the complete solution is
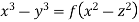
Q7) Solve 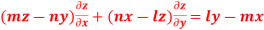
A7)
Here the subsidiary equations are 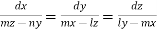
Using multipliers x,y, and z we get each fraction = 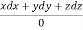
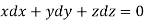 which on integration gives
which on integration gives 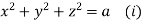
Again using multipliers l, m and n we get each fraction 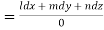
 which on integration gives lx +my +nz = b (ii)
which on integration gives lx +my +nz = b (ii)
Hence from (i) and (ii), the required solution is 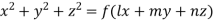
Q8) Solve-
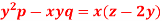
A8)
We have-
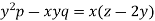
Then the auxiliary equations are-
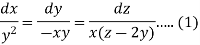
Consider the first two equations only-
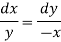
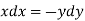
On integrating
 …….. (2)
…….. (2)
Now consider the last two equations-
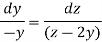
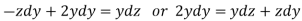
On integrating we get-
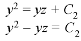 …………… (3)
…………… (3)
From equation (2) and (3)-
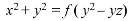
Q9) Solve-
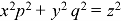
A9)
This equation can be transformed as-
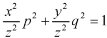
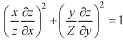
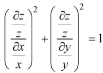 ………. (1)
………. (1)
Let
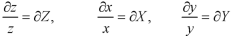
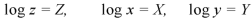
Equation (1) can be written as-
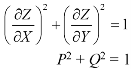 ………… (2)
………… (2)
Let the required solution be-
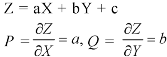
From (2) we have-
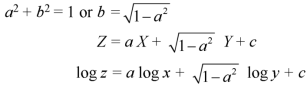
Q10) Solve-
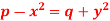
A10)
Let-
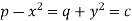
That means-
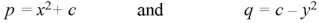
Put these values of p and q in
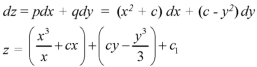
Q11) Solve-

A11)
Let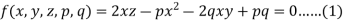
Charpit’s subsidiary equations are-
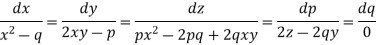
So that- dq = 0 or q = a
On putting q = a in (1) we get-
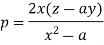
Such that-
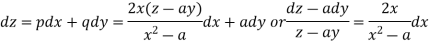
Integrating
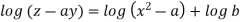
Or
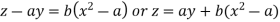
Which is the required solution.
Q12) Solve-

A12)
Let
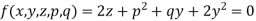
Charpit’s subsidiary equations are-
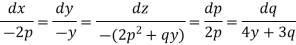
From the first and fourth ratios,
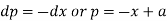
Substituting p = a – x in the given equation, we get-
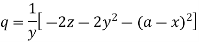
So that-
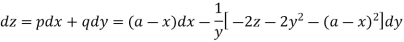
Multiply both sides by 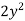 ,
,
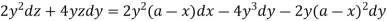
Integrating-
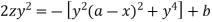
Or
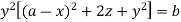
Which is the required solution.
Q13) What is D'Alembert's solution of the wave equation?
A13)
The method of d'Alembert provides a solution to the one-dimensional wave equation
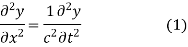
That models vibrations of a string.
The general solution can be obtained by introducing new variables 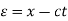 and
and 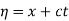 , and applying the chain rule to obtain
, and applying the chain rule to obtain
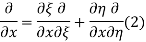
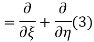
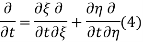

Using (4) and (5) to compute the left and right sides of (3) then gives

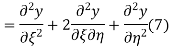

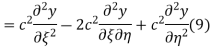
Respectively, so plugging in and expanding then gives
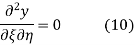
This partial differential equation has general solution
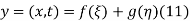
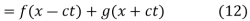
Where f and g are arbitrary functions, with f representing a right-traveling wave and g a left-traveling wave.
The initial value problem for a string located at position 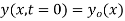 as a function of distance along the string x and vertical speed
as a function of distance along the string x and vertical speed 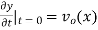 can be found as follows. From the initial condition and (12),
can be found as follows. From the initial condition and (12),
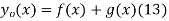
Taking the derivative with respect to 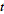 then gives
then gives
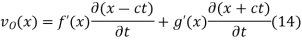
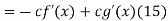
And integrating gives

Solving (13) and (16) simultaneously for f and g immediately gives
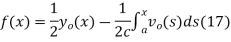
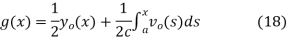
So plugging these into (13) then gives the solution to the wave equation with specified initial conditions as
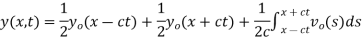
Q14) Find the deflection of a vibrating string of unit length having fixed ends with initial velocity zero and initial deflection f (x)=k (sinx –sin2x)
A14)
By d’Alembert’s method, the solution is
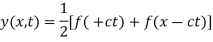

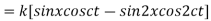
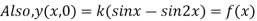
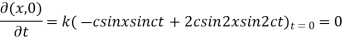
i.e., the given boundary corrections are satisfied.
Q15) Find the deflection u(x,y,t) of the square membrane with a = b = 1 and c = 1. If the initial velocity is zero and the initial deflection is f(x, y) =  .
.
A15)
Here taking a = b = 1 and f(x, y) =  in equation above (5)-
in equation above (5)-
We get-
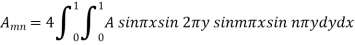
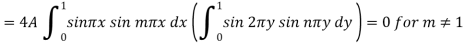
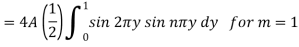

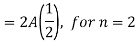
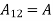
Also from equation (3) above-
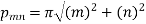
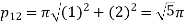
Therefore from equation (4),
The solution will be-
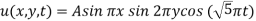
Q16) A rod of length 1 with insulated sides is initially at a uniform temperature u. Its ends are suddenly cooled to 0° Celsius and are kept at that temperature. Prove that the temperature function u (x, t) is given by
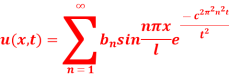
Where  is determined from the equation.
is determined from the equation.
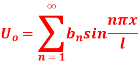
A16)
Let the equation for the conduction of heat be
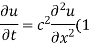
Let us assume that u = XT, where X is a function of x alone and T that of t alone.
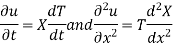
Substituting these values in (1) we get 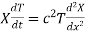
i.e. 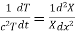
Let each side be equal to a constant 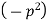
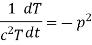
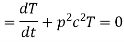
And 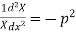
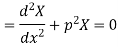
Solving (3) and (4) we have
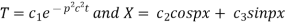
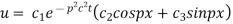
Putting x = 0, u = 0 in (5), we get
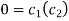
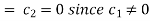
(5) becomes 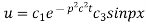
Again putting x = l, u =0 in (6), we get
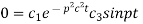
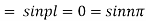
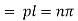
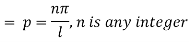
Hence (6) becomes 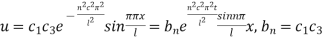
This equation satisfies the given conditions for all integral values n. Hence taking n = 1, 2, 3,…, the most general solution is
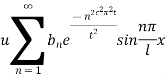
By initial conditions 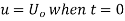
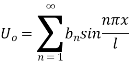
Q17) The ends A and B of a rod 20 cm long having the temperature at 30 degree Celsius and at 80 degree Celsius until steady state prevails. The temperature of the ends are changed to 40 degree Celsius and 60 degree Celsius respectively. Find the temperature distribution in the rod at time t.
A17)
The initial temperature distribution in the rod is
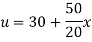
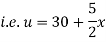
And the final distribution (i.e. steady state) is
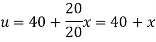
To get u in the intermediate period, reckoning time from the instant when the end temperature were changed we assumed
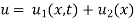
Where 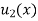 is the steady state temperature distribution in the rod (i.e. temperature after a sufficiently long time) and
is the steady state temperature distribution in the rod (i.e. temperature after a sufficiently long time) and 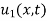 is the transient temperature distribution which tends to zero as t increases.
is the transient temperature distribution which tends to zero as t increases.
Thus, 
Now 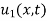 satisfies the one dimensional heat flow equation
satisfies the one dimensional heat flow equation
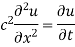
Hence u is of the form
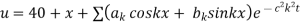
Since 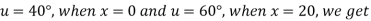
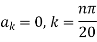
Hence 
Using the initial condition i.e.
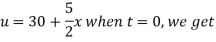
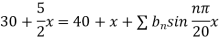
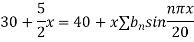
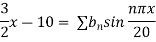
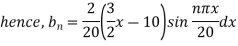

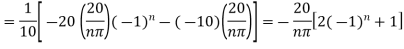
Putting this value of 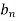 n (1), we get
n (1), we get
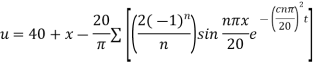
Q18) How do you find the solution of Laplace’s equation.
A18)
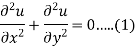
Let
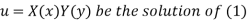
Put the value in (1), we get-
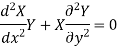
Separating the variables-
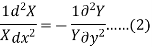
Since x and y are the independent variables, equation (2) can hold good only if each side of (2) is equal to a constant (k),
Then (2) leads the ordinary differential equation-
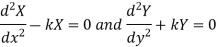
On solving these equations, we get-
- When k is positive and it is equals to
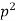 , say
, say
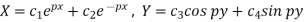
2. When k is negative and it is equals to 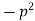 , say
, say
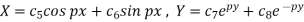
3. When k is zero-
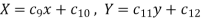
Q19) Using the method of separation of variables, solve
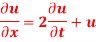
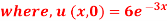
A19)
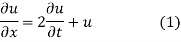
Let, u = X(x). T (t). (2)
Where X is a function of x only and T is a function of t only.
Putting the value of u in (1), we get
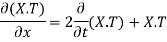
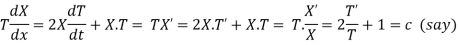
(a) 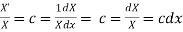
On integration log X = cx + log a = log 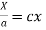
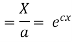
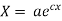
(b) 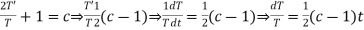
On integration 
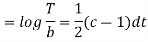

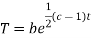
Putting the value of X and T in (2) we have
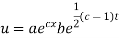
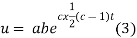
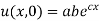
But, 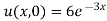
i.e. 
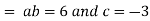
Putting the value of a b and c in (3) we have
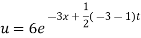
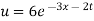
Which is the required solution.
Q20) Using the method of separation of variables, solve 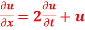 Where
Where 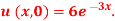
A20)
Assume the given solution 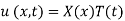
Substituting in the given equation, we have

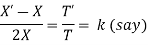
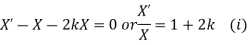
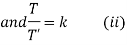
Solving (i) 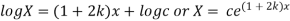
From (ii) 
Thus 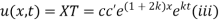
Now, 
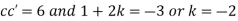
Substituting these values in (iii) we get
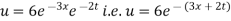
Which is the required solution.