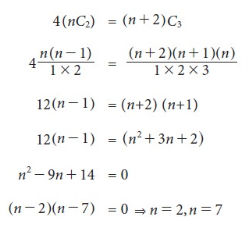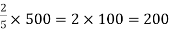 B’s share =
B’s share = 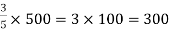 Continued ratio-A ratio is said to be in continued if consequent of one ratio is antecedent of the other.For example- a:b and b:c is in continued ratio. Example: If Rs. 74000 are to be divided among three people X, Y and Z such that
Continued ratio-A ratio is said to be in continued if consequent of one ratio is antecedent of the other.For example- a:b and b:c is in continued ratio. Example: If Rs. 74000 are to be divided among three people X, Y and Z such thatX : Y = 4 : 5 and Y : Z = 3 : 2 then what amount each person will recieve?Sol.Here X:Y:Z: = 12:15:10Here sum of the ratio is = 12 + 15 + 10 = 37Then-X will get =
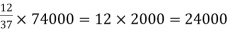 Y will get =
Y will get = 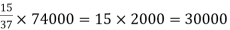 Z will get =
Z will get =  Inverse ratio- For the ratio x : y, the inverted ratio will be y:x.Example: inverse ratio of 3:4 is 4:3 Note- A ratio remains same if it is multiplied or divided by the same number.X: y = mx : my ( multiplied by ‘m’)X:y = x/m:y/m (divided by m)Example:4:5 =
Inverse ratio- For the ratio x : y, the inverted ratio will be y:x.Example: inverse ratio of 3:4 is 4:3 Note- A ratio remains same if it is multiplied or divided by the same number.X: y = mx : my ( multiplied by ‘m’)X:y = x/m:y/m (divided by m)Example:4:5 = 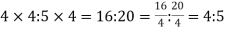 Proportion-Proportion is an equation that equates two ratios in same proportion,If the ratio a/b is equals to the ratio c/d then the following proportion can be written-
Proportion-Proportion is an equation that equates two ratios in same proportion,If the ratio a/b is equals to the ratio c/d then the following proportion can be written-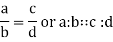 Here a and d are called the extremes of the proportion, the numbers b and c are called means of the proportion Q2) Explain proportion and properties of proportion with examples.A2) Properties of proportion-
Here a and d are called the extremes of the proportion, the numbers b and c are called means of the proportion Q2) Explain proportion and properties of proportion with examples.A2) Properties of proportion-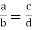 then ad = bc2. Reciprocal property: If two ratios are equal, then their reciprocals are also equal.If
then ad = bc2. Reciprocal property: If two ratios are equal, then their reciprocals are also equal.If 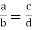 then If
then If 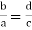 . Continued proportion-The quantities p, q, r, s, t..... are said to be in continued proportion of p:q = q:r = r:s..... Example: If 2, x and 32 are in continued proportion then find the value of x.Sol.Here it can be written as-2 : x = x:32Or
. Continued proportion-The quantities p, q, r, s, t..... are said to be in continued proportion of p:q = q:r = r:s..... Example: If 2, x and 32 are in continued proportion then find the value of x.Sol.Here it can be written as-2 : x = x:32Or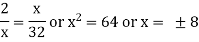 Note- if a, b, c are in continued proportion then
Note- if a, b, c are in continued proportion then 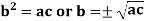 Fourth, third and mean proportional-If p:q = r:s then-
Fourth, third and mean proportional-If p:q = r:s then-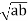 is called the mean proportional between a and b.
is called the mean proportional between a and b. Then
Then 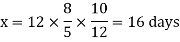 Important properties of proportion-Given quantities a, b, c, d are in proportion.
Important properties of proportion-Given quantities a, b, c, d are in proportion.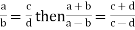
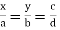 is sometimes written as x : y : z = a : b : c.
is sometimes written as x : y : z = a : b : c.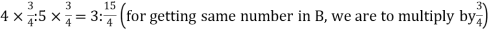 C : D = 7 : 9 =
C : D = 7 : 9 =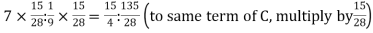 A : B : C : D = 2 : 3 :
A : B : C : D = 2 : 3 :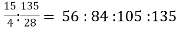 Q3) Two numbers are in the ratio of 3 : 5 and if 10 be subtracted from each of them, the remainders are in the ratio of 1 : 5, find the numbers.A3)Let the numbers be x and y, so that- x/y =3/5 or 5x = 3y … (1)Again-
Q3) Two numbers are in the ratio of 3 : 5 and if 10 be subtracted from each of them, the remainders are in the ratio of 1 : 5, find the numbers.A3)Let the numbers be x and y, so that- x/y =3/5 or 5x = 3y … (1)Again- 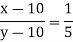 Or5x – y = 40… (2)By solving (1) and(2), we get-X = 12 and y = 20Hence the required numbers are- 12 and 20 Q4) The prime cost of an article was three times the value of material used. The cost of raw materials was increased in the ratio 3 : 4 and the productive wage was increased in the ratio 4 : 5. Find the present prime cost of an article, which could formerly be made for Rs.180. A4)Prime cost = x + y, where x = productive wage, y = material usedNow prime cost = 180 =3y or, y = 60, again x + y = 180, x = 180–y = 180–60 = 120Present material cost = 4y/3 and present wage = 5x / 4Present prime cost–
Or5x – y = 40… (2)By solving (1) and(2), we get-X = 12 and y = 20Hence the required numbers are- 12 and 20 Q4) The prime cost of an article was three times the value of material used. The cost of raw materials was increased in the ratio 3 : 4 and the productive wage was increased in the ratio 4 : 5. Find the present prime cost of an article, which could formerly be made for Rs.180. A4)Prime cost = x + y, where x = productive wage, y = material usedNow prime cost = 180 =3y or, y = 60, again x + y = 180, x = 180–y = 180–60 = 120Present material cost = 4y/3 and present wage = 5x / 4Present prime cost– Hence the present prime cost is Rs. 230. Direct and inverse proportion-Direct proportion or direct variation- Direct proportion or direct variation is the relation between two quantities where the ratio of the two is equal to a constant value. It is represented by the proportional symbol, ∝Or we can say that two values said to be in direct proportion when one variable increases then other value also increases.If we have two quantities ‘a’ and ‘b’ which are lined with each other directly then we can say- a ∝ bWhen we remove proportionality sign, the ratio of ‘a’ and ‘b’ becomes equals to a constant. a = CbFor example: if the number of individuals visiting a restaurant increases, earning of the restaurant also increases. Inverse proportion-Inverse proportion is when one value increases and the other value decreases. For example- We need more manpower to reduce the time to complete a task. They are inversely proportional. Q5) A machine in a drinking water supplying factory fills 840 bottles in six hours. How many bottles will it fill in five hours? A5)Let the bottles filled by machine in 5 hours be xWe can write the given information as follows-
Hence the present prime cost is Rs. 230. Direct and inverse proportion-Direct proportion or direct variation- Direct proportion or direct variation is the relation between two quantities where the ratio of the two is equal to a constant value. It is represented by the proportional symbol, ∝Or we can say that two values said to be in direct proportion when one variable increases then other value also increases.If we have two quantities ‘a’ and ‘b’ which are lined with each other directly then we can say- a ∝ bWhen we remove proportionality sign, the ratio of ‘a’ and ‘b’ becomes equals to a constant. a = CbFor example: if the number of individuals visiting a restaurant increases, earning of the restaurant also increases. Inverse proportion-Inverse proportion is when one value increases and the other value decreases. For example- We need more manpower to reduce the time to complete a task. They are inversely proportional. Q5) A machine in a drinking water supplying factory fills 840 bottles in six hours. How many bottles will it fill in five hours? A5)Let the bottles filled by machine in 5 hours be xWe can write the given information as follows-Number of bottles | Tine taken (in hrs) |
840 | 6 |
X | 5 |
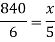
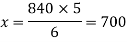 So that 700 bottles can be filled by the machine in 5 hrs. Q6) In a model of a ship, the mast is 9 m high, while the mast of the actual ship is 12 m high. If the length of the ship is 28 m, how long is the model ship?A6)Suppose the mast of the model ship be x, thenWe can write the given information as follows-
So that 700 bottles can be filled by the machine in 5 hrs. Q6) In a model of a ship, the mast is 9 m high, while the mast of the actual ship is 12 m high. If the length of the ship is 28 m, how long is the model ship?A6)Suppose the mast of the model ship be x, thenWe can write the given information as follows-
| Height of mast | Length of ship |
Model ship | 9 m | x |
Actual ship | 12m | 28m |
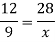
 So that the length of a model ship is 21m. Q7) 10 men can do a piece of work in 30 days then in how many days 20 men can do the same work?A7) Let ‘x’ days be taken to do the task,Then
So that the length of a model ship is 21m. Q7) 10 men can do a piece of work in 30 days then in how many days 20 men can do the same work?A7) Let ‘x’ days be taken to do the task,Then Men | Days |
10 | 30 |
20 | X |
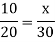
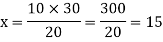 So that 20 men can do the same piece of work in 15 days. Q8) Explain logarithms and its properties.A8) A logarithm is the power to which a number must be raised in order to get some other number. For example, the base ten logarithm of 100 is 2, because ten raised to the power of two is 100:log 100 = 2because
So that 20 men can do the same piece of work in 15 days. Q8) Explain logarithms and its properties.A8) A logarithm is the power to which a number must be raised in order to get some other number. For example, the base ten logarithm of 100 is 2, because ten raised to the power of two is 100:log 100 = 2because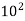 = 100This is an example of a base-ten logarithm. We call it a base ten logarithm because ten is the number that is raised to a power. The base unit is the number being raised to a power. There are logarithms using different base units. If you wanted, you could use two as a base unit. For instance, the base two logarithm of eight is three, because two raised to the power of three equals eight:
= 100This is an example of a base-ten logarithm. We call it a base ten logarithm because ten is the number that is raised to a power. The base unit is the number being raised to a power. There are logarithms using different base units. If you wanted, you could use two as a base unit. For instance, the base two logarithm of eight is three, because two raised to the power of three equals eight: 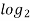 8 = 3because
8 = 3because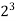 = 8In general, you write log followed by the base number as a subscript. The most common logarithms are base 10 logarithms and natural logarithms; they have special notations. A base ten log is written logand a base ten logarithmic equation is usually written in the form:log a = r A natural logarithm is writtenlnand a natural logarithmic equation is usually written in the form:ln a = rSo, when you see log by itself, it means base ten log. When you see ln, it means natural logarithm (we'll define natural logarithms below). In this course only base ten and natural logarithms will be used. Properties of Logarithms
= 8In general, you write log followed by the base number as a subscript. The most common logarithms are base 10 logarithms and natural logarithms; they have special notations. A base ten log is written logand a base ten logarithmic equation is usually written in the form:log a = r A natural logarithm is writtenlnand a natural logarithmic equation is usually written in the form:ln a = rSo, when you see log by itself, it means base ten log. When you see ln, it means natural logarithm (we'll define natural logarithms below). In this course only base ten and natural logarithms will be used. Properties of Logarithms mn =
mn = 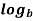 m +
m + 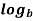 n
n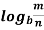 =
= 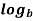 m -
m - 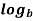 n
n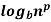 = p
= p 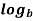 n
n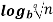 =
= 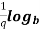 n
n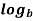 n =
n = 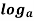 n
n 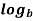 a
a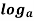 1 = 0
1 = 0
|
log x = -2.0258 = - 3 + 3 - 2.0258
= - 3 + .9742 =3.9742
Therefore, x = antilog 3.9742.
Now, from antilog table we get the number corresponding to the mantissa
.9742 as (9419 + 4) = 9423.
Again the characteristic in log x is (- 3).
Hence, there should be two zeroes between the decimal point and the first significant digit in the value of x.
Therefore, x = .009423. Q24) What do you understand by percentage? Explain with examples.A24) Percent means a part per 100. A percentage is a number that can be expressed as fraction of 100.We use the symbol % for percentage.We can represent percentage in decimal or fraction form.We use the following formula to calculate percentage-
 For example: if a student got 300 marks out of 500 then his result in percentage will be-
For example: if a student got 300 marks out of 500 then his result in percentage will be-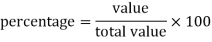
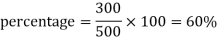
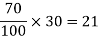 Example: A person has a monthly salary of Rs. 20,000. He spends 8000 per month on food then what percent of his monthly salary does he save?Sol.Person’s monthly salary = 20,000Savings = (20,000 – 8000) = 12,000Fraction of his saving = 12,000/20,000By converting the fraction into percentage, we get-
Example: A person has a monthly salary of Rs. 20,000. He spends 8000 per month on food then what percent of his monthly salary does he save?Sol.Person’s monthly salary = 20,000Savings = (20,000 – 8000) = 12,000Fraction of his saving = 12,000/20,000By converting the fraction into percentage, we get-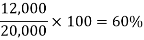 Example: A shopkeeper reduced the price of an item by 25%, the old price was 120 then find out the new price.Sol.25% of 120 will be-
Example: A shopkeeper reduced the price of an item by 25%, the old price was 120 then find out the new price.Sol.25% of 120 will be- Which means he reduced Rs. 30, then the new price is-120 – 30 = 90The new price is Rs. 90 Example: If 20% of any number is 500 then find out the number?Sol.Let the number is ‘x’, then-
Which means he reduced Rs. 30, then the new price is-120 – 30 = 90The new price is Rs. 90 Example: If 20% of any number is 500 then find out the number?Sol.Let the number is ‘x’, then-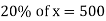
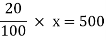
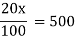
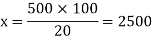 Hence the number is 2500. decrease in number = Original number – New number Q25) Explain Average.A25)Mean
Hence the number is 2500. decrease in number = Original number – New number Q25) Explain Average.A25)Mean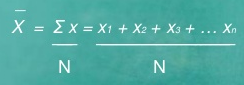 Computation of the mean for ungrouped data
Computation of the mean for ungrouped data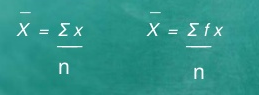 Example 1 – The marks obtained in 10 class test are 25, 10, 15, 30, 35
Example 1 – The marks obtained in 10 class test are 25, 10, 15, 30, 35
 The mean = X = 25+10+15+30+35 = 115 =23 5 5Analysis – The average performance of 5 students is 23. The implication is that students who got below 23 did not perform well. The students who got above 23 performed well in exam. Example 2 – Find the mean
The mean = X = 25+10+15+30+35 = 115 =23 5 5Analysis – The average performance of 5 students is 23. The implication is that students who got below 23 did not perform well. The students who got above 23 performed well in exam. Example 2 – Find the meanXi | 9 | 10 | 11 | 12 | 13 | 14 | 15 |
Freq (Fi) | 2 | 5 | 12 | 17 | 14 | 6 | 3 |
Xi | Freq (Fi) | XiFi |
9 | 2 | 18 |
10 | 5 | 50 |
11 | 12 | 132 |
12 | 17 | 204 |
13 | 14 | 182 |
14 | 6 | 84 |
15 | 3 | 45 |
| Fi = 59 | XiFi= 715 |
|
|
|

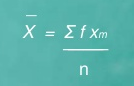
 Where, X = Mean Xm = midpoint of each class or category f = frequency in each class or category ∑f Xm = summation of the product of fXm Example 3 – the following data represent the income distribution of 100 families. Calculate mean income of 100 families?
Where, X = Mean Xm = midpoint of each class or category f = frequency in each class or category ∑f Xm = summation of the product of fXm Example 3 – the following data represent the income distribution of 100 families. Calculate mean income of 100 families?Income | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 | 90-100 |
No. of families | 8 | 12 | 25 | 22 | 16 | 11 | 6 |
Income | No. of families | Xm (Mid point) | fXm |
30-40 | 8 | 35 | 280 |
40-50 | 12 | 34 | 408 |
50-60 | 25 | 55 | 1375 |
60-70 | 22 | 65 | 1430 |
70-80 | 16 | 75 | 1200 |
80-90 | 11 | 85 | 935 |
90-100 | 6 | 95 | 570 |
| n = 100 |
| ∑f Xm = 6198 |
 X = ∑f Xm/n = 6330/100 = 63.30Mean = 63.30 Q26) Calculate the mean number of hours per week spent by each student in texting message.
X = ∑f Xm/n = 6330/100 = 63.30Mean = 63.30 Q26) Calculate the mean number of hours per week spent by each student in texting message.Time per week | 0 - 5 | 5 - 10 | 10 - 15 | 15 - 20 | 20 - 25 | 25 – 30 |
No. of students | 8 | 11 | 15 | 12 | 9 | 5 |
Time per week (X) | No. of students (F) | Mid point X | XF |
0 - 5 | 8 | 2.5 | 20 |
5 – 10 | 11 | 7.5 | 82.5 |
10 - 15 | 15 | 12.5 | 187.5 |
15 - 20 | 12 | 17.5 | 210 |
20 - 25 | 9 | 22.5 | 202.5 |
25 – 30 | 5 | 27.5 | 137.5 |
| 60 |
| 840 |
Weight (pounds) | Number of Babies |
[3−5) | 8 |
[5−7) | 25 |
[7−9) | 45 |
[9−11) | 18 |
[11−13) | 4 |
Weight (pounds) | Number of Babies | Mid point X | XF |
[3−5) | 8 | 4 | 32 |
[5−7) | 25 | 6 | 150 |
[7−9) | 45 | 8 | 360 |
[9−11) | 18 | 10 | 180 |
[11−13) | 4 | 12 | 48 |
| 100 |
| 770 |
class | frequency |
2-4 | 3 |
4-6 | 4 |
6-8 | 2 |
8-10 | 1 |
class | frequency | x | Log x | flogx |
2-4 | 3 | 3 | 1.0986 | 3.2958 |
4-6 | 4 | 5 | 1.2875 | 6.4378 |
6-8 | 2 | 7 | 0.5559 | 3.8918 |
8-10 | 1 | 9 | 0.2441 | 2.1972 |
| 10 |
|
| 15.8226 |
 GM = Antilog ∑ f logxi N = antilog 15.8226/10 = antilog 1.5823 GM = 4.866 Q29) Calculate harmonic mean
GM = Antilog ∑ f logxi N = antilog 15.8226/10 = antilog 1.5823 GM = 4.866 Q29) Calculate harmonic meanclass | frequency |
2-4 | 3 |
4-6 | 4 |
6-8 | 2 |
8-10 | 1 |
class | frequency | x | f/x |
2-4 | 3 | 3 | 1 |
4-6 | 4 | 5 | 0.8 |
6-8 | 2 | 7 | 0.28 |
8-10 | 1 | 9 | 0.11 |
| 10 |
| 2.19 |
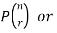 Example 1: Consider the three letters x, y, z. The arrangements of the letter x, y, ztaken two at a time are- xy, yx, xz, zx, yz, zy ∴The number of 2-arrangements are 6 i.e., the number of permutation of 3 letters taken 2 at a time
Example 1: Consider the three letters x, y, z. The arrangements of the letter x, y, ztaken two at a time are- xy, yx, xz, zx, yz, zy ∴The number of 2-arrangements are 6 i.e., the number of permutation of 3 letters taken 2 at a time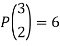 Note-A permutation of n objects taken r at a time is also called r-permutation Factorial function-The product of the positive integers from 1 to n is denoted by n!and we read it as “factorial “Expressed as-
Note-A permutation of n objects taken r at a time is also called r-permutation Factorial function-The product of the positive integers from 1 to n is denoted by n!and we read it as “factorial “Expressed as-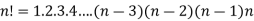 Note-
Note- 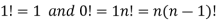 Binomial coefficient-
Binomial coefficient- Example: 1.
Example: 1. 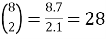
 Permutation-The arrangement of a set of n objects in a given order is called a permutation.Any arrangement of any
Permutation-The arrangement of a set of n objects in a given order is called a permutation.Any arrangement of any  of these objects in a given order is said to be r-permutation.The number of permutations of n objects taken r will be denoted as-P(n, r)Formula-
of these objects in a given order is said to be r-permutation.The number of permutations of n objects taken r will be denoted as-P(n, r)Formula- 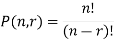 Permutation with repetitions-The number of permutations of n objects of which are
Permutation with repetitions-The number of permutations of n objects of which are  are alike,
are alike,  are alike,
are alike, 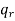 are alike is-
are alike is-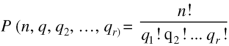 Sampling with or without replacement-Suppose we chose the samples with repetition, from example if we draw a ball from a urn then we put back that ball in the urn and again we pick a ball and we continue the process, so this is the case of sampling with repetitionThen the product rule tells us that the number of such samples is-n.n.n.n.n……….n.n =
Sampling with or without replacement-Suppose we chose the samples with repetition, from example if we draw a ball from a urn then we put back that ball in the urn and again we pick a ball and we continue the process, so this is the case of sampling with repetitionThen the product rule tells us that the number of such samples is-n.n.n.n.n……….n.n =  And if we pick a ball from the urn and we do not put it back to the urn, then this is the case of sampling without replacement.So that in this case the number of samples are given as-
And if we pick a ball from the urn and we do not put it back to the urn, then this is the case of sampling without replacement.So that in this case the number of samples are given as-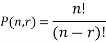 Example-Three cards are chosen one after the other from a 52-card deck. Find the number m of waysThis can be done: (a) with replacement; (b) without replacement. Sol. (a) Each card can be chosen in 52 ways. Thus m = 52(52)(52) = 140 608. (b) Here there is no replacement. Thus the first card can be chosen in 52 ways, the second in 51 ways, and the third in 50 ways. So that- P(52, 3) = 52(51)(50) = 132 600 Example-There are 4 black, 3 green and 5 red balls. In how many ways can they be arranged in a row? Solution: Total number of balls = 4 black + 3 green + 5 red = 12 The black balls are alike, The green balls are, and the red balls are alike, The number of ways in which the balls can be arranged in a row =
Example-Three cards are chosen one after the other from a 52-card deck. Find the number m of waysThis can be done: (a) with replacement; (b) without replacement. Sol. (a) Each card can be chosen in 52 ways. Thus m = 52(52)(52) = 140 608. (b) Here there is no replacement. Thus the first card can be chosen in 52 ways, the second in 51 ways, and the third in 50 ways. So that- P(52, 3) = 52(51)(50) = 132 600 Example-There are 4 black, 3 green and 5 red balls. In how many ways can they be arranged in a row? Solution: Total number of balls = 4 black + 3 green + 5 red = 12 The black balls are alike, The green balls are, and the red balls are alike, The number of ways in which the balls can be arranged in a row =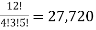 Example- A box contains 10 light bulbs. Find the number n of ordered samples of:(a) Size 3 with replacement, (b) Size 3 without replacement. Solution:(a) n=
Example- A box contains 10 light bulbs. Find the number n of ordered samples of:(a) Size 3 with replacement, (b) Size 3 without replacement. Solution:(a) n= 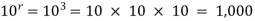 (b) P (10, 3) = 10 × 9 × 8 = 720 Circular permutations-A circular permutation of n objects is an arrangement of the objects around a circle.If the n objects are to be arranged round a circle we take an objects and fix it in one position. Now the remaining (n – 1) objects can be arranged to fill the (n – 1) positions the circle in (n – 1)! ways.Hence the number of circular permutations of n different objects = (n – 1)! Combinations-A combination of n objects taken at a time is an unordered selection of r of the nObjects (r ≤n). A combination of n objects taken r at a time is also called r-combination of n objects.The number of combinations is given by-C(n, r) Note-1. property-1
(b) P (10, 3) = 10 × 9 × 8 = 720 Circular permutations-A circular permutation of n objects is an arrangement of the objects around a circle.If the n objects are to be arranged round a circle we take an objects and fix it in one position. Now the remaining (n – 1) objects can be arranged to fill the (n – 1) positions the circle in (n – 1)! ways.Hence the number of circular permutations of n different objects = (n – 1)! Combinations-A combination of n objects taken at a time is an unordered selection of r of the nObjects (r ≤n). A combination of n objects taken r at a time is also called r-combination of n objects.The number of combinations is given by-C(n, r) Note-1. property-1 2. property-2
2. property-2 3. property-3
3. property-3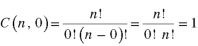 Example: From a class of 3 students, 4 students are to be chosen for competition, in how many ways they can be selected?Sol.The number of combinations will be-
Example: From a class of 3 students, 4 students are to be chosen for competition, in how many ways they can be selected?Sol.The number of combinations will be-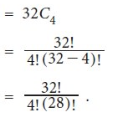 Example:
Example: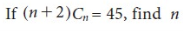 Sol.
Sol.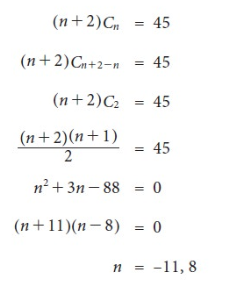 n = -11 is not possible,therefore-n = 8 Example:
n = -11 is not possible,therefore-n = 8 Example: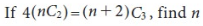 Sol.
Sol.