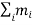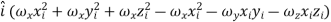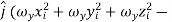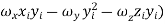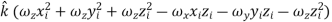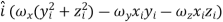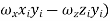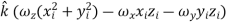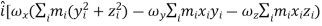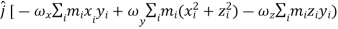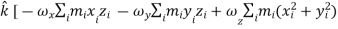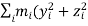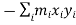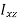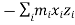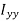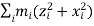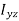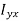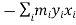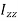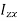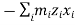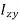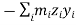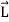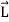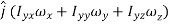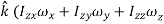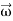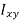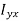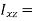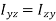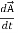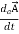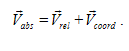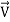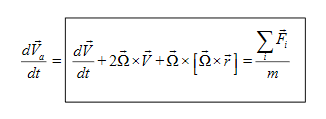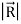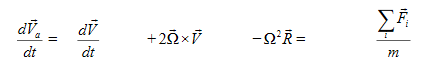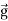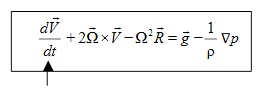|
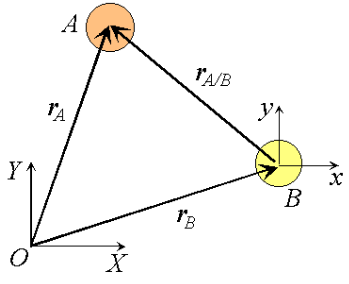
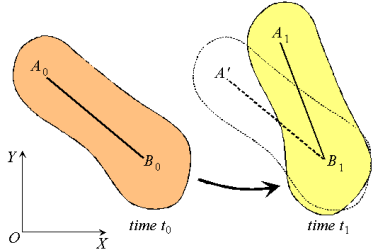
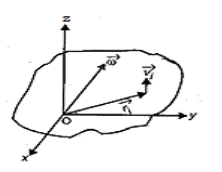
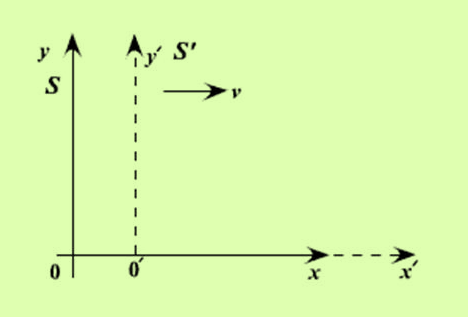
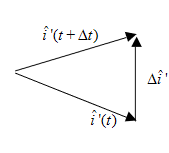
Figure 12: Observation of particle motion using a translating referenceConsider two particles A and B moving along independent trajectories in the plane, and a fixed reference O. Let rA and rB be the positions of particles A and B in the fixed reference. Instead of observing the motion of particle A relative to the fixed reference as we have done in the past, we will attach a non-rotating reference to particle B and observe the motion of A relative to the moving reference at B. Let i and j be basis vectors of the moving reference, then the position vector of A relative to the reference at B, denoted rA/B is,rA/B =xi +yj where the subscript stands for "A with respect to B" or "A relative to B". Observe that, as the moving frame does not rotate, basis vectors i and j do not change in time. Therefore, taking time derivatives, we obtain simply,

 which can be interpreted as the velocity of A relative to B. Now we can express the absolute position vector of A as,
which can be interpreted as the velocity of A relative to B. Now we can express the absolute position vector of A as,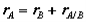 Differentiating the equation in time to obtain expressions for the absolute velocity and acceleration of particle A:
Differentiating the equation in time to obtain expressions for the absolute velocity and acceleration of particle A: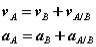 or the absolute velocity of A equals the absolute velocity of B plus the velocity of A relative to B, vA/B , and similarly for the acceleration. The relative terms are the velocity or acceleration measured by an observer attached to the moving reference at particle B.
or the absolute velocity of A equals the absolute velocity of B plus the velocity of A relative to B, vA/B , and similarly for the acceleration. The relative terms are the velocity or acceleration measured by an observer attached to the moving reference at particle B.
|
 By comparison with expressions derived previously
By comparison with expressions derived previously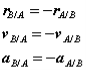 We proceed by demonstrating that every motion of a planar rigid body is associated with a single angular velocity ω and angular acceleration
We proceed by demonstrating that every motion of a planar rigid body is associated with a single angular velocity ω and angular acceleration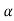 , describing the angular displacement of an arbitrary line inscribed in the body relative to a fixed direction.
, describing the angular displacement of an arbitrary line inscribed in the body relative to a fixed direction.
|
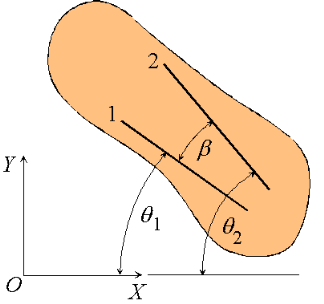
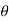 1and
1and  2 measured relative to any convenient fixed reference direction. These are related to the intermediate angle
2 measured relative to any convenient fixed reference direction. These are related to the intermediate angle 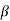 shown as,
shown as,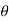 2 =
2 = 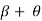 1Observe that as the body is rigid, requiring that the distance between each pair of points on the two lines 1 and 2 is constant, angle
1Observe that as the body is rigid, requiring that the distance between each pair of points on the two lines 1 and 2 is constant, angle 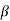 must be invariant. Differentiating the relation above with this in mind,
must be invariant. Differentiating the relation above with this in mind,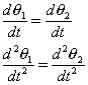 These hold for arbitrary lines attached to the body, implying in turn that the body can be associated with a unique angular velocity ω, defined as,
These hold for arbitrary lines attached to the body, implying in turn that the body can be associated with a unique angular velocity ω, defined as,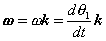 for an arbitrary line attached to the body. Arguing analogously, the body can be associated with a unique angular acceleration
for an arbitrary line attached to the body. Arguing analogously, the body can be associated with a unique angular acceleration 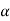 defined as,
defined as,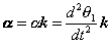
|
|
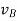 and acceleration
and acceleration 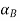 of the reference point, the rotational motion is characterized by the unique angular velocity ω and angular acceleration
of the reference point, the rotational motion is characterized by the unique angular velocity ω and angular acceleration 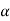 associated with the body. Thus, we have the property that the motion of a rigid body can be decomposed into a translation of an arbitrary point within the body, followed by a rigid rotation of the body about this point. Further, the motion of an arbitrary point within the body is determined completely once the translational quantities
associated with the body. Thus, we have the property that the motion of a rigid body can be decomposed into a translation of an arbitrary point within the body, followed by a rigid rotation of the body about this point. Further, the motion of an arbitrary point within the body is determined completely once the translational quantities 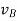 and
and 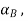 and rotational quantities ω and
and rotational quantities ω and 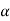 are known.Characteristics of rigid body motion:
are known.Characteristics of rigid body motion: 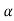 .
.
|
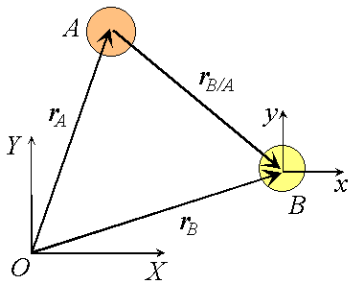
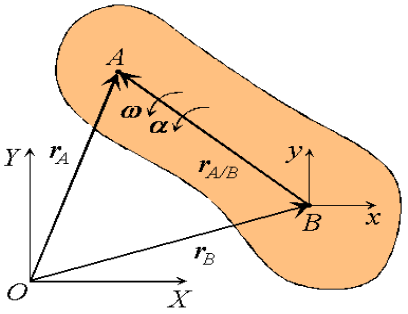
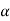 , and two arbitrary points A and B of the body. We will examine the motion of this body in both, the fixed reference O shown, as well as relative to a non-rotating reference attached to point B. Proceeding, we express the absolute position of point A in terms of the absolute position of point B as,
, and two arbitrary points A and B of the body. We will examine the motion of this body in both, the fixed reference O shown, as well as relative to a non-rotating reference attached to point B. Proceeding, we express the absolute position of point A in terms of the absolute position of point B as,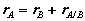 where rA/B is the position of A relative to B. An analogous expression for absolute velocities follows by taking time derivatives,
where rA/B is the position of A relative to B. An analogous expression for absolute velocities follows by taking time derivatives,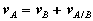 where vA/B is the velocity of point A relative to the reference at B,
where vA/B is the velocity of point A relative to the reference at B,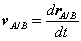
|
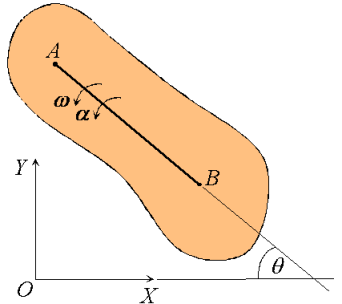
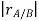 relative to point B, keeping the distance between the two unchanged. The angular velocity of this motion is simply the angular velocity ω of the rigid body. Then, using results derived previously for the time derivatives of rotating vectors we have:
relative to point B, keeping the distance between the two unchanged. The angular velocity of this motion is simply the angular velocity ω of the rigid body. Then, using results derived previously for the time derivatives of rotating vectors we have: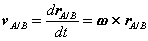 or,
or,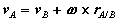 Observe that the expression reflects the decomposition of rigid body motion referred to previously. With B chosen as reference, the velocity of A is the vector sum of a translational portion vB and a rotational portion ω
Observe that the expression reflects the decomposition of rigid body motion referred to previously. With B chosen as reference, the velocity of A is the vector sum of a translational portion vB and a rotational portion ω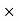 rA/B .Proceeding to derive expressions for the acceleration of an arbitrary point of a rigid body, we differentiate the equation for velocities to obtain,
rA/B .Proceeding to derive expressions for the acceleration of an arbitrary point of a rigid body, we differentiate the equation for velocities to obtain,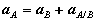 Where
Where 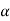 A/B , the acceleration of point A relative to the reference at B, is simply
A/B , the acceleration of point A relative to the reference at B, is simply or,
or, Thus, like the absolute velocity, the absolute acceleration of point A is the vector sum of a translational portion
Thus, like the absolute velocity, the absolute acceleration of point A is the vector sum of a translational portion 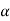 B and a rotational portion
B and a rotational portion 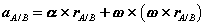 .
. mv2In pure rotation, however, the rigid body has no "over all" translation of the body. However, the body in rotation must have kinetic energy as it involves certain motion. A closer look on the rotation of rigid body reveals that though we may not be able to assign translation to the rigid body as a whole, but we can recognize translation of individual particles as each of them rotate about the axis in circular motion with different linear speeds. The speed of a particle is given by:
mv2In pure rotation, however, the rigid body has no "over all" translation of the body. However, the body in rotation must have kinetic energy as it involves certain motion. A closer look on the rotation of rigid body reveals that though we may not be able to assign translation to the rigid body as a whole, but we can recognize translation of individual particles as each of them rotate about the axis in circular motion with different linear speeds. The speed of a particle is given by:
|
 mivi2where " Ki" is the kinetic energy of "i" th particle having a speed " vi". In terms of angular speed, the kinetic energy of an individual particle is: Ki=
mivi2where " Ki" is the kinetic energy of "i" th particle having a speed " vi". In terms of angular speed, the kinetic energy of an individual particle is: Ki= mi(ωri2Ki=
mi(ωri2Ki= mi(ωri)2Now, the kinetic energy of the rigid body is sum of the kinetic energies of the particles constituting the rigid body: K=∑Ki=∑
mi(ωri)2Now, the kinetic energy of the rigid body is sum of the kinetic energies of the particles constituting the rigid body: K=∑Ki=∑ mi(ωri)2We note here that angular speeds of all particles constituting the body are same. Hence, the constant "1/2" and " ω2" can be taken out of the summation sign : K =
mi(ωri)2We note here that angular speeds of all particles constituting the body are same. Hence, the constant "1/2" and " ω2" can be taken out of the summation sign : K = ω2∑mi(ri)2However, we know that: I=∑mi(ri)2Combining two equations, we have: K=
ω2∑mi(ri)2However, we know that: I=∑mi(ri)2Combining two equations, we have: K=  Iω2This is the desired expression of kinetic energy of a rigid body rotating about a fixed axis i.e. in pure rotational motion. The form of expression of the kinetic energy here emphasizes the correspondence between linear and angular quantities. Comparing with the expression of kinetic energy for translational motion, we find that "moment of inertia (I)" corresponds to "mass (m)" and "linear speed (v)" corresponds to "angular speed (ω)". We can also interpret the result obtained above from a different perspective. We could have directly inferred that expression of kinetic energy in rotation should have an equivalent form as: K (Kinetic energy)=
Iω2This is the desired expression of kinetic energy of a rigid body rotating about a fixed axis i.e. in pure rotational motion. The form of expression of the kinetic energy here emphasizes the correspondence between linear and angular quantities. Comparing with the expression of kinetic energy for translational motion, we find that "moment of inertia (I)" corresponds to "mass (m)" and "linear speed (v)" corresponds to "angular speed (ω)". We can also interpret the result obtained above from a different perspective. We could have directly inferred that expression of kinetic energy in rotation should have an equivalent form as: K (Kinetic energy)=  x(inertia)x(speed)2In rotation, inertia to the rotation is "moment of inertia (I)" and speed of the rigid body is "angular speed (ω)". Substituting for the quantities, we have the expression for kinetic energy of rigid body in rotation as : K=
x(inertia)x(speed)2In rotation, inertia to the rotation is "moment of inertia (I)" and speed of the rigid body is "angular speed (ω)". Substituting for the quantities, we have the expression for kinetic energy of rigid body in rotation as : K=  Iω2Comparing this equation with the expression of the sum of kinetic energy of individual particles as derived earlier: K =
Iω2Comparing this equation with the expression of the sum of kinetic energy of individual particles as derived earlier: K = ω2∑mi(ri)2Clearly, I= ∑mi(ri)2This conclusion, thus, clearly establishes that the expression as given by ∑mi(ri)2 indeed represents the inertia of the rigid body in rotation. Q4) Explain the rotation in plane also derive the conditions of the instantaneous velocity vector of any particle is always perpendicular to its instantaneous position vector?A 4) When all parts of a rigid body move parallel to a fixed plane, then the motion of the object is referred to as plane motion. There are two types of plane motion, which are given as follows:The pure rotational motion: The rigid body in such a motion rotates about a fixed axis that is perpendicular to a fixed plane. In other words, the axis is fixed and does not move or change its direction relative to an inertial frame of reference. The general plane motion: The motion here can be considered as a combination of pure translational motion parallel to a fixed plane in addition to a pure rotational motion about an axis that is perpendicular to that plane. Suppose a rigid body of an arbitrary shape is in pure rotational motion about the z-axis (see Figure 19(a)). Let us analyze the motion of a particle that lies in a slice of the body in the x-y plane as in Figure 19(b). This particle (at point P) will rotate in a circle of fixed radius r which represents the perpendicular distance from P to the axis of rotation. If you look at any other particle in the object you will see that every particle will rotate in its own circle that has the axis of rotation at its center. In other words, different particles move in different circles but the center of all of these circles lies on the rotational axis. Suppose the particle moves through an arc length s starting at the positive x-axis. Its angular position is then given byθ=s/rr and θ are the polar coordinates of a point in a plane where θ is always measured from the positive x-axis. Because θ is the ratio of the arc length to the radius, it is a pure (dimensionless) number.
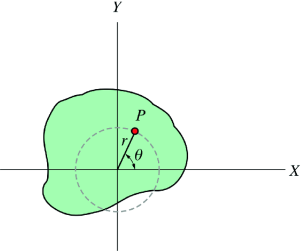
ω2∑mi(ri)2Clearly, I= ∑mi(ri)2This conclusion, thus, clearly establishes that the expression as given by ∑mi(ri)2 indeed represents the inertia of the rigid body in rotation. Q4) Explain the rotation in plane also derive the conditions of the instantaneous velocity vector of any particle is always perpendicular to its instantaneous position vector?A 4) When all parts of a rigid body move parallel to a fixed plane, then the motion of the object is referred to as plane motion. There are two types of plane motion, which are given as follows:The pure rotational motion: The rigid body in such a motion rotates about a fixed axis that is perpendicular to a fixed plane. In other words, the axis is fixed and does not move or change its direction relative to an inertial frame of reference. The general plane motion: The motion here can be considered as a combination of pure translational motion parallel to a fixed plane in addition to a pure rotational motion about an axis that is perpendicular to that plane. Suppose a rigid body of an arbitrary shape is in pure rotational motion about the z-axis (see Figure 19(a)). Let us analyze the motion of a particle that lies in a slice of the body in the x-y plane as in Figure 19(b). This particle (at point P) will rotate in a circle of fixed radius r which represents the perpendicular distance from P to the axis of rotation. If you look at any other particle in the object you will see that every particle will rotate in its own circle that has the axis of rotation at its center. In other words, different particles move in different circles but the center of all of these circles lies on the rotational axis. Suppose the particle moves through an arc length s starting at the positive x-axis. Its angular position is then given byθ=s/rr and θ are the polar coordinates of a point in a plane where θ is always measured from the positive x-axis. Because θ is the ratio of the arc length to the radius, it is a pure (dimensionless) number.
|
| ||
Figure 19(a): A rigid body of an arbitrary shape is in pure rotational motion about the z-axis
| Figure 19(b): The motion of a particle that lies in a slice of the body in the x-y plane
|
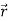 . Let b be its distance from the origin O. The rigidity condition requires that
. Let b be its distance from the origin O. The rigidity condition requires that 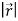 = bWhere b is constant.Squaring the above both sides we obtain
= bWhere b is constant.Squaring the above both sides we obtain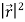 =
= 
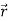 .
.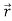 =
=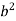 Differentiate both sides with respect to time to obtain
Differentiate both sides with respect to time to obtain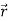 .
.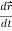 +
+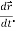
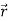 = 0
= 0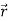 .
.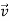 +
+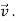
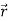 = 0
= 0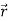 .
.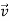 = 0This equation shown that whenever a rigid body rotates about a fixed point, the instantaneous velocity vector of any particle is always perpendicular to its instantaneous position vector. Q 5) Derive relation of angular momentum about a point of a rigid body in planar motion?A 5) Consider a rigid body which a rotating about a fixed point with an angular velocity
= 0This equation shown that whenever a rigid body rotates about a fixed point, the instantaneous velocity vector of any particle is always perpendicular to its instantaneous position vector. Q 5) Derive relation of angular momentum about a point of a rigid body in planar motion?A 5) Consider a rigid body which a rotating about a fixed point with an angular velocity 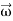 . Choose the origin O at the fixed point and axes as shown in Figure 21The velocity of particle i having position vector
. Choose the origin O at the fixed point and axes as shown in Figure 21The velocity of particle i having position vector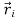 is
is 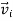 =
= 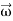
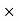
 …………(1)If mi is the mass of particle i then its angular momentum about the fixed point O is
…………(1)If mi is the mass of particle i then its angular momentum about the fixed point O is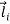 =
= 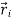
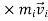 …………(2)Substituting the value of
…………(2)Substituting the value of 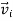 , it takes the form
, it takes the form  =
= 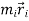

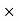
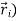 …………(3)
…………(3)
|
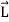 =
= 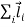
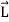 =
= 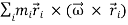 …………(4)Using the vector relation
…………(4)Using the vector relation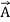
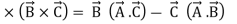 …………(5)Eq. (4) may be written as
…………(5)Eq. (4) may be written as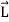 =
= 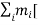
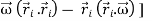
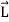 =
= 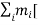
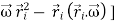 …………(6)Let (xi, yi, zi) be the cartesian coordinates of particle i, then
…………(6)Let (xi, yi, zi) be the cartesian coordinates of particle i, then 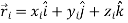 …………(7)If ωx, ωy and ωz are the components of angular velocity along coordinate axes then
…………(7)If ωx, ωy and ωz are the components of angular velocity along coordinate axes then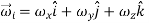 …………(8)Now take the dot product of
…………(8)Now take the dot product of 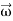 with
with 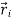 to obtain
to obtain  .
. 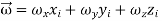 …………(9)Substitutes these values in eq. (6) to obtain
…………(9)Substitutes these values in eq. (6) to obtain
In this expansion it is useful to introduce the following abbreviations.
…………(11) These nine quantities Ixx, Ixy,….Izy are the components of the moments of intertia of the body about the fixed x,y,z axes. The components Ixx, Iyy and Izz are called the moments of intertia about x-axis, y-axis and z-axis respectively and the components Ixy,Iyz, …., Izy are called the products of intertia. A quantity such as the moment of intertia is called a tensor of second rank. In terms of the moments of intertia and products of intertia,
…………(12) This equation shows that the angular momentum vector Eq. (11) shows that
Thus the rotational behaviour of a rigid body about a given point it determined by a set of six quantities: the three moments of intertia and the three products of intertia. These moments of intertia and products of intertia will in general be function of time.
|
|
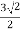 y=rsinθ=3sinπ/4=
y=rsinθ=3sinπ/4= 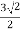 z=4Therefore point is (
z=4Therefore point is (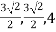 ) in rectangular coordinates.To go in the other direction (from rectangular coordinates to cylindrical), use the following set of formulas (again, the first two are exactly what we use to convert from rectangular to polar in R2:r=x2+y2θ=tan−1
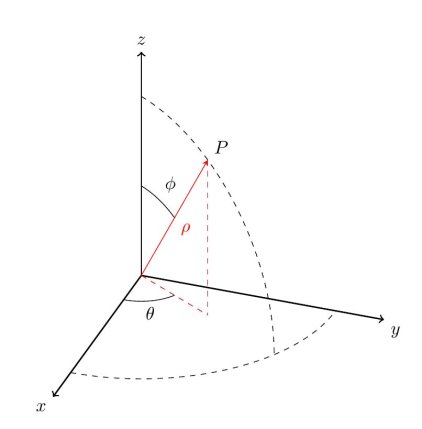
) in rectangular coordinates.To go in the other direction (from rectangular coordinates to cylindrical), use the following set of formulas (again, the first two are exactly what we use to convert from rectangular to polar in R2:r=x2+y2θ=tan−1 z=z Q 8) Plot the point with spherical coordinates (8, π/3, π/6) and express its location in both rectangular and cylindrical coordinates.A 8) Use the equations in Note to translate between spherical and cylindrical coordinates ρ=x2+y2+z2x=ρcosθsinϕ = 8sin(π/6)cos(π/3) = 8(1/2)1/2=2y=ρsinθsinϕ =8sin(π/6)sin(π/3)=8(1/2)
z=z Q 8) Plot the point with spherical coordinates (8, π/3, π/6) and express its location in both rectangular and cylindrical coordinates.A 8) Use the equations in Note to translate between spherical and cylindrical coordinates ρ=x2+y2+z2x=ρcosθsinϕ = 8sin(π/6)cos(π/3) = 8(1/2)1/2=2y=ρsinθsinϕ =8sin(π/6)sin(π/3)=8(1/2)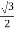 =2
=2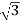 z=ρcosϕ =ρcosφ =8cos(π/6)= 8(
z=ρcosϕ =ρcosφ =8cos(π/6)= 8(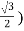 =4
=4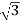 The point with spherical coordinates (8,π/3,π/6) has rectangular coordinates (2, 2
The point with spherical coordinates (8,π/3,π/6) has rectangular coordinates (2, 2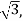 4
4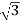 )Finding the values in cylindrical coordinates is equally straightforward:r=ρsinφ=8sinπ/6= 4θ= θz=ρcosφ=8cosπ/6= 4
)Finding the values in cylindrical coordinates is equally straightforward:r=ρsinφ=8sinπ/6= 4θ= θz=ρcosφ=8cosπ/6= 4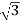 Thus, cylindrical coordinates for the point are (4,π/3, 4
Thus, cylindrical coordinates for the point are (4,π/3, 4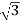 ).
).
|
|
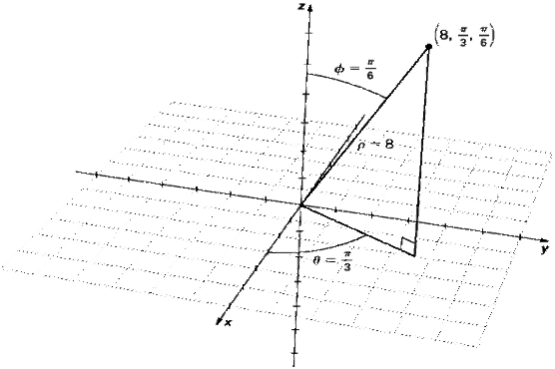
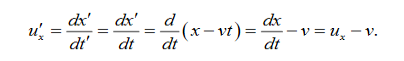 This is obvious anyway it’s just the addition of velocities formula.ux = u’x +v
This is obvious anyway it’s just the addition of velocities formula.ux = u’x +v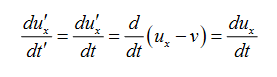 Since v is constant. That is to say, a’x =axThe acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.Q 11) Derive Lorentz Transformation equation?A 11)Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame. Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame. Expressing these relations in Cartesian coordinates givesx2+y2+z2−c2t2 =0 ………..(1)x′2+y′2+z′2−c2t′2=0 ………..(2)The left-hand sides Equations and can be set equal because both are zero. Because y=y′ and z=z′ so, we obtainx2−c2t2 =x′2−c2t′2 ………..(3)However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.To find the correct set of transformation equations, assume the two coordinate systems S and S′ The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified. Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis. The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as, x’ = Ax +Bt ………..(4)y’ = y ………..(5)z’ = z ………..(6)t’ = Cx +Dt ………..(7)
Since v is constant. That is to say, a’x =axThe acceleration is the same in both frames. This again is obvious as the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.Q 11) Derive Lorentz Transformation equation?A 11)Lorentz transformation is the relationship between two different coordinate frames that move at a constant velocity and are relative to each other. The name of the transformation comes from a Dutch physicist Hendrik Lorentz.The Galilean transformation nevertheless violates Einstein’s postulates, because the velocity equations state that a pulse of light moving with speed c along the x-axis would travel at speed c−v in the other inertial frame. Specifically, the spherical pulse has radius r=ct at time t in the unprimed frame, and also has radius r′=ct′ at time t' in the primed frame. Expressing these relations in Cartesian coordinates givesx2+y2+z2−c2t2 =0 ………..(1)x′2+y′2+z′2−c2t′2=0 ………..(2)The left-hand sides Equations and can be set equal because both are zero. Because y=y′ and z=z′ so, we obtainx2−c2t2 =x′2−c2t′2 ………..(3)However this cannot be satisfied for nonzero relative velocity v of the two frames if we assume the Galilean transformation results in t=t′ with x=x′+vt′.To find the correct set of transformation equations, assume the two coordinate systems S and S′ The laws of mechanics are invariant under Galilean transformations, whereas electrodynamics and Maxwell’s equations are varying under this transformation. This shows that the velocity of light will have different values for different observers moving with different uniform velocities. But the speed of light is invariant in all inertial frames of reference; hence, Galilean transformations need to be modified. Lorentz deduced the transformation equations, which are in agreement with the results of Michelson–Morley experiment. The Lorentz transformations are deduced as given below.Lorentz transformation equations are developed. Let x, y, z and t be the space and time coordinate in rest system or standard system. The system with x', y', z' moves with velocity 'v' along the x-axis. The origins at t=t'=0 coincide. The most general transformation equations relating the coordinates of an event in two systems can be written as, x’ = Ax +Bt ………..(4)y’ = y ………..(5)z’ = z ………..(6)t’ = Cx +Dt ………..(7)
|
The transformations are linear.If they are not linear one system would predict acceleration while in other system velocity was even constant. Because of symmetry, y' and z' axes are left unchanged by the transformation.Constant can be calculated by using the following boundary conditions.
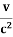 ………..(13)
………..(13)
|
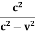 A =
A = 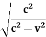 A =
A = 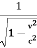 ………..(14)Substituting the Values of A, B, C, D in equation (1) and (4)So the Lorentz transformation equations would be, x’=
………..(14)Substituting the Values of A, B, C, D in equation (1) and (4)So the Lorentz transformation equations would be, x’= 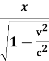 -
- 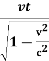 x’=
x’=  (x-vt)x’=
(x-vt)x’=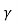 (x-vt)Where
(x-vt)Where 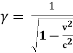 And t’ = -
And t’ = - 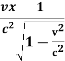 +
+ 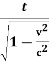 t’=
t’=  ( t -
( t -  )Lorentz transformation equations therefore arex’=
)Lorentz transformation equations therefore arex’=  (x - vt)y’ =yz’ = zt’=
(x - vt)y’ =yz’ = zt’=  ( t -
( t - 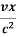 )Or these can be written asx’=
)Or these can be written asx’= 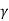 (x-vt)y’ =yz’ = zt’=
(x-vt)y’ =yz’ = zt’=  ( t -
( t - 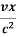 )Where
)Where 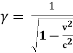 This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:x=
This set of equations, relating the position and time in the two inertial frames, is known as the Lorentz transformation. They are named in honour of H.A. Lorentz (1853–1928), who first proposed them. Interestingly, he justified the transformation on what was eventually discovered to be a fallacious hypothesis.The reverse transformation expresses by the following equations. Simply interchanging the primed and unprimed variables and substituting gives:x= 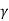 (x’+ vt)y =y’z = z’t=
(x’+ vt)y =y’z = z’t= 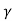 (t’ +
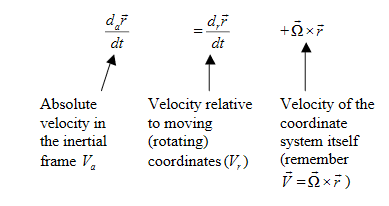
(t’ + 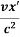 ) Q 12) Derive equation of motion and also explain Coriolis acceleration and Centripetal acceleration? A 12)Time derivatives in fixed and rotating coordinates
) Q 12) Derive equation of motion and also explain Coriolis acceleration and Centripetal acceleration? A 12)Time derivatives in fixed and rotating coordinates
|
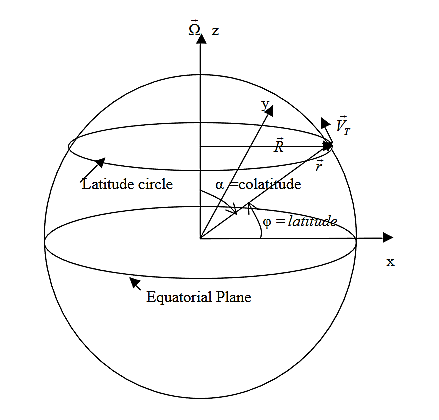
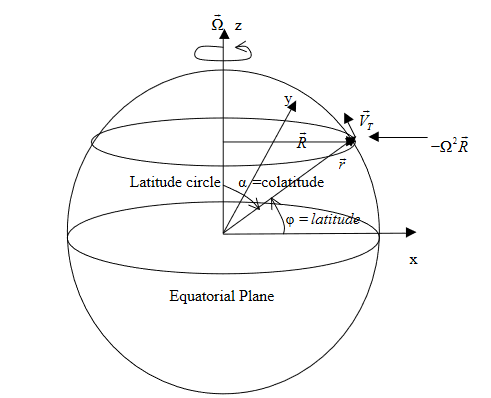
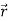 = position vector from the origin (center of the earth). Then, we can find out that the instantaneous tangential velocity of a particle at any distance
= position vector from the origin (center of the earth). Then, we can find out that the instantaneous tangential velocity of a particle at any distance 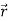 (
(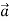 +
+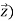 where a = mean radius of earth and z = altitude above ground) is given by
where a = mean radius of earth and z = altitude above ground) is given by ……….(1)Here
……….(1)Here 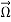 is the vector angular velocity of the earth rotation. We can see this by first looking at the magnitude of
is the vector angular velocity of the earth rotation. We can see this by first looking at the magnitude of 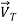 . According to the above figure,
. According to the above figure,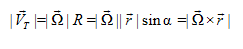 ……….(2)Now, let's try to relate
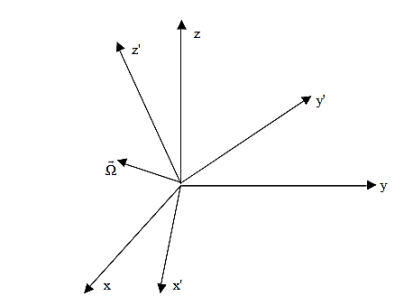
……….(2)Now, let's try to relate 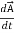 in 2 reference frames:a) Fixed (absolute) system (
in 2 reference frames:a) Fixed (absolute) system (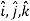 )b) Rotating (
)b) Rotating (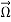 ) system (
) system (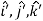 )
)
|
 is the same vector no matter what coordinate system it is viewed from. This
is the same vector no matter what coordinate system it is viewed from. This Given that the primed system is rotating, however, the time derivative of
Given that the primed system is rotating, however, the time derivative of 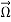 will be different if viewed from the 2 systems. Mathematically, we have'a' for absolute
will be different if viewed from the 2 systems. Mathematically, we have'a' for absolute
|
|
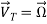
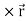 a particle rotating at angular velocity
a particle rotating at angular velocity 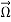 . Since
. Since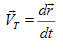 therefore
therefore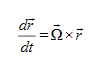 The above is correct only for a vector whose length does not change. The same is true for the unit vectors, therefore
The above is correct only for a vector whose length does not change. The same is true for the unit vectors, therefore
If we now look at (
which is a time rate of change in Thus, from (3), we can write
Let's look at a physical interpretation. If
In another word,
|
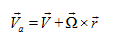 ……….(8)
……….(8)where Apply Eq.(6) to
Apply the Newton's second law
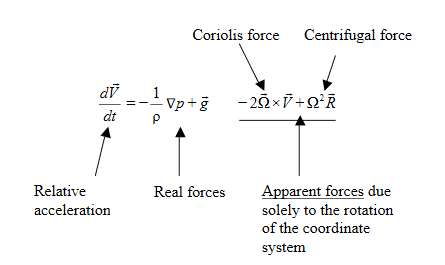
The part in the box is the equation of motion in the rotating coordinate system. It describes the change of (relative) velocity in time subjecting the net force. The forces on the right hand side are real forces, and the second and third term on the left arises because of the coordinate rotation, and there are apparent (not real) forces. We will discuss them in more details in the following. We will look at the last term one the left with respect to an earth oriented coordinate system:
Figure 11: Earth oriented coordinate system
Note that Look at the last term in (10)
We can write it as
Using this we can rewrite equation (10) as
Abs. Accel = Rel. Accel. + Coriolis accel + (-Centripetal accel ) = Net real force This is a very important equation. How do we make use of this equation? Let's look at the forces on the right hand side of (11) in the real atmosphere. They are Gravity = PGF (per unit mass) = - These are real forces (those seen and felt in an inertial / fixed reference frame), so let's put them into the equation:
this is the acceleration that we see and measure in our earth coordinate system – not an inertial frame, but the next 2 terms take that into account.
Rearranging (12)
Equation (13) is "THE EQUATION OF MOTION".
|